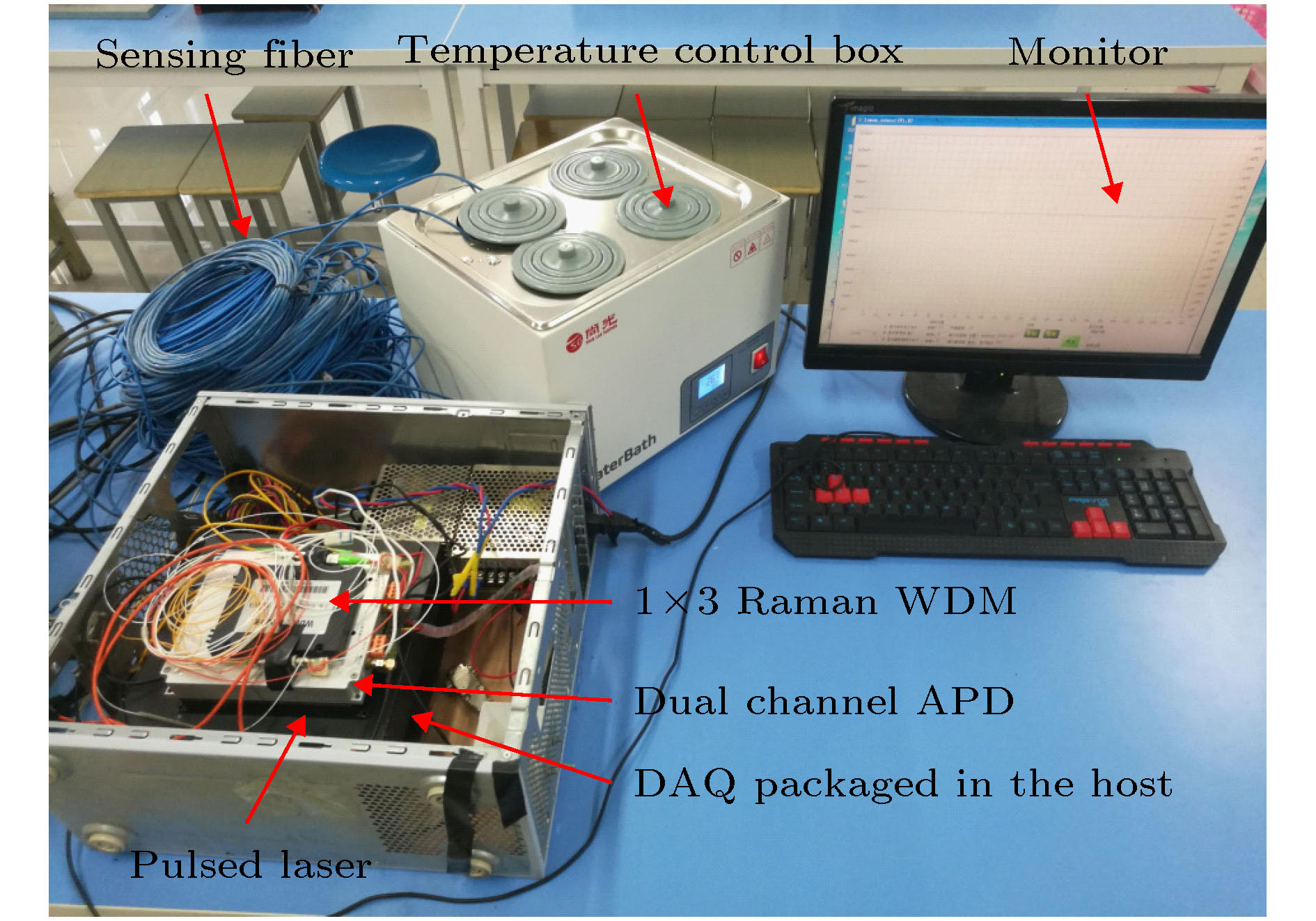
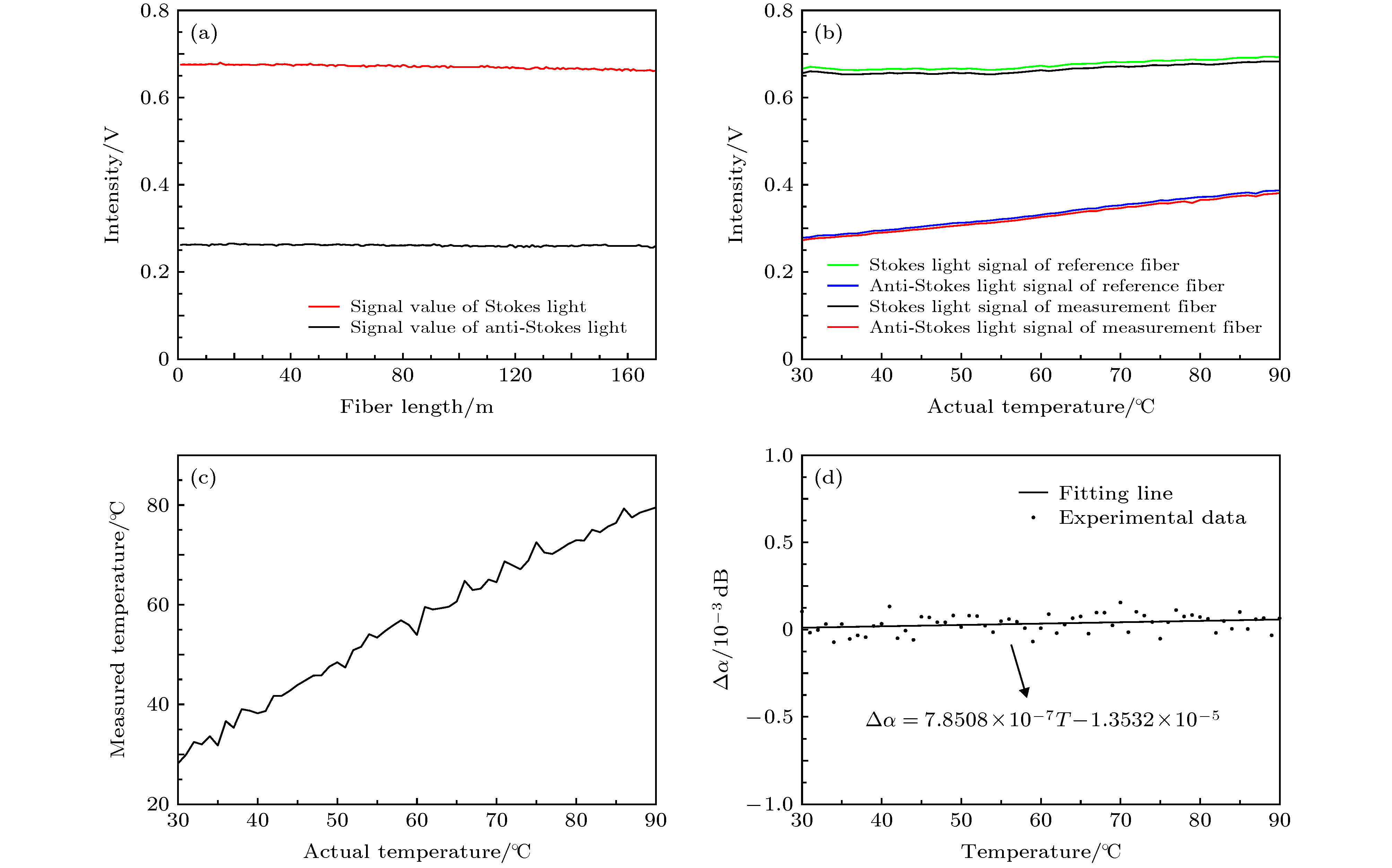
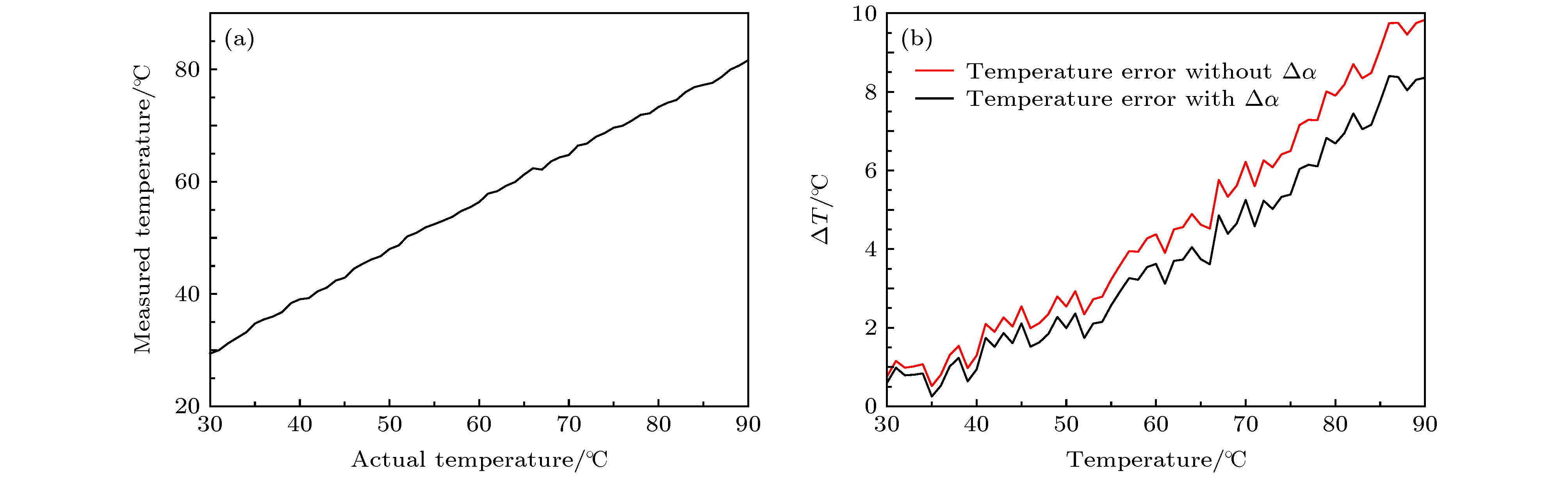
-
针对斯托克斯光和反斯托克斯光的本质损耗、附加损耗使分布式光纤温度传感器产生测温误差的问题, 通过对分布式光纤温度传感器的温度解调原理的研究, 提出了拟合斯托克斯光与反斯托克斯光之间衰减差的方法实现温度自补偿, 以此减小测温误差. 以传感光纤上不同位置的两部分作为参考段和测温段, 参考段的光信号作为测温段拟合多阶衰减差和解调温度的参量, 通过引入多阶拟合结果解调温度, 减小因斯托克斯光和反斯托克斯光的本质损耗、附加损耗导致的温度误差, 实现温度的初步修正. 改变光纤上同一位置的温度, 取3组不同温度值及对应信号值计算引入拟合衰减差前后的瑞利噪声, 分析了瑞利噪声与光纤长度和温度的关系, 通过引入拟合衰减差消除瑞利噪声, 减小了斯托克斯光和反斯托克斯光的本质损耗、附加损耗导致的瑞利噪声误差, 实现温度的再次修正. 分析比较多阶衰减差拟合结果对测温误差以及消除瑞利噪声的影响, 获得最优拟合阶次. 在拟合因参考段的附加损耗而导致的测温段的附加误差后, 通过拟合结果进行温度补偿, 完成了最终温度修正. 实验结果表明, 在30—90 ℃, 引入一阶线性拟合结果的温度修正效果最好, 经过三次修正后, 测温误差从10.50 ℃降低至0.90 ℃.The temperature error caused by the essential loss and the additional loss of Stokes light and anti-Stokes light widely exist in the distributed optical fiber temperature sensor (DTS). According to the temperature demodulation principle of the DTS, a method of fitting the attenuation difference between Stokes light and anti-Stokes light is proposed, which can realize the temperature self-compensation to reduce the temperature measurement error. Two parts at the different positions of the sensing fiber are regarded as the reference section and the temperature measuring section, respectively. The optical signal of the reference section is used as a parameter when demodulating the temperature and fitting the attenuation difference, and the attenuation difference between the Stokes light and the anti-Stokes light is multi-order fitted by the optical signal of the temperature measuring section, then the multi-order fitting results are used to demodulate the temperature for reducing the temperature error caused by the essential loss and additional loss of the Stokes light and anti-Stokes light, in order to implement the preliminary correction of the temperature. Three groups of the different measuring temperature values at the same position of the optical fiber as well as their corresponding signal values are taken in calculation for eliminating the Rayleigh noise, and the relationship of Rayleigh noise with fiber length and temperature are analyzed, and thus further calculating the Rayleigh noise based on the fitting attenuation difference. The influence of the multi-order attenuation difference on the error in temperature measurement and that on the elimination of the Rayleigh noise are compared with each other, and the Rayleigh noise error caused by the essential loss and additional loss of the Stokes light and anti-Stokes light are reduced, then the temperature is corrected again by eliminating the Rayleigh noise. The effect of the multi-order attenuation difference fitting result on the temperature measurement error and on the elimination of Rayleigh noise are analyzed and compared with each other, then the optimal fitting order is obtained. After fitting the additional error at the temperature measurement section that is caused by the additional loss at the reference section, the temperature compensation is carried out by the fitting result, then the final temperature correction is completed. The experimental results show that the temperature correction effect is best by using the first-order linear fitting results in a temperature range of 30－90 ℃, and the temperature measurement error is reduced from 10.50 ℃ to 0.90 ℃ after being corrected three times.
-
Keywords:
- temperature /
- distributed fiber /
- attenuation difference /
- self-compensation
[1] Geng J S, Sun Q, Zhang Y C, Gong W Y, Du S 2018 J. Loss Prevent. Proc. 55 144
Google Scholar
[2] O'Keefe J M K, Neace E R, Hammond M L, Hower J C, Engle M A, East J, Geboy N J, Olea R A, Henke K R, Copley G C, Lemley E, Nally R S H, Hansen A E, Richardson A R, Satterwhite A B, Stracher G B, Radke L F, Smeltzer C, Romanek C, Blake D R, Schroeder P A, Emsbo-Mattingly S D, Stout S A 2018 Int. J. Coal Geol. 195 304
Google Scholar
[3] Dunnington L, Nakagawa M 2017 Environ. Pollut. 229 139
Google Scholar
[4] Mohalik N K, Lester E, Lowndes I S, Singh V K 2016 Carbon Manag. 7 317
Google Scholar
[5] Yan W J, Hu M, Liang J R, Wang D F, Wei Y L, Qin Y X 2016 Chin. Phys. B 25 040702
Google Scholar
[6] Wang Z L, Zhang S S, Chang J, Lü G P, Wang W J, Jiang S, Liu X Z, Liu X H, Luo S, Liu Y N 2014 Optik 125 1821
Google Scholar
[7] 刘铁根, 于哲, 江俊峰, 刘琨, 张学智, 丁振扬, 王双, 胡浩丰, 韩群, 张红霞, 李志宏 2017 66 070705
Google Scholar
Liu T G, Yu Z, Jiang J F, Liu K, Zhang X Z, Ding Z Y, Wang S, Hu H F, Han Q, Zhang H X, Li Z H 2017 Acta Phys. Sin. 66 070705
Google Scholar
[8] 孙琪真, 刘德明, 王健 2007 56 5903
Google Scholar
Sun Q Z, Liu D M, Wang J 2007 Acta Phys. Sin. 56 5903
Google Scholar
[9] 饶云江 2017 66 074207
Google Scholar
Rao Y J 2017 Acta Phys. Sin. 66 074207
Google Scholar
[10] Wen S Z, Xiong W C, Huang L P, Wang Z R, Zhang X B, He Z H 2018 Chin. Phys. B 27 090701
Google Scholar
[11] Bolognini G, Hartog A 2013 Opt. Fiber Technol. 19 678
Google Scholar
[12] 王剑锋, 刘红林, 张淑琴, 余向东, 孙忠周, 金尚忠, 张在宣 2013 光谱学与光谱分析 33 865
Google Scholar
Wang J F, Liu H L, Zhang S Q, Yu X D, Sun Z Z, Jin S Z, Zhang Z X 2013 Spectrosc. Spect. Anal. 33 865
Google Scholar
[13] 余向东, 张在宣, 祝海忠, 金尚忠, 刘红林, 王剑锋 2011 光子学报 40 1870
Google Scholar
Yu X D, Zhang Z X, Zhu H Z, Jin S Z, Liu H L, Wang J F 2011 Acta. Photon. Sin. 40 1870
Google Scholar
[14] He H L, Dyck M F, Horton R, Li M, Jin H J, Si B C 2018 Adv. Agron. 148 173
Google Scholar
[15] Chai Q, Luo Y, Ren J, Zhang J Z, Yang J, Yuan L B, Peng G D 2019 Opt. Eng. 58 072007
[16] 孙苗, 汤玉泉, 杨爽, 李俊, Culshaw B, 董凤忠 2015 光电子·激光 26 2070
Sun M, Tang Y Q, Yang S, Li J, Culshaw B, Dong F Z 2015 J. Optoelectron. Laser 26 2070
[17] Cao Y L, Yang F, Xu D, Ye Q, Cai H W, Fang Z J 2016 Chin. Phys. Lett. 33 050701
Google Scholar
[18] Yang C, Wang M, Tang M, Wu H, Zhao C, Liu T Q, Fu S N, Tong W J 2018 Appl. Opt. 57 6923
Google Scholar
[19] Shang C, Wu C Q, Li Z Y, Yang S S 2011 Chin. Phys. Lett. 28 094212
Google Scholar
[20] Liu Y P, Ma L, Yang C, Tong W J, He Z Y 2018 Opt. Express 26 20562
Google Scholar
[21] Hwang D, Yoon D J, Kwon I B, Seo D C, Chung Y J 2010 Opt. Express 18 9747
Google Scholar
[22] Suh K, Lee C 2008 Opt. Lett. 33 1845
Google Scholar
[23] Wang Z L, Zhang S S, Chang J, Lü P G, Wang W J, Jiang S, Liu X Z, Liu X H, Luo S, Sun B N, Liu Y N 2013 Opt. Quant. Electron. 45 1087
Google Scholar
[24] van de Giesen N, Steele-Dunne S C, Jansen J, Hoes O, Hausner M B, Tyler S, Selker J 2012 Sensors 12 5471
Google Scholar
[25] 汤玉泉, 孙苗, 李俊, 杨爽, Culshaw B, 董凤忠 2015 光子学报 44 0506006
Google Scholar
Tang Y Q, Sun M, Li J, Yang S, Culshaw B, Dong F Z 2015 Acta. Photon. Sin. 44 0506006
Google Scholar
[26] Sun B N, Chang J, Lian J, Wang Z L, Lü G P, Liu X Z, Wang W J, Zhou S, Wei W, Jiang S, Liu Y N, Luo S, Liu X H, Liu Z, ZhangS S 2013 Opt. Commun. 306 117
Google Scholar
[27] Yin Y X, Wu Z F, Sun S W, Tian L, Wang X B, Wu Y D, Zhang D M 2019 Chin. Phys. B 28 074202
Google Scholar
[28] 汤玉泉, 孙苗, 李俊, 杨爽, Culshaw B, 董凤忠 2015 光电子·激光 26 847
Tang Y Q, Sun M, Li J, Yang S, Culshaw B, Dong F Z 2015 J. Optoelectron. Laser 26 847
[29] Wang Z L, Chang J, Zhang S S, Luo S, Jia C W, Jiang S, Sun B N, Liu Y N, Wei W, Liu X H, Lü G P 2015 Optik 126 270
Google Scholar
[30] 柴敬 2003 博士学位论文 (西安: 西安科技大学)
Chai J 2003 Ph. D. Dissertation (Xi’an: Xi’an University of Science and Technology) (in Chinese)
[31] Lin Q, Yaman F, Agrawal G P 2007 Phys. Rev. A 75 023803
Google Scholar
[32] Wang Z L, Chang J, Zhang S S, Sun B N, Jiang S, Luo S, Jia C W, Liu Y N, Liu X H, Lü G P, Liu X Z 2014 Opt. Quant. Electron. 46 821
Google Scholar
-
图 3 实验结果 (a) 20 ℃时光纤中的散射光信号; (b) 温度变化时的散射光信号; (c) Δα ≈ 0时的温度解调结果; (d)衰减差拟合结果
Fig. 3. Experimental results: (a) Scattered light signal in fiber at 20 ℃; (b) scattered light signal when temperature changes; (c) temperature demodulation results of Δα
≈ 0; (d) fitting results of attenuation difference. 
图 4 温度修正后的测量结果 (a)初步修正后的测量值; (b)温度修正前后的测温误差
Fig. 4. Temperature corrected measurement: (a) Preliminary corrected measurement; (b) temperature measurement error before and after temperature correction.
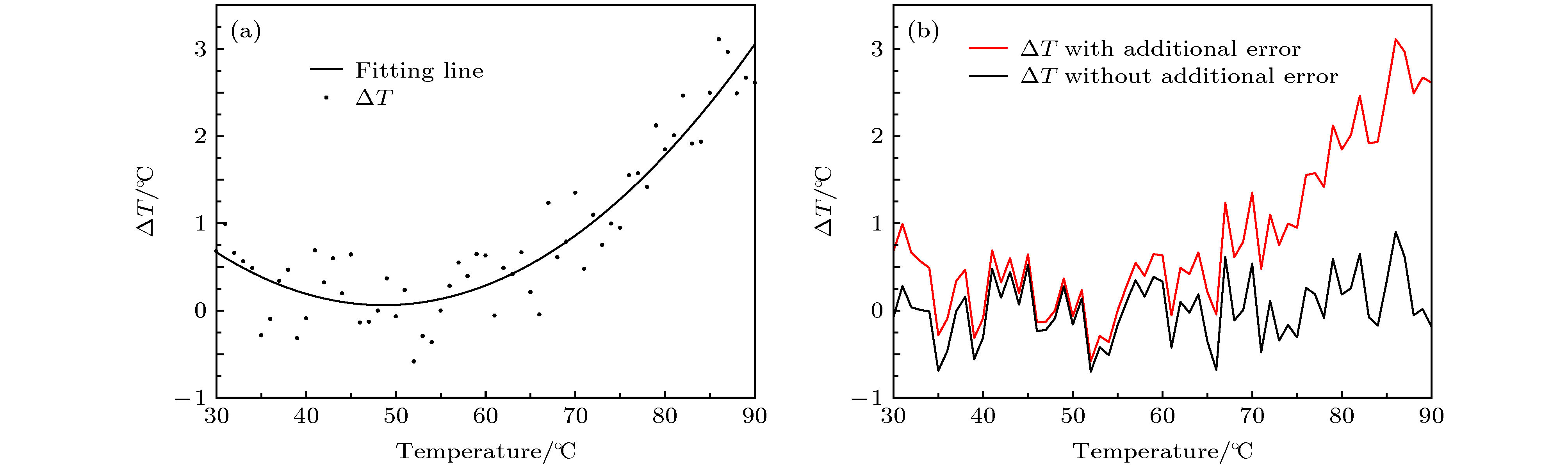
图 5 温度最终修正后的测量结果 (a) 40 ℃和60 ℃时光纤中的瑞利噪声; (b)不同温度下的瑞利噪声; (c)引入Δα前后消除瑞利噪声的测量结果; (d)引入Δα前后消除瑞利噪声的温度误差
Fig. 5. Temperature corrected final measurement results: (a) Rayleigh noise in fiber at 40 ℃ and 60 ℃; (b) rayleigh noise at different temperatures; (c) measurement results without Rayleigh noise before and after the introduction of Δα; (d) temperature error without Rayleigh noise before and after the introduction of Δα.
图 6 各阶修正效果 (a)引入各阶拟合结果二次修正后的误差; (b)引入各阶结果后二次修正的温度增量
Fig. 6. Temperature error after each order fitting: (a) The second correction error after introducing the fitting results of each order; (b) temperature increment for secondary correction after introduction of each order result.
图 7 附加误差修正 (a)附加误差拟合曲线; (b)附加误差修正前后的测温误差
Fig. 7. Additional error correction: (a) Additional error fitting result; (b) temperature error before and after additional error correction.
-
[1] Geng J S, Sun Q, Zhang Y C, Gong W Y, Du S 2018 J. Loss Prevent. Proc. 55 144
Google Scholar
[2] O'Keefe J M K, Neace E R, Hammond M L, Hower J C, Engle M A, East J, Geboy N J, Olea R A, Henke K R, Copley G C, Lemley E, Nally R S H, Hansen A E, Richardson A R, Satterwhite A B, Stracher G B, Radke L F, Smeltzer C, Romanek C, Blake D R, Schroeder P A, Emsbo-Mattingly S D, Stout S A 2018 Int. J. Coal Geol. 195 304
Google Scholar
[3] Dunnington L, Nakagawa M 2017 Environ. Pollut. 229 139
Google Scholar
[4] Mohalik N K, Lester E, Lowndes I S, Singh V K 2016 Carbon Manag. 7 317
Google Scholar
[5] Yan W J, Hu M, Liang J R, Wang D F, Wei Y L, Qin Y X 2016 Chin. Phys. B 25 040702
Google Scholar
[6] Wang Z L, Zhang S S, Chang J, Lü G P, Wang W J, Jiang S, Liu X Z, Liu X H, Luo S, Liu Y N 2014 Optik 125 1821
Google Scholar
[7] 刘铁根, 于哲, 江俊峰, 刘琨, 张学智, 丁振扬, 王双, 胡浩丰, 韩群, 张红霞, 李志宏 2017 66 070705
Google Scholar
Liu T G, Yu Z, Jiang J F, Liu K, Zhang X Z, Ding Z Y, Wang S, Hu H F, Han Q, Zhang H X, Li Z H 2017 Acta Phys. Sin. 66 070705
Google Scholar
[8] 孙琪真, 刘德明, 王健 2007 56 5903
Google Scholar
Sun Q Z, Liu D M, Wang J 2007 Acta Phys. Sin. 56 5903
Google Scholar
[9] 饶云江 2017 66 074207
Google Scholar
Rao Y J 2017 Acta Phys. Sin. 66 074207
Google Scholar
[10] Wen S Z, Xiong W C, Huang L P, Wang Z R, Zhang X B, He Z H 2018 Chin. Phys. B 27 090701
Google Scholar
[11] Bolognini G, Hartog A 2013 Opt. Fiber Technol. 19 678
Google Scholar
[12] 王剑锋, 刘红林, 张淑琴, 余向东, 孙忠周, 金尚忠, 张在宣 2013 光谱学与光谱分析 33 865
Google Scholar
Wang J F, Liu H L, Zhang S Q, Yu X D, Sun Z Z, Jin S Z, Zhang Z X 2013 Spectrosc. Spect. Anal. 33 865
Google Scholar
[13] 余向东, 张在宣, 祝海忠, 金尚忠, 刘红林, 王剑锋 2011 光子学报 40 1870
Google Scholar
Yu X D, Zhang Z X, Zhu H Z, Jin S Z, Liu H L, Wang J F 2011 Acta. Photon. Sin. 40 1870
Google Scholar
[14] He H L, Dyck M F, Horton R, Li M, Jin H J, Si B C 2018 Adv. Agron. 148 173
Google Scholar
[15] Chai Q, Luo Y, Ren J, Zhang J Z, Yang J, Yuan L B, Peng G D 2019 Opt. Eng. 58 072007
[16] 孙苗, 汤玉泉, 杨爽, 李俊, Culshaw B, 董凤忠 2015 光电子·激光 26 2070
Sun M, Tang Y Q, Yang S, Li J, Culshaw B, Dong F Z 2015 J. Optoelectron. Laser 26 2070
[17] Cao Y L, Yang F, Xu D, Ye Q, Cai H W, Fang Z J 2016 Chin. Phys. Lett. 33 050701
Google Scholar
[18] Yang C, Wang M, Tang M, Wu H, Zhao C, Liu T Q, Fu S N, Tong W J 2018 Appl. Opt. 57 6923
Google Scholar
[19] Shang C, Wu C Q, Li Z Y, Yang S S 2011 Chin. Phys. Lett. 28 094212
Google Scholar
[20] Liu Y P, Ma L, Yang C, Tong W J, He Z Y 2018 Opt. Express 26 20562
Google Scholar
[21] Hwang D, Yoon D J, Kwon I B, Seo D C, Chung Y J 2010 Opt. Express 18 9747
Google Scholar
[22] Suh K, Lee C 2008 Opt. Lett. 33 1845
Google Scholar
[23] Wang Z L, Zhang S S, Chang J, Lü P G, Wang W J, Jiang S, Liu X Z, Liu X H, Luo S, Sun B N, Liu Y N 2013 Opt. Quant. Electron. 45 1087
Google Scholar
[24] van de Giesen N, Steele-Dunne S C, Jansen J, Hoes O, Hausner M B, Tyler S, Selker J 2012 Sensors 12 5471
Google Scholar
[25] 汤玉泉, 孙苗, 李俊, 杨爽, Culshaw B, 董凤忠 2015 光子学报 44 0506006
Google Scholar
Tang Y Q, Sun M, Li J, Yang S, Culshaw B, Dong F Z 2015 Acta. Photon. Sin. 44 0506006
Google Scholar
[26] Sun B N, Chang J, Lian J, Wang Z L, Lü G P, Liu X Z, Wang W J, Zhou S, Wei W, Jiang S, Liu Y N, Luo S, Liu X H, Liu Z, ZhangS S 2013 Opt. Commun. 306 117
Google Scholar
[27] Yin Y X, Wu Z F, Sun S W, Tian L, Wang X B, Wu Y D, Zhang D M 2019 Chin. Phys. B 28 074202
Google Scholar
[28] 汤玉泉, 孙苗, 李俊, 杨爽, Culshaw B, 董凤忠 2015 光电子·激光 26 847
Tang Y Q, Sun M, Li J, Yang S, Culshaw B, Dong F Z 2015 J. Optoelectron. Laser 26 847
[29] Wang Z L, Chang J, Zhang S S, Luo S, Jia C W, Jiang S, Sun B N, Liu Y N, Wei W, Liu X H, Lü G P 2015 Optik 126 270
Google Scholar
[30] 柴敬 2003 博士学位论文 (西安: 西安科技大学)
Chai J 2003 Ph. D. Dissertation (Xi’an: Xi’an University of Science and Technology) (in Chinese)
[31] Lin Q, Yaman F, Agrawal G P 2007 Phys. Rev. A 75 023803
Google Scholar
[32] Wang Z L, Chang J, Zhang S S, Sun B N, Jiang S, Luo S, Jia C W, Liu Y N, Liu X H, Lü G P, Liu X Z 2014 Opt. Quant. Electron. 46 821
Google Scholar
下载:
计量
- 文章访问数: 8069
- PDF下载量: 116
- 被引次数: 0
