钡和铯释放的电离层扰动效应对比

朱肖丽; 胡耀垓; 赵正予; 张援农

doi:10.7498/aps.69.20191266

摘要

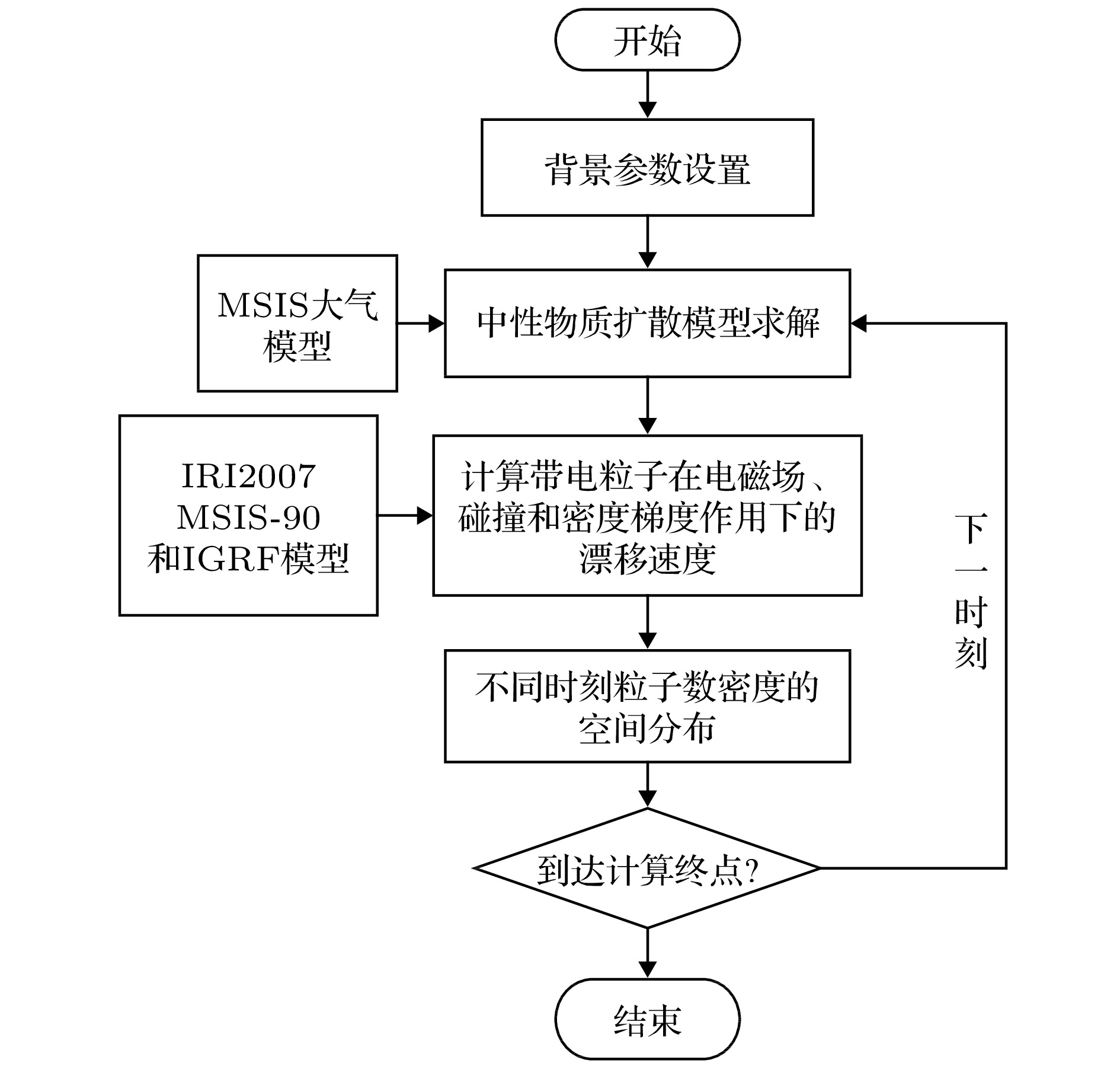
碱金属或碱土金属在电离层释放后, 迅速在太阳辐射作用下发生光电离, 产生正离子和电子, 形成人工等离子体云团. 本文基于三维双成分流体模型, 考虑释放区域水平风场的影响, 探讨了钡和铯在电离层释放后的时空演化规律, 并对钡和铯的电离层扰动效应进行了对比. 模拟结果表明, 不考虑中性风场时, 生成的等离子体云团逐渐沿磁场被拉伸成椭球形结构, 同时, 膨胀的等离子体云会推开背景氧离子, 在释放中心形成氧离子密度空洞, 并在两侧产生两个对称的密度尖峰; 水平风场的存在会使得生成的离子云逆风侧的密度梯度变陡, 释放物质对背景氧离子的扰动也更大; 对比钡与铯的释放结果发现, 由于铯的扩散系数较小, 钡云的膨胀更为迅速, Ba⁺云团的覆盖区域更广; 而由于光电离率较大, 释放相同质量下铯的离子产率更高; 此外, Cs⁺的扫雪机效应比Ba⁺扫雪机更强, 氧离子密度空穴和凸起处的扰动也更大.

关键词:

Abstract

After being released in the ionosphere, alkali-metal atoms will be rapidly photoionized by solar UV, producing positive ions and electrons, and forming artificial plasma cloud. Based on a three-dimensional two-species fluid model, considering both the loss of barium atoms due to photoionization and oxidation and the influence of horizontal wind field in the release region, the spatial-temporal evolution of the artificial plasma cloud is discussed. By taking into account the electromagnetic field force, pressure gradient, particle collisions and ion inertia, the ionospheric disturbance effects caused by barium and cesium are compared with each other. The simulation results show that the alkali metal rapidly expands after being released in the ionosphere, and the generated plasma cloud gradually forms an ellipsoidal structure from the inside to the outside under the constraint of magnetic field with considering no wind. Meanwhile, the expanded plasma cloud pushes away the background oxygen ions, forming an oxygen ion density hole in the release center and two symmetrical density bumps on both sides. In the absence of neutral wind, the plasma cloud is dominated by the movement along magnetic field, while considering the background neutral wind, the plasma cloud and background disturbance area will move along the direction of wind, so that the density gradient of plasma cloud becomes steepening on the upwind side. Although the movement of ion cloud across the magnetic field is constrained, the neutrals can pass through the magnetic field freely, so the ion cloud and neutral cloud will separate from each other slowly. Also, the presence of horizontal wind field will make a greater disturbance to the background oxygen ion. By comparing the simulation results of barium and cesium we can see that, qualitatively, the expansion characteristics of Cs⁺ and Ba⁺ as well as their effects on the background O⁺ are similar. Due to the small diffusion coefficient of cesium, the barium cloud expands more rapidly and the coverage area of Ba⁺ cloud is wider. Because of the large photoionization rate of cesium, the ionization yield of cesium is higher than that of barium when the same mass is released. In addition, the snowplow effect of Cs⁺ is stronger than that of Ba⁺, and the oxygen ion density holes and bumps caused by Cs⁺ are also larger.

Keywords:

作者及机构信息

武汉大学电子信息学院, 电离层实验室, 武汉　430079

通信作者: 胡耀垓, yaogaihu@whu.edu.cn

Authors and contacts

Ionosphere Laboratory, School of Electronics and Information, Wuhan University, Wuhan 430079, China

Corresponding author: Hu Yao-Gai, yaogaihu@whu.edu.cn

文章全文 : translate this paragraph

参考文献

[1]	Foppl H, Haerendel G, Haser L, Lutjens P, Lust R, Melzner F, Meyer B, Neuss H, Rieger E 1967 Planet. Space Sci. 15 357 Google Scholar
[2]	Haerendel G, Foppl H, Melzner F, Neuss H, Rieger E, Stocker J, Bauer O, Hofner H, Loidl J 1986 Nature 320 700 Google Scholar
[3]	Caton R G, Pedersen T R, Groves K M, et al. 2017 Radio Sci. 52 539 Google Scholar
[4]	Huba J D, Bernhardt P A, Lyon J G 1992 J. Geophys. Res. Space Phys. 97 11 Google Scholar
[5]	Lloyd K H, Haerendel G 1973 J. Geophys. Res. 78 7389 Google Scholar
[6]	Morse D L, Destler W W 1973 J. Geophys. Res. 78 7417 Google Scholar
[7]	Bernhardt P A, Roussel-Dupre R A, Pongratz M B, et al. 1987 J. Geophys. Res. 92 5777 Google Scholar
[8]	Zakharov Y P 2002 Adv. Space Res. 29 1335 Google Scholar
[9]	Haerendel G, Lust R, Rieger E 1967 Planet. Space Sci. 15 1 Google Scholar
[10]	Schunk R W, Szuszczewicz E P 1988 J. Geophys. Res. Space Phys. 93 12901 Google Scholar
[11]	Mitchell H G, Fedder J A, Huba J D, Zalesak S T 1985 J. Geophys. Res. Space Phys. 90 11091 Google Scholar
[12]	Rozhansky V A, Veselova I Y, Voskoboynikov S P 1990 Planet. Space Sci. 38 1375 Google Scholar
[13]	Drake J F, Mulbrandon M, Huba J D 1988 Phys. Fluids 31 3412 Google Scholar
[14]	Ma T Z, Schunk R W 1991 J. Geophys. Res. Space Phys. 96 5793 Google Scholar
[15]	Ma T Z, Schunk R W 1994 J. Geophys. Res. 99 6331 Google Scholar
[16]	Mendillo M, Hawkins G S, Klobuchar J A 1975 Science 18734 3
[17]	Klobuchar J A, Abdu M A 1989 J. Geophys. Res. Space Phys. 94 2721 Google Scholar
[18]	Choueiri E Y, Oraevsky V N, Dokukin V S 2001 J. Geophys. Res. 106 25673 Google Scholar
[19]	Bernhardt P A 1979 J. Geophys. Res. 84 793 Google Scholar
[20]	Mendillo M, Semeter J, Noto J 1993 Adv. Space Res. 13 55
[21]	Scales W A, Bernhardt P A, Ganguli G 1994 J. Geophys. Res. 99 373 Google Scholar
[22]	Kolomiitsev O P, Ruzhin Y Y, Egorov I B, Razinkov O G, Cherkashin Y N 1999 Phys. Chem. Earth Part C 24 393 Google Scholar
[23]	黄文耿, 古士芬 2005 空间科学学报 25 254 Google Scholar Huang W G, Gu S F 2005 Chin. J. Space Sci. 25 254 Google Scholar
[24]	黄勇, 时家明, 袁忠才 2011 地球 54 1 Google Scholar Huang Y, Shi J M, Yuan Z C 2011 Chin J. Geophys. 54 1 Google Scholar
[25]	胡耀垓, 赵正予, 张援农 2010 59 8293 Google Scholar Hu Y G, Zhao Z Y, Zhang Y N 2010 Acta Phys. Sin. 59 8293 Google Scholar
[26]	胡耀垓, 赵正予, 张援农 2013 62 209401 Google Scholar Hu Y G, Zhao Z Y, Zhang Y N 2013 Acta Phys. Sin. 62 209401 Google Scholar
[27]	汪四成, 方涵先, 杨升高, 翁利斌 2012 地球物理学研究进展 27 2464 Wang S C, Fang H X, Yang S G 2012 Progress in Geophys. 27 2464
[28]	赵海生, 许正文, 吴振森, 冯杰, 吴健, 徐彬, 徐彤, 胡艳莉 2016 65 209401 Google Scholar Zhao H S, Xu Z W, Wu Z S, Feng J, Wu J, Xu B, Xu T, Hu Y L 2016 Acta Phys. Sin. 65 209401 Google Scholar
[29]	Li L, Xu R L 2002 Chin. Phys. Lett. 19 1214 Google Scholar
[30]	胡耀垓, 赵正予, 张援农 2012 61 089401 Google Scholar Hu Y G, Zhao Z Y, Zhang Y N 2012 Acta Phys. Sin. 61 089401 Google Scholar
[31]	谢良海 2013 博士学位论文(北京: 中国科学院大学) Xie L H 2013 Ph. D. Dissertation (Beijing: University of Chinese Academy of Sciences) (in Chinese)
[32]	Scholer, M 1970 Planet. Space Sci. 18 977 Google Scholar
[33]	Pressman J, Marrmo F F, Aschenbrand L M 1960 Planet. Space Sci. 2 228 Google Scholar
[34]	Holmgren G, Kintner P M, Kelley M C 1981 Adv. Space Res. 1 311 Google Scholar
[35]	Eliason L, Lundin R, Holmgren G 1988 Adv. Space Res. 8 93
[36]	Bleecker D K, Bogaerts A, Gijbels R, Goedheer W 2004 Phys. Rev. E 69 056409 Google Scholar
[37]	Xing Z, Anbang S, Le T, Guan J Z 2019 AIP Adv. 9 015117 Google Scholar

施引文献

图 1 仿真算法流程图

Fig. 1. Flow chart of simulation algorithm.

下载: 全尺寸图片幻灯片

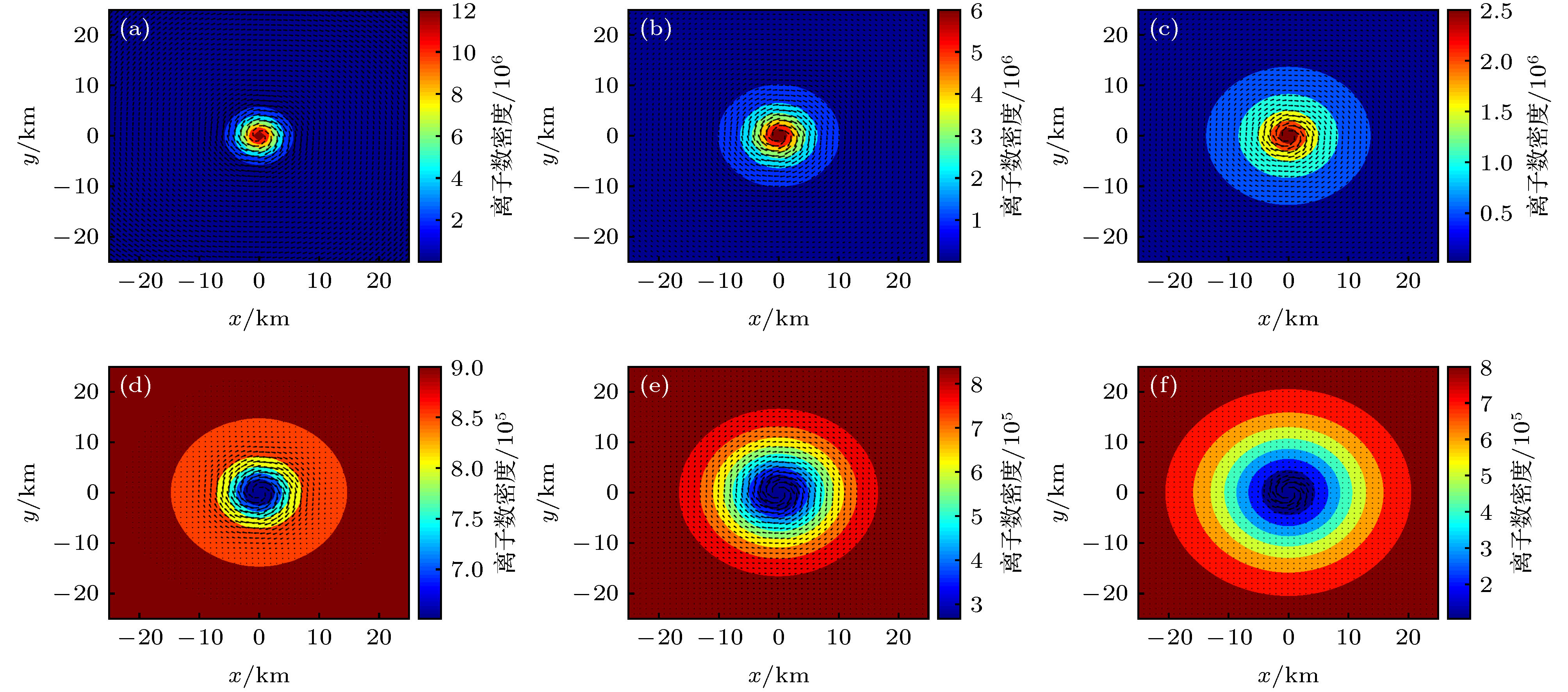
图 2 无中性风场时, 300 km高度释放10 kg钡后钡离子和氧离子的离子数密度分布(x-y平面)　(a) Ba⁺, t = 5 s; (b) Ba⁺, t = 30 s; (c) Ba⁺, t = 200 s; (d) O⁺, t = 5 s; (e) O⁺, t = 30 s; (f) O⁺, t = 200 s

Fig. 2. Density distribution of Ba⁺ and O⁺ (in x-y plane) after 10 kg barium released at 300 km while no neutral wind is considered: (a) Ba⁺, t = 5 s; (b) Ba⁺, t = 30 s; (c) Ba⁺, t = 200 s; (d) O⁺, t = 5 s; (e) O⁺, t = 30 s; (f) O⁺, t = 200 s.

下载: 全尺寸图片幻灯片

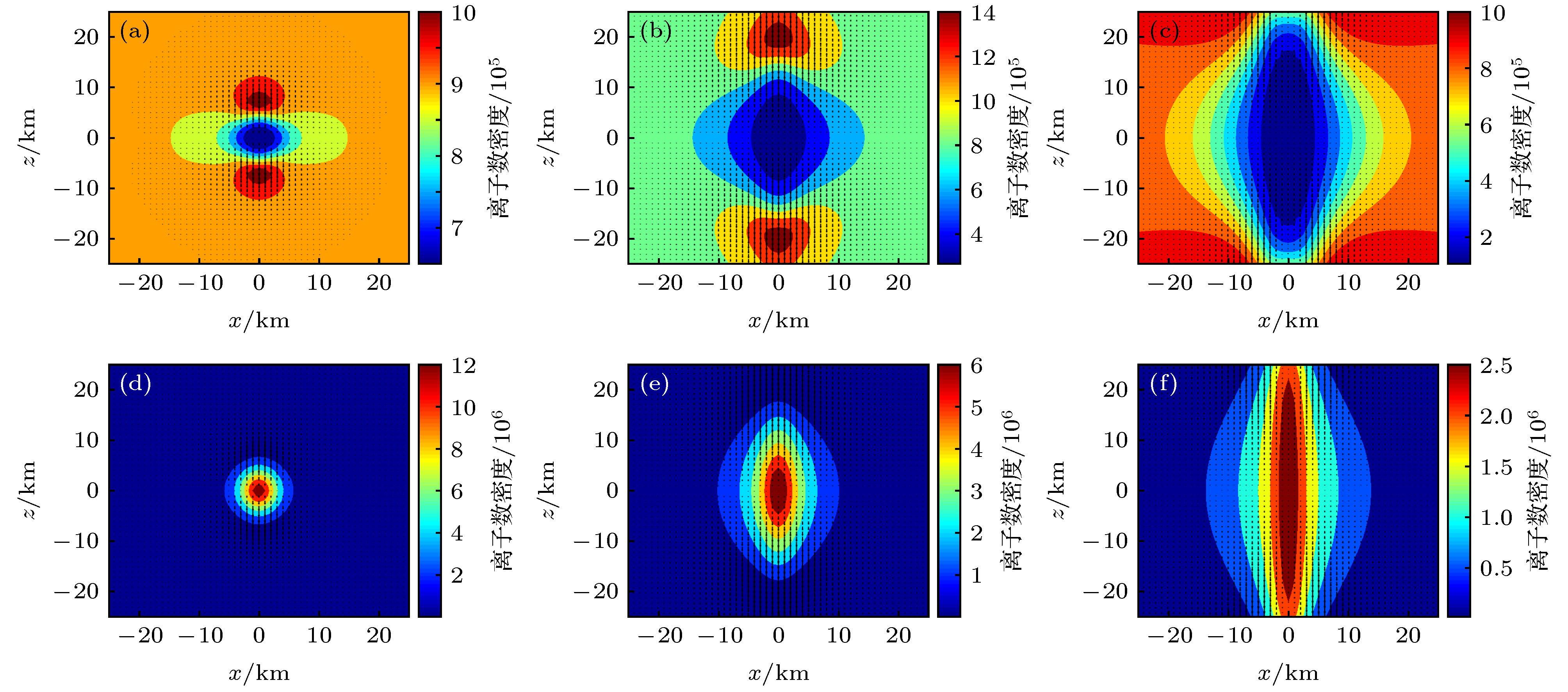
图 3 无中性风场时, 300 km高度释放10 kg钡后钡离子和氧离子的粒子数密度分布(x-z平面)　(a) O⁺, t = 5 s; (b) O⁺, t = 30 s; (c) O⁺, t = 200 s; (d) Ba⁺, t = 5 s; (e) Ba⁺, t = 30 s; (f) Ba⁺, t = 200 s

Fig. 3. Density distribution of Ba⁺ and O⁺ (in x-z plane) after 10 kg barium released at 300 km while no neutral wind is considered: (a) O⁺, t = 5 s; (b) O⁺, t = 30 s; (c) O⁺, t = 200 s; (d) Ba⁺, t = 5 s; (e) Ba⁺, t = 30 s; (f) Ba⁺, t = 200 s.

下载: 全尺寸图片幻灯片

图 4 存在x方向大小为1 km/s的中性风时, 300 km高度释放10 kg钡后钡离子和氧离子的粒子数密度分布(x-z平面)　(a) O⁺, t = 5 s; (b) O⁺, t = 30 s; (c) O⁺, t = 200 s; (d) Ba⁺, t = 5 s; (e) Ba⁺, t = 30 s; (f) Ba⁺, t = 200 s

Fig. 4. Density distribution of Ba⁺ and O⁺ (in x-z plane) after 10 kg barium released at 300 km with a neutral wind of 1 km/s in the x direction: (a) O⁺, t = 5 s;(b) O⁺, t = 30 s; (c) O⁺, t = 200 s; (d) Ba⁺, t = 5 s; (e) Ba⁺, t = 30 s; (f) Ba⁺, t = 200 s.

下载: 全尺寸图片幻灯片

图 5 钡中性云团(绿色)和离子云团(蓝色)在释放后30 s时的三维分布示意图

Fig. 5. Three-dimensional density distribution of barium neutral cloud (green sphere) and ion cloud (blue sphere) at 30 s after release

下载: 全尺寸图片幻灯片

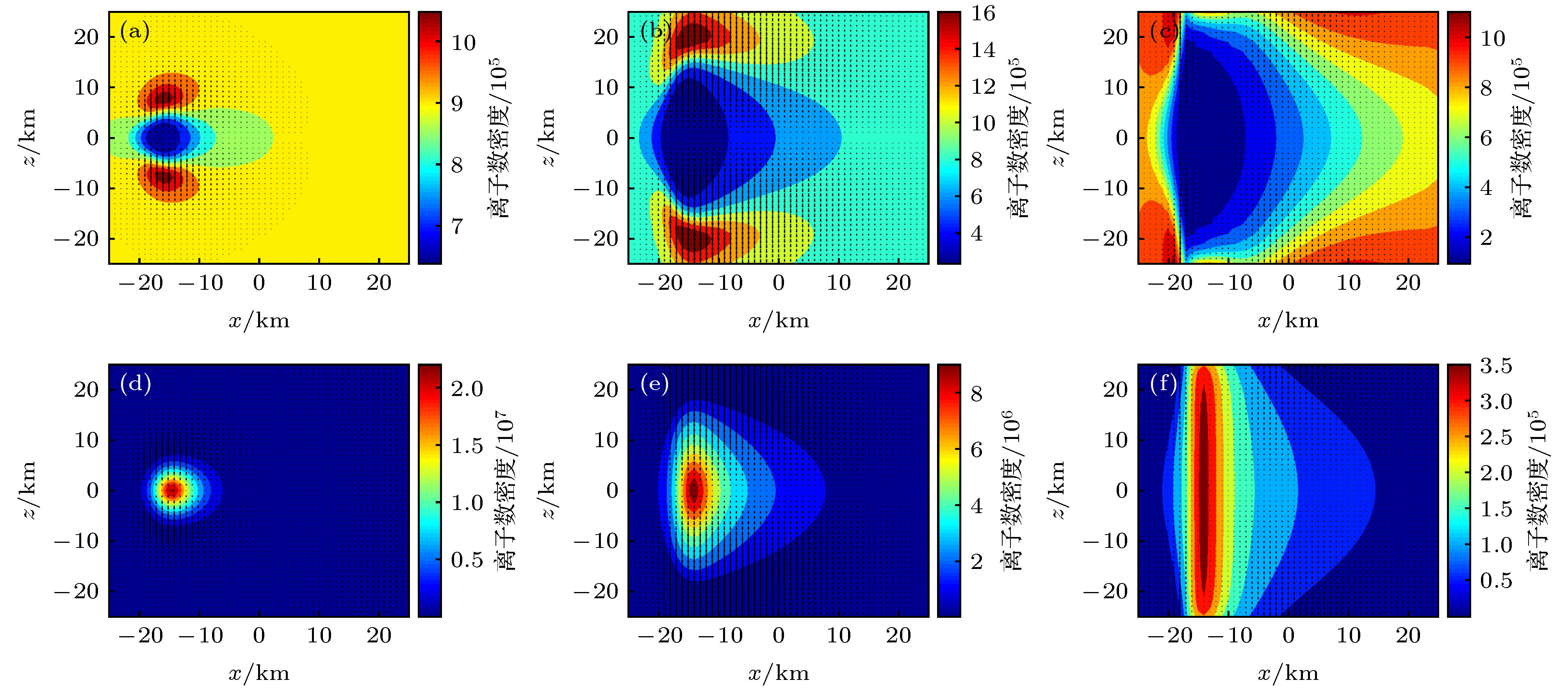
图 6 存在x方向大小为1 km/s的中性风时, 300 km高度释放10 kg铯的粒子数密度分布(x-z平面)　(a) O⁺, t = 5 s; (b) O⁺, t = 30 s; (c) O⁺, t = 200 s; (d) Cs⁺, t = 5 s; (e) Cs⁺, t = 30 s; (f) Cs⁺, t = 200 s

Fig. 6. Density distribution of Cs⁺ and O⁺ (in x-z plane) after 10 kg cesium released at 300 km with a neutral wind of 1 km/s in the x direction: (a) O⁺, t = 5 s; (b) O⁺, t = 30 s; (c) O⁺, t = 200 s; (d) Cs⁺, t = 5 s; (e) Cs⁺, t = 30 s; (f) Cs⁺, t = 200 s.

下载: 全尺寸图片幻灯片

图 7 生成的等离子体云团的密度最大值(a)和背景氧离子的最大扰动值(b)随时间的变化

Fig. 7. The maximum density of artificial plasma cloud (a) and the maximum disturbance of background oxygen ion (a) versus time.

下载: 全尺寸图片幻灯片

表 1 主要仿真参数表

Table 1. The main simulation parameters.

参数	数值(来源)
模拟时间	201709151800LT
释放地点	(22°N, 109°E)
释放高度/km	300
磁场强度/nT	38860
温度/K	860
地磁倾角	32.4°
地磁偏角	–1.9°
氧离子数密度/cm^–3	9 × 10⁵
氧原子数密度/cm^–3	3.06 × 10⁹
光电离率	0.0357(Ba)^[18]/0.05(Cs)^[24]
阻尼系数/s^–1	0.0149(Ba)/0.0208(Cs)
扩散系数/10¹⁰ $ \rm cm^2\cdot s^{-1} $	2.94(Ba)/2.17(Cs)
原子极化率/10^–24 cm³	39.7(Ba)/59.6(Cs)

下载: 导出CSV

map

[1]	Foppl H, Haerendel G, Haser L, Lutjens P, Lust R, Melzner F, Meyer B, Neuss H, Rieger E 1967 Planet. Space Sci. 15 357 Google Scholar
[2]	Haerendel G, Foppl H, Melzner F, Neuss H, Rieger E, Stocker J, Bauer O, Hofner H, Loidl J 1986 Nature 320 700 Google Scholar
[3]	Caton R G, Pedersen T R, Groves K M, et al. 2017 Radio Sci. 52 539 Google Scholar
[4]	Huba J D, Bernhardt P A, Lyon J G 1992 J. Geophys. Res. Space Phys. 97 11 Google Scholar
[5]	Lloyd K H, Haerendel G 1973 J. Geophys. Res. 78 7389 Google Scholar
[6]	Morse D L, Destler W W 1973 J. Geophys. Res. 78 7417 Google Scholar
[7]	Bernhardt P A, Roussel-Dupre R A, Pongratz M B, et al. 1987 J. Geophys. Res. 92 5777 Google Scholar
[8]	Zakharov Y P 2002 Adv. Space Res. 29 1335 Google Scholar
[9]	Haerendel G, Lust R, Rieger E 1967 Planet. Space Sci. 15 1 Google Scholar
[10]	Schunk R W, Szuszczewicz E P 1988 J. Geophys. Res. Space Phys. 93 12901 Google Scholar
[11]	Mitchell H G, Fedder J A, Huba J D, Zalesak S T 1985 J. Geophys. Res. Space Phys. 90 11091 Google Scholar
[12]	Rozhansky V A, Veselova I Y, Voskoboynikov S P 1990 Planet. Space Sci. 38 1375 Google Scholar
[13]	Drake J F, Mulbrandon M, Huba J D 1988 Phys. Fluids 31 3412 Google Scholar
[14]	Ma T Z, Schunk R W 1991 J. Geophys. Res. Space Phys. 96 5793 Google Scholar
[15]	Ma T Z, Schunk R W 1994 J. Geophys. Res. 99 6331 Google Scholar
[16]	Mendillo M, Hawkins G S, Klobuchar J A 1975 Science 18734 3
[17]	Klobuchar J A, Abdu M A 1989 J. Geophys. Res. Space Phys. 94 2721 Google Scholar
[18]	Choueiri E Y, Oraevsky V N, Dokukin V S 2001 J. Geophys. Res. 106 25673 Google Scholar
[19]	Bernhardt P A 1979 J. Geophys. Res. 84 793 Google Scholar
[20]	Mendillo M, Semeter J, Noto J 1993 Adv. Space Res. 13 55
[21]	Scales W A, Bernhardt P A, Ganguli G 1994 J. Geophys. Res. 99 373 Google Scholar
[22]	Kolomiitsev O P, Ruzhin Y Y, Egorov I B, Razinkov O G, Cherkashin Y N 1999 Phys. Chem. Earth Part C 24 393 Google Scholar
[23]	黄文耿, 古士芬 2005 空间科学学报 25 254 Google Scholar Huang W G, Gu S F 2005 Chin. J. Space Sci. 25 254 Google Scholar
[24]	黄勇, 时家明, 袁忠才 2011 地球 54 1 Google Scholar Huang Y, Shi J M, Yuan Z C 2011 Chin J. Geophys. 54 1 Google Scholar
[25]	胡耀垓, 赵正予, 张援农 2010 59 8293 Google Scholar Hu Y G, Zhao Z Y, Zhang Y N 2010 Acta Phys. Sin. 59 8293 Google Scholar
[26]	胡耀垓, 赵正予, 张援农 2013 62 209401 Google Scholar Hu Y G, Zhao Z Y, Zhang Y N 2013 Acta Phys. Sin. 62 209401 Google Scholar
[27]	汪四成, 方涵先, 杨升高, 翁利斌 2012 地球物理学研究进展 27 2464 Wang S C, Fang H X, Yang S G 2012 Progress in Geophys. 27 2464
[28]	赵海生, 许正文, 吴振森, 冯杰, 吴健, 徐彬, 徐彤, 胡艳莉 2016 65 209401 Google Scholar Zhao H S, Xu Z W, Wu Z S, Feng J, Wu J, Xu B, Xu T, Hu Y L 2016 Acta Phys. Sin. 65 209401 Google Scholar
[29]	Li L, Xu R L 2002 Chin. Phys. Lett. 19 1214 Google Scholar
[30]	胡耀垓, 赵正予, 张援农 2012 61 089401 Google Scholar Hu Y G, Zhao Z Y, Zhang Y N 2012 Acta Phys. Sin. 61 089401 Google Scholar
[31]	谢良海 2013 博士学位论文(北京: 中国科学院大学) Xie L H 2013 Ph. D. Dissertation (Beijing: University of Chinese Academy of Sciences) (in Chinese)
[32]	Scholer, M 1970 Planet. Space Sci. 18 977 Google Scholar
[33]	Pressman J, Marrmo F F, Aschenbrand L M 1960 Planet. Space Sci. 2 228 Google Scholar
[34]	Holmgren G, Kintner P M, Kelley M C 1981 Adv. Space Res. 1 311 Google Scholar
[35]	Eliason L, Lundin R, Holmgren G 1988 Adv. Space Res. 8 93
[36]	Bleecker D K, Bogaerts A, Gijbels R, Goedheer W 2004 Phys. Rev. E 69 056409 Google Scholar
[37]	Xing Z, Anbang S, Le T, Guan J Z 2019 AIP Adv. 9 015117 Google Scholar

[1]	杨雨森, 王林, 苟德梽, 唐正明. 等离子体-光子晶体阵列结构波导模型的电磁特性研究. , 2024, 73(24): . doi: 10.7498/aps.73.20241300
[2]	张东荷雨, 刘金宝, 付洋洋. 激光维持等离子体多物理场耦合模型与仿真. , 2024, 73(2): 025201. doi: 10.7498/aps.73.20231056
[3]	杨楠楠, 王尚民, 张家良, 温小琼, 赵凯. 改进型机-电模型及脉冲等离子体推力器能量转化效率分析. , 2024, 73(21): 215202. doi: 10.7498/aps.73.20241117
[4]	张津硕, 孙辉, 杜志杰, 张雪航, 肖青梅, 范金蕤, 闫慧杰, 宋健. 预填充模式下同轴枪放电等离子体加速模型分析与优化. , 2023, 72(15): 155202. doi: 10.7498/aps.72.20230463
[5]	韩小英, 李凌霄, 戴振生, 郑无敌, 古培俊, 吴泽清. 一个快速模拟热稠密非平衡等离子体的碰撞辐射模型. , 2021, 70(11): 115202. doi: 10.7498/aps.70.20201946
[6]	孙俊超, 张宗国, 董焕河, 杨红卫. 尘埃等离子体中的分数阶模型及其Lump解. , 2019, 68(21): 210201. doi: 10.7498/aps.68.20191045
[7]	陈文波, 龚学余, 路兴强, 冯军, 廖湘柏, 黄国玉, 邓贤君. 基于动理论模型的一维等离子体电磁波传输特性分析. , 2014, 63(21): 214101. doi: 10.7498/aps.63.214101
[8]	何福顺, 李刘合, 李芬, 顿丹丹, 陶婵偲. 增强辉光放电等离子体离子注入的三维PIC/MC模拟. , 2012, 61(22): 225203. doi: 10.7498/aps.61.225203
[9]	钱利波, 朱樟明, 杨银堂. 一种考虑硅通孔电阻-电容效应的三维互连线模型. , 2012, 61(6): 068001. doi: 10.7498/aps.61.068001
[10]	石兰芳, 欧阳成, 陈丽华, 莫嘉琪. 一类大气等离子体反应扩散模型的解法. , 2012, 61(5): 050203. doi: 10.7498/aps.61.050203
[11]	李向东. 基于等离子体环境涨落的原子结构计算模型. , 2011, 60(5): 053201. doi: 10.7498/aps.60.053201
[12]	段耀勇, 郭永辉, 邱爱慈, 吴刚. 碰撞辐射稳态等离子体电荷态分布的一种扩展模型. , 2010, 59(8): 5588-5595. doi: 10.7498/aps.59.5588
[13]	孟立民, 滕爱萍, 李英骏, 程涛, 张杰. 基于自相似模型的二维X射线激光等离子体流体力学. , 2009, 58(8): 5436-5442. doi: 10.7498/aps.58.5436
[14]	吴翊, 荣命哲, 杨飞, 王小华, 马强, 王伟宗. 引入6波段P-1辐射模型的三维空气电弧等离子体数值分析. , 2008, 57(9): 5761-5767. doi: 10.7498/aps.57.5761
[15]	宋法伦, 曹金祥, 王舸. 弱电离等离子体对电磁波吸收的物理模型和数值求解. , 2005, 54(2): 807-811. doi: 10.7498/aps.54.807
[16]	王龙. 等离子体中的颗粒成长模型. , 1999, 48(6): 1072-1077. doi: 10.7498/aps.48.1072
[17]	冯小兵, 章立源, 孙久勋. 双成分模型及YBCO/Pb结沿c轴的Josephson效应. , 1997, 46(3): 596-603. doi: 10.7498/aps.46.596
[18]	宫野, 温晓军, 张鹏云, 邓新绿. 圆柱模型下电子回旋共振微波等离子体离子输运过程的数值研究. , 1997, 46(12): 2376-2383. doi: 10.7498/aps.46.2376
[19]	张承福. 等离子体模型碰撞项的比较. , 1986, 35(7): 947-952. doi: 10.7498/aps.35.947
[20]	石长和. 双等离子体流模型中阿尔芬波的非几何光学近似解. , 1983, 32(1): 25-32. doi: 10.7498/aps.32.25

计量

文章访问数: 7950
PDF下载量: 69
被引次数: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

搜索

留言板