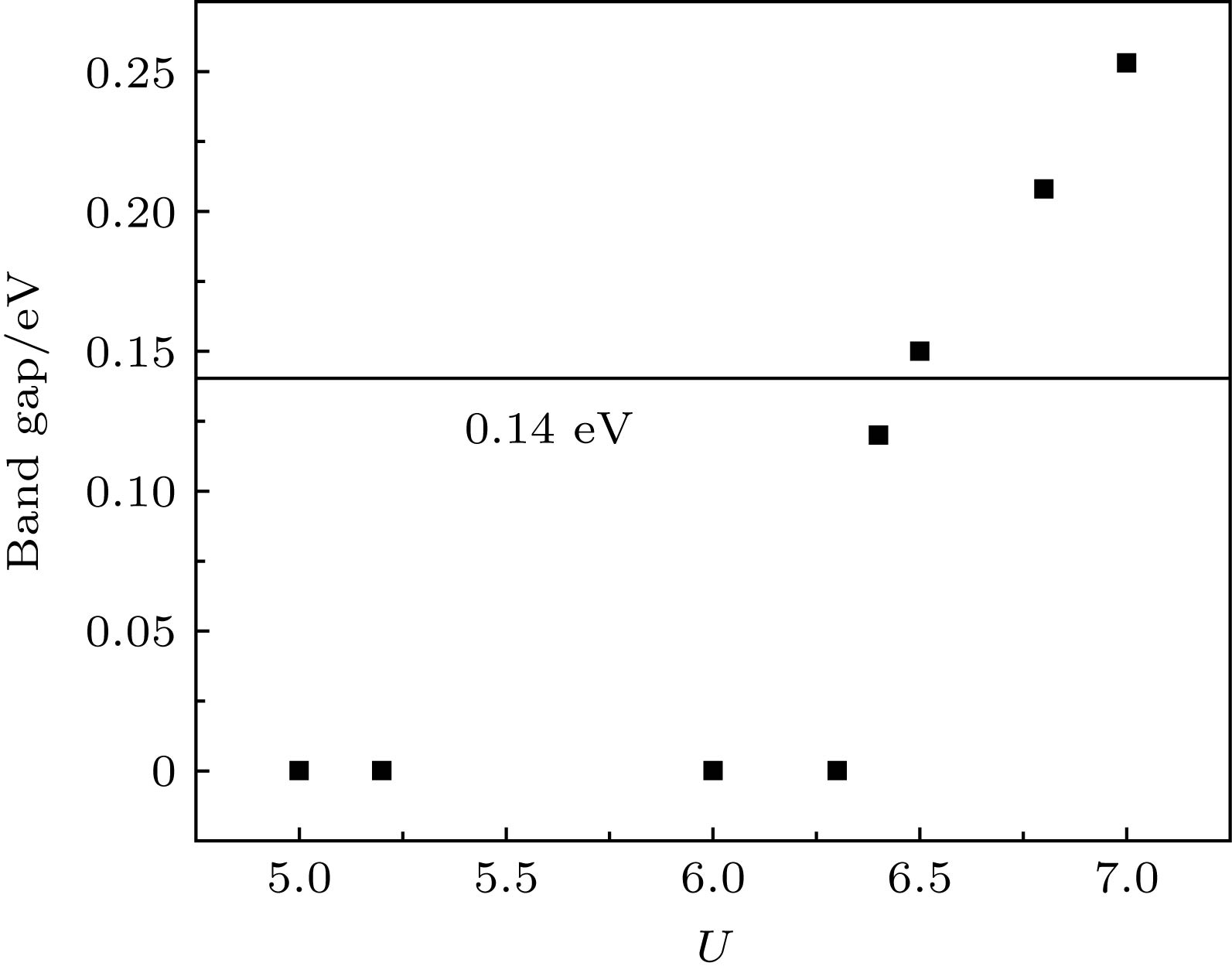
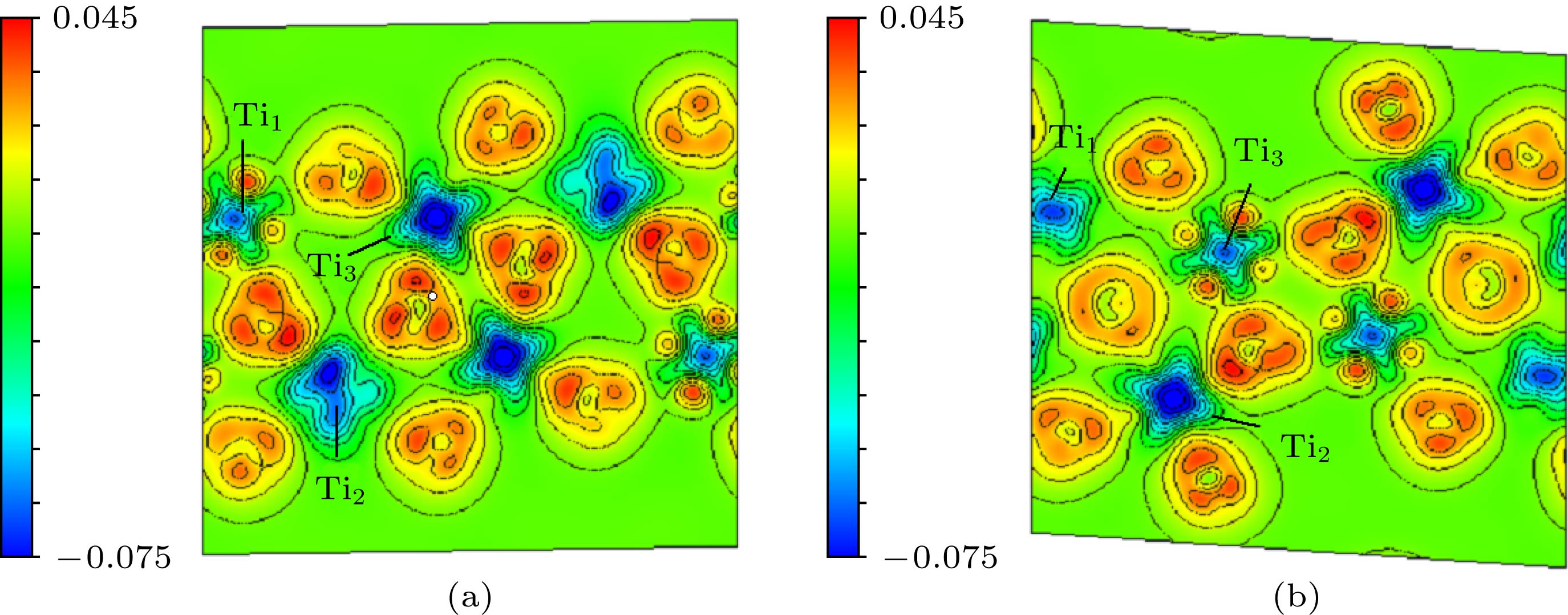
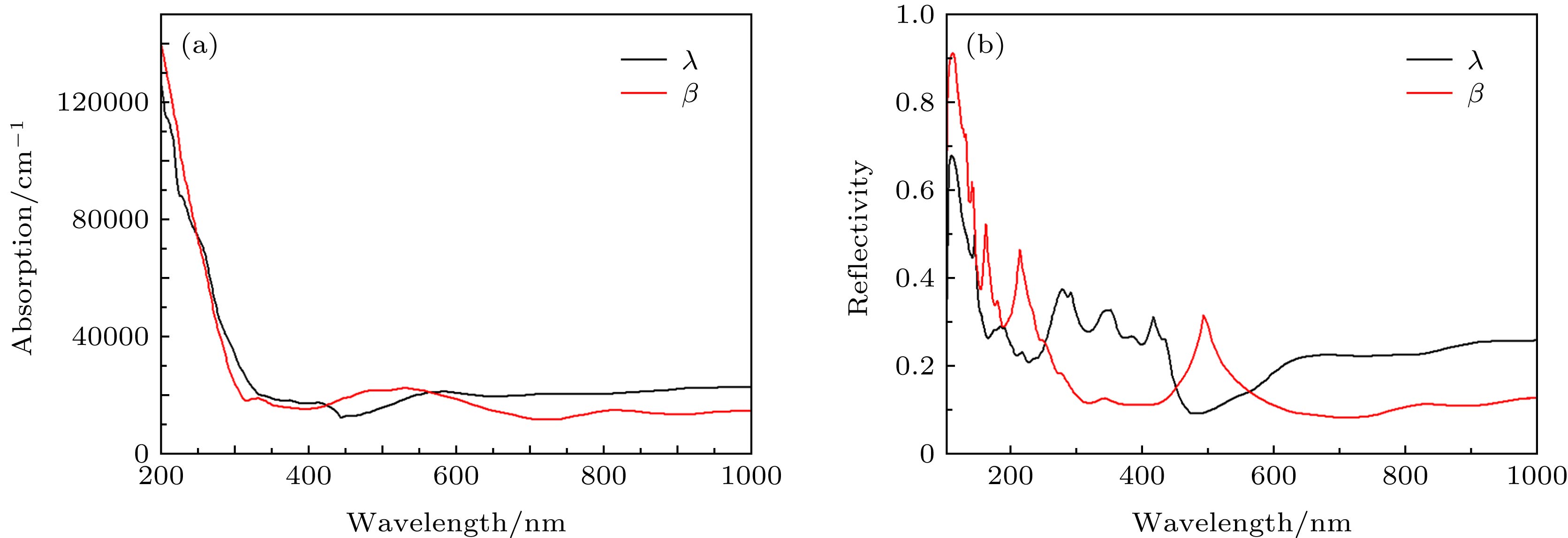
-
Ti3O5 is a highly anticipated functional material due to its intriguing physical properties and multi-incentive phase transition process. This material undergoes a reversible first-order phase transition between λ phase and β phase, accompanied by drastic changes in the electronic and atomic structure. The amazing way of changing phase such as light, heat, pressure and electric current makes this material promise to be used in future optical information storage and heat storage. In this work, structural, elastic, electronic and optical properties of λ-Ti3O5 and β-Ti3O5 are calculated by using a first-principles approach, according to density functional theory (DFT). The LSDA, GGA-PBE, GGA-91, GGA-PS and GGA-RP are compared among them to obtain a suitable method of characterizing the crystal structure and electronic structure of λ-Ti3O5 and β-Ti3O5. The energy gap at the Fermi level of β-Ti3O5 can be obtained only when the extra Coulomb correlation U effect of Ti 3d electrons is considered. A complete set of elastic parameters for both phases are first reported. The calculated elastic constant satisfies the Born stability criterion, indicating that λ phase and β phase have good mechanical structural stability. The two phases are more resistant to volume changes than to shape changes and both behave as ductile materials. On the (010) plane, the elastic anisotropy of λ phase is weaker than that of β phase. Studies on the electronic structure show that the local charge of Ti3 is transferred to Ti2, resulting in the transformation of the semiconductor β phase to the metal λ phase. There are large differences in optical property such as absorption and reflectivity between these two structures, indicating that they can be applied to the field of optical storage materials. In this paper we also present a new insight into the photoinduced phase transition process of this material. The mechanism of photoinduced phase transition from λ-Ti3O5 to β-Ti3O5 is considered as stimulated emission effect. The results are of significance particularly for practically applying Ti3O5 and understanding its phase change mechanism.
-
Keywords:
- Ti3O5 /
- first principle /
- elastic properities /
- electronic properties
[1] Tominaga J, Haratani S, Uchiyama K 1992 J. Appl. Phys. 31 2757
Google Scholar
[2] Bussjager R, Chaiken J, Getbehead M, Grucza D, Hinkel C D, McEwen T, Osman J, Voss E 2000 J. Appl. Phys. 39 789
Google Scholar
[3] Sasa N, Hayashi Y, Fujii T, Otaka K, Watada A, Kamezaki H, Komoda H 2004 J. Appl. Phys. 43 4972
Google Scholar
[4] Ovshinsky S R 1968 Phys. Rev. Lett. 21 1450
Google Scholar
[5] Li J, Hou L, Gan F 2001 Chin. J. Lasers 10 3
[6] Ohkoshi S, Tsunobuchi Y, Matsuda T, Hashimoto K, Namai A, Hakoe F, Tokoro H 2010 Nat. Chem. 2 539
Google Scholar
[7] Hakoe F, Tokoro H, Ohkoshi S 2017 Mater. Lett. 188 8
Google Scholar
[8] Kobayashi K, Taguchi M, Kobata M, Tanaka K, Tokoro H, Daimon H, Okane T, Yamagami H, Ikenaga E, Ohkoshi S 2017 Phys. Rev. B 95 085133
Google Scholar
[9] Liu R, Shang J X 2012 J. Synth. Cryst. 41 376
[10] Nasu T, Tokoro H, Tanaka K, Hakoe F, Namai A, Ohkoshi S 2014 Mat. Sci. Eng. 54 012008
Google Scholar
[11] Ould-Hamouda A, Tokoro H, Ohkoshi S, Freysz E 2014 Chem. Phys. Lett. 609 106
Google Scholar
[12] Asahara A, Watanabe H, Tokoro H, Ohkoshi S, Suemoto T 2014 Phys. Rev. B 90 014303
Google Scholar
[13] Liu G, Huang W X, Yi Y 2013 J. Inorg. Mater. 28 425
Google Scholar
[14] Shi Q W, Chai G Q, Huang W X, Shi Y L, Huang B, Wei D, Qi J Q, Su F H, Xu W, Lu T C 2016 J. Mater. Chem. C 4 10279
Google Scholar
[15] Chai G Q, Huang W X, Shi Q W, Zheng S P, Wei D 2015 J. Alloy. Compd. 621 404
Google Scholar
[16] Liu R, Shang J X 2012 Model. Simul. Mater. Sc. 20 035020
Google Scholar
[17] Wei D, Huang W X, Shi Q W, Lu T C, Huang B 2016 J. Mater. Sci.-Mater. El. 27 4216
Google Scholar
[18] Tokoro H, Yoshikiyo M, Lmoto K, Namai A, Nasu T, Nakagawa K, Ozaki N, Hakoe F, Tanaka K, Chiba K, Makiura R, Prassides K, Ohkoshi S 2015 Nat. Commun. 6 7037
Google Scholar
[19] Asbrink S, Magneli A 1959 Acta Cryst. 12 575
Google Scholar
[20] 张丽丽, 夏桐, 刘桂安, 雷博程, 赵旭才, 王少霞, 黄以能 2019 68 017401
Google Scholar
Zhang L L, Xia T, Liu G A, Lei B C, Zhao X C, Wang S X, Huang Y N 2019 Acta Phys. 68 017401
Google Scholar
[21] Dang J N, Zheng S W, Chen L, Zheng T 2019 Chin. Phys. B 28 16301
Google Scholar
[22] Monkhorst H J, Pack J D 1976 Phys. Rev. B 13 5188
Google Scholar
[23] Liu R, Shang J X, Wang F H 2014 Comput. Mater. Sci. 81 158
Google Scholar
[24] Olguin D, Vallejo E, Ponce A R 2015 Phys. Status Solidi B 252 659
Google Scholar
[25] Wu Y, Zhang Q, Wu X, Qin S, Liu J 2012 J. Solid State Chem. 192 356
Google Scholar
[26] Grimvall G, Magyari-Kope B, Ozolins V, Persson K A 2012 Rev. Mod. Phys. 84 945
Google Scholar
[27] Wu Z J, Zhao E J, Xiang H P, Hao X F, Liu X J, M J 2007 Phys. Rev. B 76 054115
Google Scholar
[28] Hill R 2002 P. Phys. Soc. A 65 349
[29] Asbrink S, Gerward L, Olsen J S 1989 J. Appl. Cryst. 22 119
Google Scholar
[30] Pugh S F 1954 Philos. Mag. 45 823
Google Scholar
[31] Jahn H A, Teller E 1937 P. Roy. Soc. Lond. A-MAT 161 220
Google Scholar
-
图 1 (a) λ-Ti3O5和(b) β-Ti3O5的晶体结构
Fig. 1. Crystal structures of (a) λ-Ti3O5 and (b) β-Ti3O5.
图 2 LSDA泛函计算得到的β-Ti3O5的禁带宽度随U值的变化
Fig. 2. The band gap of β-Ti3O5 calculated by LSDA as a function of U values.
图 3 λ-Ti3O5的(a)能带结构和(b)态密度以及β-Ti3O5的(c)能带结构和(d)态密度
Fig. 3. The calculated (a) band structure and (b) total density of states of λ-Ti3O5; the calculated (c) band structure and (d) total density of states of β-Ti3O5.
图 4 (a) λ-Ti3O5和(b) β-Ti3O5 的分态密度
Fig. 4. The partial density of states for (a) λ-Ti3O5 and (b) β-Ti3O5.
图 5 (a) λ-Ti3O5和(b) β-Ti3O5的(010)晶面上的差分电荷密度
Fig. 5. The calculated charge density differences of (010) plane for (a) λ-Ti3O5 and (b) β-Ti3O5.
图 6 λ-Ti3O5和β-Ti3O5的光吸收以及反射率谱图
Fig. 6. The calculated absorption spectra and reflection coefficients of λ-Ti3O5 and β-Ti3O5.
图 7 (a) λ-Ti3O5和(b) β-Ti3O5费米能级附近的能带结构
Fig. 7. The band structures near the Fermi level of (a) λ-Ti3O5 and (b) β-Ti3O5.
表 1 计算得到的晶格常数
Table 1. Calculated lattice parameters.
a/Å b/Å c/Å Ref. λ-Ti3O5 exp. 9.84 3.79 9.99 [6] 9.84 3.79 9.97 [6] 9.83 3.79 9.97 [18] LSDA + U 10.02 3.90 10.15 GGA-PBE + U 10.23 3.96 10.39 GGA-91 + U 10.22 3.95 10.38 GGA-PS + U 10.22 3.96 10.38 GGA-RP + U 10.32 3.98 10.49 β-Ti3O5 exp. 9.75 3.80 9.44 [19] 9.75 3.80 9.44 [18] LSDA + U 9.92 3.94 9.67 GGA-PBE + U 10.37 4.03 9.79 GGA-91 + U 10.35 4.03 9.79 GGA-PS + U 10.25 4.00 9.66 GGA-RP + U 10.45 4.06 9.90 
下载: 导出CSV
表 2 计算得到的弹性常数(单位为GPa)
Table 2. Calculated elastic constants (Cij in GPa).
C11 C22 C33 C44 C55 C66 C12 C13 C15 C25 C35 C46 C23 λ-Ti3O5 262.7 342.5 283.2 56.3 87.7 25.5 142.8 109.2 –20.4 –9.4 0.3 –2.0 113.9 β-Ti3O5 207.4 428.1 334.5 50.0 93.0 10.6 114.8 101.6 –4.5 4.6 30.8 –8.7 94.6
下载: 导出CSV
表 3 计算得到的体积模量(BH, GPa), 剪切模量(GH, GPa), 杨氏模量(YH, GPa)和泊松比(ν)
Table 3. Calculated bulk modulus (BH, in GPa), shear modulus (GH, in GPa), Young's modulus (YH, in GPa) and Poisson's ratio (ν).
BH GH YH ν BH/GH λ-Ti3O5 210.8 63.4 173.0 0.36 3.32 β-Ti3O5 187.4 52.8 144.8 0.37 3.54
下载: 导出CSV
-
[1] Tominaga J, Haratani S, Uchiyama K 1992 J. Appl. Phys. 31 2757
Google Scholar
[2] Bussjager R, Chaiken J, Getbehead M, Grucza D, Hinkel C D, McEwen T, Osman J, Voss E 2000 J. Appl. Phys. 39 789
Google Scholar
[3] Sasa N, Hayashi Y, Fujii T, Otaka K, Watada A, Kamezaki H, Komoda H 2004 J. Appl. Phys. 43 4972
Google Scholar
[4] Ovshinsky S R 1968 Phys. Rev. Lett. 21 1450
Google Scholar
[5] Li J, Hou L, Gan F 2001 Chin. J. Lasers 10 3
[6] Ohkoshi S, Tsunobuchi Y, Matsuda T, Hashimoto K, Namai A, Hakoe F, Tokoro H 2010 Nat. Chem. 2 539
Google Scholar
[7] Hakoe F, Tokoro H, Ohkoshi S 2017 Mater. Lett. 188 8
Google Scholar
[8] Kobayashi K, Taguchi M, Kobata M, Tanaka K, Tokoro H, Daimon H, Okane T, Yamagami H, Ikenaga E, Ohkoshi S 2017 Phys. Rev. B 95 085133
Google Scholar
[9] Liu R, Shang J X 2012 J. Synth. Cryst. 41 376
[10] Nasu T, Tokoro H, Tanaka K, Hakoe F, Namai A, Ohkoshi S 2014 Mat. Sci. Eng. 54 012008
Google Scholar
[11] Ould-Hamouda A, Tokoro H, Ohkoshi S, Freysz E 2014 Chem. Phys. Lett. 609 106
Google Scholar
[12] Asahara A, Watanabe H, Tokoro H, Ohkoshi S, Suemoto T 2014 Phys. Rev. B 90 014303
Google Scholar
[13] Liu G, Huang W X, Yi Y 2013 J. Inorg. Mater. 28 425
Google Scholar
[14] Shi Q W, Chai G Q, Huang W X, Shi Y L, Huang B, Wei D, Qi J Q, Su F H, Xu W, Lu T C 2016 J. Mater. Chem. C 4 10279
Google Scholar
[15] Chai G Q, Huang W X, Shi Q W, Zheng S P, Wei D 2015 J. Alloy. Compd. 621 404
Google Scholar
[16] Liu R, Shang J X 2012 Model. Simul. Mater. Sc. 20 035020
Google Scholar
[17] Wei D, Huang W X, Shi Q W, Lu T C, Huang B 2016 J. Mater. Sci.-Mater. El. 27 4216
Google Scholar
[18] Tokoro H, Yoshikiyo M, Lmoto K, Namai A, Nasu T, Nakagawa K, Ozaki N, Hakoe F, Tanaka K, Chiba K, Makiura R, Prassides K, Ohkoshi S 2015 Nat. Commun. 6 7037
Google Scholar
[19] Asbrink S, Magneli A 1959 Acta Cryst. 12 575
Google Scholar
[20] 张丽丽, 夏桐, 刘桂安, 雷博程, 赵旭才, 王少霞, 黄以能 2019 68 017401
Google Scholar
Zhang L L, Xia T, Liu G A, Lei B C, Zhao X C, Wang S X, Huang Y N 2019 Acta Phys. 68 017401
Google Scholar
[21] Dang J N, Zheng S W, Chen L, Zheng T 2019 Chin. Phys. B 28 16301
Google Scholar
[22] Monkhorst H J, Pack J D 1976 Phys. Rev. B 13 5188
Google Scholar
[23] Liu R, Shang J X, Wang F H 2014 Comput. Mater. Sci. 81 158
Google Scholar
[24] Olguin D, Vallejo E, Ponce A R 2015 Phys. Status Solidi B 252 659
Google Scholar
[25] Wu Y, Zhang Q, Wu X, Qin S, Liu J 2012 J. Solid State Chem. 192 356
Google Scholar
[26] Grimvall G, Magyari-Kope B, Ozolins V, Persson K A 2012 Rev. Mod. Phys. 84 945
Google Scholar
[27] Wu Z J, Zhao E J, Xiang H P, Hao X F, Liu X J, M J 2007 Phys. Rev. B 76 054115
Google Scholar
[28] Hill R 2002 P. Phys. Soc. A 65 349
[29] Asbrink S, Gerward L, Olsen J S 1989 J. Appl. Cryst. 22 119
Google Scholar
[30] Pugh S F 1954 Philos. Mag. 45 823
Google Scholar
[31] Jahn H A, Teller E 1937 P. Roy. Soc. Lond. A-MAT 161 220
Google Scholar
下载:
计量
- 文章访问数: 10932
- PDF下载量: 182
- 被引次数: 0
