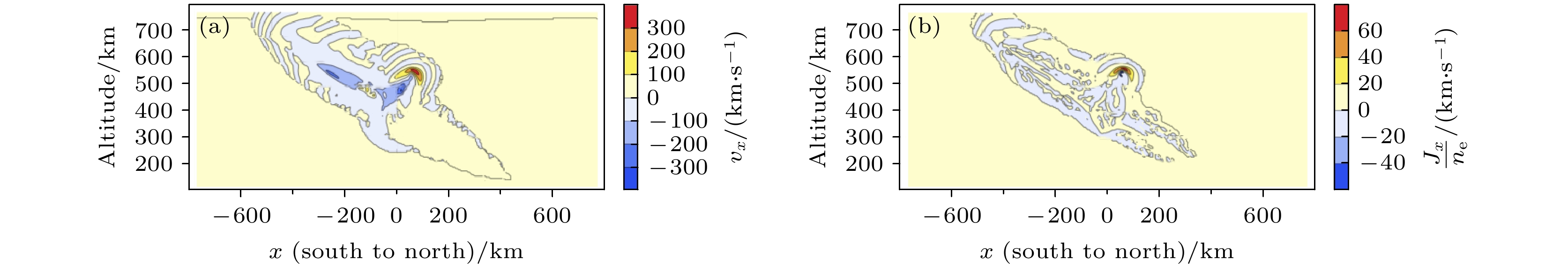
-
A Hydro-Magneto-PIC (particle-in-cell) hybrid model is proposed to describe the motion of the fission debris in high altitude nuclear explosions (HANEs). Compared with the state-of-art numerical models, our model is able to stably compute the motion of the fission debris in a broader spatial region. In a real HANE, the physical process contains many spatial scales. The upward moving debris particles manifest kinetic properties due to the fact that the dilute ambient atmosphere and the downward expanding particles manifest a fluid-like pattern and can be approximated by the usual hydro-dynamical models. Meanwhile, the debris particles receive electromagnetic forces from both the geomagnetic fields and the charged particles at all frequencies. This broad scale of frequencies can induce large- and small-scale instabilities, which cannot be solved by the usual hydrodynamic equations. Considering the motions of the debris and the different properties of the high temperature ions, the low temperature ions and the neutral atmosphere, we consistently combine three models for completely describing the debris expansion. The high temperature ions are described by the PIC model for their intrinsic kinetic behaviors, the low temperature ions are described by the magneto-hydrodynamic model for their fluid property, and fluid equations are applied to the neutral particles with no electromagnetic force. The corresponding interactions among the three components are added into the equations as the source terms. With the combination of the three models, our algorithm can stably calculate the regions that are a few thousand kilometers in altitude. Our proposed model contains both the kinetic and fluid properties, and is stable in numerical implementations. Finally, we calculate the debris motion in the Starfish experiment. The results confirm a consistency of our proposed model with the observations. The spatial scale of our simulation results is consistent with the result in the Starfish experiment. In addition, we also plot the distribution of the debris with different projection angles at various snapshots. These results give us an intuition to understand the influence of the various factors, such as the friction of atmosphere, the magnetic pressure, flute instability and the Hall currents. Our model provides a tool for implementing the HANE simulation in a broader scheme, and can also be utilized in other plasma systems.
[1] Trelat S, Sochet I, Autrusson B, Cheval K, Loiseau O 2007 J. Loss Prev. Process Ind. 5 4
[2] Winske D, Omidi N 1990 Geophys. Res. Lett. 17 2297
Google Scholar
[3] Drake J F, Mulbrandon M, Huba J D 1988 Phys. Fluids 31 3412
Google Scholar
[4] [5] Hideo A, Ikuo I, Toyoaki H, Futoshi O 1981 J. Phys. Soc. Japan 50 2729
Google Scholar
[6] Kopecky V 1968 Nucl. Fusion 8 313
Google Scholar
[7] Stirling A C 1965 J. Geophys. Res. 70 3161
Google Scholar
[8] Longmire C L, Hobbs W E 1997 Fireball Effects in Late-time Emp From Surface Bursts (Santa Barbara: Mission Research Corp) AD-A-066604
[9] [10] Durney A C, Elliot H, Hynds R J, Quenby J J 1962 Nature 195 1245
Google Scholar
[11] Hess W N 1963 J. Geophys. Res. 68 667
Google Scholar
[12] Unterberger R R, Byerly P E 1962 J. Geophys. Res. 67 4929
Google Scholar
[13] Baker R C, Strome W M 1962 J. Geophys. Res. 67 4927
Google Scholar
[14] Siebert K, Witt E 2019 Nominal Waveforms for Late-time High-altitude Electromagnetic Pulse (HEMP) DTRA-TR-19-41
[15] Stuart G W 1965 Phys. Fluids 8 603
Google Scholar
[16] 乔登江, 华鸣 1986 抗核加固 3 98
Qiao D J, Hua M 1986 Antinuclear Hardening 3 98
[17] 杨斌, 牛胜利, 朱金辉, 黄流兴 2012 61 202801
Google Scholar
Yang B, Niu S L, Zhu J H 2012 Acta Phys. Sin. 61 202801
Google Scholar
[18] John Z, Herman H, Albert G 1966 Radiation Trapped (Dordrech: Springer) p671
[19] Douglas S H 1982 J. Comput. Phys. 47 452
Google Scholar
[20] Brecht S H, Orens J H 1983 Study of Field Aligned Plasma Acceleration during a HANE Ad-A141340
[21] Thomas V A, Brecht S H 1986 Phys. Fluids 29 2444
Google Scholar
[22] Morrow D P 2014 Master Dissertation (Calhoun: Institutional Archive of the Naval Postgraduate School)
[23] Holmstrom M 2013 ASP Conference Series 474 202
[24] Thomas V A, Brecht S H 1987 J. Geophys. Res. 92 2289
Google Scholar
[25] Bai X N, Caprioli D, Sironi L, Spitkovsky A 2015 Astrophys. J. 809 55
Google Scholar
[26] Dan H H, Alfred M K, Austin A O 1977 Physics of High-altitude Nuclear Burst Effects DNA 4501F
[27] James M S, Thomas A G 2008 Astrophys. J. Suppl. Ser. 178 137
Google Scholar
[28] 王建国, 牛胜利, 张殿辉 2010 高空核爆炸效应参数手册 (北京: 原子能出版社) 第37页
Wang J G, Niu S L, Zhang D H 2010 Parameter Hand Book of High Attitude Nuclear Detonation Effects (Bejing: Atomic Energy Press) p37 (in Chinese)
-
图 1 Starfish试验照片: 爆后4 s β射线沉积区图像
Figure 1. Photos of Starfish: β patch photo after burst time 4 s
图 2 不同时刻Starfish试验模拟计算的三维碎片云密度沿子午面方向的投影面密度云图 (a) 0.1 s; (b) 0.2 s; (c) 0.5 s; (d) 1 s
Figure 2. Contour plot of three-dimensional debris density in the geomagnetic meridian plane in Starfish experiment at different time: (a) 0.1 s; (b) 0.2 s; (c) 0.5 s; (d) 1 s.
图 3 不同时刻Starfish试验模拟计算的三维碎片云密度沿磁力线方向的投影面密度云图 (a) 0.1 s; (b) 0.2 s; (c) 0.5 s; (d) 1 s
Figure 3. Contour plot of three-dimensional debris density in the XOY plane (perpendicular to the geomagnetic fields) in Starfish experiment at different time: (a) 0.1 s; (b) 0.2 s;(c) 0.5 s;(d) 1 s.
图 4 不同时刻Starfish试验模拟计算的三维碎片云密度沿高度方向的投影面密度云图 (a) 0.1 s; (b) 0.2 s; (c) 0.5 s; (d) 1 s
Figure 4. Contour plot of three-dimensional debris density in the XOY plane (perpendicular to altitude direction) in Starfish experiment at different time: (a) 0.1 s; (b) 0.2 s; (c) 0.5 s; (d) 1 s.
图 5 爆后0.2 s子午面上的x0方向速度分布: (a)离子速度; (b)霍尔速度
Figure 5. Velocity distributions in the x0 direction in meridian plane at t = 0.2 s: (a) Ion velocity; (b) Hall velocity.
-
[1] Trelat S, Sochet I, Autrusson B, Cheval K, Loiseau O 2007 J. Loss Prev. Process Ind. 5 4
[2] Winske D, Omidi N 1990 Geophys. Res. Lett. 17 2297
Google Scholar
[3] Drake J F, Mulbrandon M, Huba J D 1988 Phys. Fluids 31 3412
Google Scholar
[4] [5] Hideo A, Ikuo I, Toyoaki H, Futoshi O 1981 J. Phys. Soc. Japan 50 2729
Google Scholar
[6] Kopecky V 1968 Nucl. Fusion 8 313
Google Scholar
[7] Stirling A C 1965 J. Geophys. Res. 70 3161
Google Scholar
[8] Longmire C L, Hobbs W E 1997 Fireball Effects in Late-time Emp From Surface Bursts (Santa Barbara: Mission Research Corp) AD-A-066604
[9] [10] Durney A C, Elliot H, Hynds R J, Quenby J J 1962 Nature 195 1245
Google Scholar
[11] Hess W N 1963 J. Geophys. Res. 68 667
Google Scholar
[12] Unterberger R R, Byerly P E 1962 J. Geophys. Res. 67 4929
Google Scholar
[13] Baker R C, Strome W M 1962 J. Geophys. Res. 67 4927
Google Scholar
[14] Siebert K, Witt E 2019 Nominal Waveforms for Late-time High-altitude Electromagnetic Pulse (HEMP) DTRA-TR-19-41
[15] Stuart G W 1965 Phys. Fluids 8 603
Google Scholar
[16] 乔登江, 华鸣 1986 抗核加固 3 98
Qiao D J, Hua M 1986 Antinuclear Hardening 3 98
[17] 杨斌, 牛胜利, 朱金辉, 黄流兴 2012 61 202801
Google Scholar
Yang B, Niu S L, Zhu J H 2012 Acta Phys. Sin. 61 202801
Google Scholar
[18] John Z, Herman H, Albert G 1966 Radiation Trapped (Dordrech: Springer) p671
[19] Douglas S H 1982 J. Comput. Phys. 47 452
Google Scholar
[20] Brecht S H, Orens J H 1983 Study of Field Aligned Plasma Acceleration during a HANE Ad-A141340
[21] Thomas V A, Brecht S H 1986 Phys. Fluids 29 2444
Google Scholar
[22] Morrow D P 2014 Master Dissertation (Calhoun: Institutional Archive of the Naval Postgraduate School)
[23] Holmstrom M 2013 ASP Conference Series 474 202
[24] Thomas V A, Brecht S H 1987 J. Geophys. Res. 92 2289
Google Scholar
[25] Bai X N, Caprioli D, Sironi L, Spitkovsky A 2015 Astrophys. J. 809 55
Google Scholar
[26] Dan H H, Alfred M K, Austin A O 1977 Physics of High-altitude Nuclear Burst Effects DNA 4501F
[27] James M S, Thomas A G 2008 Astrophys. J. Suppl. Ser. 178 137
Google Scholar
[28] 王建国, 牛胜利, 张殿辉 2010 高空核爆炸效应参数手册 (北京: 原子能出版社) 第37页
Wang J G, Niu S L, Zhang D H 2010 Parameter Hand Book of High Attitude Nuclear Detonation Effects (Bejing: Atomic Energy Press) p37 (in Chinese)
DownLoad:
Catalog
Metrics
- Abstract views: 5723
- PDF Downloads: 97
- Cited By: 0
