聚焦离子束加工的硅酸钇波导中铕离子的光学与超精细跃迁

肖懿鑫; 朱天翔; 梁澎军; 王奕洋; 周宗权; 李传锋

doi:10.7498/aps.73.20241070

摘要

量子存储是大尺度量子网络的重要组成部分, 基于波导等微纳结构的可集成量子存储可以提供更好的可扩展性并实现更低的光电能耗. 在众多量子存储候选介质中, ¹⁵¹Eu³⁺:Y₂SiO₅晶体具有长达6 h的自旋相干寿命和1 h的相干光存储时间, 成为长寿命存储的优异候选材料. 本文通过聚焦离子束在¹⁵¹Eu³⁺:Y₂SiO₅晶体表面加工出三角形悬梁臂波导, 波导截面的边长为2 µm, 长度为20 µm, 并对三角形悬梁臂波导中的¹⁵¹Eu³⁺离子的⁷F₀—⁵D₀光学跃迁以及⁷F₀基态的超精细跃迁开展了研究. 结果显示, 在2 µm尺度的悬梁臂波导中¹⁵¹Eu³⁺离子基本保持了和块状晶体中¹⁵¹Eu³⁺离子一致的跃迁展宽及相干寿命, 可以支持量子存储任务的实现. 该工作为实现纳米尺度的¹⁵¹Eu³⁺离子可集成量子存储器以及单个¹⁵¹Eu³⁺离子的探测打下基础.

关键词:

Abstract

Quantum memory is a crucial element in large-scale quantum networks. Integrated quantum memories based on micro-/-nano structures, such as waveguides, can significantly enhance the scalability and reduce the consumption of optical and electrical power. ¹⁵¹Eu³⁺:Y₂SiO₅ stands out as an exceptional candidate material for quantum memory, because it possesses a spin coherence lifetime of 6 h and an optical storage lifetime of 1 h. Here we employ focused ion beam technology to fabricate a triangular nanobeam on the surface of a Y₂SiO₅ crystal. The width and length of the nanobeam are 2 μm and 20 μm, respectively. The optical lifetime and inhomogeneous broadening of ¹⁵¹Eu³⁺ in the triangular nanobeam are measured by fluorescence spectroscopy. The optical lifetime is (1.9±0.1) ms and the optical inhomogeneous broadening is (1.58±0.05) GHz at a doping level of 0.07% for ¹⁵¹Eu³⁺. The hyperfine transition spectra are measured by using optically detected magnetic resonance and spin inhomogeneous broadening of (19±3) kHz is obtained. Furthermore, we analyze the coherence properties of optical and hyperfine transitions, respectively, via transient spectral hole burning and spin echo measurement. We obtain an optical homogeneous linewidth down to (22±3) kHz, which is still limited by the instantaneous spectral diffusion. The spin coherence lifetime under the geomagnetic field is (5.1±0.6) ms. The results demonstrate that ¹⁵¹Eu³⁺ ions embedded within the 2 μm triangular nanobeam essentially retain the same optical and hyperfine transition properties as those observed in bulk crystals. Consequently, this research lays a foundation for studying the integrated quantum memories based on ¹⁵¹Eu³⁺ ensembles and the detection of the single ¹⁵¹Eu³⁺ ion based on the focused ion beam technique.

Keywords:

作者及机构信息

1.
中国科学技术大学, 中国科学院量子信息重点实验室, 合肥　230026

2.
中国科学技术大学, 量子网络安徽省重点实验室, 合肥　230026

3.
合肥国家实验室, 合肥 230088

通信作者: 周宗权, zq_zhou@ustc.edu.cn ; 李传锋, cfli@ustc.edu.cn

基金项目: 科技创新2030—“量子通信与量子计算机”重大项目(批准号: 2021ZD0301200)和国家自然科学基金(批准号: 12222411, 11821404)资助的课题.

Authors and contacts

1.
CAS Key Laboratory of Quantum Information, University of Science and Technology of China, Hefei 230026, China

2.
Anhui Province Key Laboratory of Quantum Network, University of Science and Technology of China, Hefei 230026, China

3.
Hefei National Laboratory, Hefei 230088, China

Corresponding author: Zhou Zong-Quan, zq_zhou@ustc.edu.cn ; Li Chuan-Feng, cfli@ustc.edu.cn

Funds: Project supported by the Innovation Program for Quantum Science and Technology (Grant No. 2021ZD0301200) and the National Natural Science Foundation of China (Grant Nos. 12222411, 11821404).

文章全文 : translate this paragraph

参考文献

[1]	Lukin M D 2003 Rev. Mod. Phys. 75 457 Google Scholar
[2]	Zhou Z, Liu C, Li C, Guo G, Oblak D, Lei M, Faraon A, Mazzera M, De Riedmatten H 2023 Laser Photonics Rev. 17 2300257 Google Scholar
[3]	Lei Y, Kimiaee Asadi F, Zhong T, Kuzmich A, Simon C, Hosseini M 2023 Optica 10 1511 Google Scholar
[4]	Briegel H J, Dür W, Cirac J I, Zoller P 1998 Phys. Rev. Lett. 81 5932 Google Scholar
[5]	Sangouard N, Simon C, de Riedmatten H, Gisin N 2011 Rev. Mod. Phys. 83 33 Google Scholar
[6]	Cirac J I, Zoller P, Kimble H J, Mabuchi H 1997 Phys. Rev. Lett. 78 3221 Google Scholar
[7]	Kimble H J 2008 Nature 453 1023 Google Scholar
[8]	Lvovsky A I, Sanders B C, Tittel W 2009 Nat. Photonics 3 706 Google Scholar
[9]	Hong C K, Mandel L 1986 Phys. Rev. Lett. 56 58 Google Scholar
[10]	Nunn J, Reim K, Lee K C, Lorenz V O, Sussman B J, Walmsley I A, Jaksch D 2008 Phys. Rev. Lett. 101 260502 Google Scholar
[11]	Davidson O, Yogev O, Poem E, Firstenberg O 2023 Phys. Rev. Lett. 131 033601 Google Scholar
[12]	Imamoḡlu A 2002 Phys. Rev. Lett. 89 163602 Google Scholar
[13]	Clausen C, Sangouard N, Drewsen M 2013 New J. Phys. 15 025021 Google Scholar
[14]	Liu X, Hu X M, Zhu T X, Zhang C, Xiao Y X, Miao J L, Ou Z W, Li P Y, Liu B H, Zhou Z Q, Li C F, Guo G C 2024 Nat. Commun. 15 8529 Google Scholar
[15]	Thiel C, Böttger T, Cone R 2011 J. Lumin. 131 353 Google Scholar
[16]	De Riedmatten H, Afzelius M, Staudt M U, Simon C, Gisin N 2008 Nature 456 773 Google Scholar
[17]	Longdell J J, Alexander A L, Sellars M J 2006 Phys. Rev. B 74 195101 Google Scholar
[18]	Fraval E, Sellars M J, Longdell J J 2004 Phys. Rev. Lett. 92 077601 Google Scholar
[19]	Zhong M, Hedges M P, Ahlefeldt R L, Bartholomew J G, Beavan S E, Wittig S M, Longdell J J, Sellars M J 2015 Nature 517 177 Google Scholar
[20]	Rančić M, Hedges M P, Ahlefeldt R L, Sellars M J 2018 Nature Phys. 14 50 Google Scholar
[21]	Ortu A, Holzäpfel A, Etesse J, Afzelius M 2022 npj Quantum Inf. 8 29 Google Scholar
[22]	Ma Y, Ma Y Z, Zhou Z Q, Li C F, Guo G C 2021 Nat. Commun. 12 2381 Google Scholar
[23]	Saglamyurek E, Sinclair N, Jin J, Slater J A, Oblak D, Bussières F, George M, Ricken R, Sohler W, Tittel W 2011 Nature 469 512 Google Scholar
[24]	Usmani I, Afzelius M, De Riedmatten H, Gisin N 2010 Nat. Commun. 1 12 Google Scholar
[25]	Businger M, Nicolas L, Mejia T S, Ferrier A, Goldner P, Afzelius M 2022 Nat. Commun. 13 6438 Google Scholar
[26]	Yang T S, Zhou Z Q, Hua Y L, Liu X, Li Z F, Li P Y, Ma Y, Liu C, Liang P J, Li X, Xiao Y X, Hu J, Li C F, Guo G C 2018 Nat. Commun. 9 3407 Google Scholar
[27]	Seri A, Lago-Rivera D, Lenhard A, Corrielli G, Osellame R, Mazzera M, de Riedmatten H 2019 Phys. Rev. Lett. 123 080502 Google Scholar
[28]	Chen F, De Aldana J R V 2014 Laser Photonics Rev. 8 251 Google Scholar
[29]	Liu C, Zhu T X, Su M X, Ma Y Z, Zhou Z Q, Li C F, Guo G C 2020 Phys. Rev. Lett. 125 260504 Google Scholar
[30]	Zhu T X, Liu C, Zheng L, Zhou Z Q, Li C F, Guo G C 2020 Phys. Rev. Appl. 14 054071 Google Scholar
[31]	Zhu T X, Liu C, Jin M, Su M X, Liu Y P, Li W J, Ye Y, Zhou Z Q, Li C F, Guo G C 2022 Phys. Rev. Lett. 128 180501 Google Scholar
[32]	Liu D C, Li P Y, Zhu T X, Zheng L, Huang J Y, Zhou Z Q, Li C F, Guo G C 2022 Phys. Rev. Lett. 129 210501 Google Scholar
[33]	Zhong T, Kindem J M, Miyazono E, Faraon A 2015 Nat. Commun. 6 8206 Google Scholar
[34]	Miyazono E, Zhong T, Craiciu I, Kindem J M, Faraon A 2016 Appl. Phys. Lett. 108 011111 Google Scholar
[35]	Zhong T, Rochman J, Kindem J M, Miyazono E, Faraon A 2016 Opt. Express 24 536 Google Scholar
[36]	Kindem J M, Ruskuc A, Bartholomew J G, Rochman J, Huan Y Q, Faraon A 2020 Nature 580 201 Google Scholar
[37]	Dibos A M, Raha M, Phenicie C M, Thompson J D 2018 Phys. Rev. Lett. 120 243601 Google Scholar
[38]	Weiss L, Gritsch A, Merkel B, Reiserer A 2021 Optica 8 40 Google Scholar
[39]	Zhu T X, Su M X, Liu C, Liu Y P, Wang C F, Liu P X, Han Y J, Zhou Z Q, Li C F, Guo G C 2024 National Sci. Rev. 161 Google Scholar
[40]	Bayn I, Meyler B, Salzman J, Kalish R 2011 New J. Phys. 13 025018 Google Scholar
[41]	Zhong T, Kindem J M, Bartholomew J G, Rochman J, Craiciu I, Verma V, Nam S W, Marsili F, Shaw M D, Beyer A D, Faraon A 2018 Phys. Rev. Lett. 121 183603 Google Scholar
[42]	Zhong T, Kindem J M, Bartholomew J G, Rochman J, Craiciu I, Miyazono E, Bettinelli M, Cavalli E, Verma V, Nam S W, Marsili F, Shaw M D, Beyer A D, Faraon A 2017 Science 357 1392 Google Scholar
[43]	Craiciu I, Lei M, Rochman J, Kindem J M, Bartholomew J G, Miyazono E, Zhong T, Sinclair N, Faraon A 2019 Phys. Rev. Appl. 12 024062 Google Scholar
[44]	梁澎军, 朱天翔, 肖懿鑫, 王奕洋, 韩永建, 周宗权, 李传锋 2024 73 100301 Google Scholar Liang P J, Zhu T X, Xiao Y X, Wang Y Y, Han Y J, Zhou Z Q, Li C F 2024 Acta Phys. Sin. 73 100301 Google Scholar
[45]	Könz F, Sun Y, Thiel C W, Cone R L, Equall R W, Hutcheson R L, Macfarlane R M 2003 Phys. Rev. B 68 085109 Google Scholar
[46]	Stoneham A M 1969 Rev. Mod. Phys. 41 82 Google Scholar
[47]	Lafitte-Houssat E, Ferrier A, Welinski S, Morvan L, Afzelius M, Berger P, Goldner P 2022 Opt. Mater.: X 14 100153 Google Scholar
[48]	Louchet-Chauvet A, Ahlefeldt R, Chanelière T 2019 Rev. Sci. Instrum. 90 034901 Google Scholar
[49]	Gritsch A, Weiss L, Früh J, Rinner S, Reiserer A 2022 Phys. Rev. X 12 041009 Google Scholar
[50]	Bartholomew J G, de Oliveira Lima K, Ferrier A, Goldner P 2017 Nano Lett. 17 778 Google Scholar
[51]	Szabo A 1975 Phys. Rev. B 11 4512 Google Scholar
[52]	Völker S 1989 Annu. Rev. Phys. Chem. 40 499 Google Scholar
[53]	Reiserer A 2022 Rev. Mod. Phys. 94 041003 Google Scholar
[54]	Meiboom S, Gill D 1958 Rev. Sci. Instrum. 29 688 Google Scholar
[55]	Arcangeli A, Lovrić M, Tumino B, Ferrier A, Goldner P 2014 Phys. Rev. B 89 184305 Google Scholar
[56]	Robledo L, Bernien H, van Weperen I, Hanson R 2010 Phys. Rev. Lett. 105 177403 Google Scholar
[57]	Ma Y Z, Lü Y C, Yang T S, Ma Y, Zhou Z Q, Li C F, Guo G C 2023 Phys. Rev. B 107 014310 Google Scholar
[58]	Alexander A L, Longdell J J, Sellars M J 2007 J. Opt. Soc. Am. B: Opt. Phys. 24 2479 Google Scholar
[59]	Hahn E L 1950 Phys. Rev. 80 580 Google Scholar

施引文献

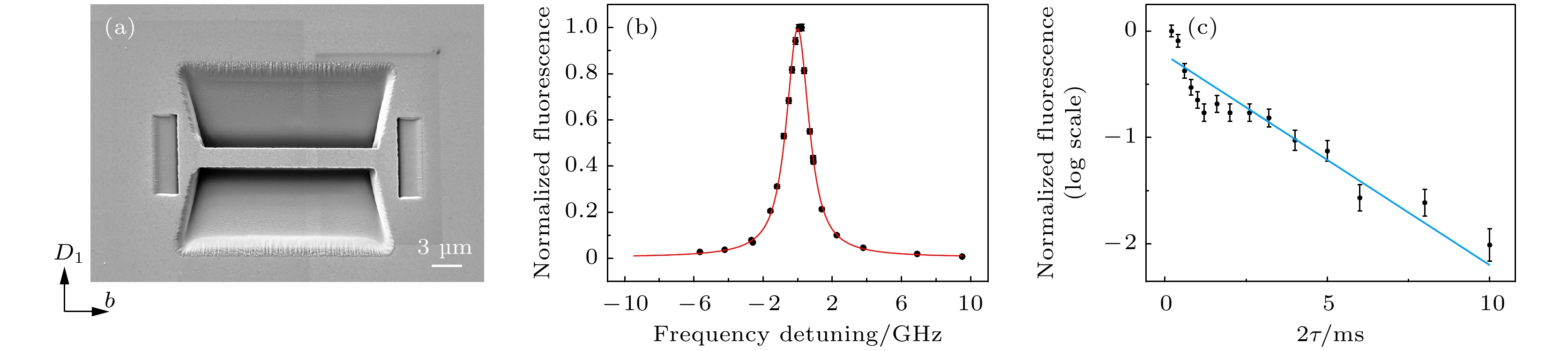
图 1 (a)浓度为0.07%的¹⁵¹Eu³⁺:Y₂SiO₅晶体经过聚焦离子束加工后的扫描电子显微镜图像, 在晶体D₁×b面的俯视图中, 三角形悬梁臂波导的宽度沿D₁轴为2 µm, 长度沿b轴为20 µm, 悬梁臂的输入输出端面为45°斜面. (b) Y₂SiO₅晶体中的site-1 ¹⁵¹Eu³⁺离子在地磁场环境下的基态⁷F₀和激发态⁵D₀的超精细能级结构图

Fig. 1. (a) Scanning electron microscope (SEM) image of ¹⁵¹Eu³⁺:Y₂SiO₅ crystal (doping level: 0.07%) fabricated by focused ion beam milling. In the top view of the D₁×b plane, the width (D₁ axis) and length (b axis) of the triangular nanobeam structure are 2 µm and 20 µm, respectively. Two slopes at 45° relative to the surface of the crystal are milled at both ends of the triangular nanobeam. (b) Energy-level diagram for the ground state ⁷F₀ and the excited state ⁵D₀ of site-1 ¹⁵¹Eu³⁺ ions in Y₂SiO₅ crystal at the geomagnetic field.

下载: 全尺寸图片幻灯片

图 2 实验装置图. 黄色直线代表580 nm激光的路径, 蓝色曲线代表单模光纤, PBS是偏振分束器, BS是非偏振分束器, AOM是声光调制器, AWG是任意波形发生器, SPD是单光子探测器, $ \lambda/2 $是半波片. 激光通过调制光路再由单模光纤传输到耦合光路, 虚线方框以内的部分为笼式系统, 其中发散的白光源(diffuse source)经过BS₂照射到晶体表面. 蓝色阴影区代表低温恒温器, 内部装有待测样品与亥姆霍兹线圈. 样品出射的信号光再一次经过笼式系统, 在BS₁另一端通过狭缝(slit). 可以通过可翻转的反射镜(flip mirror)反射光场在相机上观察成像, 也可以通过AOM光开关后由SPD进行探测

Fig. 2. Experimental setup. The yellow line represents the transmission path for 580-nm laser and the blue curve represents a single-mode fiber. PBS, polarization beam splitter; BS, beam splitter; AOM, acousto-optic modulator; AWG, arbitrary waveform generator; SPD, single photon detector; $ \lambda/2 $ represents a half-wave plate. The 580-nm laser is modulated by AOMs and collected with a single-mode fiber. The cage system within the dotted box includes a diffuse source which is a white-light source to illuminate the crystal surface through BS₂. The blue shadowed area indicates a cryostat with the sample and Helmholtz coils inside. The signal light emitted from the sample is transmitted through the cage system again and spatially filtered by a slit at the another side of BS₁. If the flip mirror is turned on then the sample can be imaged on the camera, otherwise, the signal is detected by a SPD after being gated by a double-passed AOM.

下载: 全尺寸图片幻灯片

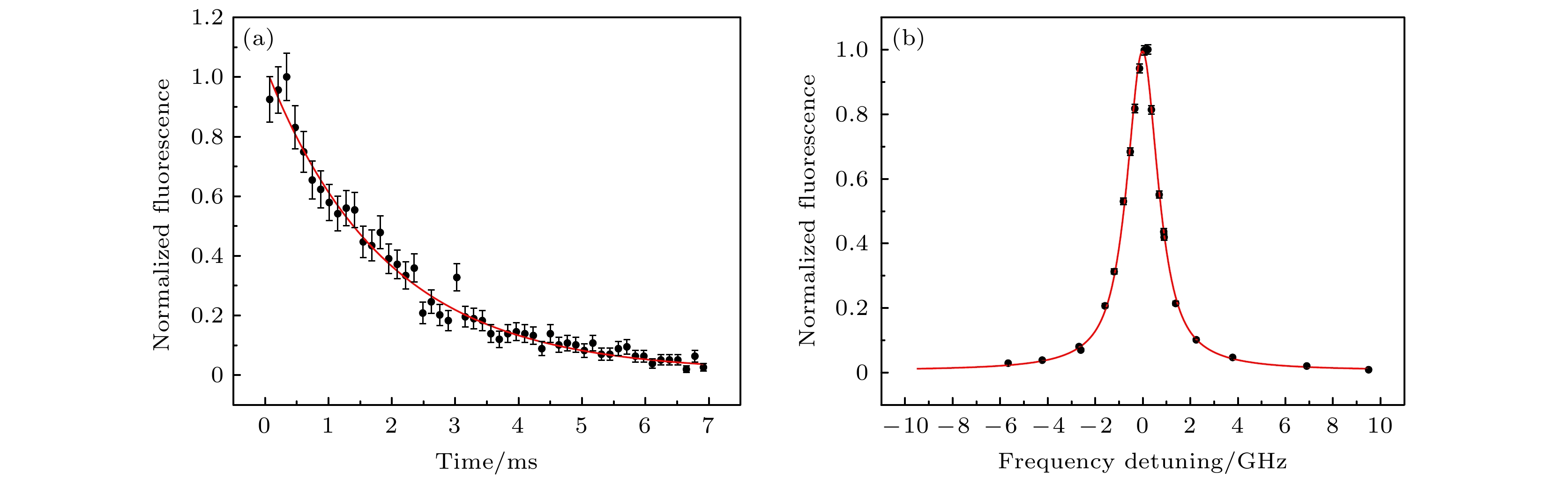
图 3 (a) Y₂SiO₅悬梁臂波导中¹⁵¹Eu³⁺的⁷F₀ $ \rightarrow $ ⁵D₀跃迁荧光寿命图. 黑色圆点为数据点, 红色曲线是拟合的指数衰减曲线, 误差条表示为一个标准差, 本文中误差条均代表同一含义. (b) Y₂SiO₅悬梁臂波导中¹⁵¹Eu³⁺的⁷F₀ $ \rightarrow $ ⁵D₀跃迁荧光激发谱. 黑色圆点为数据点, 红色曲线是由(1)式拟合的pseudo-Voigt型曲线, 中心频率点代表516.8478 THz

Fig. 3. (a) Fluorescence decay for the ⁷F₀ $ \rightarrow $ ⁵D₀ transition of ¹⁵¹Eu³⁺ in the Y₂SiO₅ nanobeam. The black dots are the fluorescence data and the red curve is fitted by a single exponential decay. Error bars indicate one standard deviation with the same meaning in whole paper. (b) Fluorescence excitation spectrum for the ⁷F₀ $ \rightarrow $ ⁵D₀ transition of ¹⁵¹Eu³⁺ in the Y₂SiO₅ nanobeam. The black dots are fluorescence data, and the red curve is fitted by pseudo-Voigt function according to Eq.(1). The center frequency is 516.8478 THz.

下载: 全尺寸图片幻灯片

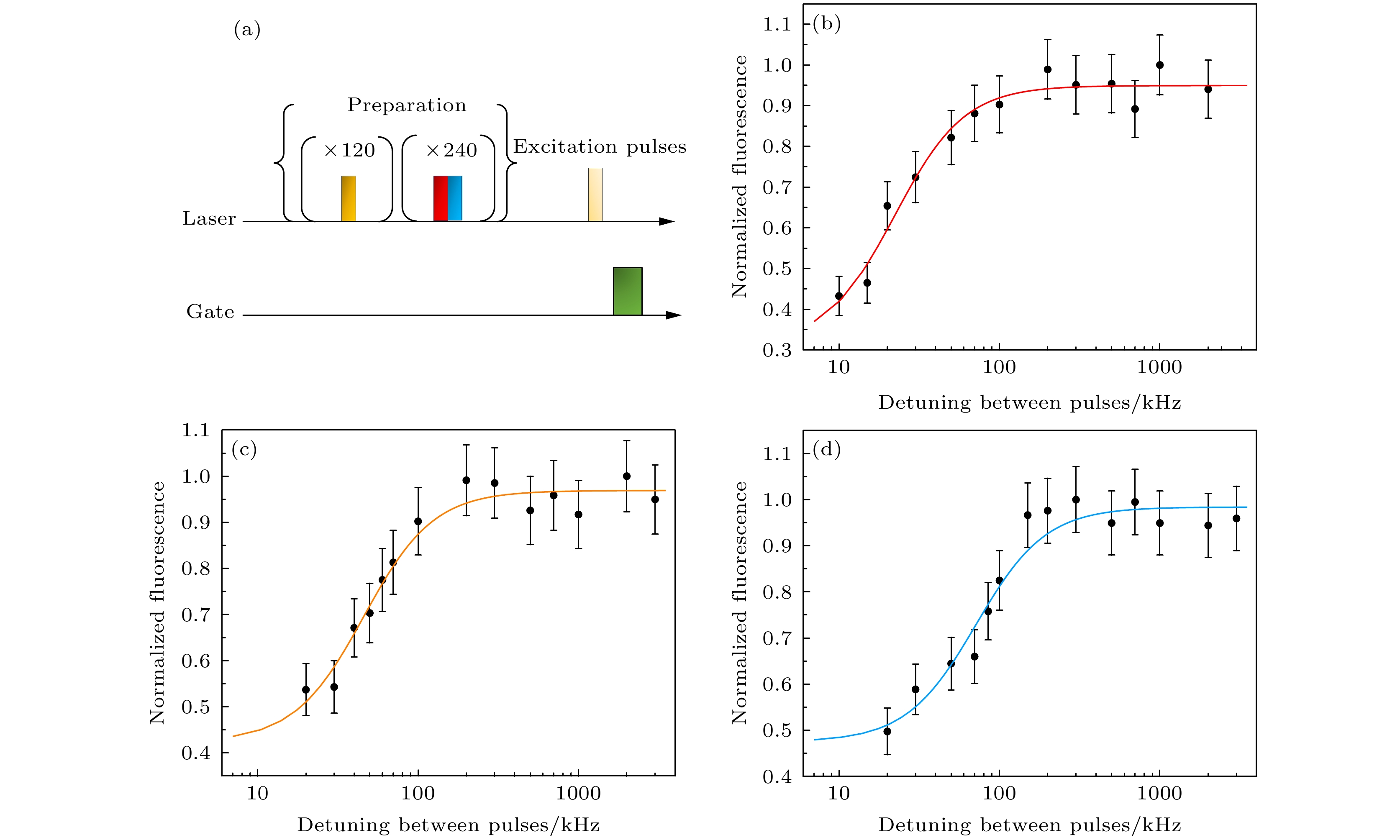
图 4 (a)测试Y₂SiO₅悬梁臂波导中¹⁵¹Eu³⁺光学均匀线宽的脉冲时序图. 首先是准备阶段, 其目的是为了初始化布居数. 在准备阶段结束40 ms后, 同时输入三束功率和频率间隔一致且时间宽度0.15 ms的高斯单频激发光. 在10 µs后, 光开关打开一个2 ms的窗口用于测量荧光信号. (b)—(d) 220 nW (b), 440 nW (c)和660 nW (d)的激发光下, 荧光信号随激发光的频率间隔的变化曲线图. 不同颜色的曲线是拟合的洛伦兹曲线

Fig. 4. (a) Pulse sequence for measuring the optical homogeneous linewidth of ¹⁵¹Eu³⁺ in the Y₂SiO₅ nanobeam. Preparation process initializes the atomic population for each repeat of measurements. 40 ms after the preparation process, we apply the excitation pulses which are three Gaussian pulses with a duration of 0.15 ms with equal frequency spacing. 10 µs after the excitation, the detection gate opens for 2 ms to detect the fluorescence signal. (b)–(d) Fluorescence signal depending on the frequency spacing of excitation pulses, with excitation powers of 220 nW (b), 440 nW (c) and 660 nW (d). Solid lines are fitted in accordance with Lorentzian lineshape.

下载: 全尺寸图片幻灯片

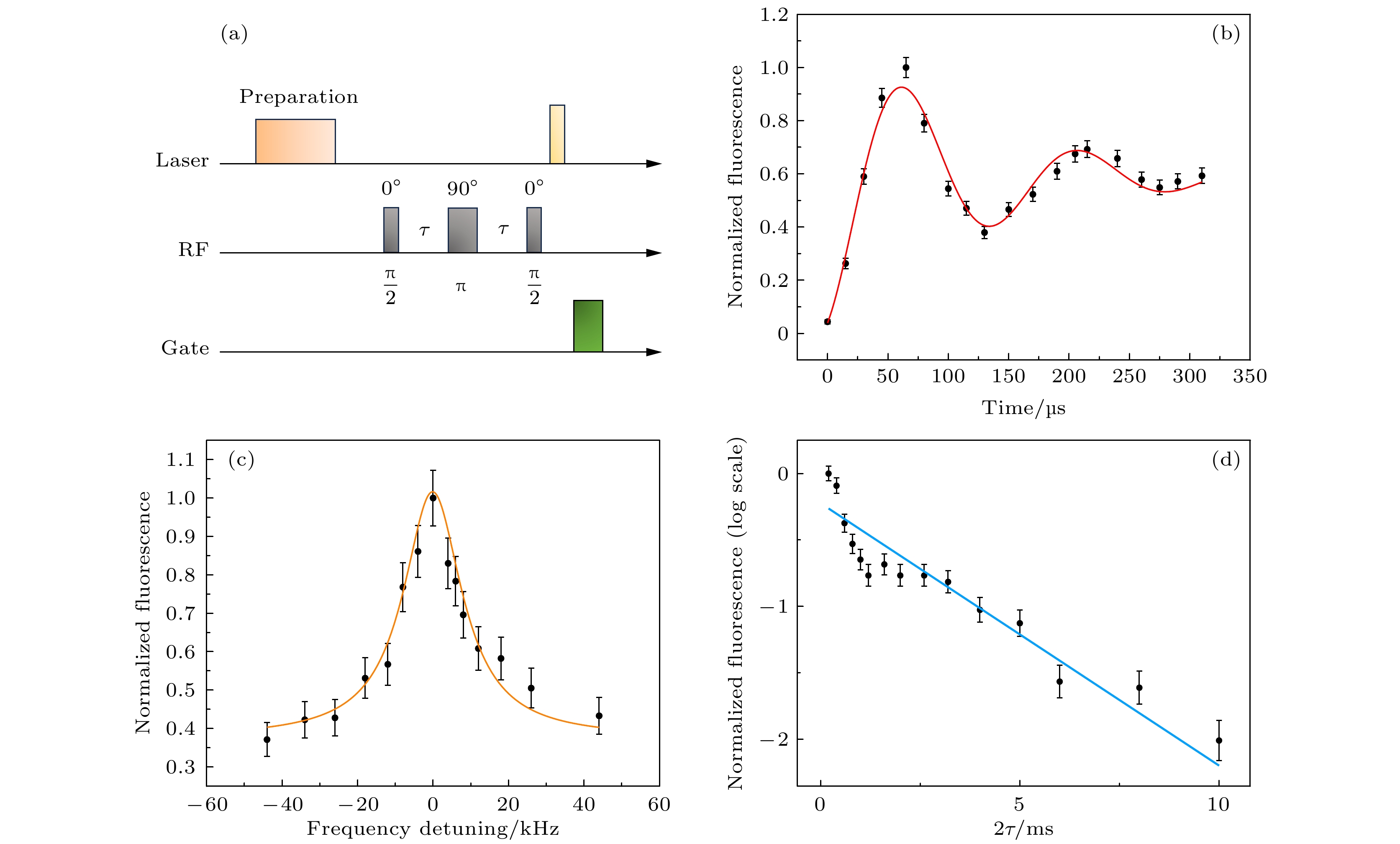
图 5 (a)测试Y₂SiO₅悬梁臂波导中¹⁵¹Eu³⁺离子的自旋相干寿命的序列图. 在准备阶段后, 通过线圈施加$ \pi/2-\pi-\pi/2 $的自旋回波(spin echo)脉冲序列, 每个射频脉冲的时间间隔τ相等, 射频脉冲的相位依次为$ 0^\circ-90^\circ-0^\circ $. 射频脉冲结束后, 施加一个啁啾带宽3 MHz和时间宽度500 µs的啁啾型光脉冲, 并随后探测2 ms的荧光信号; (b) Y₂SiO₅悬梁臂波导中¹⁵¹Eu³⁺离子$ |{\pm1/2}\rangle_{\mathrm{g}} $ $ \leftrightarrow $$ |{\pm3/2}\rangle_{\mathrm{g}} $的spin nutation测试. 红色曲线是拟合的阻尼正弦函数曲线; (c) Y₂SiO₅悬梁臂波导中¹⁵¹Eu³⁺离子$ |{\pm1/2}\rangle_{\mathrm{g}} $ $ \leftrightarrow $ $ |{\pm3/2}\rangle_{\mathrm{g}} $的超精细跃迁谱, 橙色曲线是拟合的pseudo-Voigt型曲线; (d)荧光信号随自旋回波序列的总时间的变化图

Fig. 5. (a) Pulse sequence for measuring spin coherence lifetime of ¹⁵¹Eu³⁺ in the Y₂SiO₅ nanobeam. The spin-echo sequence (RF pulses $ \pi/2-\pi-\pi/2 $) are applied by the Helmholtz coils after the preparation process. The phases of the RF pulses are $ 0^\circ-90^\circ-0^\circ $. After the RF pulses, the sample is excited by a chirp pulse with a duration of 500 µs and a bandwidth of 3 MHz, and then fluorescence signal is detected with a 2-ms window. (b) Spin nutation measurement on the $ |{\pm1/2}\rangle_{\mathrm{g}} $ $ \leftrightarrow $ $ |{\pm3/2}\rangle_{\mathrm{g}} $ transition of ¹⁵¹Eu³⁺ in the Y₂SiO₅ nanobeam. The red curve is fitted by damped sine lineshape. (c) Fluorescence-detected spectrum for $ |{\pm1/2}\rangle_{\mathrm{g}} $ $ \leftrightarrow $ $ |{\pm3/2}\rangle_{\mathrm{g}} $ transition. The orange curve is fitted by pseudo-Voigt lineshape. (d) Fluorescence signal as a function of the total time of the spin-echo sequence.

下载: 全尺寸图片幻灯片

下载: 全尺寸图片幻灯片

map

[1]	Lukin M D 2003 Rev. Mod. Phys. 75 457 Google Scholar
[2]	Zhou Z, Liu C, Li C, Guo G, Oblak D, Lei M, Faraon A, Mazzera M, De Riedmatten H 2023 Laser Photonics Rev. 17 2300257 Google Scholar
[3]	Lei Y, Kimiaee Asadi F, Zhong T, Kuzmich A, Simon C, Hosseini M 2023 Optica 10 1511 Google Scholar
[4]	Briegel H J, Dür W, Cirac J I, Zoller P 1998 Phys. Rev. Lett. 81 5932 Google Scholar
[5]	Sangouard N, Simon C, de Riedmatten H, Gisin N 2011 Rev. Mod. Phys. 83 33 Google Scholar
[6]	Cirac J I, Zoller P, Kimble H J, Mabuchi H 1997 Phys. Rev. Lett. 78 3221 Google Scholar
[7]	Kimble H J 2008 Nature 453 1023 Google Scholar
[8]	Lvovsky A I, Sanders B C, Tittel W 2009 Nat. Photonics 3 706 Google Scholar
[9]	Hong C K, Mandel L 1986 Phys. Rev. Lett. 56 58 Google Scholar
[10]	Nunn J, Reim K, Lee K C, Lorenz V O, Sussman B J, Walmsley I A, Jaksch D 2008 Phys. Rev. Lett. 101 260502 Google Scholar
[11]	Davidson O, Yogev O, Poem E, Firstenberg O 2023 Phys. Rev. Lett. 131 033601 Google Scholar
[12]	Imamoḡlu A 2002 Phys. Rev. Lett. 89 163602 Google Scholar
[13]	Clausen C, Sangouard N, Drewsen M 2013 New J. Phys. 15 025021 Google Scholar
[14]	Liu X, Hu X M, Zhu T X, Zhang C, Xiao Y X, Miao J L, Ou Z W, Li P Y, Liu B H, Zhou Z Q, Li C F, Guo G C 2024 Nat. Commun. 15 8529 Google Scholar
[15]	Thiel C, Böttger T, Cone R 2011 J. Lumin. 131 353 Google Scholar
[16]	De Riedmatten H, Afzelius M, Staudt M U, Simon C, Gisin N 2008 Nature 456 773 Google Scholar
[17]	Longdell J J, Alexander A L, Sellars M J 2006 Phys. Rev. B 74 195101 Google Scholar
[18]	Fraval E, Sellars M J, Longdell J J 2004 Phys. Rev. Lett. 92 077601 Google Scholar
[19]	Zhong M, Hedges M P, Ahlefeldt R L, Bartholomew J G, Beavan S E, Wittig S M, Longdell J J, Sellars M J 2015 Nature 517 177 Google Scholar
[20]	Rančić M, Hedges M P, Ahlefeldt R L, Sellars M J 2018 Nature Phys. 14 50 Google Scholar
[21]	Ortu A, Holzäpfel A, Etesse J, Afzelius M 2022 npj Quantum Inf. 8 29 Google Scholar
[22]	Ma Y, Ma Y Z, Zhou Z Q, Li C F, Guo G C 2021 Nat. Commun. 12 2381 Google Scholar
[23]	Saglamyurek E, Sinclair N, Jin J, Slater J A, Oblak D, Bussières F, George M, Ricken R, Sohler W, Tittel W 2011 Nature 469 512 Google Scholar
[24]	Usmani I, Afzelius M, De Riedmatten H, Gisin N 2010 Nat. Commun. 1 12 Google Scholar
[25]	Businger M, Nicolas L, Mejia T S, Ferrier A, Goldner P, Afzelius M 2022 Nat. Commun. 13 6438 Google Scholar
[26]	Yang T S, Zhou Z Q, Hua Y L, Liu X, Li Z F, Li P Y, Ma Y, Liu C, Liang P J, Li X, Xiao Y X, Hu J, Li C F, Guo G C 2018 Nat. Commun. 9 3407 Google Scholar
[27]	Seri A, Lago-Rivera D, Lenhard A, Corrielli G, Osellame R, Mazzera M, de Riedmatten H 2019 Phys. Rev. Lett. 123 080502 Google Scholar
[28]	Chen F, De Aldana J R V 2014 Laser Photonics Rev. 8 251 Google Scholar
[29]	Liu C, Zhu T X, Su M X, Ma Y Z, Zhou Z Q, Li C F, Guo G C 2020 Phys. Rev. Lett. 125 260504 Google Scholar
[30]	Zhu T X, Liu C, Zheng L, Zhou Z Q, Li C F, Guo G C 2020 Phys. Rev. Appl. 14 054071 Google Scholar
[31]	Zhu T X, Liu C, Jin M, Su M X, Liu Y P, Li W J, Ye Y, Zhou Z Q, Li C F, Guo G C 2022 Phys. Rev. Lett. 128 180501 Google Scholar
[32]	Liu D C, Li P Y, Zhu T X, Zheng L, Huang J Y, Zhou Z Q, Li C F, Guo G C 2022 Phys. Rev. Lett. 129 210501 Google Scholar
[33]	Zhong T, Kindem J M, Miyazono E, Faraon A 2015 Nat. Commun. 6 8206 Google Scholar
[34]	Miyazono E, Zhong T, Craiciu I, Kindem J M, Faraon A 2016 Appl. Phys. Lett. 108 011111 Google Scholar
[35]	Zhong T, Rochman J, Kindem J M, Miyazono E, Faraon A 2016 Opt. Express 24 536 Google Scholar
[36]	Kindem J M, Ruskuc A, Bartholomew J G, Rochman J, Huan Y Q, Faraon A 2020 Nature 580 201 Google Scholar
[37]	Dibos A M, Raha M, Phenicie C M, Thompson J D 2018 Phys. Rev. Lett. 120 243601 Google Scholar
[38]	Weiss L, Gritsch A, Merkel B, Reiserer A 2021 Optica 8 40 Google Scholar
[39]	Zhu T X, Su M X, Liu C, Liu Y P, Wang C F, Liu P X, Han Y J, Zhou Z Q, Li C F, Guo G C 2024 National Sci. Rev. 161 Google Scholar
[40]	Bayn I, Meyler B, Salzman J, Kalish R 2011 New J. Phys. 13 025018 Google Scholar
[41]	Zhong T, Kindem J M, Bartholomew J G, Rochman J, Craiciu I, Verma V, Nam S W, Marsili F, Shaw M D, Beyer A D, Faraon A 2018 Phys. Rev. Lett. 121 183603 Google Scholar
[42]	Zhong T, Kindem J M, Bartholomew J G, Rochman J, Craiciu I, Miyazono E, Bettinelli M, Cavalli E, Verma V, Nam S W, Marsili F, Shaw M D, Beyer A D, Faraon A 2017 Science 357 1392 Google Scholar
[43]	Craiciu I, Lei M, Rochman J, Kindem J M, Bartholomew J G, Miyazono E, Zhong T, Sinclair N, Faraon A 2019 Phys. Rev. Appl. 12 024062 Google Scholar
[44]	梁澎军, 朱天翔, 肖懿鑫, 王奕洋, 韩永建, 周宗权, 李传锋 2024 73 100301 Google Scholar Liang P J, Zhu T X, Xiao Y X, Wang Y Y, Han Y J, Zhou Z Q, Li C F 2024 Acta Phys. Sin. 73 100301 Google Scholar
[45]	Könz F, Sun Y, Thiel C W, Cone R L, Equall R W, Hutcheson R L, Macfarlane R M 2003 Phys. Rev. B 68 085109 Google Scholar
[46]	Stoneham A M 1969 Rev. Mod. Phys. 41 82 Google Scholar
[47]	Lafitte-Houssat E, Ferrier A, Welinski S, Morvan L, Afzelius M, Berger P, Goldner P 2022 Opt. Mater.: X 14 100153 Google Scholar
[48]	Louchet-Chauvet A, Ahlefeldt R, Chanelière T 2019 Rev. Sci. Instrum. 90 034901 Google Scholar
[49]	Gritsch A, Weiss L, Früh J, Rinner S, Reiserer A 2022 Phys. Rev. X 12 041009 Google Scholar
[50]	Bartholomew J G, de Oliveira Lima K, Ferrier A, Goldner P 2017 Nano Lett. 17 778 Google Scholar
[51]	Szabo A 1975 Phys. Rev. B 11 4512 Google Scholar
[52]	Völker S 1989 Annu. Rev. Phys. Chem. 40 499 Google Scholar
[53]	Reiserer A 2022 Rev. Mod. Phys. 94 041003 Google Scholar
[54]	Meiboom S, Gill D 1958 Rev. Sci. Instrum. 29 688 Google Scholar
[55]	Arcangeli A, Lovrić M, Tumino B, Ferrier A, Goldner P 2014 Phys. Rev. B 89 184305 Google Scholar
[56]	Robledo L, Bernien H, van Weperen I, Hanson R 2010 Phys. Rev. Lett. 105 177403 Google Scholar
[57]	Ma Y Z, Lü Y C, Yang T S, Ma Y, Zhou Z Q, Li C F, Guo G C 2023 Phys. Rev. B 107 014310 Google Scholar
[58]	Alexander A L, Longdell J J, Sellars M J 2007 J. Opt. Soc. Am. B: Opt. Phys. 24 2479 Google Scholar
[59]	Hahn E L 1950 Phys. Rev. 80 580 Google Scholar

[1]	梁澎军, 朱天翔, 肖懿鑫, 王奕洋, 韩永建, 周宗权, 李传锋. 浓度依赖的掺铕硅酸钇晶体的光学和自旋非均匀展宽. , 2024, 73(10): 100301. doi: 10.7498/aps.73.20240116
[2]	王云飞, 周颖, 王英, 颜辉, 朱诗亮. 量子存储性能及应用分析. , 2023, 72(20): 206701. doi: 10.7498/aps.72.20231203
[3]	郭牧城, 汪福东, 胡肇高, 任苗苗, 孙伟业, 肖婉婷, 刘书萍, 钟满金. 微纳尺度稀土掺杂晶体的量子相干性能及其应用研究进展. , 2023, 72(12): 120302. doi: 10.7498/aps.72.20222166
[4]	周宗权. 量子存储式量子计算机与无噪声光子回波. , 2022, 71(7): 070305. doi: 10.7498/aps.71.20212245
[5]	邢雪燕, 李霞霞, 陈宇辉, 张向东. 基于光子晶体微腔的回波光量子存储. , 2022, 71(11): 114201. doi: 10.7498/aps.71.20220083
[6]	周湃, 李霞霞, 邢雪燕, 陈宇辉, 张向东. 基于掺铒晶体的光量子存储和调控. , 2022, 71(6): 064203. doi: 10.7498/aps.71.20211803
[7]	李宗峰, 刘端程, 周宗权, 李传锋. 基于EuCl₃·6H₂O晶体的光存储. , 2021, 70(16): 160302. doi: 10.7498/aps.70.20210648
[8]	杨天书, 周宗权, 李传锋, 郭光灿. 多模式固态量子存储. , 2019, 68(3): 030303. doi: 10.7498/aps.68.20182207
[9]	汪野, 张静宁, 金奇奂. 相干时间超过10 min的单离子量子比特. , 2019, 68(3): 030306. doi: 10.7498/aps.68.20181729
[10]	史保森, 丁冬生, 张伟, 李恩泽. 基于拉曼协议的量子存储. , 2019, 68(3): 034203. doi: 10.7498/aps.68.20182215
[11]	窦建鹏, 李航, 庞晓玲, 张超妮, 杨天怀, 金贤敏. 量子存储研究进展. , 2019, 68(3): 030307. doi: 10.7498/aps.68.20190039
[12]	李明, 陈阳, 郭光灿, 任希锋. 表面等离激元量子信息应用研究进展. , 2017, 66(14): 144202. doi: 10.7498/aps.66.144202
[13]	邓瑞婕, 闫智辉, 贾晓军. 基于电磁诱导透明机制的压缩光场量子存储. , 2017, 66(7): 074201. doi: 10.7498/aps.66.074201
[14]	孙颖, 赵尚弘, 东晨. 基于量子存储的长距离测量设备无关量子密钥分配研究. , 2015, 64(14): 140304. doi: 10.7498/aps.64.140304
[15]	邢莉娟, 李卓, 张武军. 加强的量子汉明限. , 2011, 60(5): 050304. doi: 10.7498/aps.60.050304
[16]	刘春旭, 张继森, 刘俊业, 金光. Er3+：YAlO3晶体中Λ型四能级系统的量子相干左手性. , 2009, 58(8): 5778-5783. doi: 10.7498/aps.58.5778
[17]	李卓, 邢莉娟. 量子Generalized Reed-Solomon码. , 2008, 57(1): 28-30. doi: 10.7498/aps.57.28
[18]	许兴胜, 熊志刚, 金爱子, 陈弘达, 张道中. 聚焦离子束研制半导体材料光子晶体. , 2007, 56(2): 916-921. doi: 10.7498/aps.56.916
[19]	许兴胜, 熊志刚, 孙增辉, 杜伟, 鲁琳, 陈弘达, 金爱子, 张道中. 半导体量子阱材料微加工光子晶体的光学特性. , 2006, 55(3): 1248-1252. doi: 10.7498/aps.55.1248
[20]	张权, 唐朝京, 高峰. 量子Turbo码. , 2002, 51(1): 15-20. doi: 10.7498/aps.51.15

计量

文章访问数: 373
PDF下载量: 14
被引次数: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

搜索

留言板

聚焦离子束加工的硅酸钇波导中铕离子的光学与超精细跃迁