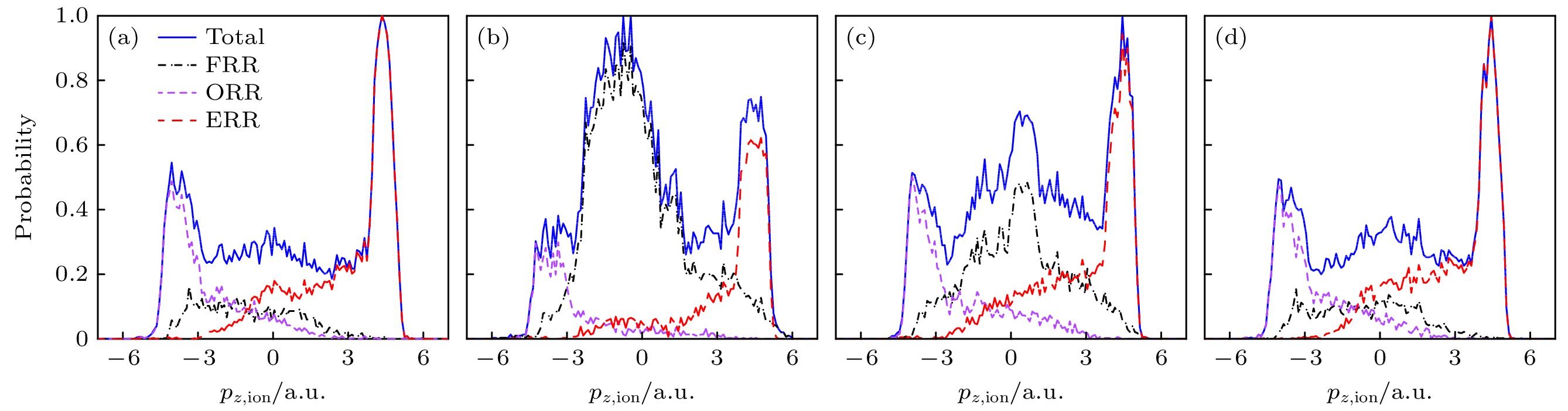
-
本文利用三维经典系综模型研究了平行偏振三色场中He原子的非次序双电离. 利用强度相同的1600 nm和800 nm脉冲组成驱动场, 低强度的400 nm脉冲作为控制场. 研究结果表明, 第一次返回碰撞轨道、奇数次返回碰撞轨道(不含第一次返回碰撞)和偶数次返回碰撞轨道释放的电子对和离子分别处在电子关联动量谱和离子动量谱上的不同区域. 通过改变控制场的相位, 可以很好地控制不同返回次数碰撞轨道在双电离中的占比, 进而实现对电子动量分布和离子动量分布的控制. 另外, 多次返回碰撞导致的双电离以碰撞电离机制为主, 而第一次返回碰撞导致的双电离以碰撞激发电离机制为主, 所以通过改变控制场的相位也能实现对双电离机制的调控.Nonsequential double ionization (NSDI) of He atoms in a parallel polarized three-color field is investigated by using a three-dimensional classical ensemble model. The driving field is composed of 1600-nm and 800-nm laser pulses with equal intensity. A weak 400-nm laser pulse is used as a controlling field. The results indicate that in the correlated electron momentum distribution and ion momentum distribution, the electron pairs and ions of the first returning recollision (FRR) trajectory, the odd-returning recollision (ORR) trajectory (excluding FRR), and the even-returning recollision (ERR) trajectory are located in different regions separated well from each other. The electron pairs from FRR trajectories mainly distribute around the origin, and those electron pairs from ORR and ERR trajectories respectively cluster in the first quadrant and the third quadrant. With the increase of the phase of the controlling field, the proportion of FRR trajectories in NSDI first increases and then decreases, and the proportions of those trajectories with the returning number more than one first decrease and then increase, which leads to the fact that with the increase of the phase of the controlling field, the anticorrelated emissions first increase and then decrease and correspondingly the ion momentum distribution evolves from a double-hump to a triple-hump and then to a double-hump structure. Moreover, NSDI from multiple-returning recollision trajectories mainly occur through recollision-induced direct ionization (RDI) mechanism, while NSDI from the FRR trajectories mainly occurs through recollision-induced excitation with subsequent ionization (RESI) mechanism. Thus the dominant NSDI ionization mechanism can also be controlled by changing the phase of the controlling field.
-
Keywords:
- nonsequential double ionization /
- parallel polarized three-color field /
- recollision /
- ultrafast dynamics
[1] Fittinghoff D N, Bolton P R, Chang B, Kulander K C 1992 Phys. Rev. Lett. 69 2642
Google Scholar
[2] Becker W, Liu X, Jo Ho P, Eberly J H 2012 Rev. Mod. Phys. 84 1011
Google Scholar
[3] Wang X, Eberly J H 2010 Phys. Rev. Lett. 105 083001
Google Scholar
[4] Weber Th, Weckenbrock M, Staudte A, Spielberger L, Jagutzki O, Mergel V, Afaneh F, Urbasch G, Vollmer M, Giessen H, Dörner R 2000 Phys. Rev. Lett. 84 443
Google Scholar
[5] Chen J, Nam C H 2002 Phys. Rev. A 66 053415
Google Scholar
[6] Wang Y, Xu S, Quan W, Gong C, Lai X, Hu S, Liu M, Chen J, Liu X 2016 Phys. Rev. A 94 053412
Google Scholar
[7] Li H, Chen J, Jiang H, Liu J, Fu P, Gong Q, Yan Z, Wang B 2009 J. Phys. B 42 125601
Google Scholar
[8] Weber Th, Giessen H, Weckenbrock M, Urbasch G, Staudte A, Spielberger L, Jagutzki O, Mergel V, Vollmer M, Dörner R 2000 Nature 405 658
Google Scholar
[9] Zhou Y, Liao Q, Zhang Q, Hong W, Lu P 2010 Opt. Express 18 632
Google Scholar
[10] Corkum P B 1993 Phys. Rev. Lett. 71 1994
Google Scholar
[11] Kang H, Chen S, Wang Y, Chu W, Yao J, Chen J, Liu X, Cheng Y, Xu Z 2019 Phys. Rev. A 100 033403
Google Scholar
[12] Huang C, Zhong M, Wu Z 2019 Opt. Express 27 7616
Google Scholar
[13] Li Y, Wang X, Yu B, Tang Q, Wang G, Wan J 2016 Sci. Rep. 6 37413
Google Scholar
[14] Liu Y, Fu L, Ye D, Liu J, Li M, Wu C, Gong Q, Moshammer R, Ullrich J 2014 Phys. Rev. Lett. 112 013003
Google Scholar
[15] He T, Liu Z, Li Y, Yu B, Huang C 2024 Opt. Laser Technol. 178 111215
Google Scholar
[16] Liao Q, Winney A H, Lee S K, Lin Y F, Adhikari P, Li W 2017 Phys. Rev. A 96 023401
Google Scholar
[17] Ye D, Li M, Fu L, Liu J, Gong Q, Liu Y, Ullrich J 2015 Phys. Rev. Lett. 115 123001
Google Scholar
[18] Hao X, Chen J, Li W, Wang B, Wang X, Becker W 2014 Phys. Rev. Lett. 112 073002
Google Scholar
[19] Li X, Qiao Y, Wu D, Yu R, Chen J, Wang J, Guo F, Yang Y 2023 Chinese Phys. B 33 013302
Google Scholar
[20] Xu T, Zhu Q, Chen J, Ben S, Zhang J, Liu X 2018 Opt. Express 26 1645
Google Scholar
[21] Lin K, Jia X, Yu Z, He F, Ma J, Li H, Gong X, Song Q, Ji Q, Zhang W, Li H, Lu P, Zeng H, Chen J, Wu J 2017 Phys. Rev. Lett 119 203202
Google Scholar
[22] Dong S, Chen X, Zhang J, Ren X 2016 Phys. Rev. A 93 053410
Google Scholar
[23] Rudenko A, Jesus V L B, Ergler Th, Zrost K, Feuerstein B, Schröter C D, Moshammer R, Ullrich J 2007 Phys. Rev. Lett. 99 263003
Google Scholar
[24] Chen Z, Li S, Kang H, Morishita T, Bartschat K 2022 Opt. Express 30 44039
Google Scholar
[25] Chen X, Ruiz C, He F, Zhang J 2020 Opt. Express 28 14884
Google Scholar
[26] Huang C, Zhong M, Wu Z 2016 Opt. Express 24 28361
Google Scholar
[27] Ma X, Zhou Y, Li N, Li M, Lu P 2018 Opt. Laser Technol. 108 235
Google Scholar
[28] Luo S, Ma X, Xie H, Li M, Zhou Y, Cao W, Lu P 2018 Opt. Express 26 13666
Google Scholar
[29] He T, Liu Z, Liao J, Li Y, Yu B, Huang C 2024 Phys. Rev. A 109 043109
Google Scholar
[30] He L, Li Y, Zhang Q, Lu P 2013 Opt. Express 21 2683
Google Scholar
[31] Qin Y, Guo F, Li S, Yang Y, Chen G 2014 Chin. Phys. B 23 093205
Google Scholar
[32] Ho P J, Panfili R, Haan S L, Eberly J H 2005 Phys. Rev. Lett. 94 093002
Google Scholar
[33] Liu Z, Huang C, He T, Liao J, Li Y, Yu B 2024 Phys. Chem. Chem. Phys. 26 4572
Google Scholar
[34] Mauger F, Chandre C, Uzer T 2009 Phys. Rev. Lett. 102 173002
Google Scholar
[35] 廖健颖, 贺佟佟, 苏杰, 刘子超, 李盈傧, 余本海, 黄诚 2023 72 193202
Google Scholar
Liao J Y, He T T, Su J, Liu Z C, Li Y B, Yu B H, Huang C 2023 Acta Phys. Sin. 72 193202
Google Scholar
[36] 黄诚, 苏杰, 廖健颖, 刘子超, 贺佟佟, 李盈傧 2023 光子学报 52 1126003
Google Scholar
Huang C, Su J, Liao J Y, Liu Z C, He T T, Li Y B 2023 Acta Photonica Sin. 52 1126003
Google Scholar
[37] Tong A, Li Q, Ma X, Zhou Y, Lu P 2019 Opt. Express 27 6415
Google Scholar
-
图 1 φ = 0.8π时, 一个光周期内的激光电场波形
Fig. 1. Waveform of the laser electric field within an optical cycle for φ = 0.8π.
图 2 激光偏振方向上的关联电子动量分布 (a) φ = 0; (b) φ = 0.6π; (c) φ = 1.2π; (d) φ = 1.8π
Fig. 2. Correlated electron momentum distributions along the laser polarization direction: (a) φ = 0; (b) φ = 0.6π; (c) φ = 1.2π; (d) φ = 1.8π.
图 3 φ = 1.2π时, 前5次返回碰撞事件的关联电子动量分布
Fig. 3. Correlated electron momentum distributions of NSDI for those trajectories with return numbers from 1 to 5 for φ = 1.2π.
图 4 激光偏振方向上的离子动量分布 (a) φ = 0; (b) φ = 0.6π; (c) φ = 1.2π; (d) φ = 1.8π
Fig. 4. Ion momentum distributions along the laser polarization direction: (a) φ = 0; (b) φ = 0.6π; (c) φ = 1.2π; (d) φ = 1.8π.
图 5 前7次返回碰撞轨道在NSDI中的占比对控制场相位的依赖
Fig. 5. Proportions of those trajectories with different return numbers in NSDI as a function of the phase of the controlling pulse.
图 6 三类轨道(黑色, FRR轨道; 紫色, ORR轨道; 红色, ERR轨道)的单电离时间(a), (d), (g)、碰撞时间(b), (e), (h)和双电离时间分布(c), (f), (i) (a)—(c) φ = 0; (d)—(f) φ = 0.6π; (g)—(i) φ = 1.2π
Fig. 6. Time distributions of single ionization (a), (d), (g), recollision (b), (e), (h) and double ionization (c), (f), (i) for FRR trajectories (black bars), ORR trajectories (purple bars) and ERR trajectories (red bars): (a)–(c) φ = 0; (d)–(f) φ = 0.6π; (g)–(i) φ = 1.2π.
图 7 各类轨道中RDI机制的比例对控制场相位的依赖
Fig. 7. Proportions of RDI mechanism for different types of trajectories as a function of the phase of the controlling pulse.
图 8 三类轨道的返回能量分布 (a) φ = 0.8π; (b) φ = 1.2π
Fig. 8. Returning energy distributions for the three types of trajectories: (a) φ = 0.8π; (b) φ = 1.2π.
-
[1] Fittinghoff D N, Bolton P R, Chang B, Kulander K C 1992 Phys. Rev. Lett. 69 2642
Google Scholar
[2] Becker W, Liu X, Jo Ho P, Eberly J H 2012 Rev. Mod. Phys. 84 1011
Google Scholar
[3] Wang X, Eberly J H 2010 Phys. Rev. Lett. 105 083001
Google Scholar
[4] Weber Th, Weckenbrock M, Staudte A, Spielberger L, Jagutzki O, Mergel V, Afaneh F, Urbasch G, Vollmer M, Giessen H, Dörner R 2000 Phys. Rev. Lett. 84 443
Google Scholar
[5] Chen J, Nam C H 2002 Phys. Rev. A 66 053415
Google Scholar
[6] Wang Y, Xu S, Quan W, Gong C, Lai X, Hu S, Liu M, Chen J, Liu X 2016 Phys. Rev. A 94 053412
Google Scholar
[7] Li H, Chen J, Jiang H, Liu J, Fu P, Gong Q, Yan Z, Wang B 2009 J. Phys. B 42 125601
Google Scholar
[8] Weber Th, Giessen H, Weckenbrock M, Urbasch G, Staudte A, Spielberger L, Jagutzki O, Mergel V, Vollmer M, Dörner R 2000 Nature 405 658
Google Scholar
[9] Zhou Y, Liao Q, Zhang Q, Hong W, Lu P 2010 Opt. Express 18 632
Google Scholar
[10] Corkum P B 1993 Phys. Rev. Lett. 71 1994
Google Scholar
[11] Kang H, Chen S, Wang Y, Chu W, Yao J, Chen J, Liu X, Cheng Y, Xu Z 2019 Phys. Rev. A 100 033403
Google Scholar
[12] Huang C, Zhong M, Wu Z 2019 Opt. Express 27 7616
Google Scholar
[13] Li Y, Wang X, Yu B, Tang Q, Wang G, Wan J 2016 Sci. Rep. 6 37413
Google Scholar
[14] Liu Y, Fu L, Ye D, Liu J, Li M, Wu C, Gong Q, Moshammer R, Ullrich J 2014 Phys. Rev. Lett. 112 013003
Google Scholar
[15] He T, Liu Z, Li Y, Yu B, Huang C 2024 Opt. Laser Technol. 178 111215
Google Scholar
[16] Liao Q, Winney A H, Lee S K, Lin Y F, Adhikari P, Li W 2017 Phys. Rev. A 96 023401
Google Scholar
[17] Ye D, Li M, Fu L, Liu J, Gong Q, Liu Y, Ullrich J 2015 Phys. Rev. Lett. 115 123001
Google Scholar
[18] Hao X, Chen J, Li W, Wang B, Wang X, Becker W 2014 Phys. Rev. Lett. 112 073002
Google Scholar
[19] Li X, Qiao Y, Wu D, Yu R, Chen J, Wang J, Guo F, Yang Y 2023 Chinese Phys. B 33 013302
Google Scholar
[20] Xu T, Zhu Q, Chen J, Ben S, Zhang J, Liu X 2018 Opt. Express 26 1645
Google Scholar
[21] Lin K, Jia X, Yu Z, He F, Ma J, Li H, Gong X, Song Q, Ji Q, Zhang W, Li H, Lu P, Zeng H, Chen J, Wu J 2017 Phys. Rev. Lett 119 203202
Google Scholar
[22] Dong S, Chen X, Zhang J, Ren X 2016 Phys. Rev. A 93 053410
Google Scholar
[23] Rudenko A, Jesus V L B, Ergler Th, Zrost K, Feuerstein B, Schröter C D, Moshammer R, Ullrich J 2007 Phys. Rev. Lett. 99 263003
Google Scholar
[24] Chen Z, Li S, Kang H, Morishita T, Bartschat K 2022 Opt. Express 30 44039
Google Scholar
[25] Chen X, Ruiz C, He F, Zhang J 2020 Opt. Express 28 14884
Google Scholar
[26] Huang C, Zhong M, Wu Z 2016 Opt. Express 24 28361
Google Scholar
[27] Ma X, Zhou Y, Li N, Li M, Lu P 2018 Opt. Laser Technol. 108 235
Google Scholar
[28] Luo S, Ma X, Xie H, Li M, Zhou Y, Cao W, Lu P 2018 Opt. Express 26 13666
Google Scholar
[29] He T, Liu Z, Liao J, Li Y, Yu B, Huang C 2024 Phys. Rev. A 109 043109
Google Scholar
[30] He L, Li Y, Zhang Q, Lu P 2013 Opt. Express 21 2683
Google Scholar
[31] Qin Y, Guo F, Li S, Yang Y, Chen G 2014 Chin. Phys. B 23 093205
Google Scholar
[32] Ho P J, Panfili R, Haan S L, Eberly J H 2005 Phys. Rev. Lett. 94 093002
Google Scholar
[33] Liu Z, Huang C, He T, Liao J, Li Y, Yu B 2024 Phys. Chem. Chem. Phys. 26 4572
Google Scholar
[34] Mauger F, Chandre C, Uzer T 2009 Phys. Rev. Lett. 102 173002
Google Scholar
[35] 廖健颖, 贺佟佟, 苏杰, 刘子超, 李盈傧, 余本海, 黄诚 2023 72 193202
Google Scholar
Liao J Y, He T T, Su J, Liu Z C, Li Y B, Yu B H, Huang C 2023 Acta Phys. Sin. 72 193202
Google Scholar
[36] 黄诚, 苏杰, 廖健颖, 刘子超, 贺佟佟, 李盈傧 2023 光子学报 52 1126003
Google Scholar
Huang C, Su J, Liao J Y, Liu Z C, He T T, Li Y B 2023 Acta Photonica Sin. 52 1126003
Google Scholar
[37] Tong A, Li Q, Ma X, Zhou Y, Lu P 2019 Opt. Express 27 6415
Google Scholar
下载:
计量
- 文章访问数: 790
- PDF下载量: 43
- 被引次数: 0
