-
本文采用实时傅里叶变换光谱探测技术, 研究了基于泵浦强度调制的超快光纤激光器中锁模脉冲产生与湮灭动力学过程. 结果表明: 当泵浦调制电压处于高电平时, 激光器输出稳定的锁模脉冲. 随着调制电压跳变至低电平, 锁模脉冲的强度不断降低, 而后经历一段衰减振荡阶段后发生湮灭, 在~5 μs后孤子从噪声中重建, 这一过程伴随着调Q不稳定性的产生. 在低电平阶段, 激光腔内的湮灭过程连续发生, 其周期为~55 μs. 通过改变调制泵浦的占空比, 能够操控在低电平调制下孤子连续湮灭的次数. 进一步, 锁模与孤子湮灭的连续切换过程与泵浦调制频率有关, 调制频率的升高能够有效缩短两种状态的持续时间从而减少孤子湮灭的次数. 此外, 通过减小低电平的值, 能够降低激光腔内的增益, 使得孤子连续湮灭的周期缩短. 研究结果有利于深入了解孤子的形成与湮灭动力学, 并为超快激光器各种运行机制的发展提供了新的视角.In this paper, the mode-locked pulse generation and annihilation dynamics in ultrafast fiber lasers based on pump intensity modulation are investigated by using real-time Fourier transform spectral probing. The results show that the laser outputs stable mode-locked pulses when the pump modulation voltage is at a high level. As the modulation voltage jumps to a low level, the intensity of the mode-locked pulse decreases, and then undergoes a period of decaying oscillation before annihilation occurs, and after ~5 μs the soliton is reconstructed from the noise, accompanied by the generation of the Q-modulation instability. In the low-level phase, the annihilation process in the laser cavity occurs continuously with a period of ~55 μs. By changing the duty cycle of the modulation pump, it is possible to control the the number of times solitons continuously annihilate under low-level modulation. Further, the continuous switching process of mode-locking and soliton annihilation is related to the modulation frequency of the pump, and the increase of the modulation frequency can effectively shorten the duration of the two states, thus reducing the number of soliton annihilations. In addition, by reducing the value of the low level, the gain in the laser cavity can be reduced, resulting in a shorter period of successive soliton annihilation. The results of the study are conducive to an in-depth understanding of the formation and annihilation dynamics of solitons, and provide new perspectives for developing various operation mechanisms of ultrafast lasers.
-
Keywords:
- pump intensity modulation /
- soliton annihilation /
- pulse state switching /
- dispersive Fourier transform
[1] Yun L, Qiu Y, Yang C H, Xing J, Yu K H, Xu X X, Wei W 2018 Photon. Res. 6 1028
Google Scholar
[2] Malinauskas M, Žukauskas A, Hasegawa S, Hayasaki Y, Mizeikis V, Buividas R, Juodkazis S 2016 Light Sci. Appl. 5 16133
Google Scholar
[3] Mao D, Zheng Y, Zeng C, Lu H, Wang C, Zhang H, Zhang W D, Mei T, Zhao J L 2021 Adv. Photon. 3 014002
[4] Cao B, Gao C X, Liu K W, Xiao X S, Yang C X, Bao C Y 2023 Light Sci. Appl. 12 260
Google Scholar
[5] Wise F W, Chong A, Renninger W H 2008 Laser Photon. Rev. 2 58
Google Scholar
[6] Fermann M E, Hartl I 2013 Nat. Photon. 7 868
Google Scholar
[7] Huang L, Zhang Y S, Liu X M 2020 Nanophotonics 9 2731
Google Scholar
[8] Zhang Y S, Cui Y D, Huang L, Tong L M, Liu X M 2020 Opt. Lett. 45 6246
Google Scholar
[9] Kieu K, Renninger W H, Chong A, Wise F W 2009 Opt. Lett. 34 000593
Google Scholar
[10] Zhao L M, Tang D Y, Zhang H, Cheng T H, Tam H Y, Lu C 2007 Opt. Lett. 32 001806
Google Scholar
[11] Renninger W H, Chong A, Wise F W 2008 Phys. Rev. A 77 023814
Google Scholar
[12] Liu X M 2010 Phys. Rev. A 81 023811
Google Scholar
[13] Li H, Ouzounov D G, Wise F W 2010 Opt. Lett. 35 002403
Google Scholar
[14] Goda K, Tsia K K, Jalali B 2009 Nature 458 1145
Google Scholar
[15] Mahjoubfar A, Churkin D V, Barland S, Broderick N, Turitsyn S K, Jalali B 2017 Nat. Photon. 11 341
Google Scholar
[16] Xu Y Q, Wei X M, Ren Z B, Wong K K Y, Tsia K K 2016 Sci. Rep. 6 27937
Google Scholar
[17] Lu M X, Wang X D, Li K X, Geng X, Pan J Y, Sun M Q, Li S W 2022 Laser Phys. 33 015101
[18] Song Y G, Zou D F, Gat O, Hu M L, Grelu P 2023 Laser Photon. Rev. 17 2300066
Google Scholar
[19] Sheng C, Liu H, Chen H Y, Zhu S N 2018 Nat. Commun. 9 4271
Google Scholar
[20] Cui H, Liu X M 2019 Photon. Res. 7 000423
Google Scholar
[21] Zhou Y, Ren Y X, Shi J W, Mao H D, Wong K K Y 2020 Optica 7 965
Google Scholar
[22] Han D D, Wang Y J, Hui Z Q, Zhang Z X, Ren K, Zheng Y P, Zhao F, Zhu L P, Gong J M 2021 Infrared Phys. Techn. 116 103786
Google Scholar
[23] Korobko D A, Ribenek V A, Itrin P A, Fotiadi A A 2023 Opt. Fiber Technol. 75 103216
Google Scholar
[24] Jiang C, Zhou R, Fang Z, Wan Y, Sun B, Mou C B, Liu Y Q, Zhang Z X 2023 Opt. Lett. 48 5875
Google Scholar
[25] 方振, 余游, 赵秋烨, 张昱冬, 王治强, 张祖兴 2023 73 014202
Google Scholar
Fang Z, Yu Y, Zhao Q Y, Zhang Y D, Wang Z Q, Zhang Z X 2023 Acta Phys. Sin. 73 014202
Google Scholar
[26] Peng J S, Boscolo S, Zhao Z H, Zeng H P 2019 Sci. Adv. 5 1110
[27] Gui L, Wang P, Ding Y H, Zhao K J, Bao C Y, Xiao X S, Yang C X 2018 Appl. Sci. 8 201
Google Scholar
-
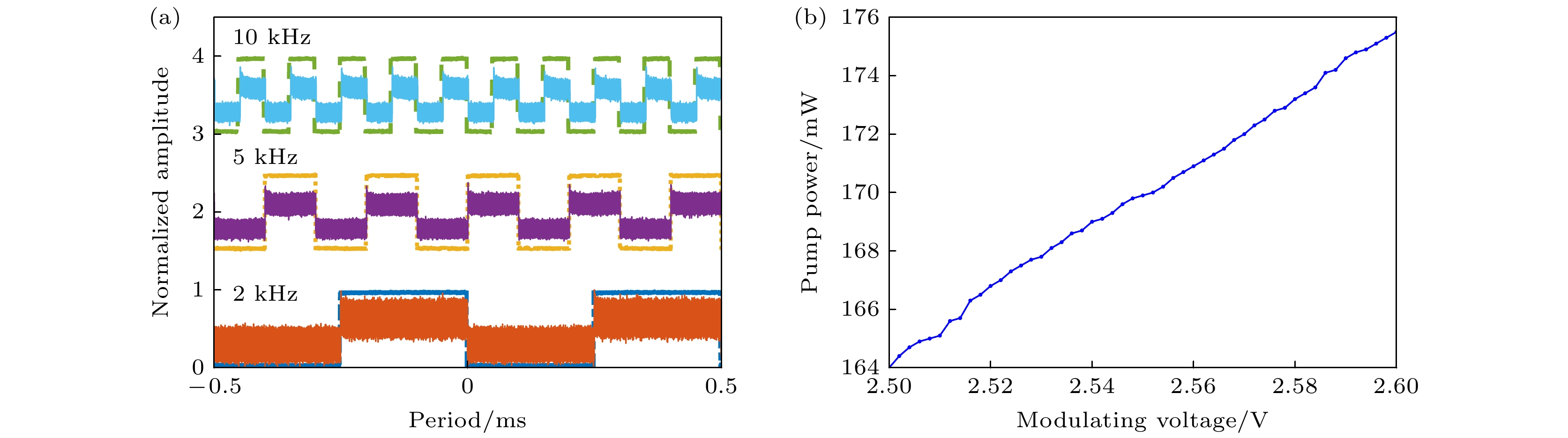
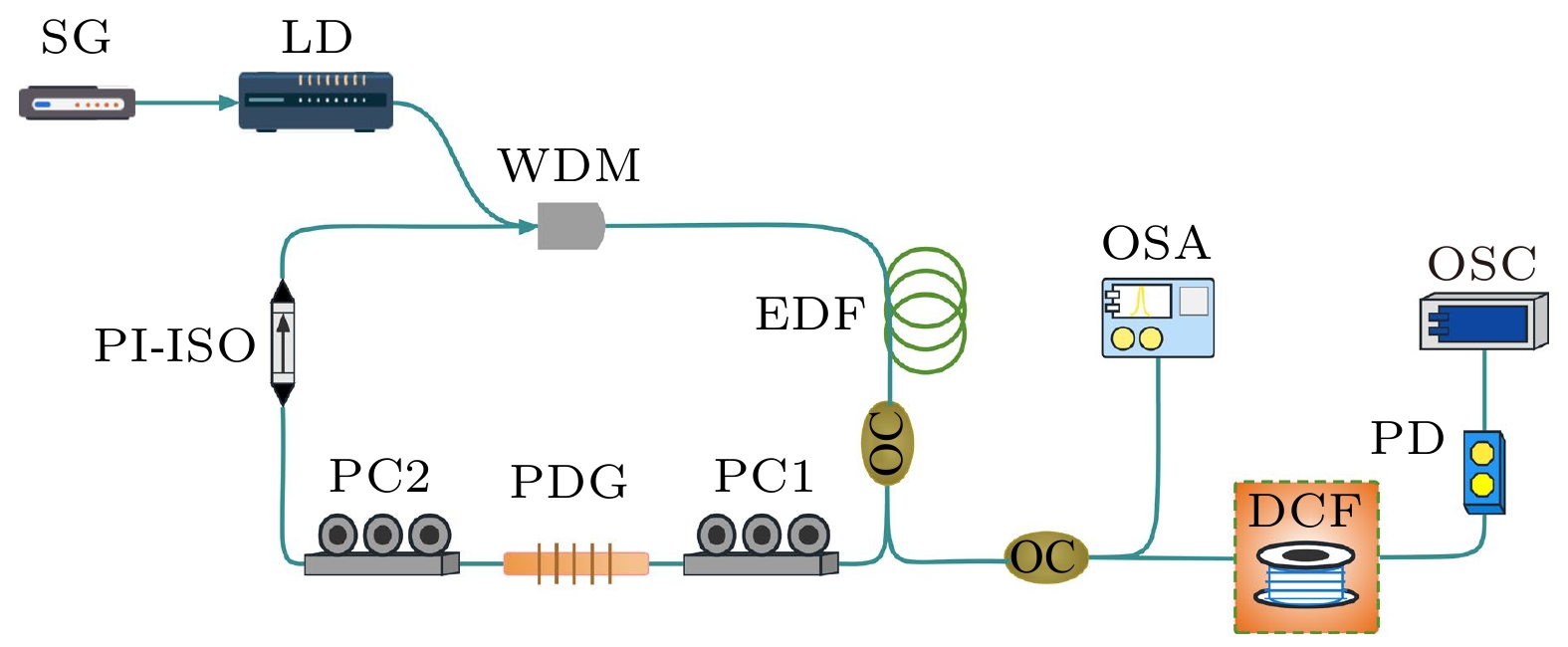
图 1 实验装置图(SG-信号发生器; LD-半导体光源; WDM-波分复用器; PC-偏振控制器; OC-光耦合器; PDG-偏振相关光栅; PI-ISO-偏振无关隔离器; DCF-色散补偿光纤; PD-光电探测器; OSA-光谱仪; OSC-示波器)
Fig. 1. Diagram of experimental setup(SG-signal generator; LD-semiconductor light source; WDM-wavelength division multiplexer; PC-polarization controller; OC-90:10 optical coupler; PDG-polarization-dependent grating; PI-ISO-polarization-independent isolator; DCF-dispersion compensation fiber; PD-photodetector; OSA-optical spectrum analyzer; OSC-oscilloscope).
图 2 调制泵浦的性能表征 (a) 2, 5和10 kHz调制频率下的输入泵浦脉冲波形和载波信号波形; (b)调制电平和泵浦功率关系图
Fig. 2. Performance characterization of modulated pump: (a) Input pump pulse waveform and carrier signal waveform at 2, 5, and 10 kHz modulation frequencies; (b) plot of modulation level versus pump power.
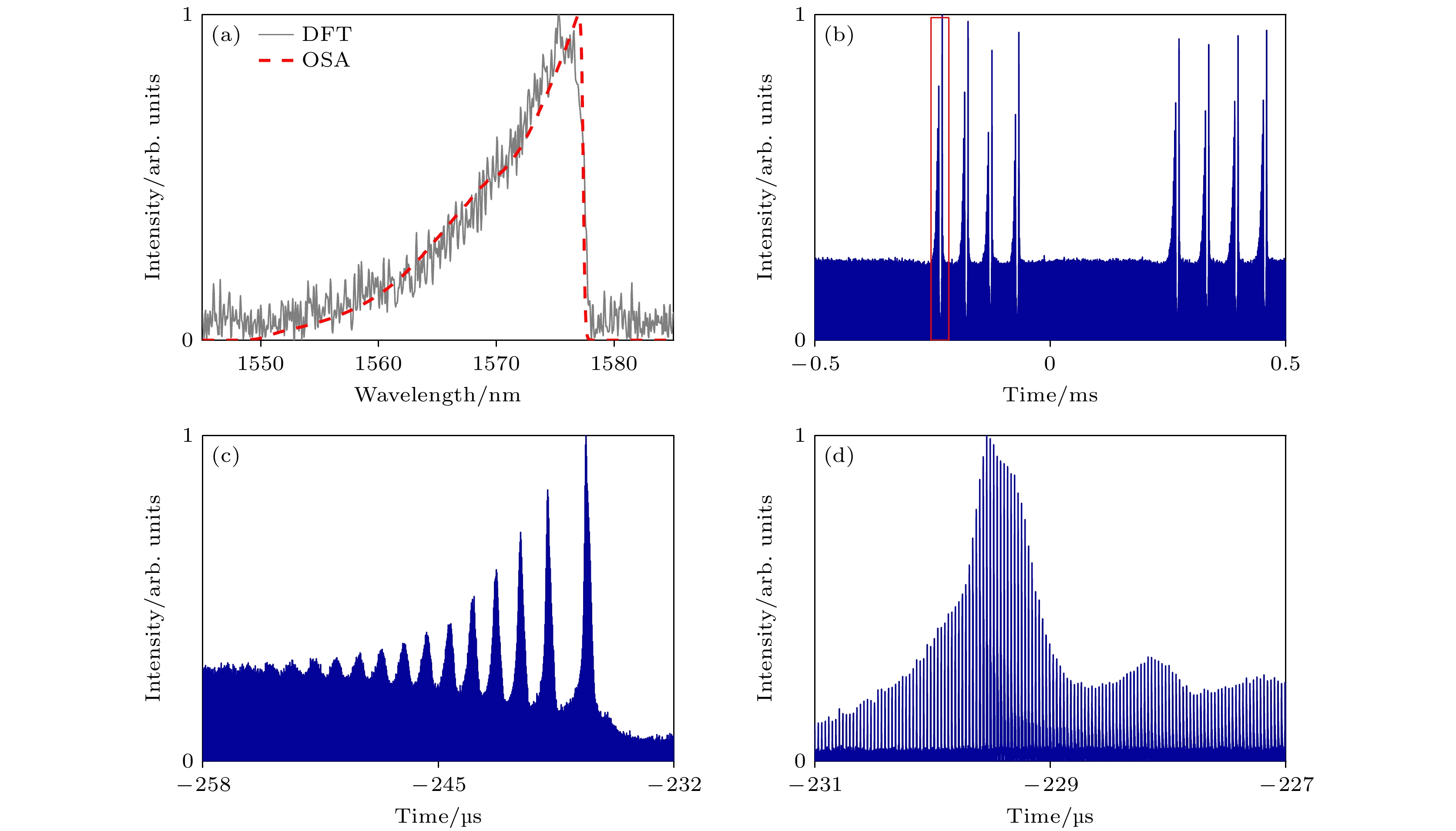
图 3 DFT准确性验证及脉冲状态切换过程脉冲序列 (a)用OSA记录的光谱(虚线)和从DFT测量结果提取出的单次光谱(实线); (b)切换过程相应的脉冲序列; (c), (d)孤子湮灭前后的细节放大图
Fig. 3. DFT accuracy verification and pulse trains during pulse state switching process: (a) Spectra recorded with OSA (dashed line) and single spectra extracted from DFT measurements (solid line); (b) pulse sequence corresponding to the switching process; (c), (d) detailed enlargements of the soliton before and after its annihilation.
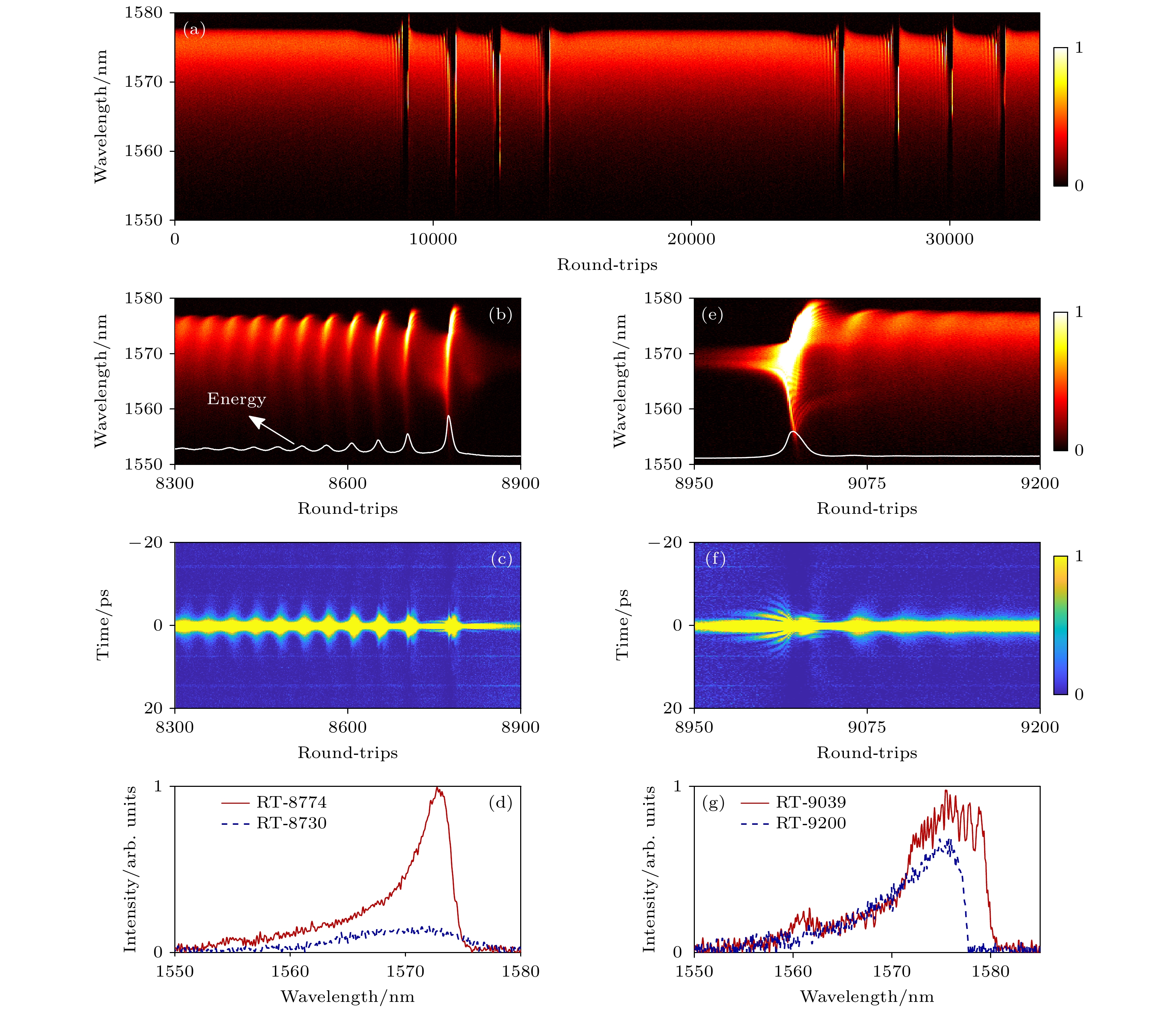
图 4 稳定锁模与孤子湮灭切换过程的实验实时数据 (a) DFT光谱演化图; (b)孤子湮灭前的细节放大; (c)相应的场自相关迹演化; (d)一个呼吸周期内最宽和最窄脉宽的光谱图; (e)孤子重建时的细节放大; (f)相应的场自相关迹演化; (g)调Q不稳定性状态下与稳定锁模状态下的光谱
Fig. 4. Experimental real-time data for stable mode-locking and soliton annihilation switching processes: (a) DFT spectral evolution diagram; (b) detailed enlargement of the soliton before its annihilation; (c) corresponding field autocorrelation trace evolution; (d) spectrograms of the widest and narrowest pulse widths in one breathing period; (e) detailed zoom in on the reconstruction of the soliton; (f) corresponding field autocorrelation trace evolution; (g) spectra of the Q-switched instability state versus the stable mode-locked state.
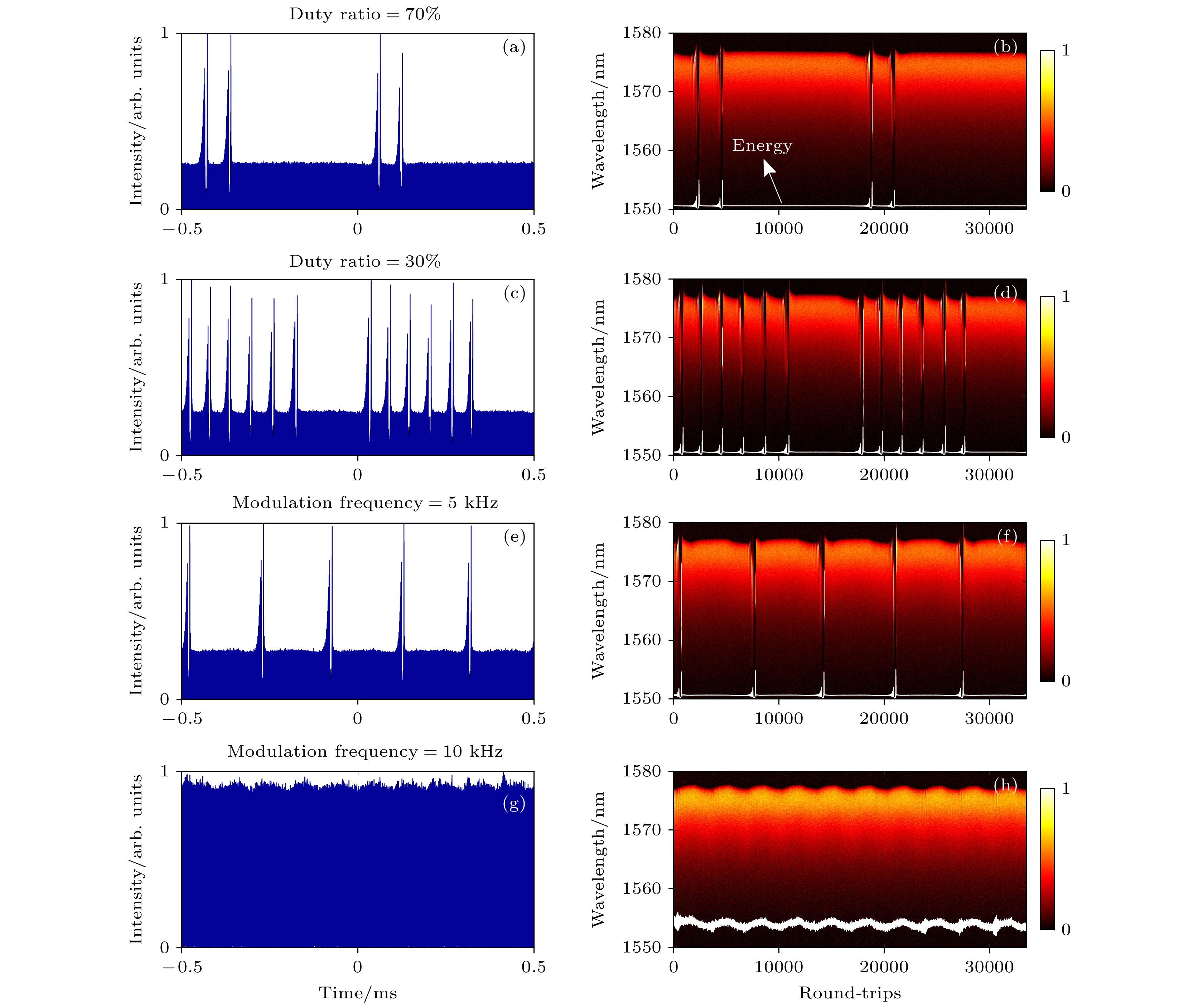
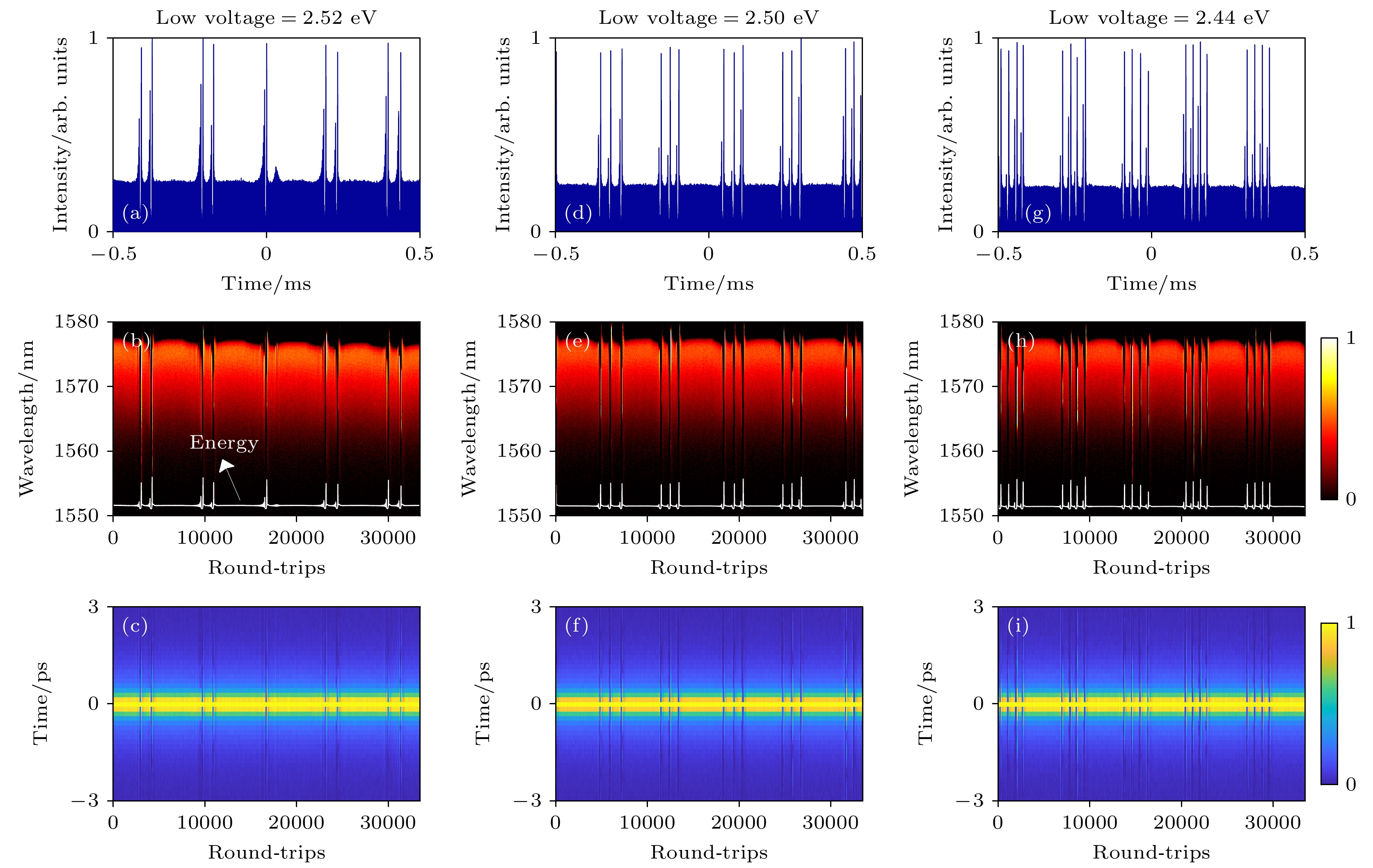
图 5 不同占空比和调制频率下切换过程的脉冲序列和DFT光谱图 (a), (b)低电平调制占空比为30%; (c), (d)低电平调制占比为70%; (e), (f) 5 kHz调制频率; (g), (h) 10 kHz调制频率
Fig. 5. Pulse trains and DFT spectra of the switching process at different duty ratios and modulation frequencies: (a), (b) 30% duty ratio for low-level modulation; (c), (d) 70% duty ratio for low-level modulation; (e), (f) 5 kHz modulation frequency; (g), (h) 10 kHz modulation frequency.
图 6 不同低电平下切换过程的脉冲序列、DFT光谱图及自相关迹演化 (a), (b), (c) 2.52 V; (d), (e), (f) 2.50 V; (g), (h), (i) 2.44 V
Fig. 6. Pulse sequence, DFT spectra, and autocorrelation trace evolution of switching process at different low levels: (a), (b), (c) 2.52 V; (d), (e), (f) 2.50 V; (h), (g), (i) 2.44 V.
-
[1] Yun L, Qiu Y, Yang C H, Xing J, Yu K H, Xu X X, Wei W 2018 Photon. Res. 6 1028
Google Scholar
[2] Malinauskas M, Žukauskas A, Hasegawa S, Hayasaki Y, Mizeikis V, Buividas R, Juodkazis S 2016 Light Sci. Appl. 5 16133
Google Scholar
[3] Mao D, Zheng Y, Zeng C, Lu H, Wang C, Zhang H, Zhang W D, Mei T, Zhao J L 2021 Adv. Photon. 3 014002
[4] Cao B, Gao C X, Liu K W, Xiao X S, Yang C X, Bao C Y 2023 Light Sci. Appl. 12 260
Google Scholar
[5] Wise F W, Chong A, Renninger W H 2008 Laser Photon. Rev. 2 58
Google Scholar
[6] Fermann M E, Hartl I 2013 Nat. Photon. 7 868
Google Scholar
[7] Huang L, Zhang Y S, Liu X M 2020 Nanophotonics 9 2731
Google Scholar
[8] Zhang Y S, Cui Y D, Huang L, Tong L M, Liu X M 2020 Opt. Lett. 45 6246
Google Scholar
[9] Kieu K, Renninger W H, Chong A, Wise F W 2009 Opt. Lett. 34 000593
Google Scholar
[10] Zhao L M, Tang D Y, Zhang H, Cheng T H, Tam H Y, Lu C 2007 Opt. Lett. 32 001806
Google Scholar
[11] Renninger W H, Chong A, Wise F W 2008 Phys. Rev. A 77 023814
Google Scholar
[12] Liu X M 2010 Phys. Rev. A 81 023811
Google Scholar
[13] Li H, Ouzounov D G, Wise F W 2010 Opt. Lett. 35 002403
Google Scholar
[14] Goda K, Tsia K K, Jalali B 2009 Nature 458 1145
Google Scholar
[15] Mahjoubfar A, Churkin D V, Barland S, Broderick N, Turitsyn S K, Jalali B 2017 Nat. Photon. 11 341
Google Scholar
[16] Xu Y Q, Wei X M, Ren Z B, Wong K K Y, Tsia K K 2016 Sci. Rep. 6 27937
Google Scholar
[17] Lu M X, Wang X D, Li K X, Geng X, Pan J Y, Sun M Q, Li S W 2022 Laser Phys. 33 015101
[18] Song Y G, Zou D F, Gat O, Hu M L, Grelu P 2023 Laser Photon. Rev. 17 2300066
Google Scholar
[19] Sheng C, Liu H, Chen H Y, Zhu S N 2018 Nat. Commun. 9 4271
Google Scholar
[20] Cui H, Liu X M 2019 Photon. Res. 7 000423
Google Scholar
[21] Zhou Y, Ren Y X, Shi J W, Mao H D, Wong K K Y 2020 Optica 7 965
Google Scholar
[22] Han D D, Wang Y J, Hui Z Q, Zhang Z X, Ren K, Zheng Y P, Zhao F, Zhu L P, Gong J M 2021 Infrared Phys. Techn. 116 103786
Google Scholar
[23] Korobko D A, Ribenek V A, Itrin P A, Fotiadi A A 2023 Opt. Fiber Technol. 75 103216
Google Scholar
[24] Jiang C, Zhou R, Fang Z, Wan Y, Sun B, Mou C B, Liu Y Q, Zhang Z X 2023 Opt. Lett. 48 5875
Google Scholar
[25] 方振, 余游, 赵秋烨, 张昱冬, 王治强, 张祖兴 2023 73 014202
Google Scholar
Fang Z, Yu Y, Zhao Q Y, Zhang Y D, Wang Z Q, Zhang Z X 2023 Acta Phys. Sin. 73 014202
Google Scholar
[26] Peng J S, Boscolo S, Zhao Z H, Zeng H P 2019 Sci. Adv. 5 1110
[27] Gui L, Wang P, Ding Y H, Zhao K J, Bao C Y, Xiao X S, Yang C X 2018 Appl. Sci. 8 201
Google Scholar
下载:
计量
- 文章访问数: 708
- PDF下载量: 28
- 被引次数: 0
