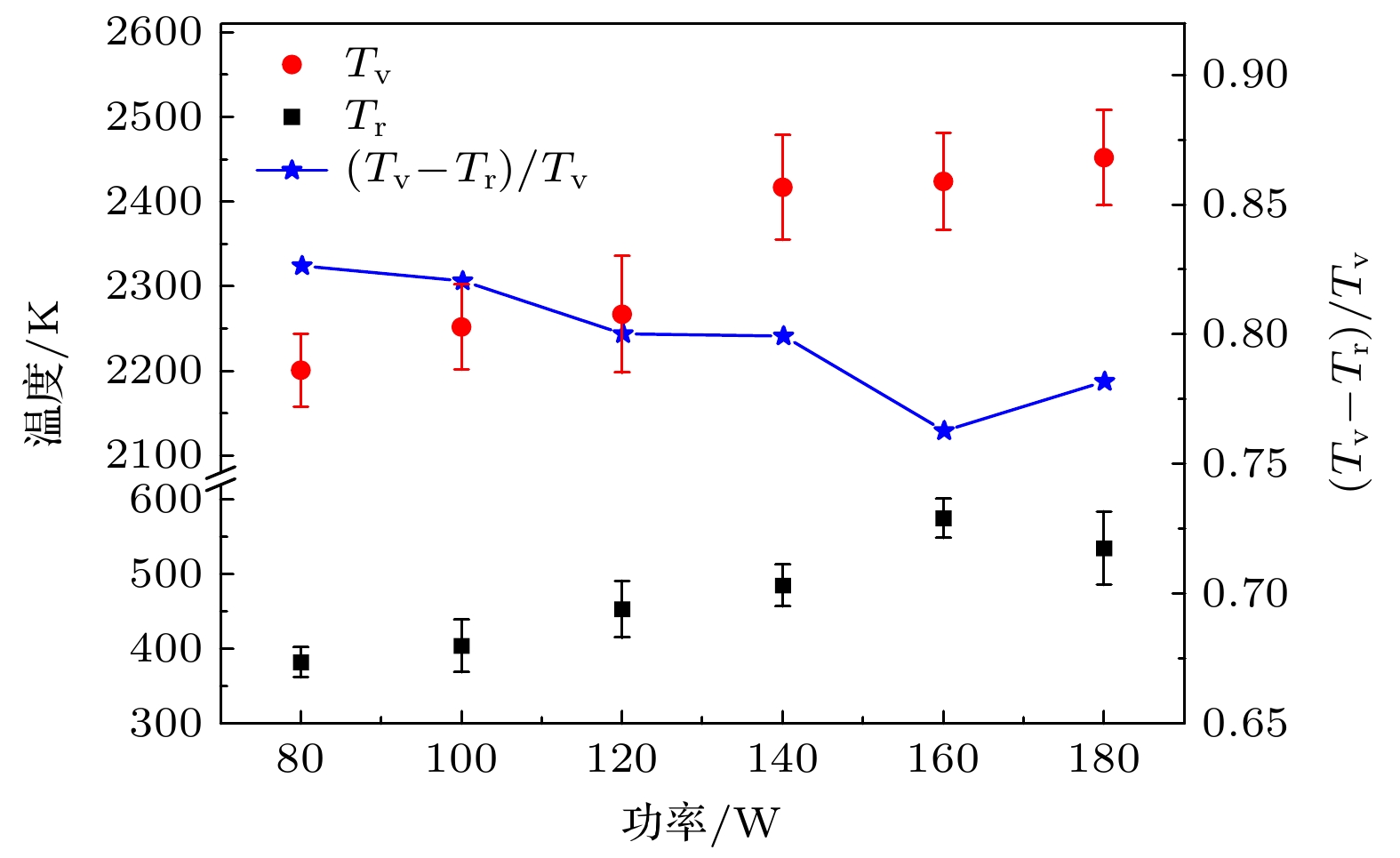
-
高温非平衡问题是高超声速流动中最基本的科学问题之一, 而热力学非平衡特性的准确表征是理解高温非平衡问题和高超声速空气动力学的基础, 如何准确可靠地表征流场的热力学非平衡特性是解决高超声速飞行器在稀薄流域高温非平衡问题的关键. 本文基于相干反斯托克斯拉曼散射基本原理, 开发了面向非平衡流场的振转温度反演算法, 并在宽温度范围静态环境开展验证. 搭建了非平衡等离子体流场相干反斯托克斯拉曼散射测温实验平台并开展实验验证, 结果表明微波等离子体处于热力学非平衡状态, 并且振动温度和转动温度与微波功率成正比, 而热力学非平衡度与微波功率成反比, 当微波功率从80 W增加至180 W时, 等离子体电子数密度增加, 中性粒子通过与电子碰撞获得能量使振动温度从(2201 ± 43) K增加至(2452 ± 56) K、转动温度从(382 ± 20) K增加至(535 ± 49) K; 而处于振动激发态的分子通过振动-平动弛豫过程(对于N2分子弛豫速率与温度成正比)将部分振动能转化为平动能, 导致振动温度与转动温度的差异降低, 等离子体热力学非平衡度从0.83降低至0.78.
-
关键词:
- 热力学非平衡 /
- 振动温度 /
- 转动温度 /
- 相干反斯托克斯拉曼散射
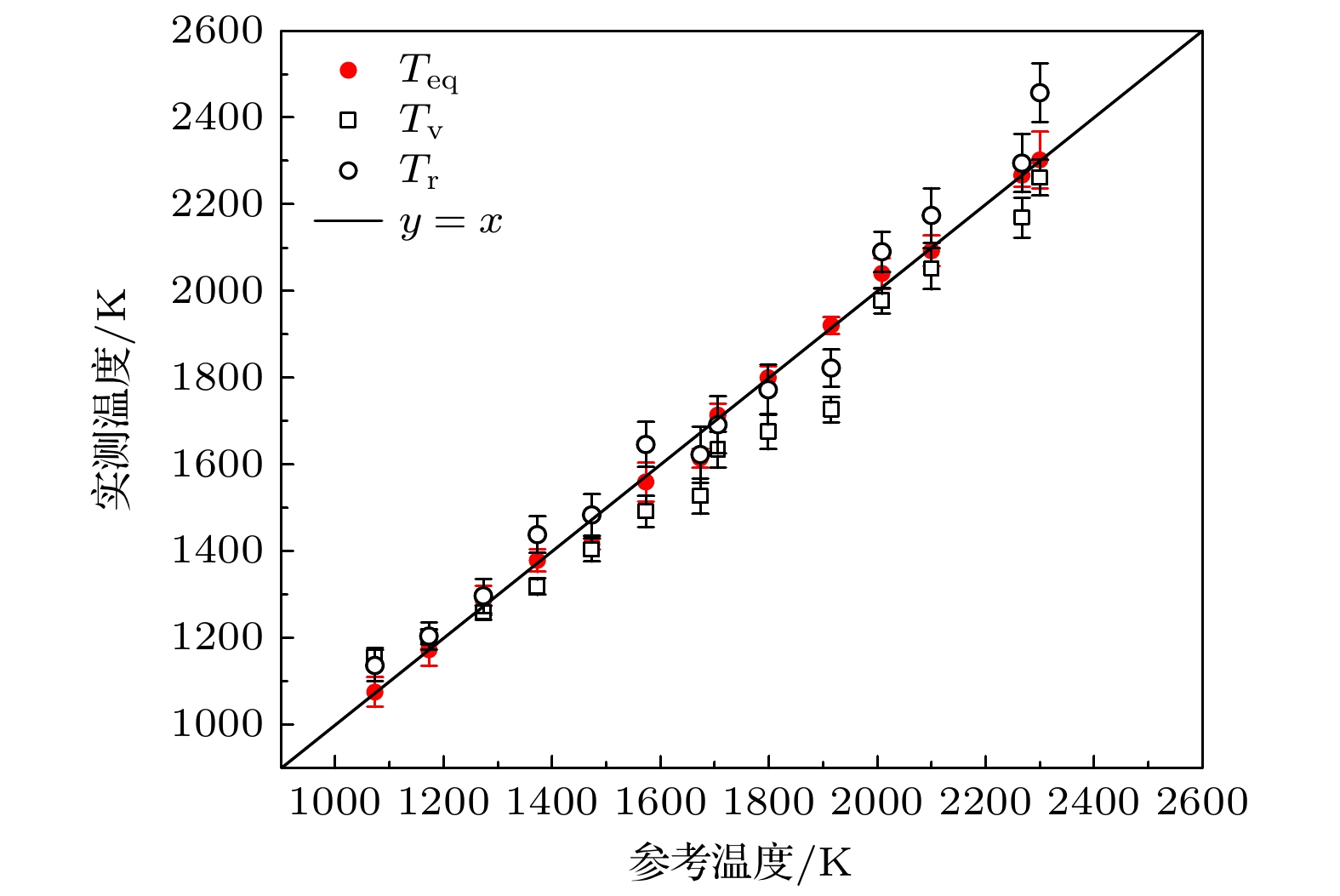
How to characterize thermodynamic non-equilibrium characteristics of flow field accurately and reliably is the key to solving the thermal and chemical non-equilibrium problem, which is one of the most basic scientific problems in hypersonic aerodynamcis. Based on the principles of coherent anti-Stokes Raman scattering (CARS) and modified exponential gap (MEG) Raman linewidth model, a CARS spectral computation and vib-rotational temperature inversion program is proposed for characterizing the thermodynamic non-equilibrium properties of high-temperature gas flow field. The influence of vibrational temperature and rotational temperature on Raman linewidth and CARS spectral characteristics are studied theoretically. A CARS system is built and the corresponding accuracy in a wide temperature range is verified in a static environment that is established by using a high-temperature tube furnace and a McKenna burner. The results show that the average relative deviation of the vibration temperature Tv and rotational temperature Tr from the equilibrium temperature Teq are 4.28% and 3.34% respectively in a range of 1000 to 2300 K, and the corresponding average repeatability is 1.95% and 3.03% respectively. These results indicate that the vibrational temperature and rotational temperature obtained by the non-equilibrium program are in good agreement with those obtained from the thermal equilibrium program. Finally, a non-equilibrium microwave plasma flow is built and its vibrational temperature and rotational temperature are obtained by using the developed program. The results show that the microwave plasma is in thermodynamic non-equilibrium, and the vibrational temperature and rotational temperature are proportional to microwave power, while the thermodynamic non-equilibrium degree exhibits an opposite trend. With microwave power increasing from 80 to 180 W, the vibrational temperature of plasma increases from (2201 ± 43) K to (2452 ± 56) K, the rotational temperature increases from (382 ± 20) K to (535 ± 49) K, for which the principal reasons are that the increase in microwave power leads to an increase in electron number density, and neutral particles obtain energy through collision with electrons, resulting in the increase of vibrational temperature, rotational temperature, and translational temperature. The thermodynamic non-equilibrium degree decreases from 0.83 to 0.78 with the microwave power increasing, which is due to the V-T relaxation rate increasing. The molecules in the excited vibrational states lose energy through collision with ground state molecules (i.e. V-T relaxation process), leading the vibrational energy to be converted into translational energy. For N2 molecules, the V-T relaxation rate is directly proportional to the temperature, which causes the difference between vibrational temperature and rotational temperature to decrease with microwave power increasing, and non-equilibrium degree to decrease with microwave power increasing as well.-
Keywords:
- thermodynamic non-equilibrium /
- vibrational temperature /
- rotational temperature /
- coherent anti-Stokes Raman scattering
[1] 姜宗林, 李进平, 胡宗民, 刘云峰, 俞鸿儒 2018 力学学报 50 1283
Google Scholar
Jiang Z L, Li J P, Hu Z M, Liu Y F, Yu H R 2018 Chin. J. Theor. Appl. Mech. 50 1283
Google Scholar
[2] Knisely C P, Zhong X L 2020 AIAA J. 58 1704
Google Scholar
[3] Chen X L, Wang L, Fu S 2021 Phys. Fluids 33 034132
Google Scholar
[4] Yang X F, Gui Y W, Xiao G M, Du Y X, Liu L, Wei D 2020 Int. J. Heat Mass Transfer 146 118869
Google Scholar
[5] Chen X H, Chen F, Zhang S T, Liu H 2016 54th AIAA Aerospace Sciences Meeting San Diego, USA, January 4–8, 2016 p1252
[6] Li W J, Huang J, Zhang Z W, Wang L Y, Huang H M, Liang J 2021 Acta Astronaut. 183 101
Google Scholar
[7] Park C 2013 Int. J. Aeronaut. Space Sci. 14 1
Google Scholar
[8] Holden M S, Wadhams T P, MacLean M, Dufrene M, Mundy E 2012 50th AIAA Aerospace Science Meeting including the New Horizons Forum and Aerospace Exposition Nashville, Tennessee, January 9–12, 2012 p469
[9] Park C 2009 J. Thermophys. Heat Transfer 23 417
Google Scholar
[10] Matsuyama S, Ohnishi N, Sasoh A, Sawada K 2005 J. Thermophys. Heat Transfer 19 28
Google Scholar
[11] Niu Q L, He Z H, Dong S K 2016 Chin. J. Aeronaut. 29 1367
Google Scholar
[12] Furudate M, Nonaka S, Sawada K 1999 J. Thermophys. Heat Transfer 13 424
Google Scholar
[13] Winter M W, Srinivasan C, Charnigo R 2015 46th AIAA Plasma dynamics and Lasers Conference Dallas, USA, June 22–26, 2015 p2963
[14] 吕俊明, 李飞, 林鑫, 程晓丽, 余西龙, 俞继军 2019 实验流体力学 33 25
Google Scholar
Lü J M, Li F, Lin X, Cheng X L, Yu X L, Yu J J 2019 J. Exp. Fluid Mech. 33 25
Google Scholar
[15] 曾徽, 余西龙, 李飞, 张少华 2015 实验流体力学 29 79
Google Scholar
Zeng H, Yu X L, Li F, Zhang S H 2015 J. Exp. Fluid Mech. 29 79
Google Scholar
[16] Sharma S P, Ruffin S M, Gillcspic W D, Meyer S A 1992 27th Thermophysics Conference Nashville, USA, July 6–8, 1992 p2855
[17] Takayanagi H, Mizuno M, Fujii K, Suzuki T, Fujita K 2009 41st AIAA Thermophysics Conference San Antonio, USA, June 22, 2009 p4241
[18] Grisch F, Bouchardy P, Péalat M 1993 23rd Fluid Dynamics, Plasmadynamics and Lasers Conference Orlando, USA, July 6, 1993 p3047
[19] Grisch F, Bouchardy P, Joly V, Marmignon C, Koch U, Gulhan A 2000 AIAA J. 38 1669
Google Scholar
[20] Kim M, Koch U, Esser B, Koch U 2011 42nd AIAA Thermophysics Conference Honolulu, USA, June 27, 2011 p3777
[21] Gülhan A, Esser B, Koch U, Fischer M, Magens E, Hannemann V 2018 AIAA J. 56 1072
Google Scholar
[22] Dogariu A, Dogariu L, Smith M S, Lafferty J, Miles R B 2019 AIAA SciTech Forum San Diego, USA, January 7–11, 2019 p1089
[23] Dogariu A, Dogariu L, Smith M S, McManamen B, Lafferty J F, Miles R B 2021 AIAA SciTech Forum January 11–15 & 19–21, Virtual Event, 2021 p0020
[24] Montello A, Nishihara M, Rich JW, Adamovich I V, Lempert W R 2012 50th AIAA Aerospace Sciences Meeting including the New Horizons Forum and Aerospace Exposition Nashville, USA, January 9–12, 2012 p0239
[25] 田子阳, 赵会杰, 尉昊赟, 李岩 2021 70 214203
Google Scholar
Tian Z Y, Zhao H J, Wei H Y, Li Y 2021 Acta Phys. Sin. 70 214203
Google Scholar
[26] Yang W B, Qi X H, Ye J W, Li M, Zhou J N, Chen S, Mu J H 2020 Proc. of SPIE, Global Intelligent Industry Conference Guangzhou, China, October 22–24 p11780L
[27] Huang A, Xu Z Y, Deng H, Yang W B, Qi X H, Li J, Kan R F 2023 Microwave Opt. Technol. Lett. 66 1
Google Scholar
[28] Hu Z Y, Liu J R, Ye J F, Zhang Z R, Tao B, Zhang L R 2012 2nd International Symposium on Laser Interaction with Matter Xi’an China, May 16, 2013 87961G
[29] 张虎 2009 博士学位论文 (哈尔滨: 哈尔滨工业大学)
Zhang H 2009 Ph. D. Dissertation (Harbin: Harbin Institute of Technology
[30] Dedic C E 2017 Ph. D. Dissertation (Ames: Iowa State University
[31] Rahn L, Palmer R 1986 J. Opt. Soc. Am. B 3 1164
Google Scholar
[32] Weigand P, Rainer L, Meier W 2003 http://www.dlr.de/VT/Datenarchiv
[33] Xiao W, Zeng X Y, Zhu H C, Huang K M 2018 Vac. Electron. 1 48
Google Scholar
[34] Capitelli M, Ferreira C M, Gordiets, Osipov A I 2000 Plasma Kinetics in Atmospheric Gases (Berlin: Springer Press) pp106, 107
-
图 2 振转温度反演流程示意图
Fig. 2. Schematic diagram of vibrational-rotational temperature retrieval process.
图 3 (a) CARS测量系统示意图; (b) USED相位匹配方式下各个光束的空间位置示意图; (c)非平衡等离子体振转温度测量实验
Fig. 3. (a) Schematic diagram of the CARS measurement system; (b) schematic diagram of the spatial positions of various beams in the USED phase matching method; (c) experiment on the measurement of vibrational-rotational temperature of non-equilibrium plasma.
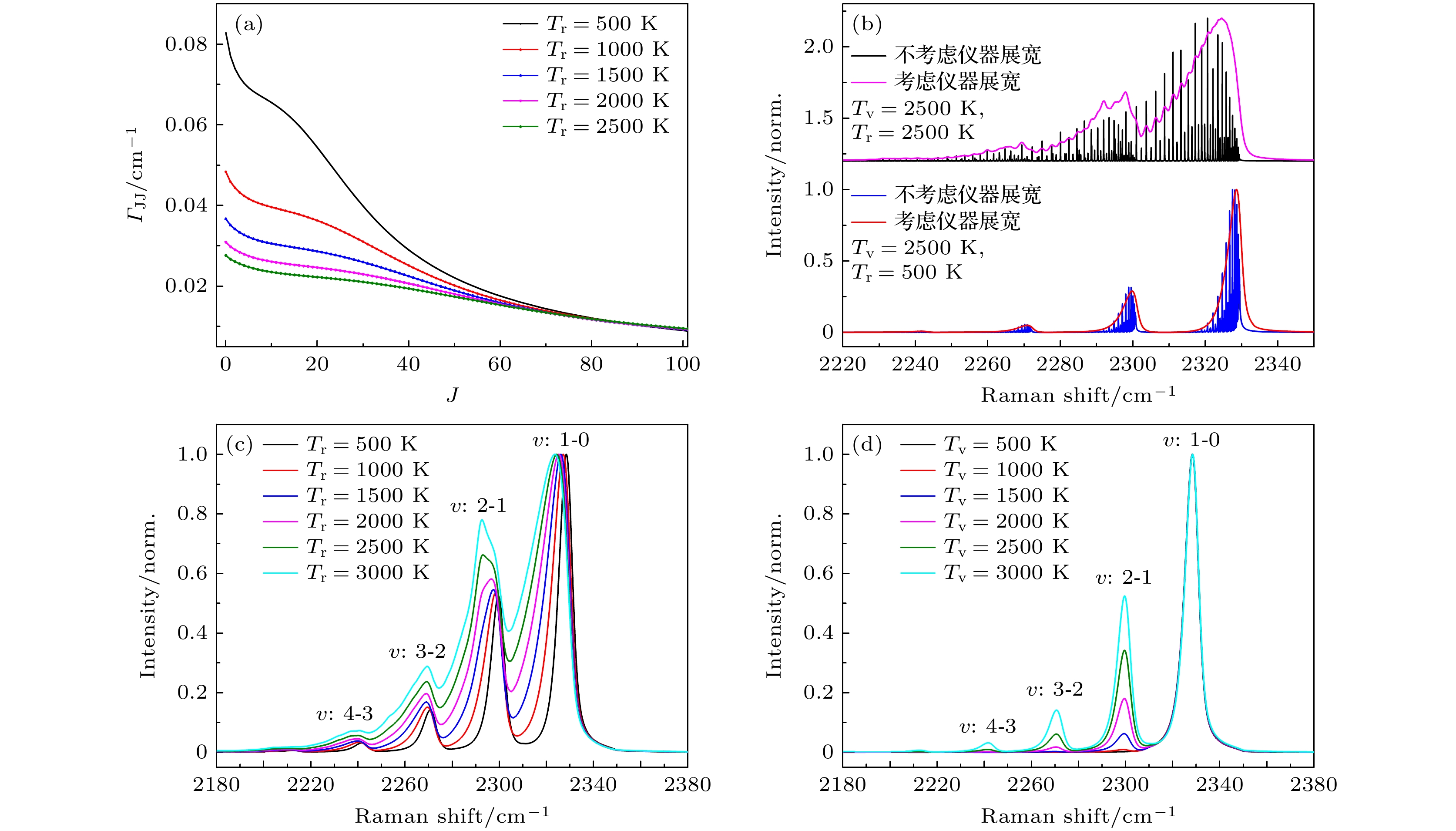
图 4 (a) 拉曼线宽计算结果; (b) 热力学平衡和非平衡时的理论CARS光谱; (c) 不同转动温度下的理论CARS光谱; (d) 不同振动温度下的理论CARS光谱
Fig. 4. (a) Calculation results of Raman linewidth; (b) theoretical spectra in thermodynamic equilibrium and non-thermodynamic equilibrium; (c), (d) theoretical spectra with different (c) rotational temperature and (d) vibrational temperature.
图 5 宽范围振转温度验证结果
Fig. 5. Measurement results of vibrational and rotational temperature in wide-range.
图 6 (a) 实测CARS光谱及振转温度反演结果; (b) 振转温度统计分布
Fig. 6. (a) Measurement CARS spectrum and corresponding fitting results; (b) statistical distributions of vibrational and rotational temperature.
图 7 不同微波功率下的振转温度
Fig. 7. Vibrational and rotational temperatures in different microwave power.
表 1 计算跃迁能级项所用N2分子常数
Table 1. Nitrogen molecular constants used in transition energy terms.
常数 数值/cm–1 参数 数值/cm–1 ωe 2358 γe –0.315×10–4 xeωe 14.29 De 0.5774×10–5 yeωe –0.0059 βe 0.155×10–7 zeωe –0.00024 δe 0 Be 1.998 H0 0.3×10–11 αe 0.0173 He 0.18×10–11 
下载: 导出CSV
表 2 MEG模型中N2分子所用参数
Table 2. Structural parameters of capillary of different kind of fluid.
参数 数值 参数 数值 A0 0.020 cm–1·amg–1 N –0.5 T0 295 K β 1.67 a 1.5 δ 1.21 m 0.1487
下载: 导出CSV
表 3 验证实验工况
Table 3. Test conditions for different cases.
Case 流量/(L·min–1) Tref/K Teq/K Tv/K Tr/K CH4 Air O2 1 1.100 15.00 — 1706 1713±27 1634±41 1691±66 2 1.420 15.00 — 1799 1800±27 1675±40 1772±58 3 2.287 15.00 — 1915 1920±19 1726±29 1822±43 4 2.550 24.14 — 2009 2040±35 1977±29 2090±47 5 3.420 32.40 — 2100 2092±35 2051±47 2174±63 6 4.480 28.32 3.02 — 2267±26 2169±46 2295±67 7 4.930 26.67 4.21 — 2302±65 2261±41 2457±68
下载: 导出CSV
-
[1] 姜宗林, 李进平, 胡宗民, 刘云峰, 俞鸿儒 2018 力学学报 50 1283
Google Scholar
Jiang Z L, Li J P, Hu Z M, Liu Y F, Yu H R 2018 Chin. J. Theor. Appl. Mech. 50 1283
Google Scholar
[2] Knisely C P, Zhong X L 2020 AIAA J. 58 1704
Google Scholar
[3] Chen X L, Wang L, Fu S 2021 Phys. Fluids 33 034132
Google Scholar
[4] Yang X F, Gui Y W, Xiao G M, Du Y X, Liu L, Wei D 2020 Int. J. Heat Mass Transfer 146 118869
Google Scholar
[5] Chen X H, Chen F, Zhang S T, Liu H 2016 54th AIAA Aerospace Sciences Meeting San Diego, USA, January 4–8, 2016 p1252
[6] Li W J, Huang J, Zhang Z W, Wang L Y, Huang H M, Liang J 2021 Acta Astronaut. 183 101
Google Scholar
[7] Park C 2013 Int. J. Aeronaut. Space Sci. 14 1
Google Scholar
[8] Holden M S, Wadhams T P, MacLean M, Dufrene M, Mundy E 2012 50th AIAA Aerospace Science Meeting including the New Horizons Forum and Aerospace Exposition Nashville, Tennessee, January 9–12, 2012 p469
[9] Park C 2009 J. Thermophys. Heat Transfer 23 417
Google Scholar
[10] Matsuyama S, Ohnishi N, Sasoh A, Sawada K 2005 J. Thermophys. Heat Transfer 19 28
Google Scholar
[11] Niu Q L, He Z H, Dong S K 2016 Chin. J. Aeronaut. 29 1367
Google Scholar
[12] Furudate M, Nonaka S, Sawada K 1999 J. Thermophys. Heat Transfer 13 424
Google Scholar
[13] Winter M W, Srinivasan C, Charnigo R 2015 46th AIAA Plasma dynamics and Lasers Conference Dallas, USA, June 22–26, 2015 p2963
[14] 吕俊明, 李飞, 林鑫, 程晓丽, 余西龙, 俞继军 2019 实验流体力学 33 25
Google Scholar
Lü J M, Li F, Lin X, Cheng X L, Yu X L, Yu J J 2019 J. Exp. Fluid Mech. 33 25
Google Scholar
[15] 曾徽, 余西龙, 李飞, 张少华 2015 实验流体力学 29 79
Google Scholar
Zeng H, Yu X L, Li F, Zhang S H 2015 J. Exp. Fluid Mech. 29 79
Google Scholar
[16] Sharma S P, Ruffin S M, Gillcspic W D, Meyer S A 1992 27th Thermophysics Conference Nashville, USA, July 6–8, 1992 p2855
[17] Takayanagi H, Mizuno M, Fujii K, Suzuki T, Fujita K 2009 41st AIAA Thermophysics Conference San Antonio, USA, June 22, 2009 p4241
[18] Grisch F, Bouchardy P, Péalat M 1993 23rd Fluid Dynamics, Plasmadynamics and Lasers Conference Orlando, USA, July 6, 1993 p3047
[19] Grisch F, Bouchardy P, Joly V, Marmignon C, Koch U, Gulhan A 2000 AIAA J. 38 1669
Google Scholar
[20] Kim M, Koch U, Esser B, Koch U 2011 42nd AIAA Thermophysics Conference Honolulu, USA, June 27, 2011 p3777
[21] Gülhan A, Esser B, Koch U, Fischer M, Magens E, Hannemann V 2018 AIAA J. 56 1072
Google Scholar
[22] Dogariu A, Dogariu L, Smith M S, Lafferty J, Miles R B 2019 AIAA SciTech Forum San Diego, USA, January 7–11, 2019 p1089
[23] Dogariu A, Dogariu L, Smith M S, McManamen B, Lafferty J F, Miles R B 2021 AIAA SciTech Forum January 11–15 & 19–21, Virtual Event, 2021 p0020
[24] Montello A, Nishihara M, Rich JW, Adamovich I V, Lempert W R 2012 50th AIAA Aerospace Sciences Meeting including the New Horizons Forum and Aerospace Exposition Nashville, USA, January 9–12, 2012 p0239
[25] 田子阳, 赵会杰, 尉昊赟, 李岩 2021 70 214203
Google Scholar
Tian Z Y, Zhao H J, Wei H Y, Li Y 2021 Acta Phys. Sin. 70 214203
Google Scholar
[26] Yang W B, Qi X H, Ye J W, Li M, Zhou J N, Chen S, Mu J H 2020 Proc. of SPIE, Global Intelligent Industry Conference Guangzhou, China, October 22–24 p11780L
[27] Huang A, Xu Z Y, Deng H, Yang W B, Qi X H, Li J, Kan R F 2023 Microwave Opt. Technol. Lett. 66 1
Google Scholar
[28] Hu Z Y, Liu J R, Ye J F, Zhang Z R, Tao B, Zhang L R 2012 2nd International Symposium on Laser Interaction with Matter Xi’an China, May 16, 2013 87961G
[29] 张虎 2009 博士学位论文 (哈尔滨: 哈尔滨工业大学)
Zhang H 2009 Ph. D. Dissertation (Harbin: Harbin Institute of Technology
[30] Dedic C E 2017 Ph. D. Dissertation (Ames: Iowa State University
[31] Rahn L, Palmer R 1986 J. Opt. Soc. Am. B 3 1164
Google Scholar
[32] Weigand P, Rainer L, Meier W 2003 http://www.dlr.de/VT/Datenarchiv
[33] Xiao W, Zeng X Y, Zhu H C, Huang K M 2018 Vac. Electron. 1 48
Google Scholar
[34] Capitelli M, Ferreira C M, Gordiets, Osipov A I 2000 Plasma Kinetics in Atmospheric Gases (Berlin: Springer Press) pp106, 107
下载:
计量
- 文章访问数: 1423
- PDF下载量: 35
- 被引次数: 0
