-
本文基于带有不同变系数和势的分数薛定谔方程, 研究了二次相位调制(QPM)下厄米-高斯光束的演化特性. 在自由空间中, 光束聚焦位置随着正QPM系数的增加或莱维指数的减小而变大. QPM为负时, 光束聚焦消失. 在余弦调制和QPM共同作用下, 光束的传输不再遵循余弦规律振荡, 而是表现出一大一小的呼吸结构, 其演化周期会随调制频率的增加而降低. 引入线性调制时, 分裂光束的运动轨迹呈现抛物线状. 在线性调制和QPM共同影响下, 光束呈现出聚焦或聚焦消失的特性. 当考虑幂函数调制和正QPM共同影响时, 在莱维指数较小时, 光束在一定传输距离内保持不失真的直线传输. 当线性势作用时, 光束的分裂随着线性系数的增加而逐渐消失, 最终呈现周期性演化. 在加入QPM后, 光束会得到明显放大. 另外, 光束演化周期与线性系数成反比, 横向振幅随着莱维指数的增加而变大. 当抛物势和QPM共同作用时, 光束会呈现出自动聚焦, 散焦效应, 聚焦频率会随着莱维指数和抛物系数的增加而变大. 这些特性在光学操纵, 光学聚焦等领域具有潜在的应用价值.The Hermite-Gaussian (HG) beam has many potential advanced applications in optical communications, electron acceleration, nonlinear optics and bio-optical disease detection, owing to its distinctive mode and intensity distribution. The research on HG beam are significant in the development of optics, medicine and quantum technology. However, the controlling of the evolutions of HG beam with quadratic phase modulation (QPM) in fractional systems under variable coefficients and potentials has been rarely studied. In this work, the propagation dynamic behaviors of the HG beam with QPM are investigated based on the fractional Schrödinger equation (FSE) under different variable coefficients and potentials by using a split-step Fourier algorithm. In the free space, the focusing spot of the beam becomes larger as the positive QPM coefficient increases or the Lévy index decreases. The QPM coefficient has little effect on the focusing amplitude when the Lévy index is 2. When the QPM coefficient is negative, the focusing of the beam disappears. Under the joint action of cosine modulations and QPM, the transmission of the beam oscillates not by the cosine law, but presents a large and a small breathing structure. The positive and the negative coefficient of QPM only alter the breathing sequence. The evolution period and width of the beam decrease as the modulation frequency increases. The trajectory of split beams turns into a parabolic shape under the linear modulation. In the joint influence of linear modulations and QPM, the HG beam exhibits either focusing or not focusing. Furthermore, the focusing position and focal plane of the beam decrease as the Lévy index increases. When the Lévy index is small, the beam keeps a straight-line transmission without distortion at a longer distance under the joint effect of the power function modulation and a positive QPM. The transmission of the beam also stabilizes and the beam width becomes larger with a negative QPM. Under a linear potential, the splitting of the HG beam disappears with the increase of the linear coefficient and shows a periodic evolution. The propagation trajectory of the beam shows a serrated pattern. By adding QPM, the beam is significantly amplified. Additionally, the evolution period of the beam is inversely proportional to the linear coefficient, and the transverse amplitude turns larger as the Lévy index increases. The interference among beams is strong, but it also exhibits an autofocus-defocusing effect under the joint action of a parabolic potential and QPM. In addition, the positive coefficient and the negative coefficient of QPM only affect the focusing time of the beam. The frequency of focusing increases as the Lévy index and parabolic coefficient rise. These features are important for applications in optical manipulations and optical focusing.
-
Keywords:
- fractional Schrödinger equation /
- Hermite-Gaussian beam /
- quadratic phase modulation /
- variable coefficients and potentials
[1] Wang S L, Xu J P, Yang Y P, Cheng M J 2024 Opt. Commun. 556 130258
Google Scholar
[2] Zhou J H, Hu Q S 2023 Opt. Express 31 38334
Google Scholar
[3] Qiu Y Z, Liu Z R 2024 Results Phys. 58 107457
Google Scholar
[4] Sun Z Y, Deng D, Pang Z G, Yang Z J 2024 Chaos, Solitons Fractals 178 114398
Google Scholar
[5] Sun Z Y, Li J, Bian R, Deng D, Yang Z J 2024 Opt. Express 32 9201
Google Scholar
[6] Arfan M, Khaleel N, Ghaffar A, Razzaz F, Saeed S M, Alanazi T M 2024 Opt. Quantum Electron. 56 135
Google Scholar
[7] Wang Q, Zhu J Y, Wang J, Yu H Y, Hu B B 2024 Chaos, Solitons Fractals 180 114580
Google Scholar
[8] 周王哲, 李雪鹏, 杨晶, 杨天利, 王小军, 刘炳杰, 王浩竹, 杨俊波, 彭钦军 2023 72 014204
Google Scholar
Zhou W Z, Li X P, Yang J, Yang T L, Wang X J, Liu B J, Wang H Z, Yang J B, Peng Q J 2023 Acta Phys. Sin. 72 014204
Google Scholar
[9] Wang S, Wang L, Zhang F R, Kong L J 2022 Chin. Phys. Lett. 39 104101
Google Scholar
[10] Wu S M, Wang Q, Gao X H, Wang Y 2018 Results Phys. 10 607
Google Scholar
[11] Song L M, Yang Z J, Li X L, Zhang S M 2020 Appl. Math. Lett. 102 106114
Google Scholar
[12] Fan X L, Ji X L, Wang H, Deng Y, Zhang H 2021 J. Opt. Soc. Am. A 38 168
Google Scholar
[13] Sharma V, Thakur V, Singh A, Kant N 2021 Chin. J. Phys. 71 312
Google Scholar
[14] Ebel S, Talebi N 2023 Commun. Phys. 6 179
Google Scholar
[15] Che J R, Zheng Y X, Liang G, Guo Q 2023 Chin. Phys. B 32 104207
Google Scholar
[16] Saad F, Benzehoua H, Belafhal A 2024 Opt. Quantum Electron. 56 130
Google Scholar
[17] Laskin N 2000 Phys. Lett. A 268 298
Google Scholar
[18] Longhi S 2015 Opt. Lett. 40 1117
Google Scholar
[19] Zhang L F, Li C X, Zhong H Z, Xu C G, Lei D J, Li Y, Fan D Y 2016 Opt. Express 24 14406
Google Scholar
[20] Huang X W, Deng Z X, Shi X H, Fu X Q 2017 J. Opt. Soc. Am. B: Opt. Phys. 34 2190
Google Scholar
[21] Zhang L F, Zhang X, Wu H Z, Li C X, Pierangeli D, Gao Y X, Fan D Y 2019 Opt. Express 27 27936
Google Scholar
[22] Zang F, Wang Y, Li L 2018 Opt. Express 26 23740
Google Scholar
[23] Xin W, Song L J, Li L 2021 Opt. Commun. 480 126483
Google Scholar
[24] Huang X W, Deng Z X, Fu X Q 2017 J. Opt. Soc. Am. B: Opt. Phys. 34 976
Google Scholar
[25] Chen W J, Wang T, Wang J, Mu Y N 2021 Opt. Commun. 496 127136
Google Scholar
[26] Tan C, Lei T, Zou M, Liang Y, Tang P H, Liu M W 2024 Opt. Commun. 557 130358
Google Scholar
[27] Wen J J, Wang H W, Gao R, Ren S M, Guo T, Xiao Y 2023 Optik 276 170586
Google Scholar
[28] Tan C, Liang Y, Zou M, Lei T, Tang P H, Liu M W 2024 J. Opt. Soc. Am. B: Opt. Phys. 41 921
Google Scholar
[29] Zhang L F, Liu K, Zhong H Z, Zhang J G, Deng J Q, Li Y, Fan D Y 2015 Sci. Rep. 5 11843
Google Scholar
[30] Zhang J G, He J 2017 IEEE Photonics J. 9 1
Google Scholar
[31] Zhan K Y, Jiao R Y, Wang J, Zhang W Q, Yang Z D, Liu B 2020 Ann. Phys. 532 1900546
Google Scholar
[32] Zhan K Y, Zhang W Q, Jiao R Y, Dou L C, Liu B 2020 Opt. Commun. 474 126156
Google Scholar
[33] Jiao C Y, Huang X W, Bai Y F, Fu X Q 2023 J. Opt. Soc. Am. A 40 2019
Google Scholar
-
图 1 不同莱维指数$ \alpha $和QPM系数p下HG光束演化图, 其中$D\left( z \right) = 1$, $ V(x) = 0 $
Fig. 1. Evolution of HG beams for different Lévy index $ \alpha $ and QPM coefficient p, here $D\left( z \right) = 1$, $ V(x) = 0 $.
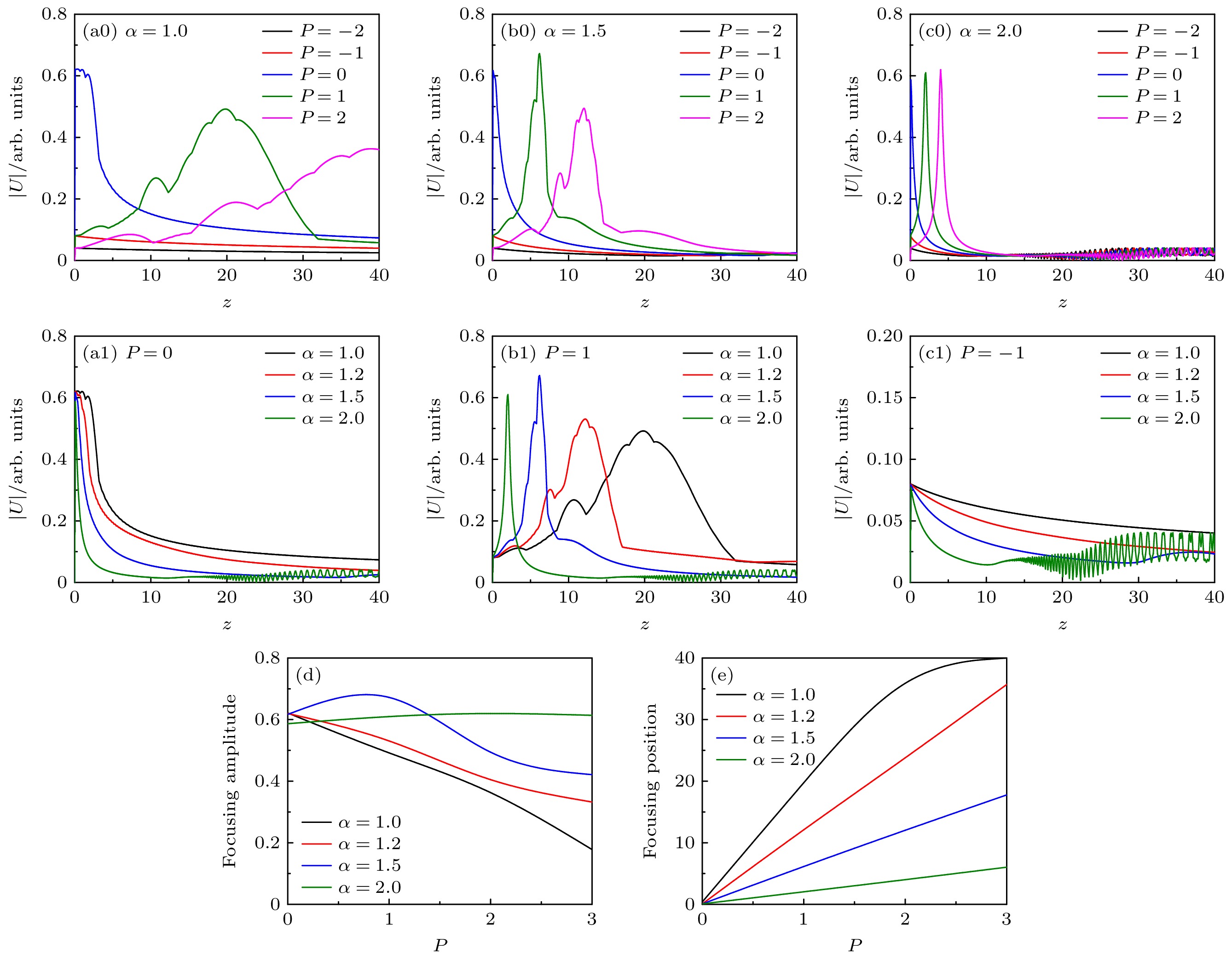
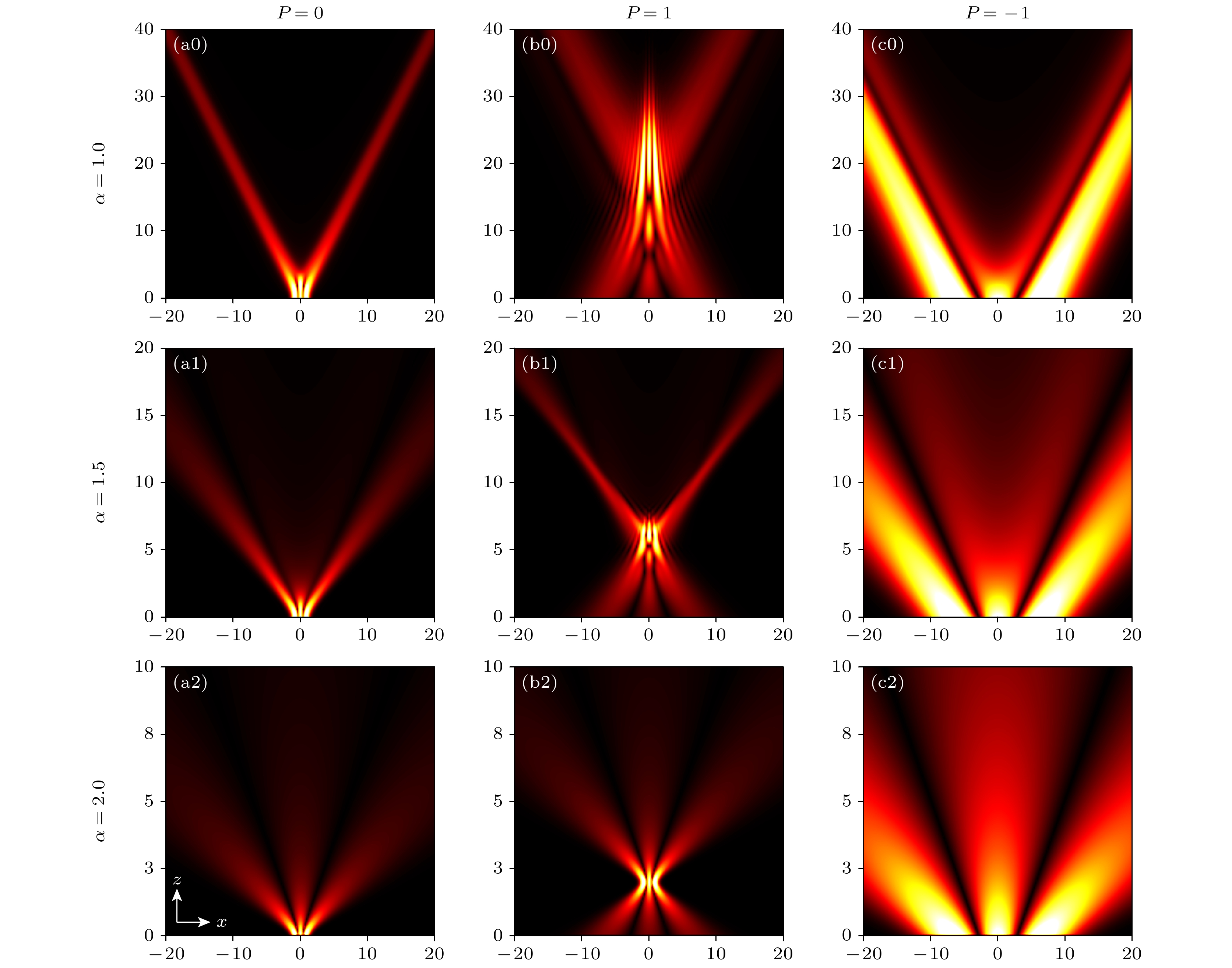
图 2 (a0)—(c1)不同莱维指数$ \alpha $和QPM系数p下HG光束的包络图; 不同莱维指数$ \alpha $下(d)聚焦振幅和(e)聚焦位置与QPM系数p的关系
Fig. 2. (a0)–(c1) Envelopes of HG beams for different Lévy index $ \alpha $ and QPM coefficient p; (d) focusing amplitude and (e) focusing position versus QPM coefficient p under different Lévy index $ \alpha $, respectively.
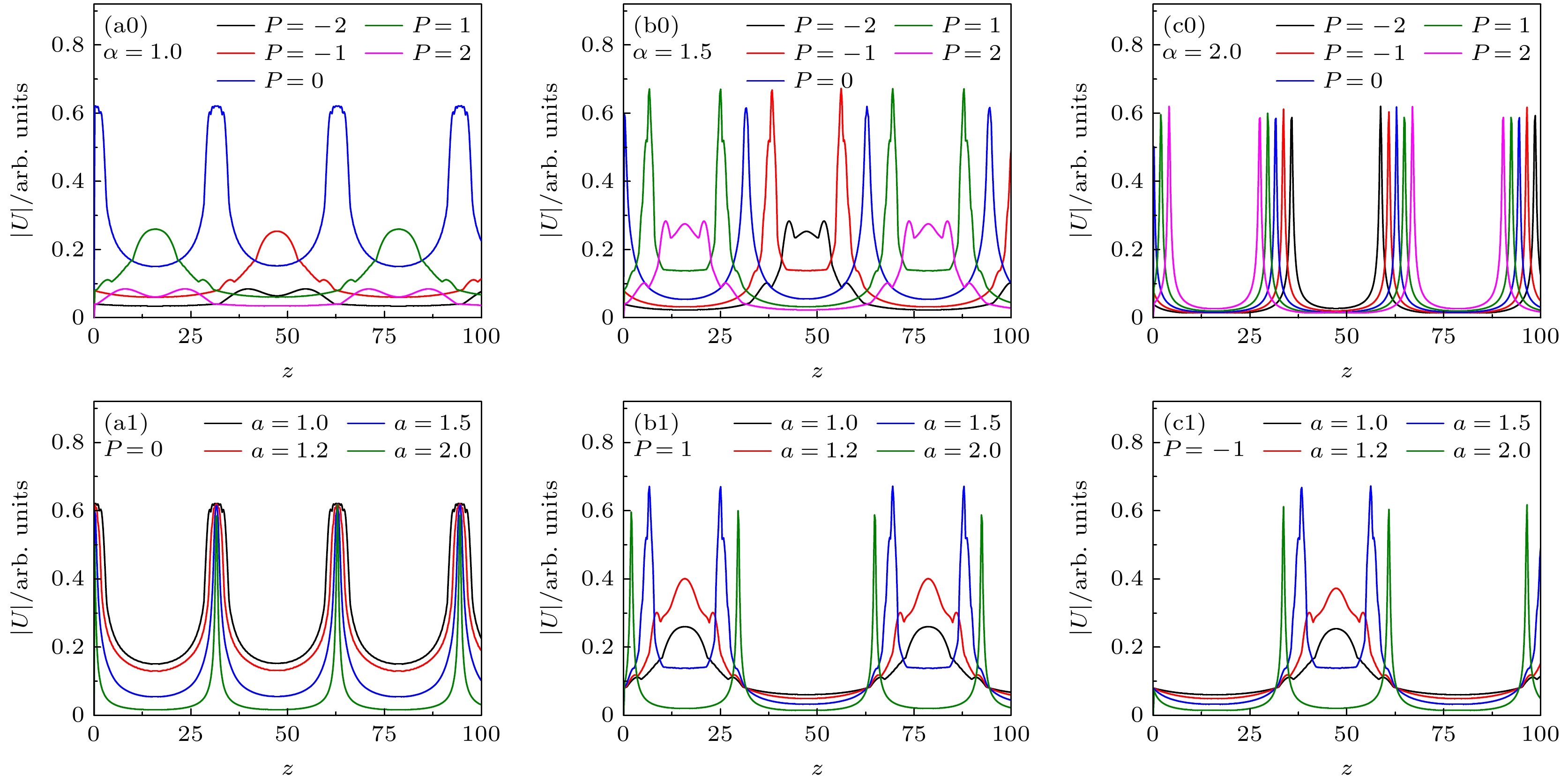
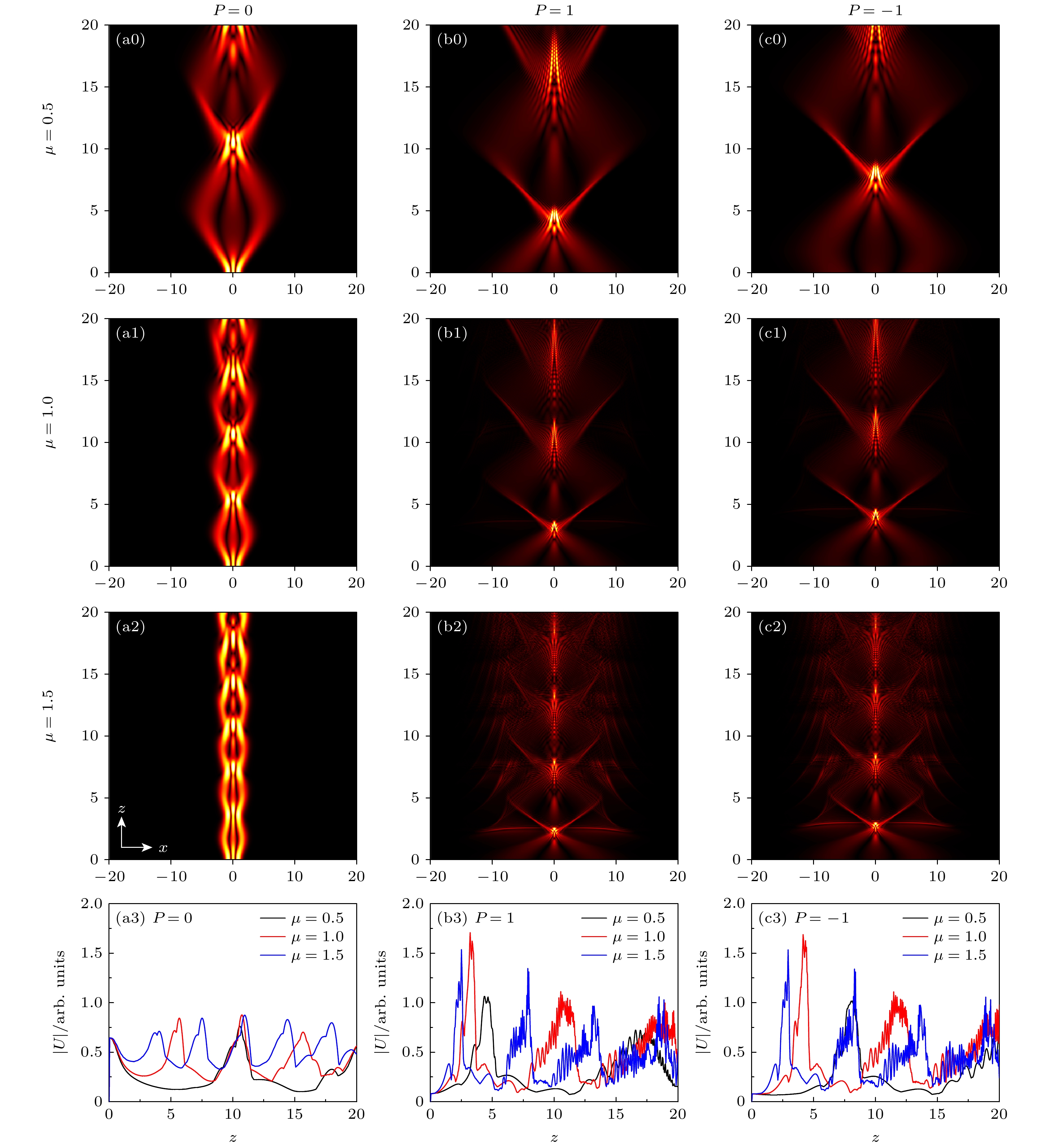
图 3 (a0)—(c2) 余弦调制$D\left( z \right) = \cos \left( {\varOmega z} \right)$下不同莱维指数$ \alpha $和QPM系数p的HG光束演化图, $\varOmega = 0.1$; (a3)—(c3)束宽随传输距离z变化图
Fig. 3. (a0)–(c2) Evolution of HG beams with different Lévy index $ \alpha $ and QPM coefficient p under cosine modulation $D\left( z \right) = \cos \left( {\varOmega z} \right)$, here $\varOmega = 0.1$; (a3)–(c3) beam width varies with transmission distance z.
图 4 $\varOmega = 0.1$时, 余弦调制$D\left( z \right) = \cos \left( {\varOmega z} \right)$下不同莱维指数$ \alpha $和QPM系数p的HG光束包络图
Fig. 4. Envelopes of HG beams for different Lévy index $ \alpha $ and QPM coefficient p under cosine modulation $D\left( z \right) = \cos \left( {\varOmega z} \right)$, here $\varOmega = 0.1$.
图 5 (a0)—(c2)不同调制频率$\varOmega $和QPM系数p下HG光束演化图, $\alpha = 1$; (a3)—(c3)束宽随传输距离z变化图
Fig. 5. (a0)–(c2) Evolution of HG beams for different modulation frequence and QPM coefficient p, here $\alpha = 1$; (a3)–(c3) the beam width varies with transmission distance z.
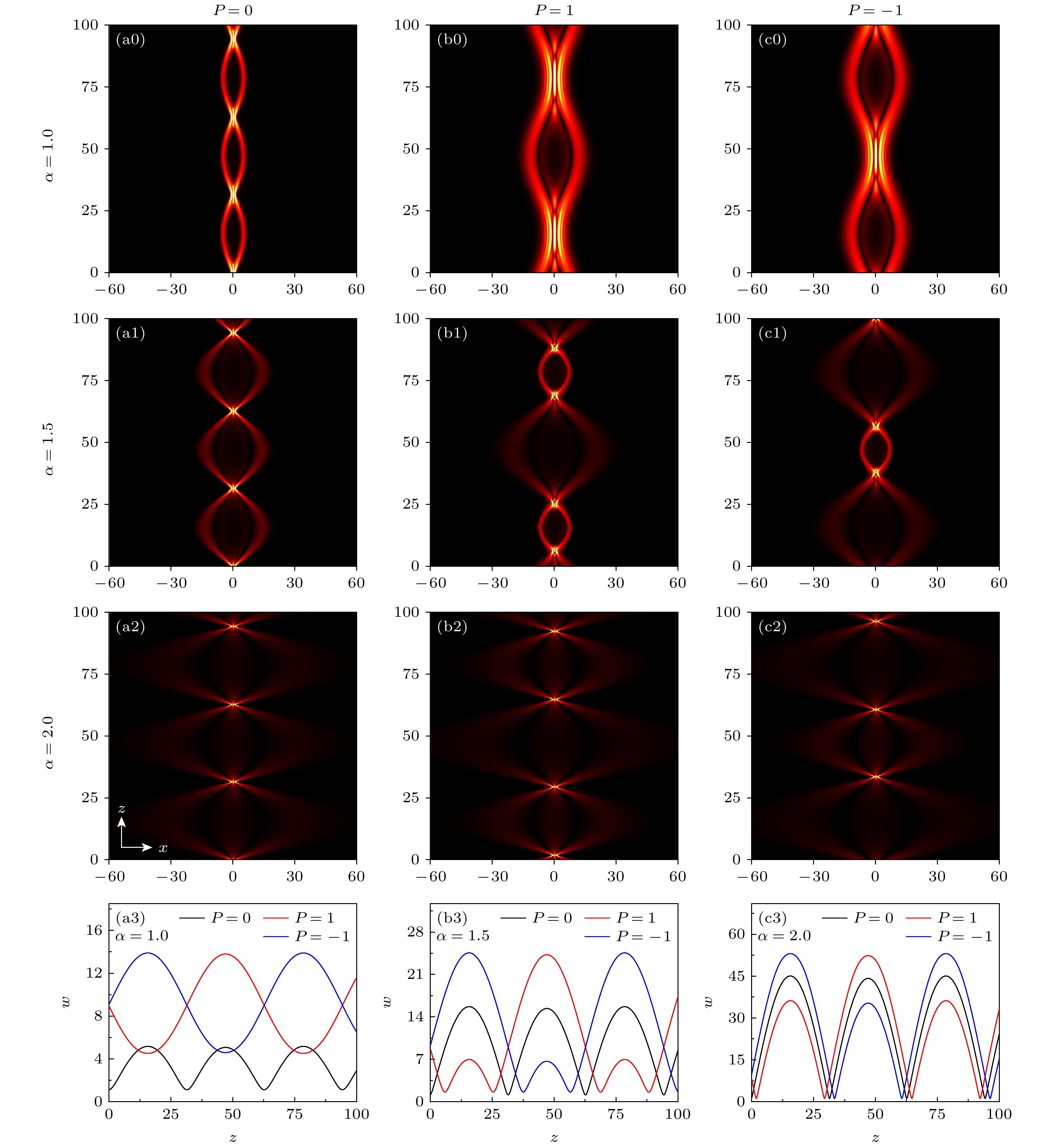
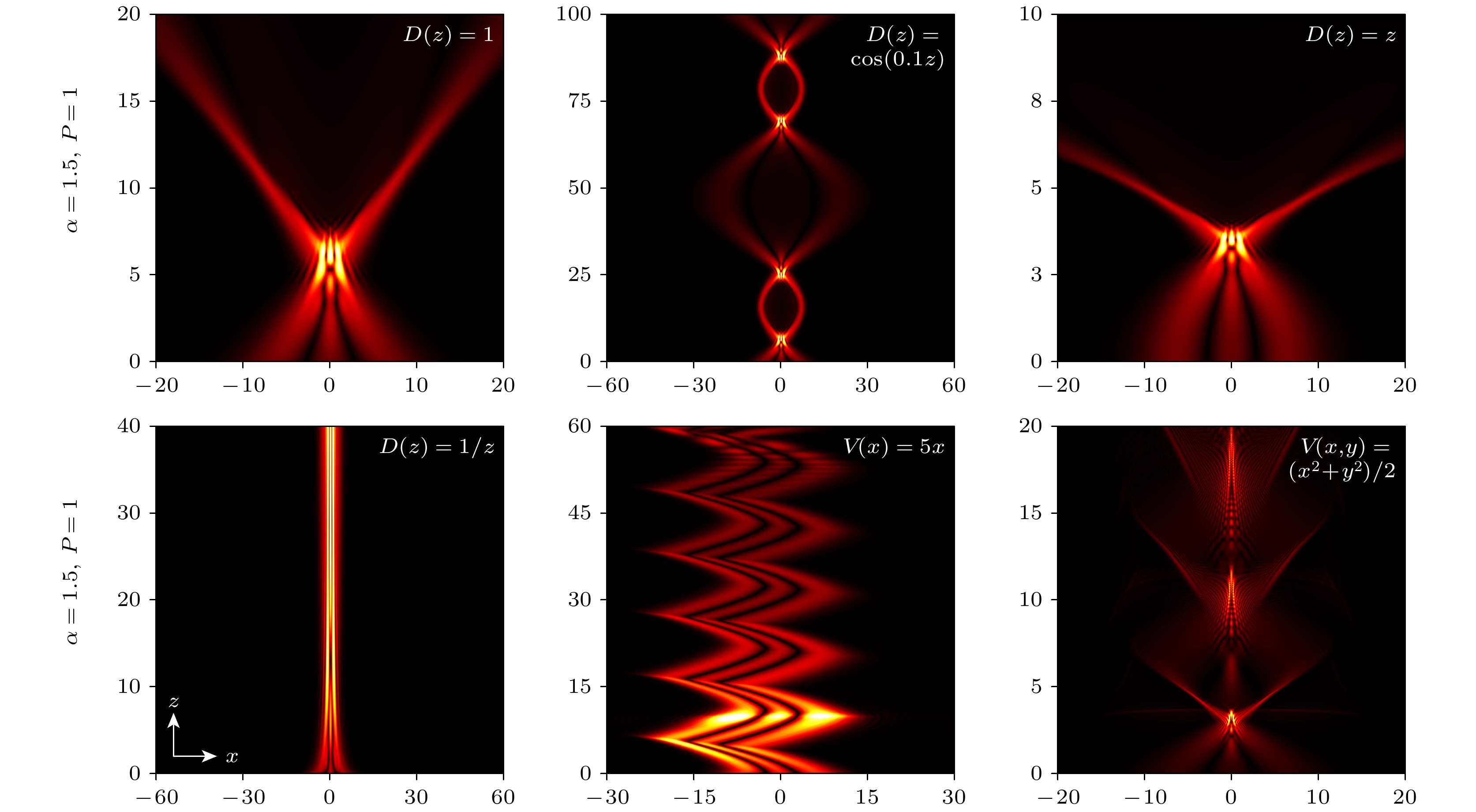
图 6 (a0)—(c2)线性调制$D\left( z \right) = z$下不同莱维指数$\alpha $和QPM系数p的HG光束演化图; (a3)—(c3) 图(a0)—(c2)对应的包络图
Fig. 6. (a0)–(c2) Evolution of HG beams with different Lévy index $\alpha $ and QPM coefficient p under linear modulation $D\left( z \right) = z$; (a3)–(c3) the corresponding envelopes to panels (a0)–(c2).
图 7 (a0)—(c2)幂函数调制$D(z) = 1/z$下不同莱维指数$\alpha $和QPM系数p的HG光束演化图; (a3)—(c3) 图(a0)—(c2)对应的包络图
Fig. 7. (a0)–(c2) Evolution of HG beams with different Lévy index $\alpha $ and QPM coefficient p under power function modulation $D\left( z \right) = {1 \mathord{\left/ {\vphantom {1 z}} \right. } z}$; (a3)–(c3) the corresponding envelopes to panels (a0)–(c2).
图 8 (a0)—(c2)不同线性系数$\beta $和QPM系数p下HG光束演化图, $\alpha = 1$; (a3)—(c3) 图(a0)—(c2)对应的包络图
Fig. 8. (a0)–(c2) Evolution of the HG beam for different linear coefficient $\beta $ and QPM coefficient p, here $\alpha = 1$; (a3)–(c3) the corresponding envelopes to panels (a0)–(c2).
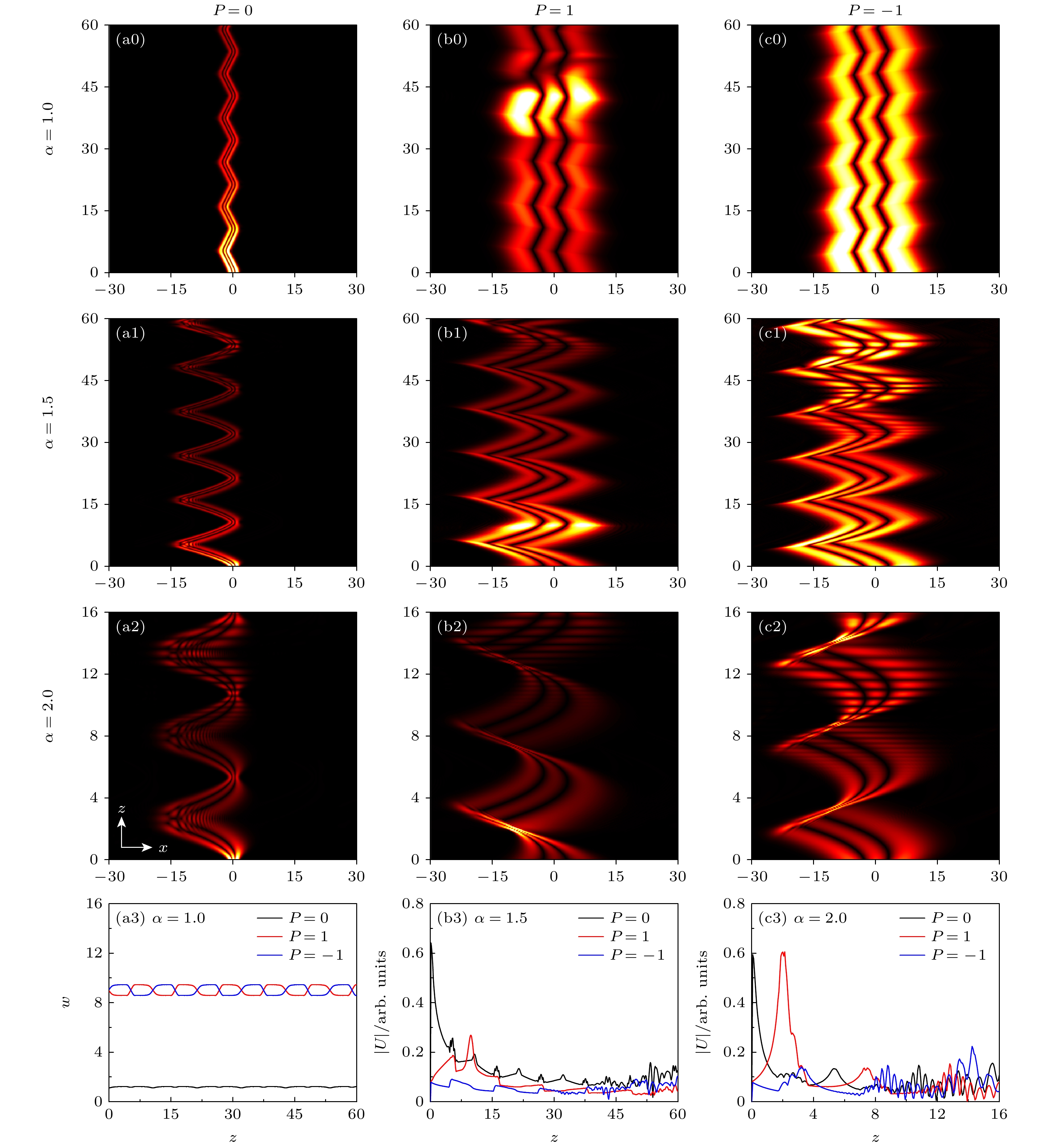
图 9 (a0)—(c2) 不同莱维指数$\alpha $和QPM系数p下HG光束演化图, $\beta = 5$; (a3)束宽随传输距离z变化图; (b3), (c3) 图(a1)—(c2)对应的包络图
Fig. 9. (a0)–(c2) Evolution of HG beams for different Lévy index $\alpha $ and QPM coefficient p, here $\beta = 5$; (a3) the beam width varies with transmission distance z; (b3), (c3) the corresponding envelopes to panels (a1)–(c2).
图 10 (a0)—(c2) 不同莱维指数$\alpha $和QPM系数p下HG光束演化图, $\mu = 1$; (a3)—(c3) 图(a0)—(c2)对应的包络图
Fig. 10. (a0)–(c2) Evolution of HG beams for different Lévy index $\alpha $ and QPM coefficient p, here $\mu = 1$; (a3)–(c3) the corresponding envelopes to panels (a0)–(c2).
图 11 (a0)—(c2) 不同抛物系数$\mu $和QPM系数p下HG光束演化图, $\alpha = 1.5$; (a3)—(c3) 图(a0)—(c2)对应的包络图
Fig. 11. (a0)–(c2) Evolution of HG beams for different parabolic coefficient $\mu $ and QPM coefficient p, here $\alpha = 1.5$; (a3)–(c3) the corresponding envelopes to panels (a0)–(c2).
-
[1] Wang S L, Xu J P, Yang Y P, Cheng M J 2024 Opt. Commun. 556 130258
Google Scholar
[2] Zhou J H, Hu Q S 2023 Opt. Express 31 38334
Google Scholar
[3] Qiu Y Z, Liu Z R 2024 Results Phys. 58 107457
Google Scholar
[4] Sun Z Y, Deng D, Pang Z G, Yang Z J 2024 Chaos, Solitons Fractals 178 114398
Google Scholar
[5] Sun Z Y, Li J, Bian R, Deng D, Yang Z J 2024 Opt. Express 32 9201
Google Scholar
[6] Arfan M, Khaleel N, Ghaffar A, Razzaz F, Saeed S M, Alanazi T M 2024 Opt. Quantum Electron. 56 135
Google Scholar
[7] Wang Q, Zhu J Y, Wang J, Yu H Y, Hu B B 2024 Chaos, Solitons Fractals 180 114580
Google Scholar
[8] 周王哲, 李雪鹏, 杨晶, 杨天利, 王小军, 刘炳杰, 王浩竹, 杨俊波, 彭钦军 2023 72 014204
Google Scholar
Zhou W Z, Li X P, Yang J, Yang T L, Wang X J, Liu B J, Wang H Z, Yang J B, Peng Q J 2023 Acta Phys. Sin. 72 014204
Google Scholar
[9] Wang S, Wang L, Zhang F R, Kong L J 2022 Chin. Phys. Lett. 39 104101
Google Scholar
[10] Wu S M, Wang Q, Gao X H, Wang Y 2018 Results Phys. 10 607
Google Scholar
[11] Song L M, Yang Z J, Li X L, Zhang S M 2020 Appl. Math. Lett. 102 106114
Google Scholar
[12] Fan X L, Ji X L, Wang H, Deng Y, Zhang H 2021 J. Opt. Soc. Am. A 38 168
Google Scholar
[13] Sharma V, Thakur V, Singh A, Kant N 2021 Chin. J. Phys. 71 312
Google Scholar
[14] Ebel S, Talebi N 2023 Commun. Phys. 6 179
Google Scholar
[15] Che J R, Zheng Y X, Liang G, Guo Q 2023 Chin. Phys. B 32 104207
Google Scholar
[16] Saad F, Benzehoua H, Belafhal A 2024 Opt. Quantum Electron. 56 130
Google Scholar
[17] Laskin N 2000 Phys. Lett. A 268 298
Google Scholar
[18] Longhi S 2015 Opt. Lett. 40 1117
Google Scholar
[19] Zhang L F, Li C X, Zhong H Z, Xu C G, Lei D J, Li Y, Fan D Y 2016 Opt. Express 24 14406
Google Scholar
[20] Huang X W, Deng Z X, Shi X H, Fu X Q 2017 J. Opt. Soc. Am. B: Opt. Phys. 34 2190
Google Scholar
[21] Zhang L F, Zhang X, Wu H Z, Li C X, Pierangeli D, Gao Y X, Fan D Y 2019 Opt. Express 27 27936
Google Scholar
[22] Zang F, Wang Y, Li L 2018 Opt. Express 26 23740
Google Scholar
[23] Xin W, Song L J, Li L 2021 Opt. Commun. 480 126483
Google Scholar
[24] Huang X W, Deng Z X, Fu X Q 2017 J. Opt. Soc. Am. B: Opt. Phys. 34 976
Google Scholar
[25] Chen W J, Wang T, Wang J, Mu Y N 2021 Opt. Commun. 496 127136
Google Scholar
[26] Tan C, Lei T, Zou M, Liang Y, Tang P H, Liu M W 2024 Opt. Commun. 557 130358
Google Scholar
[27] Wen J J, Wang H W, Gao R, Ren S M, Guo T, Xiao Y 2023 Optik 276 170586
Google Scholar
[28] Tan C, Liang Y, Zou M, Lei T, Tang P H, Liu M W 2024 J. Opt. Soc. Am. B: Opt. Phys. 41 921
Google Scholar
[29] Zhang L F, Liu K, Zhong H Z, Zhang J G, Deng J Q, Li Y, Fan D Y 2015 Sci. Rep. 5 11843
Google Scholar
[30] Zhang J G, He J 2017 IEEE Photonics J. 9 1
Google Scholar
[31] Zhan K Y, Jiao R Y, Wang J, Zhang W Q, Yang Z D, Liu B 2020 Ann. Phys. 532 1900546
Google Scholar
[32] Zhan K Y, Zhang W Q, Jiao R Y, Dou L C, Liu B 2020 Opt. Commun. 474 126156
Google Scholar
[33] Jiao C Y, Huang X W, Bai Y F, Fu X Q 2023 J. Opt. Soc. Am. A 40 2019
Google Scholar
下载:
计量
- 文章访问数: 1715
- PDF下载量: 48
- 被引次数: 0
