色味锁夸克物质与夸克星

初鹏程; 刘鹤; 杜先斌

doi:10.7498/aps.73.20231649

摘要

讨论了零温、强磁场下基于准粒子模型的奇异夸克物质、色味锁夸克物质的热力学性质. 结果表明色味锁夸克物质比奇异夸克物质更稳定, 压强会随着色味锁态能隙常数的增大而增加. 并且发现强磁场下磁星的最大质量会随着色味锁夸克物质的能隙常数的增加而增加, 磁星的潮汐形变率会随着能隙常数的增加而增加, 磁星最大质量的中心密度会随着能隙常数的增加而降低. 结果还说明考虑色味锁态得到的磁星质量半径关系可以满足最近实验观测 PSR J0740 + 6620, PSR J0030 + 0451, 和 HESS J1731-347所给出的质量半径约束.

关键词:

夸克物质 /
夸克星 /
磁星

Abstract

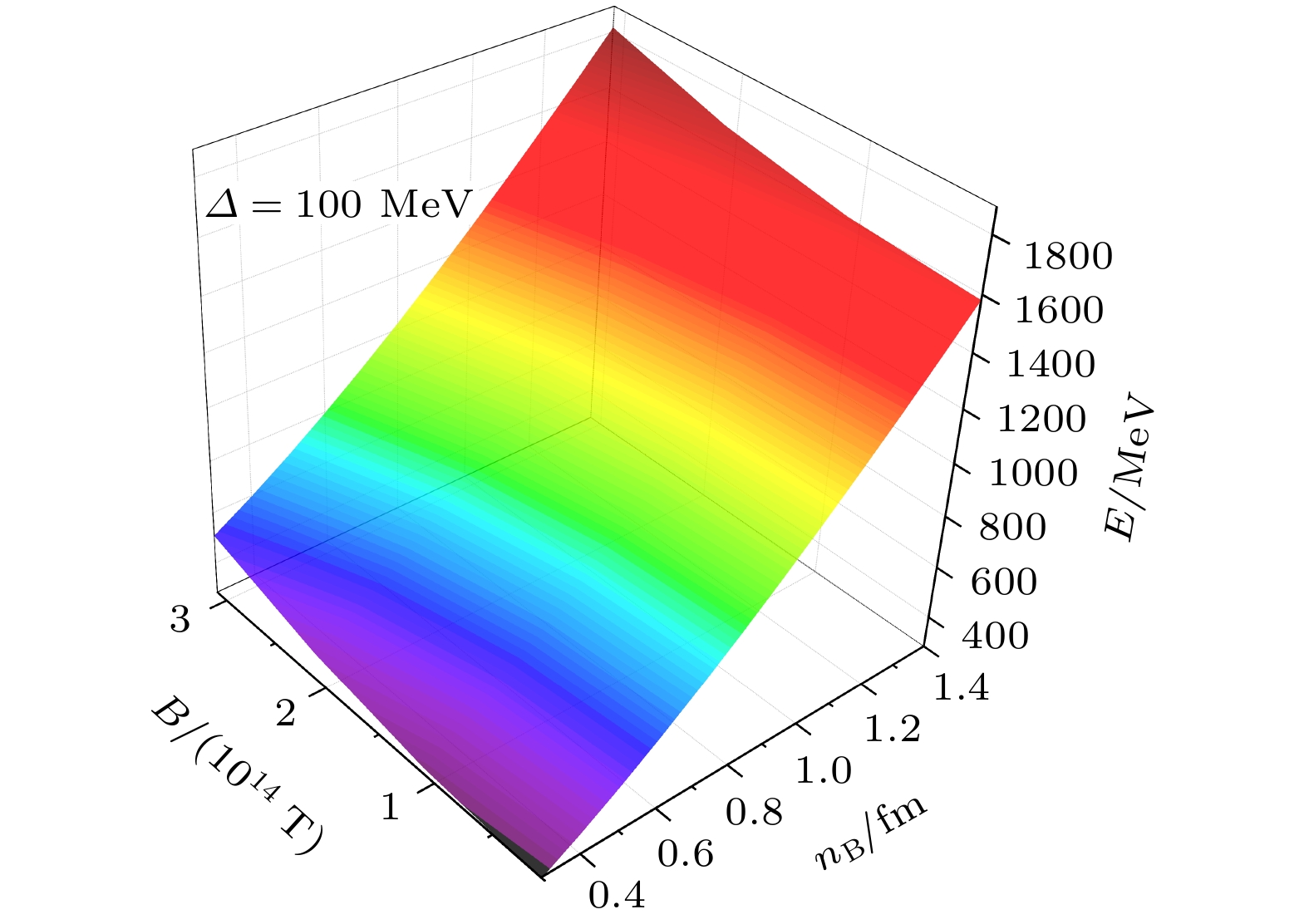
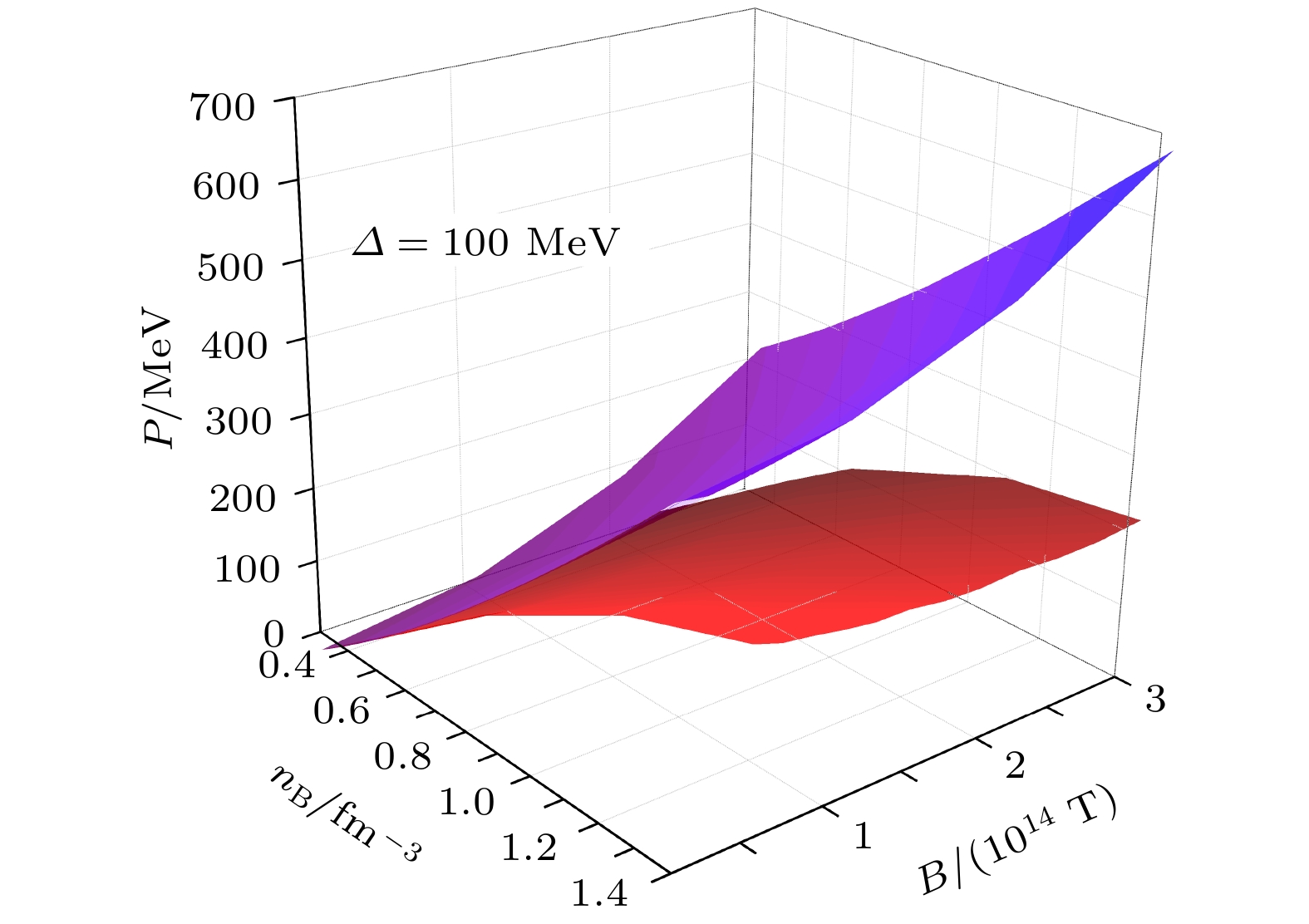
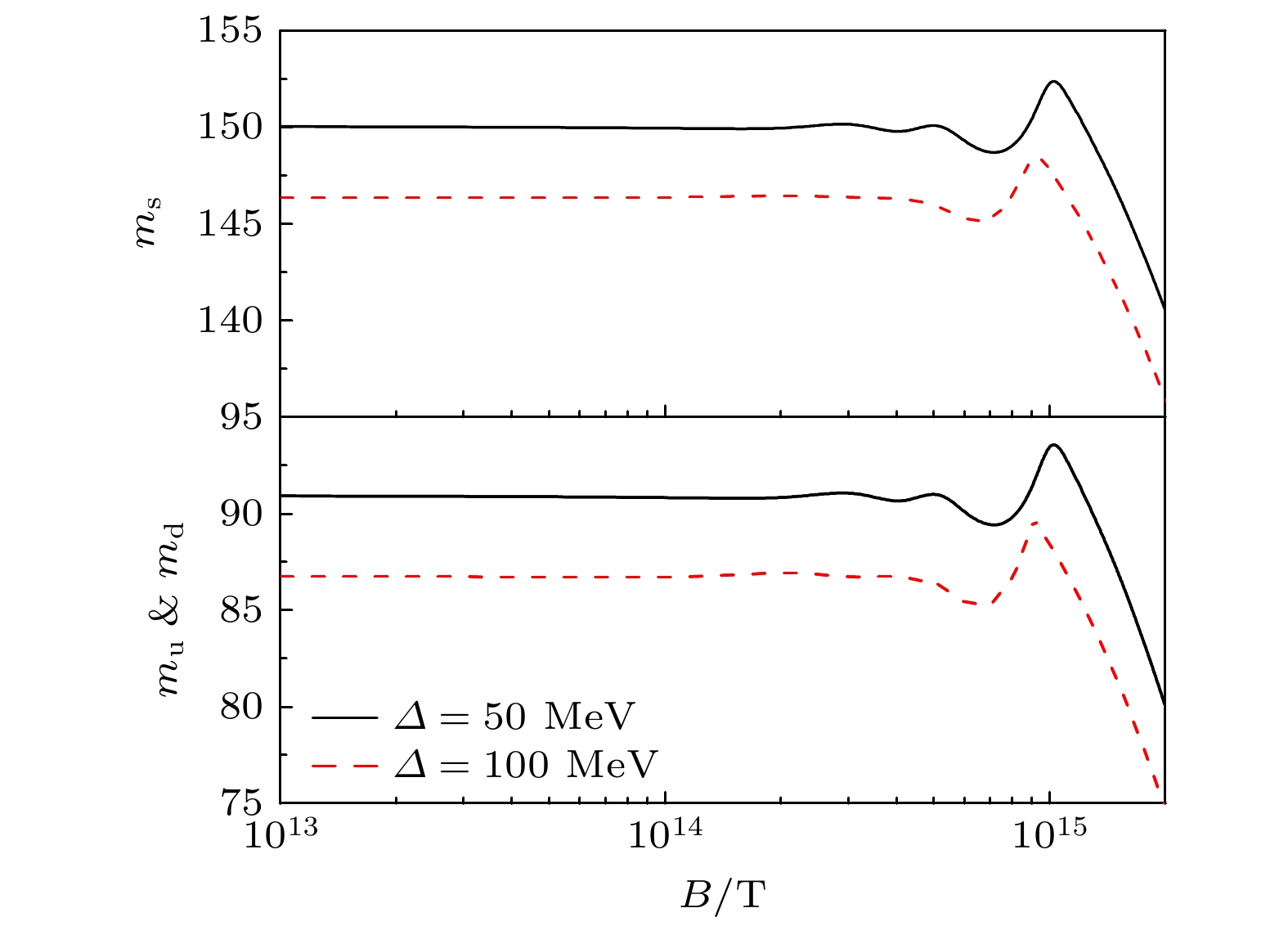
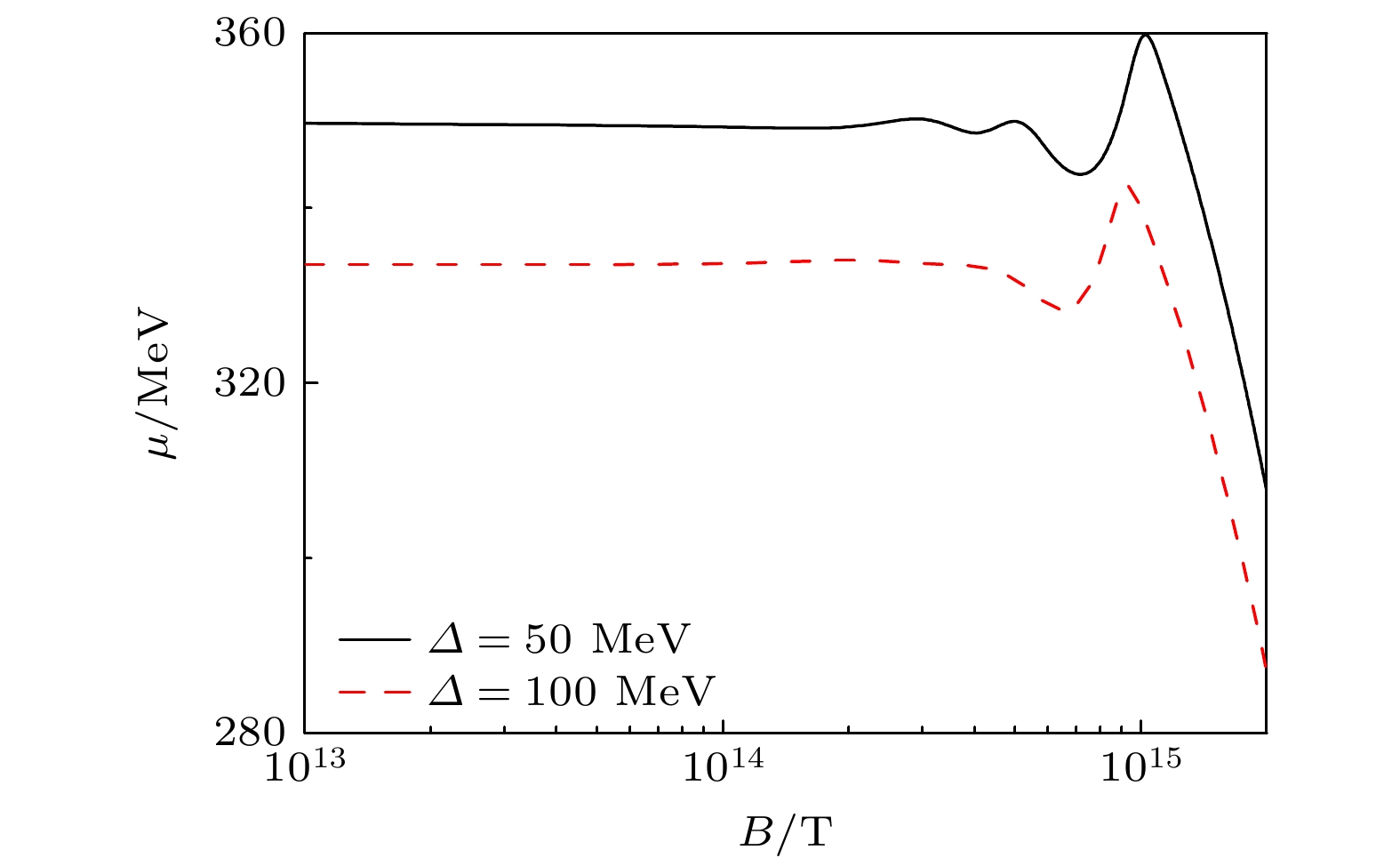
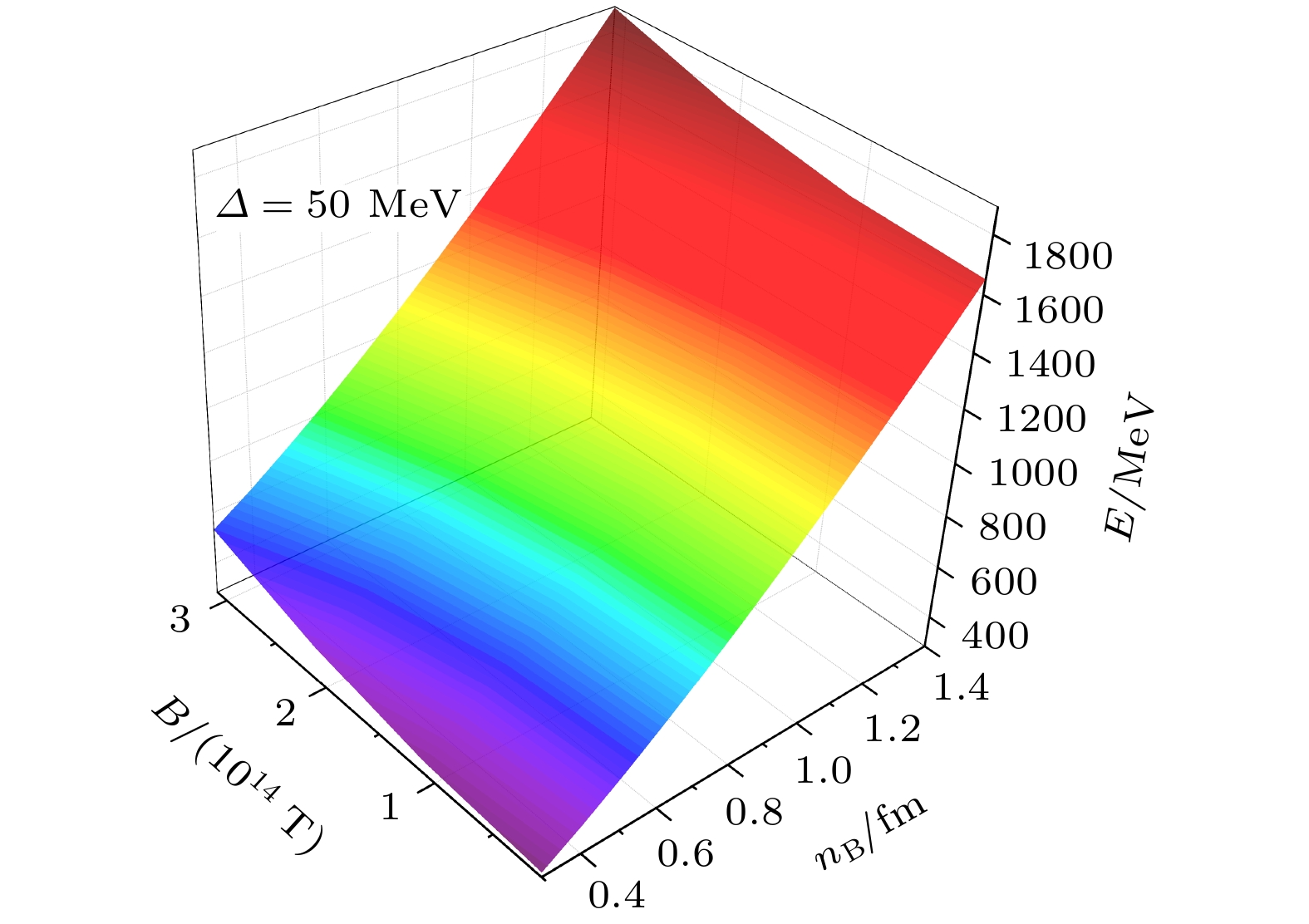
In this work, we investigate the thermodynamical properties of strange quark matter (SQM) and color-flavor-locked (CFL) quark matter under strong magnetic fields by using a quasiparticle model. We calculate the energy density and the corresponding anisotropic pressure of both SQM and CFL quark matter. Our results indicate that CFL quark matter exhibits greater stability than the SQM, and the pressure of CFL quark matter increases with the energy gap constant $\varDelta $ increasing. We also observe that the oscillation effects coming from the lowest Landau level can be reduced by increasing the energy gap constant $ \varDelta $, which cannot be observed in SQM under a similar strong magnetic field. The equivalent quark mass for u, d, and s quark and the chemical potential for each flavor of quarks decrease with the energy gap constant $ \varDelta $ increasing, which matches the conclusion that CFL quark matter is more stable than SQM. From the calculations of the magnetars with SQM and CFL quark matter, we find that the maximum mass of magnetars increases with the energy gap constant $\varDelta $ increasing for both the longitudinal and the transverse orientation distribution of magnetic field. Additionally, the tidal deformability of the magnetars increases with the $\varDelta $ increasing. On the other hand, the central baryon density of the maximum mass of the magnetars decreases with the $\varDelta $ increasing. The results also indicate that the mass-radius lines of the CFL quark star can also satisfy the new estimates of the mass-radius region from PSR J0740 + 6620, PSR J0030 + 0451, and HESS J1731-347.

Keywords:

quark matter /
quark star /
magnetar

作者及机构信息

青岛理工大学理学院, 青岛　266033

通信作者: 初鹏程, kyois@126.com ; 刘鹤, liuhe@qut.edu.cn ;

基金项目: 国家自然科学基金(批准号: 11975132, 12205158, 11505100)和山东省自然科学基金(批准号: ZR2022JQ04, ZR2021QA037, ZR2019YQ01)资助的课题.

Authors and contacts

School of Science, Qingdao University of Technology, Qingdao 266033, China

Corresponding author: Chu Peng-Cheng, kyois@126.com ; Liu He, liuhe@qut.edu.cn ;

Funds: Project supported by the National Natural Science Foundation of China (Grant Nos. 11975132, 12205158, 11505100) and the Natural Science Foundation of Shandong Province, China(Grant Nos. ZR2022JQ04, ZR2021QA037, ZR2019YQ01).

文章全文 : translate this paragraph

参考文献

[1]	Glendenning N K 2000 Compact Stars (2nd Ed.) (New York: Spinger-Verlag, Inc.
[2]	Weber F 1999 Pulsars as Astrophyical Laboratories for Nuclear and Particle Physics (London: IOP Publishing Ltd.
[3]	Lattimer J M, Prakash M 2004 Science 304 536 Google Scholar
[4]	Steiner A W, Prakash M, Lattimer J M, Ellis P J 2005 Phys. Rep. 410 325 Google Scholar
[5]	Demorest P 2010 Nature 467 1081 Google Scholar
[6]	Antoniadis J 2013 Science 340 6131 Google Scholar
[7]	Shahbaz T, Casares J 2018 Astrophys. J. 859 54 Google Scholar
[8]	Cromartie H T, Fonseca E, Ransom S M et al. 2020 Nat. Astron. Lett. 4 72
[9]	Fonseca E, Cromartie H T, Pennucci T T, et al. 2021 Astrophys. J. Lett. 915 L12 Google Scholar
[10]	Miller M C, Lamb F K, Dittmann A J, et al. 2021 Astrophys. J. Lett. 918 L28 Google Scholar
[11]	Abbott R 2020 Astrophys. J. Lett. 896 L44 Google Scholar
[12]	Ivanenko D, Kurdgelaidze D F 1969 Lett. Nuovo Cimento 2 13 Google Scholar
[13]	Itoh N 1970 Prog. Theor. Phys. 44 291 Google Scholar
[14]	Bodmer A R 1971 Phys. Rev. D 4 1601 Google Scholar
[15]	Witten E 1984 Phys. Rev. D 30 272 Google Scholar
[16]	Farhi E, Jaffe R L 1984 Phys. Rev. D 30 2379 Google Scholar
[17]	Alcock C, Farh E, Olinto A 1986 Astrophys. J. 310 261 Google Scholar
[18]	Weber F 2005 Prog. Part. Nucl. Phys. 54 193 Google Scholar
[19]	Bombaci I, Parenti I, Vidana I 2004 Astrophys. J. 614 314 Google Scholar
[20]	Staff J, Ouyed R, Bagchi M 2007 Astrophys. J. 667 340 Google Scholar
[21]	Herzog T M, Röpke F K 2011 Phys. Rev. D 84 083002 Google Scholar
[22]	Stephanov M A, Rajagopal K, Shuryak E V 1998 Phys. Rev. Lett. 81 4816 Google Scholar
[23]	Terazawa H 1979 INS-Report (Tokyo: Univ. of Tokyo) p336
[24]	Alford M, Reddy S 2003 Phys. Rev. D 67 074024 Google Scholar
[25]	Alford M, Jotwani P, Kouvaris C, Kundu J, Rajagopal K 2005 Phys. Rev. D 71 114011 Google Scholar
[26]	Baldo M 2003 Phys. Lett. B 562 153 Google Scholar
[27]	Ippolito N D, Ruggieri M, Rischke D H, Sedrakian A, Weber F 2008 Phys. Rev. D 77 023004 Google Scholar
[28]	Lai X Y, Xu R X 2011 Res. Astron. Astrophys. 11 687 Google Scholar
[29]	Avellar M G B de, Horvath J E, Paulucci L 2011 Phys. Rev. D 84 043004 Google Scholar
[30]	Bonanno L, Sedrakian A 2012 A&A 539 A16 Google Scholar
[31]	Chu P C, Wang B, Jia Y Y, Dong Y M, Wang S M, Li X H, Zhang L, Zhang X M, Ma H Y 2016 Phys. Rev. D 94 123014 Google Scholar
[32]	Chu P C, Li X H, Wang B, Dong Y M, Jia Y Y, Wang S M, Ma H Y 2017 Eur. Phys. J. C 77 512 Google Scholar
[33]	Chu P C, Zhou Y, Chen C, Li X H, Ma H Y 2020 J. Phys. G: Nucl. Part. Phys. 47 085201 Google Scholar
[34]	Bailin D and Love A 1984 Phys. Rep. 107 325 Google Scholar
[35]	Alford M G, Rajagopal K, Reddy S, Wilczek F 2001 Phys. Rev. D 64 074017 Google Scholar
[36]	Shovkovy I A 2005 Found. Phys. 35 1309 Google Scholar
[37]	Rajagopal K, Wilczek F 2001 Phys. Rev. L 86 3492 Google Scholar
[38]	Alford M G, Rajagopal K, Schaefer T, Schmitt A 2008 Rev. Mod. Phys. 80 1455 Google Scholar
[39]	Lugones G, Horvath J E 2003 Astron. Astrophys. 403 173 Google Scholar
[40]	Horvath J E, Lugones G 2004 Astron. Astrophys. 422 L1 Google Scholar
[41]	Woltjer L 1964 Astrophys. J. 140 1309 Google Scholar
[42]	Mihara T A 1990 Nature 346 250 Google Scholar
[43]	Chanmugam G 1992 Annu. Rev. Astron. Astrophys. 30 143 Google Scholar
[44]	Lai D, Shapiro S L 1991 Astrophys. J. 383 745 Google Scholar
[45]	Ferrer E J, Incera V, Keith J P, Portillo I, Springsteen P L 2010 Phys. Rev. C 82 065802 Google Scholar
[46]	Bandyopadhyay D, Chakrabarty S, Pal S 1997 Phys Rev. Lett. 79 2176 Google Scholar
[47]	Bandyopadhyay D, Pal S, Chakrabarty S 1998 J. Phys. G: Nucl. Part. Phys. 24 1647 Google Scholar
[48]	Menezes D P, Pinto M, Benghi, Avancini S, Providência C 2009 Phys. Rev. C 79 035807 Google Scholar
[49]	Menezes D P, Pinto M, Benghi, Avancini S, Providência C 2009 Phys. Rev. C 80 065805 Google Scholar
[50]	Ryu C Y, Kim K S, Cheoun Myung-Ki 2010 Phys. Rev. C 82 025804 Google Scholar
[51]	Ryu C Y, Cheoun Myung-Ki, Kajino T, Maruyama T, Mathews Grant J 2012 Astropart. Phys. 38 25 Google Scholar
[52]	Li X H, Gao Z F, Li X D, Xu Y, Wang P, WangN, Peng Q H 2016 Int. J. Mod. Phys. D 25(1) 1650002 Google Scholar
[53]	Gao Z F, Wang N, Shan H, L i, X D, Wang W 2017 Astrophys. J. 849 19 Google Scholar
[54]	Deng Z L, Gao Z F, Li X D, Shao Y 2020 Astrophys. J. 892 4 Google Scholar
[55]	Yan F Z, Gao Z F, Yang W S, Dong A J 2021 Astron. Nachr. 342 249 Google Scholar
[56]	Wang H, Gao Z F, Jia H Y, Wang N, Li X 2020 Universe 6 63 Google Scholar
[57]	Li B P, Gao Z F 2023 Astron. Nachr. 344 e20220111
[58]	Deng Z L, Li X D, Gao Z F, Shao Y 2021 Astrophys. J. 909 174 Google Scholar
[59]	G ao, Z F, Omar N, Shi X C, Wang N 2019 Astron. Nachr. 340 1030 Google Scholar
[60]	Lander, S K 2023 Astrophys.J. 947 L16 Google Scholar
[61]	Dong J M 2021 Mon. Not. R. Astron. Soc. 500 1505
[62]	Fu G Z, Xing C C, Wang N 2020 Eur. Phys. J. C 80 582 Google Scholar
[63]	Schertler K, Greiner C, Thoma M H, Schertler K, Greiner C, Thoma M H 1997 Nucl. Phys. A 616 659 Google Scholar
[64]	Pisarski R D 1989 Nucl. Phys. A 498 423
[65]	Wen X J 2009 J. Phys. G: Nucl. Part. Phys. 36 025011 Google Scholar
[66]	Zhang Z, Chu P C, Li X H, Liu H, Zhang X M 2021 Phys. Rev. D 103 103021 Google Scholar
[67]	Chu P C, Chen L W 2014 Astrophys. J. 780 135 Google Scholar
[68]	Chu P C 2018 Phys. Lett. B 778 447 Google Scholar
[69]	Chu P C, Chen L W 2017 Phys. Rev. D 96 103001 Google Scholar
[70]	Chodos A, Jaffe R L, Ohnson K, Thorn C B, Weisskopf V F 1974 Phys. Rev. D 9 3471 Google Scholar
[71]	Alford M, Braby M, Paris M, Reddy S 2005 Astrophys. J. 629 969 Google Scholar
[72]	Rehberg P, Klevansky S P, Hüfner J 1996 Phys. Rev. C 53 410 Google Scholar
[73]	Hanauske M, Satarov L M, Mishustin I N, Stocker H, Greiner W 2001 Phys. Rev. D 64 043005 Google Scholar
[74]	Rüster S B, Rischke D H 2004 Phys. Rev. D 69 045011 Google Scholar
[75]	Menezes D P, Providencia C, Melrose D B 2006 J. Phys. G 32 1081 Google Scholar
[76]	Chao J Y, Chu P C, Huang M 2013 Phys. Rev. D 88 054009 Google Scholar
[77]	Chu P C, Wang X, Chen L W, Huang M 2015 Phys. Rev. D 91 023003 Google Scholar
[78]	Chu P C, Wang B, Ma H Y, Dong Y M, Chang S L, Zheng C H, Liu J T, Zhang X M 2016 Phys. Rev. D 93 094032 Google Scholar
[79]	Chu P C, Chen L W 2017 Phys. Rev. D 96 083019 Google Scholar
[80]	Roberts C D, Williams A G 1994 Prog. Part. Nucl. Phys. 33 477 Google Scholar
[81]	Zong H S, Chang L, Hou F Y, Sun W M, Liu Y X 2005 Phys. Rev. C 71 015205 Google Scholar
[82]	Peng G X, Chiang H C, Yang J J, Li L, Liu B 1999 Phys. Rev. C 61 015201 Google Scholar
[83]	Peng G X, Chiang H C, Zou B S, Ning P Z, Luo S J 2000 Phys. Rev. C 62 025801 Google Scholar
[84]	Peng G X, Li A, Lombardo U 2008 Phys. Rev. C 77 065807 Google Scholar
[85]	Li A, Peng G X, Lu J F 2011 Res. Astron. Astrophys. 11 482 Google Scholar
[86]	Schertler K, Greiner C, Thoma M H 1997 Nucl. Phys. A 616 659
[87]	Schertler K, Greiner C, Sahu P K, Thoma M H 1998 Nucl. Phys. A 637 451
[88]	Alford M, Kouvaris C, Rajagopal K 2005 Phys. Rev. D 71 054009 Google Scholar
[89]	Giannakis I, Hou D, Ren H C, et al. 2004 Phys. Rev. Lett. 93 232301 Google Scholar
[90]	董爱军, 高志福, 杨晓峰等 2023 72 030502 Google Scholar Dong A J, Gao Z F, Yang X F, et al 2023 Acta Phys. Sin. 72 030502 Google Scholar
[91]	Ferrer E J, Vivian de la Incera 2005 Phys. Rev. Lett. 95 152002 Google Scholar
[92]	Ferrer E J, Vivian de la Incera, Cristina Manuel 2006 Nucl. Phys. B 747 88 Google Scholar
[93]	Feng B, Ferrer E J, Vivian de la Incera 2011 Nucl. Phys. B 853 213 Google Scholar
[94]	Paulucci L, Ferrer E J, Vivian de la Incera, Horvath J E 2011 Phys. Rev. D 83 043009 Google Scholar
[95]	Isayev A A, Yang J 2011 Phys. Rev. C 84 065802 Google Scholar
[96]	Isayev A A, Yang J 2012 Phys. Lett. B 707 163 Google Scholar
[97]	Isayev A A, Yang J 2013 J. Phys. G: Nucl. Part. Phys. 40 035105 Google Scholar
[98]	Feng B, Hou D F, Ren H C, Wu P P 2010 Phys. Rev. Lett. 105 042001 Google Scholar
[99]	Oppenheimer J R, Volkoff G M 1939 Phys. Rev. 33 374
[100]	Gao Z F, Li X D, Wang N, Yuan J P, Wang P, Peng Q H, Du Y J 2016 Mon. Not. R. Astron. Soc. 456 55 Google Scholar
[101]	Gao Z F, Wang N, Peng Q H, Li X D, Du Y J 2013 Mod. Phys. Lett. A 28 1350138 Google Scholar
[102]	Chu P C, Chen L W, Wang X 2014 Phys. Rev. D 90 063013 Google Scholar
[103]	Miller M C, Lamb F K, Dittmannet A J, et al. 2019 Astrophys. J. Lett. 887 L24 Google Scholar
[104]	Doroshenko V, Suleimanov V, Pühlhofer G, Santangelo A 2022 Nat. Astron. 6 1444 Google Scholar

施引文献

图 1 $ \varDelta = 50 $ MeV时色味锁夸克物质的能量密度随重子数密度与磁场的变化

Fig. 1. Energy density of CFL quark matter as functions of baryon density and magnetic field with $ \varDelta = 50 $ MeV.

下载: 全尺寸图片幻灯片

图 2 $ \varDelta = 100 $ MeV时色味锁夸克物质的能量密度随重子数密度与磁场的变化

Fig. 2. Energy density of CFL quark matter as functions of baryon density and magnetic field with $ \varDelta = 100 $ MeV.

下载: 全尺寸图片幻灯片

图 3 $ \varDelta = 50 $ MeV时色味锁夸克物质的压强随重子数密度与磁场的变化

Fig. 3. Pressure of CFL quark matter as functions of baryon density and magnetic field with $ \varDelta = 50 $ MeV.

下载: 全尺寸图片幻灯片

图 4 $ \varDelta = 100 $ MeV时色味锁夸克物质的压强随重子数密度与磁场的变化

Fig. 4. Pressure of CFL quark matter as functions of baryon density and magnetic field with $ \varDelta = 100 $ MeV.

下载: 全尺寸图片幻灯片

图 5 $ \varDelta = 50 $ MeV和$ \varDelta = 100 $ MeV时色味锁夸克物质的压强不对称度随磁场的变化

Fig. 5. Pressure anisotropy of CFL quark matter as functions of magnetic field with $ \varDelta = 50, 100 $ MeV.

下载: 全尺寸图片幻灯片

图 6 $ \varDelta = 50 $ MeV和$ \varDelta = 100 $ MeV时u, d, s三味夸克的有效质量随磁场的变化规律

Fig. 6. Equivalent quark mass for u, d, and s quarks as functions of magnetic fields B with $ \varDelta = 50 $ MeV and $ \varDelta = 100 $ MeV.

下载: 全尺寸图片幻灯片

图 7 $ \varDelta = 50 $ MeV和$ \varDelta = 100 $ MeV时u, d, s三味夸克的化学势随磁场的变化规律

Fig. 7. Chemical potential of u, d, and s quarks as functions of magnetic fields B with $ \varDelta = 50 $ MeV and $ \varDelta = 100 $ MeV

下载: 全尺寸图片幻灯片

图 8 磁场与零磁场下色味锁相夸克星质量半径关系

Fig. 8. Mass-radius relation of QSs with CFL quark phase under magnetic fields.

下载: 全尺寸图片幻灯片

图 9 奇异夸克星与色味锁相磁星最大质量随磁场的变化关系

Fig. 9. Maximum star mass of magnetars as a function of magnetic field $ B_0 $ with SQM and CFL quark phase by considering transverse magnetic field orientation and longitudinal orientation.

下载: 全尺寸图片幻灯片

表 1 不同磁场方向分布情况下($ B_0 = 4\times \text{10}^{18} $G)磁星最大质量中心密度、1.4倍太阳质量潮汐形变率随Δ的变化

Table 1. The central density and tidal deformability of the magnetars considering “radial orientation” and “transverse orientation” at $ B_0 = 4\times \text{10}^{18} $G with g-2 within quasiparticle model with different Δ.

$ B_{{/ /}} $ $ B_{{/ /}} $ $ B_{\perp} $ $ B_{\perp} $

Δ/MeV 50 100 50 100

$ n_{\mathrm{c}} $/$ \text{fm}^{-3} $ 0.95 0.82 0.91 0.8

$ \varLambda_{1.4} $/MeV 741 1256 805 1351

下载: 导出CSV

map

[1]	Glendenning N K 2000 Compact Stars (2nd Ed.) (New York: Spinger-Verlag, Inc.
[2]	Weber F 1999 Pulsars as Astrophyical Laboratories for Nuclear and Particle Physics (London: IOP Publishing Ltd.
[3]	Lattimer J M, Prakash M 2004 Science 304 536 Google Scholar
[4]	Steiner A W, Prakash M, Lattimer J M, Ellis P J 2005 Phys. Rep. 410 325 Google Scholar
[5]	Demorest P 2010 Nature 467 1081 Google Scholar
[6]	Antoniadis J 2013 Science 340 6131 Google Scholar
[7]	Shahbaz T, Casares J 2018 Astrophys. J. 859 54 Google Scholar
[8]	Cromartie H T, Fonseca E, Ransom S M et al. 2020 Nat. Astron. Lett. 4 72
[9]	Fonseca E, Cromartie H T, Pennucci T T, et al. 2021 Astrophys. J. Lett. 915 L12 Google Scholar
[10]	Miller M C, Lamb F K, Dittmann A J, et al. 2021 Astrophys. J. Lett. 918 L28 Google Scholar
[11]	Abbott R 2020 Astrophys. J. Lett. 896 L44 Google Scholar
[12]	Ivanenko D, Kurdgelaidze D F 1969 Lett. Nuovo Cimento 2 13 Google Scholar
[13]	Itoh N 1970 Prog. Theor. Phys. 44 291 Google Scholar
[14]	Bodmer A R 1971 Phys. Rev. D 4 1601 Google Scholar
[15]	Witten E 1984 Phys. Rev. D 30 272 Google Scholar
[16]	Farhi E, Jaffe R L 1984 Phys. Rev. D 30 2379 Google Scholar
[17]	Alcock C, Farh E, Olinto A 1986 Astrophys. J. 310 261 Google Scholar
[18]	Weber F 2005 Prog. Part. Nucl. Phys. 54 193 Google Scholar
[19]	Bombaci I, Parenti I, Vidana I 2004 Astrophys. J. 614 314 Google Scholar
[20]	Staff J, Ouyed R, Bagchi M 2007 Astrophys. J. 667 340 Google Scholar
[21]	Herzog T M, Röpke F K 2011 Phys. Rev. D 84 083002 Google Scholar
[22]	Stephanov M A, Rajagopal K, Shuryak E V 1998 Phys. Rev. Lett. 81 4816 Google Scholar
[23]	Terazawa H 1979 INS-Report (Tokyo: Univ. of Tokyo) p336
[24]	Alford M, Reddy S 2003 Phys. Rev. D 67 074024 Google Scholar
[25]	Alford M, Jotwani P, Kouvaris C, Kundu J, Rajagopal K 2005 Phys. Rev. D 71 114011 Google Scholar
[26]	Baldo M 2003 Phys. Lett. B 562 153 Google Scholar
[27]	Ippolito N D, Ruggieri M, Rischke D H, Sedrakian A, Weber F 2008 Phys. Rev. D 77 023004 Google Scholar
[28]	Lai X Y, Xu R X 2011 Res. Astron. Astrophys. 11 687 Google Scholar
[29]	Avellar M G B de, Horvath J E, Paulucci L 2011 Phys. Rev. D 84 043004 Google Scholar
[30]	Bonanno L, Sedrakian A 2012 A&A 539 A16 Google Scholar
[31]	Chu P C, Wang B, Jia Y Y, Dong Y M, Wang S M, Li X H, Zhang L, Zhang X M, Ma H Y 2016 Phys. Rev. D 94 123014 Google Scholar
[32]	Chu P C, Li X H, Wang B, Dong Y M, Jia Y Y, Wang S M, Ma H Y 2017 Eur. Phys. J. C 77 512 Google Scholar
[33]	Chu P C, Zhou Y, Chen C, Li X H, Ma H Y 2020 J. Phys. G: Nucl. Part. Phys. 47 085201 Google Scholar
[34]	Bailin D and Love A 1984 Phys. Rep. 107 325 Google Scholar
[35]	Alford M G, Rajagopal K, Reddy S, Wilczek F 2001 Phys. Rev. D 64 074017 Google Scholar
[36]	Shovkovy I A 2005 Found. Phys. 35 1309 Google Scholar
[37]	Rajagopal K, Wilczek F 2001 Phys. Rev. L 86 3492 Google Scholar
[38]	Alford M G, Rajagopal K, Schaefer T, Schmitt A 2008 Rev. Mod. Phys. 80 1455 Google Scholar
[39]	Lugones G, Horvath J E 2003 Astron. Astrophys. 403 173 Google Scholar
[40]	Horvath J E, Lugones G 2004 Astron. Astrophys. 422 L1 Google Scholar
[41]	Woltjer L 1964 Astrophys. J. 140 1309 Google Scholar
[42]	Mihara T A 1990 Nature 346 250 Google Scholar
[43]	Chanmugam G 1992 Annu. Rev. Astron. Astrophys. 30 143 Google Scholar
[44]	Lai D, Shapiro S L 1991 Astrophys. J. 383 745 Google Scholar
[45]	Ferrer E J, Incera V, Keith J P, Portillo I, Springsteen P L 2010 Phys. Rev. C 82 065802 Google Scholar
[46]	Bandyopadhyay D, Chakrabarty S, Pal S 1997 Phys Rev. Lett. 79 2176 Google Scholar
[47]	Bandyopadhyay D, Pal S, Chakrabarty S 1998 J. Phys. G: Nucl. Part. Phys. 24 1647 Google Scholar
[48]	Menezes D P, Pinto M, Benghi, Avancini S, Providência C 2009 Phys. Rev. C 79 035807 Google Scholar
[49]	Menezes D P, Pinto M, Benghi, Avancini S, Providência C 2009 Phys. Rev. C 80 065805 Google Scholar
[50]	Ryu C Y, Kim K S, Cheoun Myung-Ki 2010 Phys. Rev. C 82 025804 Google Scholar
[51]	Ryu C Y, Cheoun Myung-Ki, Kajino T, Maruyama T, Mathews Grant J 2012 Astropart. Phys. 38 25 Google Scholar
[52]	Li X H, Gao Z F, Li X D, Xu Y, Wang P, WangN, Peng Q H 2016 Int. J. Mod. Phys. D 25(1) 1650002 Google Scholar
[53]	Gao Z F, Wang N, Shan H, L i, X D, Wang W 2017 Astrophys. J. 849 19 Google Scholar
[54]	Deng Z L, Gao Z F, Li X D, Shao Y 2020 Astrophys. J. 892 4 Google Scholar
[55]	Yan F Z, Gao Z F, Yang W S, Dong A J 2021 Astron. Nachr. 342 249 Google Scholar
[56]	Wang H, Gao Z F, Jia H Y, Wang N, Li X 2020 Universe 6 63 Google Scholar
[57]	Li B P, Gao Z F 2023 Astron. Nachr. 344 e20220111
[58]	Deng Z L, Li X D, Gao Z F, Shao Y 2021 Astrophys. J. 909 174 Google Scholar
[59]	G ao, Z F, Omar N, Shi X C, Wang N 2019 Astron. Nachr. 340 1030 Google Scholar
[60]	Lander, S K 2023 Astrophys.J. 947 L16 Google Scholar
[61]	Dong J M 2021 Mon. Not. R. Astron. Soc. 500 1505
[62]	Fu G Z, Xing C C, Wang N 2020 Eur. Phys. J. C 80 582 Google Scholar
[63]	Schertler K, Greiner C, Thoma M H, Schertler K, Greiner C, Thoma M H 1997 Nucl. Phys. A 616 659 Google Scholar
[64]	Pisarski R D 1989 Nucl. Phys. A 498 423
[65]	Wen X J 2009 J. Phys. G: Nucl. Part. Phys. 36 025011 Google Scholar
[66]	Zhang Z, Chu P C, Li X H, Liu H, Zhang X M 2021 Phys. Rev. D 103 103021 Google Scholar
[67]	Chu P C, Chen L W 2014 Astrophys. J. 780 135 Google Scholar
[68]	Chu P C 2018 Phys. Lett. B 778 447 Google Scholar
[69]	Chu P C, Chen L W 2017 Phys. Rev. D 96 103001 Google Scholar
[70]	Chodos A, Jaffe R L, Ohnson K, Thorn C B, Weisskopf V F 1974 Phys. Rev. D 9 3471 Google Scholar
[71]	Alford M, Braby M, Paris M, Reddy S 2005 Astrophys. J. 629 969 Google Scholar
[72]	Rehberg P, Klevansky S P, Hüfner J 1996 Phys. Rev. C 53 410 Google Scholar
[73]	Hanauske M, Satarov L M, Mishustin I N, Stocker H, Greiner W 2001 Phys. Rev. D 64 043005 Google Scholar
[74]	Rüster S B, Rischke D H 2004 Phys. Rev. D 69 045011 Google Scholar
[75]	Menezes D P, Providencia C, Melrose D B 2006 J. Phys. G 32 1081 Google Scholar
[76]	Chao J Y, Chu P C, Huang M 2013 Phys. Rev. D 88 054009 Google Scholar
[77]	Chu P C, Wang X, Chen L W, Huang M 2015 Phys. Rev. D 91 023003 Google Scholar
[78]	Chu P C, Wang B, Ma H Y, Dong Y M, Chang S L, Zheng C H, Liu J T, Zhang X M 2016 Phys. Rev. D 93 094032 Google Scholar
[79]	Chu P C, Chen L W 2017 Phys. Rev. D 96 083019 Google Scholar
[80]	Roberts C D, Williams A G 1994 Prog. Part. Nucl. Phys. 33 477 Google Scholar
[81]	Zong H S, Chang L, Hou F Y, Sun W M, Liu Y X 2005 Phys. Rev. C 71 015205 Google Scholar
[82]	Peng G X, Chiang H C, Yang J J, Li L, Liu B 1999 Phys. Rev. C 61 015201 Google Scholar
[83]	Peng G X, Chiang H C, Zou B S, Ning P Z, Luo S J 2000 Phys. Rev. C 62 025801 Google Scholar
[84]	Peng G X, Li A, Lombardo U 2008 Phys. Rev. C 77 065807 Google Scholar
[85]	Li A, Peng G X, Lu J F 2011 Res. Astron. Astrophys. 11 482 Google Scholar
[86]	Schertler K, Greiner C, Thoma M H 1997 Nucl. Phys. A 616 659
[87]	Schertler K, Greiner C, Sahu P K, Thoma M H 1998 Nucl. Phys. A 637 451
[88]	Alford M, Kouvaris C, Rajagopal K 2005 Phys. Rev. D 71 054009 Google Scholar
[89]	Giannakis I, Hou D, Ren H C, et al. 2004 Phys. Rev. Lett. 93 232301 Google Scholar
[90]	董爱军, 高志福, 杨晓峰等 2023 72 030502 Google Scholar Dong A J, Gao Z F, Yang X F, et al 2023 Acta Phys. Sin. 72 030502 Google Scholar
[91]	Ferrer E J, Vivian de la Incera 2005 Phys. Rev. Lett. 95 152002 Google Scholar
[92]	Ferrer E J, Vivian de la Incera, Cristina Manuel 2006 Nucl. Phys. B 747 88 Google Scholar
[93]	Feng B, Ferrer E J, Vivian de la Incera 2011 Nucl. Phys. B 853 213 Google Scholar
[94]	Paulucci L, Ferrer E J, Vivian de la Incera, Horvath J E 2011 Phys. Rev. D 83 043009 Google Scholar
[95]	Isayev A A, Yang J 2011 Phys. Rev. C 84 065802 Google Scholar
[96]	Isayev A A, Yang J 2012 Phys. Lett. B 707 163 Google Scholar
[97]	Isayev A A, Yang J 2013 J. Phys. G: Nucl. Part. Phys. 40 035105 Google Scholar
[98]	Feng B, Hou D F, Ren H C, Wu P P 2010 Phys. Rev. Lett. 105 042001 Google Scholar
[99]	Oppenheimer J R, Volkoff G M 1939 Phys. Rev. 33 374
[100]	Gao Z F, Li X D, Wang N, Yuan J P, Wang P, Peng Q H, Du Y J 2016 Mon. Not. R. Astron. Soc. 456 55 Google Scholar
[101]	Gao Z F, Wang N, Peng Q H, Li X D, Du Y J 2013 Mod. Phys. Lett. A 28 1350138 Google Scholar
[102]	Chu P C, Chen L W, Wang X 2014 Phys. Rev. D 90 063013 Google Scholar
[103]	Miller M C, Lamb F K, Dittmannet A J, et al. 2019 Astrophys. J. Lett. 887 L24 Google Scholar
[104]	Doroshenko V, Suleimanov V, Pühlhofer G, Santangelo A 2022 Nat. Astron. 6 1444 Google Scholar

[1]	阮丽娟, 许长补, 杨驰. 夸克物质中的超子整体极化与矢量介子自旋排列. , 2023, 72(11): 112401. doi: 10.7498/aps.72.20230496
[2]	董爱军, 高志福, 杨晓峰, 王娜, 刘畅, 彭秋和. 在超强磁场中修正的相对论电子压强. , 2023, 72(3): 030502. doi: 10.7498/aps.72.20220092
[3]	周淑英, 沈婉萍, 毛鸿. 强子夸克相变表面张力解析求解. , 2022, 71(21): 211101. doi: 10.7498/aps.71.20220659
[4]	王谊农, 初鹏程, 姜瑶瑶, 庞晓迪, 王圣博, 李培新. 基于准粒子模型的原生磁星研究. , 2022, 71(22): 222101. doi: 10.7498/aps.71.20220795
[5]	龚武坤, 郭文军. 混合中子星内强子-夸克退禁闭相变. , 2020, 69(24): 242101. doi: 10.7498/aps.69.20200925
[6]	沈婉萍, 尤仕佳, 毛鸿. 夸克介子模型的相图和表面张力. , 2019, 68(18): 181101. doi: 10.7498/aps.68.20190798
[7]	陈建玲, 王辉, 贾焕玉, 马紫微, 李永宏, 谭俊. 超强磁场下中子星壳层的电导率和磁星环向磁场欧姆衰变. , 2019, 68(18): 180401. doi: 10.7498/aps.68.20190760
[8]	吉日木图, 敖登, 薛康. 坐标空间中构造的Breit夸克势与介子和夸克偶素的质量劈裂. , 2018, 67(9): 091201. doi: 10.7498/aps.67.20172155
[9]	赵云辉, 海文华, 朱钱泉. 重夸克偶素的高阶变分-积分微扰修正. , 2009, 58(2): 734-739. doi: 10.7498/aps.58.734
[10]	黄金书, 罗鹏晖, 鲁公儒. 关于光子对撞机上底夸克对产生的研究. , 2009, 58(12): 8166-8173. doi: 10.7498/aps.58.8166
[11]	赖祥军, 罗志全, 刘晶晶, 刘宏林. 超新星核中的夸克相变及夸克质量效应. , 2008, 57(3): 1535-1541. doi: 10.7498/aps.57.1535
[12]	陈　洪, 梅　花, 沈彭年, 姜焕清. 重夸克偶素质量谱的相对论夸克模型研究（已撤稿）. , 2005, 54(3): 1136-1141. doi: 10.7498/aps.54.1136
[13]	贺泽君, 龙家丽, 马国亮, 马余刚, 张家驹, 刘波. 化学非平衡夸克-胶子物质中等质量双轻子的产生. , 2003, 52(11): 2831-2835. doi: 10.7498/aps.52.2831
[14]	贺泽君, 周文杰, 蒋维洲, 张家驹, 刘波. 膨胀的热夸克胶子物质的中等质量双轻子的增强. , 2002, 51(6): 1312-1316. doi: 10.7498/aps.51.1312
[15]	戴子高, 陆埮, 彭秋和. 中子星内部非奇异-奇异夸克物质的相变. , 1993, 42(8): 1210-1215. doi: 10.7498/aps.42.1210
[16]	鲍淑清, 薛晓舟. SU(10)手征亚夸克大统一模型. , 1988, 37(2): 347-352. doi: 10.7498/aps.37.347
[17]	谢凤仙. t夸克偶素能谱的计算. , 1987, 36(6): 778-784. doi: 10.7498/aps.36.778
[18]	何启智, 杨建华, 程国均, 杨荣辅. 用夸克模型探索π-N相互作用. , 1985, 34(1): 1-9. doi: 10.7498/aps.34.1
[19]	何祚庥, 林大航, 赵培贞. 考虑胶子质量的重夸克偶素位模型. , 1982, 31(4): 525-531. doi: 10.7498/aps.31.525
[20]	王家珠, 毕品镇, 殷鹏程. 重夸克对强子的椭球袋模型. , 1981, 30(12): 1707-1712. doi: 10.7498/aps.30.1707

计量

文章访问数: 1352
PDF下载量: 49
被引次数: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

搜索

留言板

色味锁夸克物质与夸克星