基于四态协议的半量子密钥分发诱骗态模型的有限码长分析

詹绍康; 王金东; 董双; 黄偲颖; 侯倾城; 莫乃达; 弥赏; 向黎冰; 赵天明; 於亚飞; 魏正军; 张智明

doi:10.7498/aps.72.20230849

摘要

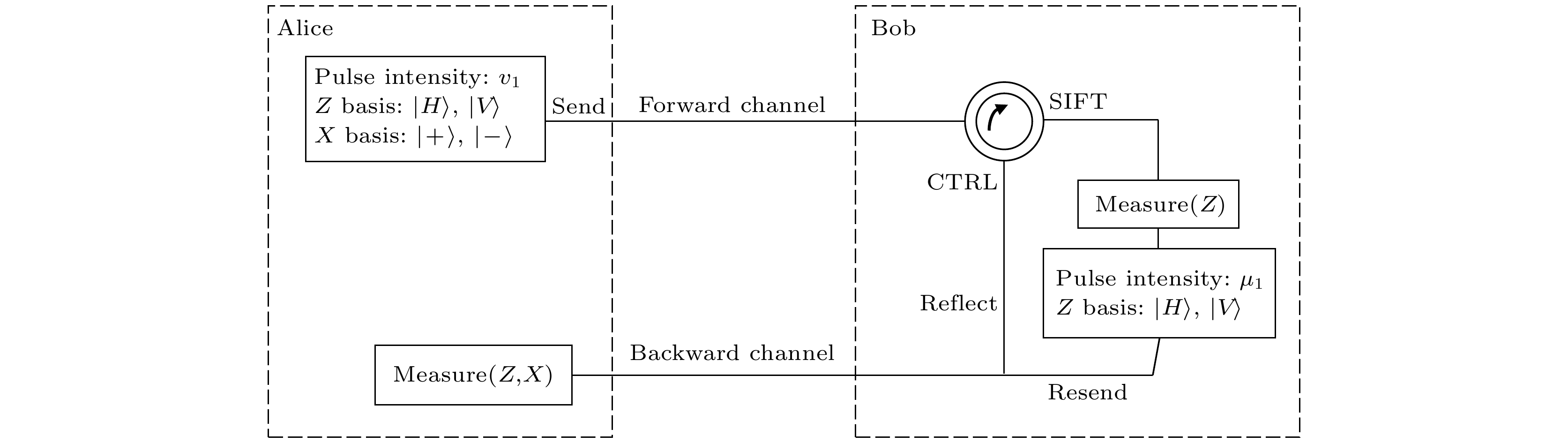
半量子密钥分发允许一个全量子用户Alice和一个经典用户Bob共享一对由物理原理保障的安全密钥. 在半量子密钥分发被提出的同时其鲁棒性获得了证明, 随后半量子密钥分发系统的无条件安全性被理论验证. 2021年基于镜像协议的半量子密钥分发系统的可行性被实验验证. 然而, 可行性实验系统仍旧采用强衰减的激光脉冲, 已有文献证明, 半量子密钥分发系统在受到光子数分裂攻击时仍旧面临密钥比特泄露的风险, 因此, 在密钥分发过程中引入诱骗态并且进行有限码长分析, 可以进一步合理评估密钥分发的实际安全性. 本文基于四态协议的半量子密钥分发系统, 针对仅在发送端Alice处加入单诱骗态的模型, 利用Hoeffding不等式进行了有限码长情况的安全密钥长度分析, 进而求得安全密钥率公式, 其数值模拟结果表明, 当选择样本量大小为$ {10}^{5} $时, 能够在近距离情况下获得$ {10}^{-4} $bit/s安全密钥速率, 与渐近情况下的安全密钥率相近, 这对半量子密钥分发系统的实际应用具有非常重要的意义.

关键词:

Abstract

Semi-quantum key distribution allows a full quantum user Alice and a classical user Bob to share a pair of security keys guaranteed by physical principles. Semi-quantum key distribution is proposed while verifying its robustness. Subsequently, its unconditional security of semi-quantum key distribution system is verified theoretically. In 2021, the feasibility of semi-quantum key distribution system based on mirror protocol was verified experimentally. However, the feasibility experimental system still uses the laser pulse with strong attenuation. It has been proved in the literature that the semi-quantum key distribution system still encounters the risk of secret key leakage under photon number splitting attack. Therefore, the actual security of key distribution can be further reasonably evaluated by introducing the temptation state and conducting the finite-key analysis in the key distribution process. In this work, for the model of adding one-decoy state only to Alice at the sending based on a four state semi-quantum key distribution system, the length of the security key in the case of finite-key is analyzed by using Hoeffding inequality, and then the formula of the security key rate is obtained. It is found in the numerical simulation that when the sample size is $ {10}^{5} $, the security key rate of $ {10}^{-4} $, which is close to the security key rate of the asymptotic limits, can be obtained in the case of close range. It is very important for the practical application of semi-quantum key distribution system.

Keywords:

作者及机构信息

1.
华南师范大学信息光电子科技学院, 广东省量子调控工程与材料重点实验室, 广州　510006

2.
华南师范大学信息光电子科技学院, 广东省微纳光子功能材料与器件重点实验室, 广州　510006

通信作者: 王金东, wangjindong@m.scnu

基金项目: 国家自然科学基金(批准号: 62071186, 61771205)和广东省重点实验室基金(批准号: 2020B1212060066)资助的课题.

Authors and contacts

1.
Guangdong Provincial Key Laboratory of Quantum Control Engineering and Materials, School of Information and Optoelectronic Science and Engineering, South China Normal University, Guangzhou 510006, China

2.
Guangdong Provincial Key Laboratory of Micro-nanophotonic Functional Materials and Devices, School of Information and Optoelectronic Science and Engineering, South China Normal University, Guangzhou 510006, China

Corresponding author: Wang Jin-Dong, wangjindong@m.scnu

Funds: Project supported by the National Natural Science Foundation of China (Grant Nos. 62071186, 61771205) and the Key Laboratory Foundation of Guangdong Province, China (Grant No. 2020B1212060066).

文章全文 : translate this paragraph

参考文献

[1]	Bennett C H, Brassard G 2014 Theor. Comput. Sci. 560 7 Google Scholar
[2]	Muller A, Herzog T, Huttner B, Tittel W, Zbinden H, Gisin N 1997 Appl. Phys. Lett. 70 793 Google Scholar
[3]	Wang J, Qin X, Jiang Y, Wang X, Chen L, Zhao F, Wei Z, Zhang Z 2016 Opt. Express 24 8302 Google Scholar
[4]	Mo X F, Zhu B, Han Z F, Gui Y Z, Guo G C 2005 Opt. Lett. 30 2632 Google Scholar
[5]	Kraus B, Gisin N, Renner R 2005 Phys. Rev. Lett. 95 080501 Google Scholar
[6]	Hwang W Y, Ahn D, Hwang S W 2001 Phys. Lett. A 279 133 Google Scholar
[7]	Duˇsek M, Haderka O, Hendrych M 1999 Opt. Commun. 169 103 Google Scholar
[8]	Lutkenhaus N, Jahma M 2002 New J. Phys. 4 44.1 Google Scholar
[9]	Bennett C H 1992 Phys. Rev. Lett. 68 3121 Google Scholar
[10]	Huttner B, Imoto N, Gisin N, Mor T 1995 Phys. Rev. A 51 1863 Google Scholar
[11]	Chaiwongkhot P, Zhong J Q, Huang A, Qin H, Shi S C, Makarov V 2022 EPJ Quantum Technol. 9 23 Google Scholar
[12]	Lydersen L, Wiechers C, Wittmann C, Elser D, Skaar J, Makarov A 2010 Nat. Photonics 4 686 Google Scholar
[13]	Lim C C W, Walenta N, Legré N, Gisin N, Zbinden H 2015 IEEE J. Sel. Top. Quantum Electron. 21 6601305 Google Scholar
[14]	Carlos N M, Juan Carlos G E 2021 Quantum Inf. Process. 20 196 Google Scholar
[15]	Kim C M, Kim Y W, Park Y J 2011 Curr. Appl. Phys. 11 1006 Google Scholar
[16]	Lu H, Fung C H F, Cai Q Y 2013 Phys. Rev. A 88 044302 Google Scholar
[17]	Chen Y P, Liu J Y, Sun M S, Zhou X X, Zhang C H, Li J, Wang Q 2021 Opt. Lett. 46 3729 Google Scholar
[18]	Zhou X Y, Zhang CH, Zhang C M, Wang Q 2019 Phys. Rev. A 99 062316 Google Scholar
[19]	Zeng P, Zhou H Y, Wu W J, Ma X F 2022 Nat. Commun. 13 3903 Google Scholar
[20]	Gu J, Cao X Y, Fu Y, He Z W, Yin Z J, Yin H L, Chen Z B 2022 Sci. Bull. 67 2167 Google Scholar
[21]	Cui C H, Yin Z Q, Wang R, Chen W, Wang S, Guo G C, Han Z F 2019 Phys. Rev. A 11 034053 Google Scholar
[22]	Xie Y M, Weng C X, Lu Y S, Fu Y, Wang Y, Yin H L, Chen Z B 2023 Phys. Rev. A 107 042603 Google Scholar
[23]	Curty M, Azuma K, Lo H K 2019 NPJ Quantum Inf. 5 64 Google Scholar
[24]	Xie Y M, Lu Y S, Weng C X, Cao X Y, Jia Z Y, Bao Y, Wang Y, Fu Y, Yin H L, Chen Z B 2022 PRX Quantum 3 020315 Google Scholar
[25]	Hwang W Y 2003 Phys. Rev. Lett. 91 057901 Google Scholar
[26]	Lo H K, Ma X, Chen K 2005 Phys. Rev. Lett. 94 230504 Google Scholar
[27]	Wang X B 2005 Phys. Rev. Lett. 94 230503 Google Scholar
[28]	Ma X, Qi B, Zhao Y, Lo H K 2005 Phys. Rev. A 72 012326 Google Scholar
[29]	Wang Q, Wang X B, Guo G C 2007 Phys. Rev. A 75 012312 Google Scholar
[30]	Ma X, Fung C H F, Dupuis F, Chen K, Tamaki K, Lo H K 2006 Phys. Rev. A 74 032330 Google Scholar
[31]	Scarani V, Ac´ın A, Ribordy G, Gisin N 2004 Phys. Rev. Lett. 92 057901 Google Scholar
[32]	Curty M, Xu F, Cui W, Lim C C W, Tamaki K, Lo H K 2014 Nat. Commun. 5 3732 Google Scholar
[33]	Mafu M, Garapo K, Petruccione F 2013 Phys. Rev. A 88 1 Google Scholar
[34]	Zhao L Y, Li H W, Yin Z Q, Chen W, You J, Han Z F 2014 Chin. Phys. B 23 100304 Google Scholar
[35]	Lim C C W, Curty M, Walenta N, Xu F H, Zbinden H 2014 Phys. Rev. A 89 022307 Google Scholar
[36]	Rusca D, Boaron A, Grünenfelder F, Martin A, Zbinden H 2018 Appl. Phys. Lett. 112 171104 Google Scholar
[37]	Boyer M, Kenigsberg D, Mor T 2007 Phys. Rev. Lett. 99 140501 Google Scholar
[38]	Zou X, Qiu D, Li L, Wu L, Li L 2009 Phys. Rev. A 79 052312 Google Scholar
[39]	Boyer M, Katz M, Liss R, Mor T 2017 Phys. Rev. A 96 062335 Google Scholar
[40]	Amer O, Krawec W O 2019 Phys. Rev. A 100 022319 Google Scholar
[41]	Krawec W O 2015 IEEE International Symposium Information Theory Hong Kong, China, June 14–19, 2015 p686
[42]	Boyer M, Liss R, Mor T 2018 Entropy 20 536 Google Scholar
[43]	Krawec W O, Liss R, Mor T 2023 IEEE Trans. Quantum Eng. 4 2100316 Google Scholar
[44]	Zhang W, Qiu D, Mateus P 2020 Int. J. Quantum Inf. 18 2050013 Google Scholar
[45]	Han S Y, Huang Y F, Mi S, Qin X, Wang J D, Yu Y F, Wei Z J, Zhang Z M 2021 EPJ Quantum Technol. 8 28 Google Scholar
[46]	Mi S, Dong S, Hou Q C, Wang J D, Yu Y F, Wei Z J, Zhang Z M 2022 Front. Phys. 10 1029552 Google Scholar
[47]	Hoeffding W 1963 J. Amer. Stat. Assoc. 58 13 Google Scholar
[48]	Renner R 2008 Int. J. Quantum Inf. 6 1 Google Scholar
[49]	Vitanov A, Dupuis F, Tomamichel M, Renner R 2013 IEEE Trans. Inf. Theory 59 2603 Google Scholar
[50]	Tomamichel M, Renner R 2011 Phys. Rev. Lett. 106 110506 Google Scholar
[51]	Fung C H F, Ma X F, Chau H F 2010 Phys. Rev. A 81 012318 Google Scholar
[52]	Dong S, Mi S, Hou Q C, Huang Y T, Wang J D, Yu Y F, Wei Z J, Zhang Z M, Fang J B 2023 EPJ Quantum Technol. 10 18 Google Scholar
[53]	Yin H L, Fu Y, Li C L, Weng C X, Li B H, Gu J, Lu Y S, Huang S, Chen Z B 2023 Nati. Sci. Rev. 10 nwac228 Google Scholar
[54]	Zhang X Z, Gong W G, Tan Y G, Ren Z Z, Guo X T 2009 Chin. Phys. B 18 2143 Google Scholar

施引文献

图 1 BKM-07协议模型

Fig. 1. BKM-07 protocol model.

下载: 全尺寸图片幻灯片

图 2 基于BKM-07协议的诱骗态SQKD模型

Fig. 2. Decoy SQKD model based on BKM-07 protocol.

下载: 全尺寸图片幻灯片

图 3 (a) 使用1 GHz的脉冲频率时不同码长$ {n}_{{\mathrm{Z}}} $之间的安全密钥率的比较, $ {n}_{{\mathrm{Z}}} $的取值为$ {10}^{s} $(s = [4, 5, 6, 7]), 当$ {n}_{{\mathrm{Z}}}={10}^{5} $时安全密钥速率与渐近情况的安全密钥率相近, 安全密钥率随光纤长度的增长而急剧衰减, 但在约30 km内能够保持10^–5的安全密钥率; (b) 考虑1 GHz的脉冲频率时三种不同$ {n}_{{\mathrm{Z}}} $之间近距离安全密钥率的比较, $ {n}_{{\mathrm{Z}}} $的取值为$ {10}^{s} $(s = [5, 6, 7])

Fig. 3. (a) The comparison of secret key rate of different key sizes $ {n}_{{\mathrm{Z}}} $, when using the pulse frequency of 1 GHz. The value of $ {n}_{{\mathrm{Z}}} $ are $ {10}^{s} $(where s = [4, 5, 6, 7]). When $ {10}^{5} $ is chosen to be $ {n}_{{\mathrm{Z}}} $, the secret key rate is close to the asymptotic limit’s. The secret key rate decreases sharply with the increase of fiber length, but it can maintain a secret key rate of 10^–5 for about 30 km; (b) the comparison of the proximity security key rates between six different $ {n}_{{\mathrm{Z}}} $ when considering a pulse frequency of 1 GHz. The value of $ {n}_{{\mathrm{Z}}} $ are $ {10}^{s} $(where s = [5, 6, 7]).

下载: 全尺寸图片幻灯片

表 1 50 km传播距离中每5 km处取得最大安全密钥率时脉冲强度$ {\mu }_{1} $, $ {v}_{1} $, $ {v}_{2} $的取值和得到的安全密钥率

Table 1. Pulse strength $ {\mu }_{1} $, $ {v}_{1} $, $ {v}_{2} $ and the number of Secret key ratio every 5 km in a 50 km transmission distance.

传输距离/km	$ {\mu }_{1} $	$ {v}_{1} $	$ {v}_{2} $	密钥率
0	0.68	0.48	0.07	0.001745822
5	0.68	0.48	0.07	0.000785235
10	0.68	0.48	0.07	0.000483058
15	0.68	0.48	0.07	0.000331894
20	0.68	0.48	0.07	0.000240990
25	0.68	0.48	0.07	0.000180301
30	0.68	0.48	0.08	0.000137404
35	0.68	0.48	0.08	0.000105438
40	0.68	0.49	0.09	0.000081846
45	0.68	0.49	0.10	0.000063533
50	0.68	0.49	0.10	0.000049545

下载: 导出CSV

map

[1]	Bennett C H, Brassard G 2014 Theor. Comput. Sci. 560 7 Google Scholar
[2]	Muller A, Herzog T, Huttner B, Tittel W, Zbinden H, Gisin N 1997 Appl. Phys. Lett. 70 793 Google Scholar
[3]	Wang J, Qin X, Jiang Y, Wang X, Chen L, Zhao F, Wei Z, Zhang Z 2016 Opt. Express 24 8302 Google Scholar
[4]	Mo X F, Zhu B, Han Z F, Gui Y Z, Guo G C 2005 Opt. Lett. 30 2632 Google Scholar
[5]	Kraus B, Gisin N, Renner R 2005 Phys. Rev. Lett. 95 080501 Google Scholar
[6]	Hwang W Y, Ahn D, Hwang S W 2001 Phys. Lett. A 279 133 Google Scholar
[7]	Duˇsek M, Haderka O, Hendrych M 1999 Opt. Commun. 169 103 Google Scholar
[8]	Lutkenhaus N, Jahma M 2002 New J. Phys. 4 44.1 Google Scholar
[9]	Bennett C H 1992 Phys. Rev. Lett. 68 3121 Google Scholar
[10]	Huttner B, Imoto N, Gisin N, Mor T 1995 Phys. Rev. A 51 1863 Google Scholar
[11]	Chaiwongkhot P, Zhong J Q, Huang A, Qin H, Shi S C, Makarov V 2022 EPJ Quantum Technol. 9 23 Google Scholar
[12]	Lydersen L, Wiechers C, Wittmann C, Elser D, Skaar J, Makarov A 2010 Nat. Photonics 4 686 Google Scholar
[13]	Lim C C W, Walenta N, Legré N, Gisin N, Zbinden H 2015 IEEE J. Sel. Top. Quantum Electron. 21 6601305 Google Scholar
[14]	Carlos N M, Juan Carlos G E 2021 Quantum Inf. Process. 20 196 Google Scholar
[15]	Kim C M, Kim Y W, Park Y J 2011 Curr. Appl. Phys. 11 1006 Google Scholar
[16]	Lu H, Fung C H F, Cai Q Y 2013 Phys. Rev. A 88 044302 Google Scholar
[17]	Chen Y P, Liu J Y, Sun M S, Zhou X X, Zhang C H, Li J, Wang Q 2021 Opt. Lett. 46 3729 Google Scholar
[18]	Zhou X Y, Zhang CH, Zhang C M, Wang Q 2019 Phys. Rev. A 99 062316 Google Scholar
[19]	Zeng P, Zhou H Y, Wu W J, Ma X F 2022 Nat. Commun. 13 3903 Google Scholar
[20]	Gu J, Cao X Y, Fu Y, He Z W, Yin Z J, Yin H L, Chen Z B 2022 Sci. Bull. 67 2167 Google Scholar
[21]	Cui C H, Yin Z Q, Wang R, Chen W, Wang S, Guo G C, Han Z F 2019 Phys. Rev. A 11 034053 Google Scholar
[22]	Xie Y M, Weng C X, Lu Y S, Fu Y, Wang Y, Yin H L, Chen Z B 2023 Phys. Rev. A 107 042603 Google Scholar
[23]	Curty M, Azuma K, Lo H K 2019 NPJ Quantum Inf. 5 64 Google Scholar
[24]	Xie Y M, Lu Y S, Weng C X, Cao X Y, Jia Z Y, Bao Y, Wang Y, Fu Y, Yin H L, Chen Z B 2022 PRX Quantum 3 020315 Google Scholar
[25]	Hwang W Y 2003 Phys. Rev. Lett. 91 057901 Google Scholar
[26]	Lo H K, Ma X, Chen K 2005 Phys. Rev. Lett. 94 230504 Google Scholar
[27]	Wang X B 2005 Phys. Rev. Lett. 94 230503 Google Scholar
[28]	Ma X, Qi B, Zhao Y, Lo H K 2005 Phys. Rev. A 72 012326 Google Scholar
[29]	Wang Q, Wang X B, Guo G C 2007 Phys. Rev. A 75 012312 Google Scholar
[30]	Ma X, Fung C H F, Dupuis F, Chen K, Tamaki K, Lo H K 2006 Phys. Rev. A 74 032330 Google Scholar
[31]	Scarani V, Ac´ın A, Ribordy G, Gisin N 2004 Phys. Rev. Lett. 92 057901 Google Scholar
[32]	Curty M, Xu F, Cui W, Lim C C W, Tamaki K, Lo H K 2014 Nat. Commun. 5 3732 Google Scholar
[33]	Mafu M, Garapo K, Petruccione F 2013 Phys. Rev. A 88 1 Google Scholar
[34]	Zhao L Y, Li H W, Yin Z Q, Chen W, You J, Han Z F 2014 Chin. Phys. B 23 100304 Google Scholar
[35]	Lim C C W, Curty M, Walenta N, Xu F H, Zbinden H 2014 Phys. Rev. A 89 022307 Google Scholar
[36]	Rusca D, Boaron A, Grünenfelder F, Martin A, Zbinden H 2018 Appl. Phys. Lett. 112 171104 Google Scholar
[37]	Boyer M, Kenigsberg D, Mor T 2007 Phys. Rev. Lett. 99 140501 Google Scholar
[38]	Zou X, Qiu D, Li L, Wu L, Li L 2009 Phys. Rev. A 79 052312 Google Scholar
[39]	Boyer M, Katz M, Liss R, Mor T 2017 Phys. Rev. A 96 062335 Google Scholar
[40]	Amer O, Krawec W O 2019 Phys. Rev. A 100 022319 Google Scholar
[41]	Krawec W O 2015 IEEE International Symposium Information Theory Hong Kong, China, June 14–19, 2015 p686
[42]	Boyer M, Liss R, Mor T 2018 Entropy 20 536 Google Scholar
[43]	Krawec W O, Liss R, Mor T 2023 IEEE Trans. Quantum Eng. 4 2100316 Google Scholar
[44]	Zhang W, Qiu D, Mateus P 2020 Int. J. Quantum Inf. 18 2050013 Google Scholar
[45]	Han S Y, Huang Y F, Mi S, Qin X, Wang J D, Yu Y F, Wei Z J, Zhang Z M 2021 EPJ Quantum Technol. 8 28 Google Scholar
[46]	Mi S, Dong S, Hou Q C, Wang J D, Yu Y F, Wei Z J, Zhang Z M 2022 Front. Phys. 10 1029552 Google Scholar
[47]	Hoeffding W 1963 J. Amer. Stat. Assoc. 58 13 Google Scholar
[48]	Renner R 2008 Int. J. Quantum Inf. 6 1 Google Scholar
[49]	Vitanov A, Dupuis F, Tomamichel M, Renner R 2013 IEEE Trans. Inf. Theory 59 2603 Google Scholar
[50]	Tomamichel M, Renner R 2011 Phys. Rev. Lett. 106 110506 Google Scholar
[51]	Fung C H F, Ma X F, Chau H F 2010 Phys. Rev. A 81 012318 Google Scholar
[52]	Dong S, Mi S, Hou Q C, Huang Y T, Wang J D, Yu Y F, Wei Z J, Zhang Z M, Fang J B 2023 EPJ Quantum Technol. 10 18 Google Scholar
[53]	Yin H L, Fu Y, Li C L, Weng C X, Li B H, Gu J, Lu Y S, Huang S, Chen Z B 2023 Nati. Sci. Rev. 10 nwac228 Google Scholar
[54]	Zhang X Z, Gong W G, Tan Y G, Ren Z Z, Guo X T 2009 Chin. Phys. B 18 2143 Google Scholar

[1]	张云杰, 王旭阳, 张瑜, 王宁, 贾雁翔, 史玉琪, 卢振国, 邹俊, 李永民. 基于硬件同步的四态离散调制连续变量量子密钥分发. , 2024, 73(6): 060302. doi: 10.7498/aps.73.20231769
[2]	马洛嘉, 丁华建, 陈子骐, 张春辉, 王琴. 一种态制备误差容忍的量子数字签名协议. , 2024, 73(2): 020301. doi: 10.7498/aps.73.20231190
[3]	赖红. 基于广义等距张量的压缩多光子纠缠态量子密钥分发. , 2023, 72(17): 170301. doi: 10.7498/aps.72.20230589
[4]	曾柏云, 辜鹏宇, 蒋世民, 贾欣燕, 樊代和. Markov环境下“X”态基于CHSH不等式的量子非局域关联检验. , 2023, 72(5): 050301. doi: 10.7498/aps.72.20222218
[5]	曾柏云, 辜鹏宇, 胡强, 贾欣燕, 樊代和. 基于CHSH不等式几何解释的“X”态量子非局域关联检验. , 2022, 71(17): 170302. doi: 10.7498/aps.71.20220445
[6]	叶世强, 陈小余. 基于量子相干性的四体贝尔不等式构建. , 2017, 66(20): 200301. doi: 10.7498/aps.66.200301
[7]	孙伟, 尹华磊, 孙祥祥, 陈腾云. 基于相干叠加态的非正交编码诱骗态量子密钥分发. , 2016, 65(8): 080301. doi: 10.7498/aps.65.080301
[8]	赵翠兰, 王丽丽, 赵丽丽. 有限深抛物势量子盘中极化子的激发态性质. , 2015, 64(18): 186301. doi: 10.7498/aps.64.186301
[9]	孙颖, 赵尚弘, 东晨. 基于量子存储的长距离测量设备无关量子密钥分配研究. , 2015, 64(14): 140304. doi: 10.7498/aps.64.140304
[10]	东晨, 赵尚弘, 赵卫虎, 石磊, 赵顾颢. 非对称信道传输效率的测量设备无关量子密钥分配研究. , 2014, 63(3): 030302. doi: 10.7498/aps.63.030302
[11]	邓伟胤, 朱瑞, 邓文基. 有限尺寸石墨烯的电子态. , 2013, 62(8): 087301. doi: 10.7498/aps.62.087301
[12]	周媛媛, 张合庆, 周学军, 田培根. 基于标记配对相干态光源的诱骗态量子密钥分配性能分析. , 2013, 62(20): 200302. doi: 10.7498/aps.62.200302
[13]	赵加强, 曹连振, 逯怀新, 王晓芹. 三比特类GHZ态的Bell型不等式和非定域性. , 2013, 62(12): 120301. doi: 10.7498/aps.62.120301
[14]	赵加强, 曹连振, 王晓芹, 逯怀新. 三光子GHZ态中不同Bell型不等式的实验研究. , 2012, 61(17): 170301. doi: 10.7498/aps.61.170301
[15]	周媛媛, 周学军. 基于弱相干态光源的非正交编码被动诱骗态量子密钥分配. , 2011, 60(10): 100301. doi: 10.7498/aps.60.100301
[16]	胡华鹏, 王金东, 黄宇娴, 刘颂豪, 路巍. 基于条件参量下转换光子对的非正交编码诱惑态量子密钥分发. , 2010, 59(1): 287-292. doi: 10.7498/aps.59.287
[17]	何广强, 郭红斌, 李昱丹, 朱思维, 曾贵华. 基于二进制均匀调制相干态的量子密钥分发方案. , 2008, 57(4): 2212-2217. doi: 10.7498/aps.57.2212
[18]	权东晓, 裴昌幸, 朱畅华, 刘丹. 一种新的预报单光子源诱骗态量子密钥分发方案. , 2008, 57(9): 5600-5604. doi: 10.7498/aps.57.5600
[19]	何广强, 易智, 朱俊, 曾贵华. 基于双模压缩态的量子密钥分发方案. , 2007, 56(11): 6427-6433. doi: 10.7498/aps.56.6427
[20]	董传华. 具有有限温度的热克尔态. , 1997, 46(3): 467-473. doi: 10.7498/aps.46.467

计量

文章访问数: 2355
PDF下载量: 78
被引次数: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

搜索

留言板