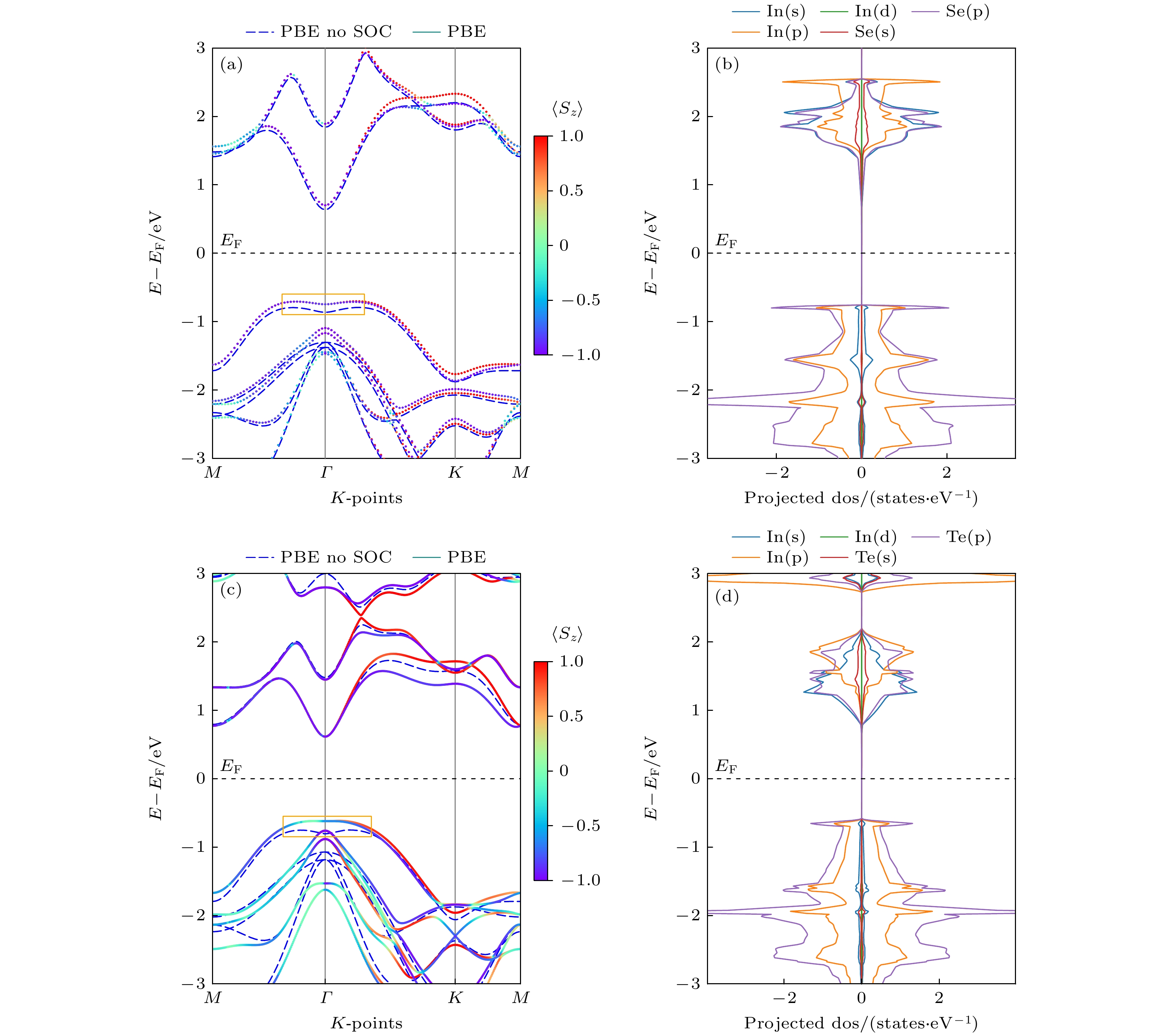
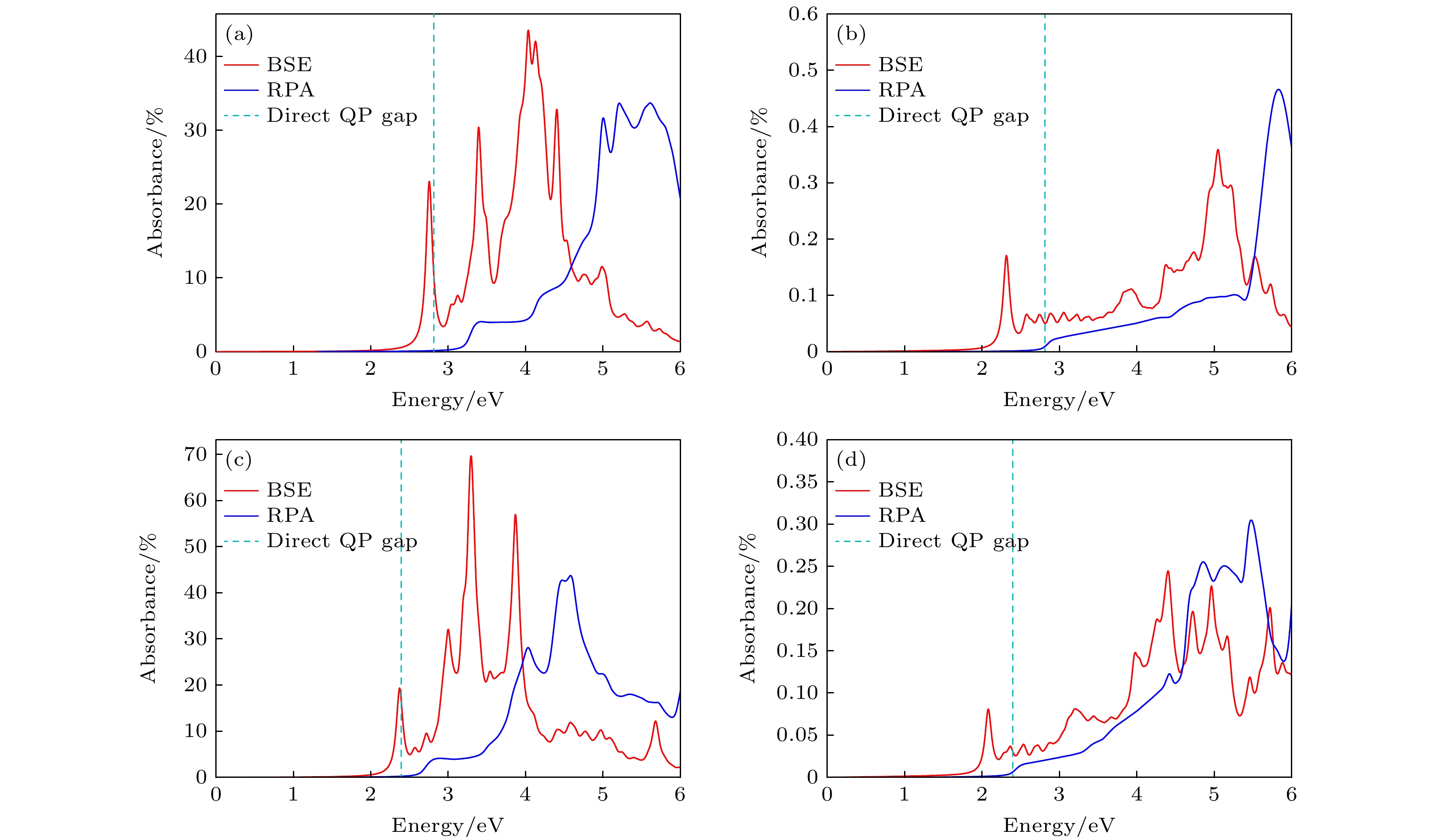
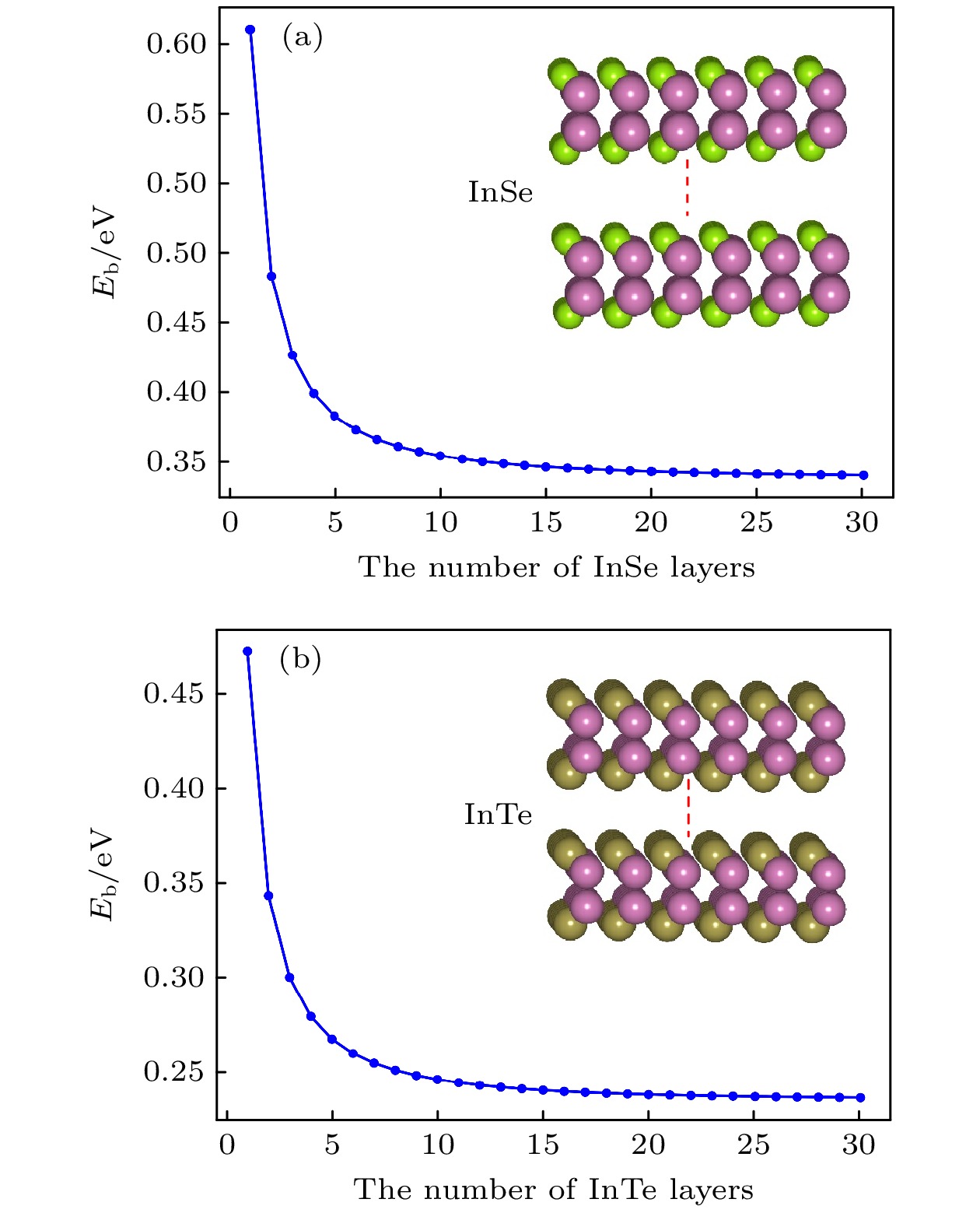
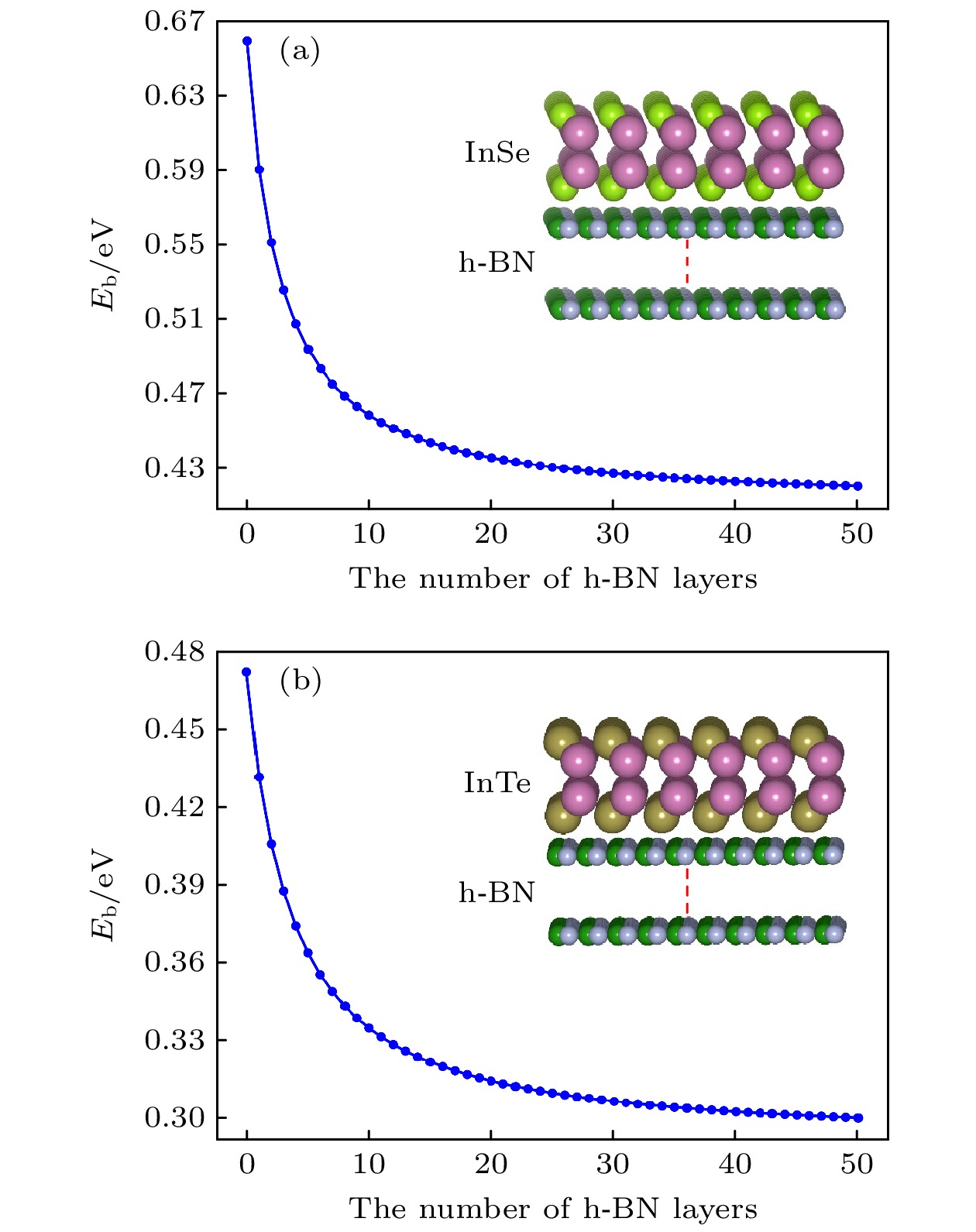
-
采用基于格林函数的GW方法计算发现: 孤立二维单层硒化铟(InSe)和碲化铟(InTe)具有吸收可见光的理想带隙, 高的电子迁移率以及适合光解水的电子能带结构, 电子自旋轨道耦合(SOC)效应使单层InTe从间接带隙半导体转变为直接带隙半导体. 在准粒子能级计算的基础之上, 通过求解Bethe-Salpeter方程 (BSE)发现, 孤立单层InSe和InTe的激子结合能远大于常温下激子的自发解离能. 另一方面, 实际应用的二维半导体为了维持其力学稳定性, 大都需要依附在衬底上, 另外, 不同实验室样品自身原子层厚度也各异, 这些因素必然改变二维半导体的介电环境. 进一步的计算发现, 二维InSe和InTe的激子结合能随自身原子层厚度以及衬底厚度的变大而减小, 这说明可以通过调控二维半导体自身原子层以及衬底厚度的方式实现对激子结合能的精确调控, 本文结果能够为将来精确调控二维InSe和InTe的激子结合能大小提供重要的理论依据.The calculations using GW method based on Green’s function show that two-dimensional monolayer InSe and InTe have desired electronic band gaps for absorbing visible light, high electron mobilities, and suitable electronic band structures for water splitting, and that the spin orbit coupling (SOC) leads to an indirect-to -direct band gap transition for monolayer InTe. On the basis of quasi-particle energy levels, the calculations via solving Bethe-Salpter equation (BSE) show that the exciton binding energy of isolated monolayer InSe and InTe are much higher than that of the dissociation energy of exciton at room temperature. On the other hand, two-dimensional semiconductors in laboratory are often supported by substrates for mechanical stability, and the atomic thickness values of two-dimensional semiconductors are also various in different experiments. These factors will change the dielectric environments of two-dimensional semiconductor, and the further calculations show that the exciton binding energy of InSe and InTe decrease with the increase of the thickness of InSe and InTe and also the thickness of their substrates, also revealing that the exciton binding energy can be accurately controlled by engineering the thickness of two-dimensional semiconductors and the substrates. Our results provide important theoretical basis for accurately controlling the binding energy of two-dimensional InSe and InTe.
-
Keywords:
- two-dimensional material /
- electronic structure /
- screening effects /
- exciton
[1] Dai M, Gao C, Nie Q, Wang Q J, Lin, Y, F, Chu J, Li W 2022 Adv. Mater. Technol. 7 2200321
Google Scholar
[2] Bandurin D A, Tyurnina A V, Yu G L, Mishchenko A, Zolyomi V, Morozov S V, Kumar R K, Gorbachev R V, Kudrynskyi Z R, Pezzini S, Kovalyuk Z D, Zeitler U, Novoselov K S, Patanè A, Eaves L, Grigorieva I V, Fal’ko V I, Geim A K, Cao Y 2017 Nat. Nanotechnol. 12 223
Google Scholar
[3] Li Z Y, Cheng H Y, Kung S H, Yao H C, Inbaraj C R P, Sankar R, Ou M N, Chen Y F, Lee C C, Lin K H 2023 Nanomaterials 13 750
Google Scholar
[4] Feng W, Zhou X, Tian W Q, Zheng W, Hu P 2015 Phys. Chem. Chem. Phys. 17 3653
Google Scholar
[5] Mudd G W, Svatek S A, Hague L, Makarovsky O, Kudrynskyi Z R, Mellor C J, Beton P H, Eaves L, Novoselov K S, Kovalyuk Z D, Vdovin E E, Marsden A J, Wilson N R, Patane A 2015 Adv. Mater. 27 3760
Google Scholar
[6] 张芳, 贾利群, 孙现亭, 戴宪起, 黄奇祥, 李伟 2020 69 157302
Google Scholar
Zhang F, Jia L Q, Sun X T, Dai X Q, Huang Q X, Li W 2020 Acta Phys. Sin. 69 157302
Google Scholar
[7] Mudd G W, Svatek S A, Ren T, Patanè A, Makarovsky O, Eaves L, Beton P H, Kovalyuk Z D, Lashkarev G V, Kudrynskyi Z R, Dmitriev A I 2013 Adv. Mater. 25 5714
Google Scholar
[8] Henck H, Pierucci D, Zribi J, Bisti F, Papalazarou E, Girard J C, Chaste J., Bertran F, Fèvre P L, Sirotti F, Perfetti L, Giorgetti C, Shukla A, Rault J E, Ouerghi A 2019 Phys. Rev. Mater. 3 034004
Google Scholar
[9] Lei S, Ge L, Najmaei S, George A, Kappera R, Lou J, Chhowalla M, Yamaguchi H, Gupta G, Vajtai R, Mohite A D, Ajayan P M 2014 ACS Nano 8 1263
Google Scholar
[10] Zhou J, Shi J, Zeng Q, Chen Y, Niu L, Liu F, Yu T, Suenaga Kazu, Liu X, Lin J, Liu Z 2018 2D Mater. 5 025019
Google Scholar
[11] Sucharitakul S, Goble N J, Kumar U R, Sankar R, Bogorad Z A, Chou F, Chen Y T, Gao Xuan P A 2015 Nano Lett. 156 3815
Google Scholar
[12] Lauth J, Gorris F E S, Khoshkhoo M S, Chassé T, Friedrich W, Lebedeva V, Meyer A, Klinke C, Kornowski A, Scheele M, Weller H 2016 Chem. Mater. 28 1728
Google Scholar
[13] 范人杰, 江先燕, 陶奇睿, 梅期才, 唐颖菲, 陈志权, 苏贤礼, 唐新峰 2021 70 137102
Google Scholar
Fan R J, Jiang X Y, Tao Q R, Mei Q C, Tang Y F, Chen Z Q, Su X L, Tang X F 2021 Acta Phys. Sin. 70 137102
Google Scholar
[14] Pal S, Bose D N 1996 Solid State Commun. 97 725
Google Scholar
[15] Zólyomi V, Drummond N D, Falko V I 2014 Phys. Rev. B 89 205416
Google Scholar
[16] Jain M, Chelikowsky J R, Louie S, G, 2011 Phys. Rev. Lett. 107 216806
Google Scholar
[17] Ma H, Feng J, Jin F, Wei M, Liu C, Ma Y 2018 Nanoscale 10 15624
Google Scholar
[18] Yi Z, Wu M, Pang Y, Jia R, Xu R R 2021 Appl. Surf. Sci. 567 150842
Google Scholar
[19] Klots A R, Newaz A K M, Wang B, Prasai D, Krzyzanowska H, Lin Junhao, Caudel D, Ghimire N J, Yan J, Ivanov B L, Velizhanin K A, Burger A, Mandrus D G, Tolk N H, Pantelides S T, Bolotin K I 2014 Sci. Rep. 4 6608
Google Scholar
[20] Thygesen K S 2017 2D Mater. 4 022004
Google Scholar
[21] Jiang Y, Chen S, Zheng W, Zheng B, Pan A 2021 Light-Sci. Appl. 10 72
Google Scholar
[22] Qiu, D. Y. Jornada F da H, Louie S G 2013 Phys. Rev. Lett. 111 216805
Google Scholar
[23] Komsa, H P, Krasheninnikov A V 2012 Phys. Rev. B 86 241201(R
Google Scholar
[24] Shi H L, Pan H, Zhang Y W, Yakobson B I 2013 Phys. Rev. B 87 155304
Google Scholar
[25] Qiu D Y, Felipe H da J, Louie, S G 2017 Nano Lett. 17 4706
Google Scholar
[26] Raja A, Chaves A, Yu J, Arefe G, Hill Heather M, Rigosi Albert F, Berkelbach Timothy C, Nagler P, Schüller C, Korn T, Nuckolls C, Hone J, Brus Louis E, Heinz Tony F, Reichman David R, Chernikov A 2017 Nat. Commun. 8 15251
Google Scholar
[27] Ugeda Miguel M, Bradley Aaron J, Shi S F, Jornada Felipe H da, Zhang Y, Qiu Diana Y, Ruan W, Mo S K, Hussain Z, Shen Z X, Wang F, Louie Steven G, Crommie Michael F 2014 Nat. Mater. 13 1091
Google Scholar
[28] Enkovaara J, Rostgaard C, Mortensen J J, Chen J, Dułak M, Ferrighi L, Gavnholt J, Glinsvad C, Haikola V, Hansen H A, Kristoffersen H H, Kuisma M, Larsen A H, Lehtovaara L, Ljungberg M, Lopez-Acevedo O, Moses P G, Ojanen J, Olsen T, Petzold V, Romero N A, Stausholm-Møller J, Strange M, Tritsaris G A, Vanin M, Walter M, Hammer B, Häkkinen H, Madsen G K H, Nieminen R M, Nørskov J K, Puska M, Rantala T T, Schiøtz J, Thygesen K S, Jacobsen K W 2010 J. Phys. Condens. Mater. 22 253202
Google Scholar
[29] Blöchl P E 1994 Phys. Rev. B 50 17953
Google Scholar
[30] Perdew J P, Burke K 1996 Phys. Rev. Lett. 77 3865
Google Scholar
[31] Babaie-Kafaki S, Aminifard Z 2019 Numer. Algorithms 82 1345
Google Scholar
[32] Hüser F, Olsen T, Thygesen K S 2013 Phys. Rev. B 87 235132
Google Scholar
[33] Yan J, Mortensen J J, Jacobsen K W, Thygesen K S 2011 Phys. Rev. B 83 245122
Google Scholar
[34] Yi Z, Ma Y, Zheng Y, Duan Y, Li H 2019 Adv. Mater. Interfaces 6 1801175
Google Scholar
[35] Rozzi C A, Varsano D, Marini A, Gross E K U, Rubio A 2006 Phys. Rev. B 73 205119
Google Scholar
[36] Smail-Beigi S 2006 Phys. Rev. B 73 233103
Google Scholar
[37] Rasmussen F A, Schmidt P S, Winther K T, Thygesen K S 2016 Phys. Rev. B 94 155406
Google Scholar
[38] Olsen T 2016 Phys. Rev. B 94 235106
Google Scholar
[39] Tiago M L, Ismail-Beigi S, Louie S G 2004 Phys. Rev. B 69 125212
Google Scholar
[40] Klimeš J, Kaltak M, Kresse G 2014 Phys. Rev. B 90 075125
Google Scholar
[41] Haastrup S, Strange Mikke, Pandey M, Deilmann T, Schmidt Per S, Hinsche Nicki F, Gjerding Morten N, Torelli D, Larsen Peter M, Riis-Jensen Anders C 2018 2D Mater. 5 042002
Google Scholar
[42] Onida G, Reining L and Rubio A 2002 Rev. Mod. Phys. 74 601
Google Scholar
[43] 隋国民, 严桂俊, 杨光, 张宝, 冯亚青 2022 71 208801
Google Scholar
Sui G M, Yan G J, Yang G, Zhang B, Feng Y Q 2022 Acta Phys. Sin. 71 208801
Google Scholar
[44] Andersen K, Latini S, Thygesen K S 2015 Nano Lett. 15 4616
Google Scholar
[45] Pandey T, Parker David S, Lindsay L 2017 Nanotechnology 28 455706
Google Scholar
[46] Pang Y, Wu M, Zhang J, Yi Z 2021 J. Phys. Chem. C 125 3027
Google Scholar
-
图 1 (a) 孤立单层InX (X = Se/Te) 几何结构; (b) InX原胞的第一布里渊区以及高对称位置K点分布
Fig. 1. (a) Geometric structure of isolated monolayer InX (X = Se/Te); (b) the first Brillouin zone and high-symmetry K point distributions of primitive unit cell for InX.
图 2 孤立单层InSe (a), (b) 和InTe (c), (d) 的能带结构(左)和原子投影分态密度(右)
Fig. 2. The band structures (left) and atom projected density of states (right) of isolated monolayer InSe (a), (b) and InTe (c), (d).
图 3 (a) 基于PBE和GW方法得到的孤立单层InSe和InTe带边能级排列示意图; (b) 准粒子能级, 光学带隙以及激子结合能示意图
Fig. 3. (a) The band edge alignments of isolated monolayer InSe and InTe based on PBE and GW method; (b) quasi-particle energy level, optical band gap and schematic diagram of exciton binding energy.
图 4 单层InSe (a), (b) 和 单层InTe (c), (d)分别沿x和z方向的光学吸收谱
Fig. 4. The absorption spectrum of monolayer InSe (a), (b) and monolayer InTe (c), (d) along x and z polarization directions.
图 5 InSe (a)和InTe (b)的激子结合能随自身原子层数量的变化关系
Fig. 5. The relationship diagrams of exciton binding energies of InSe and InTe with the variation of their self-atomic layer numbers.
图 6 单层InSe/InTe和环境之间的结构示意图
Fig. 6. The structural diagram between monolayer InSe/InTe and environment.
图 7 单层InSe (a) 和InTe (b) 的激子结合能随h-BN原子层数量的变化关系
Fig. 7. The relationship diagrams of exciton binding energies of monolayer InSe and InTe with the variation of number of h-BN atomic layers.
图 B1 InSe和InTe沿a (图中方向1)和b (图中方向2)两个方向导带边和价带边抛物线拟合
Fig. B1. Fitted results of conduction band edge and valence band edge of InSe and InTe along both a (direction 1 in the figure) and b (direction 2 in the figure).
表 1 InSe和InTe导带和价带带边电子和空穴的有效质量
Table 1. The effective masses of electrons and holes of conduction and valence band edges for InSe and InTe.
n (InSe/InTe) 1 5 10 20 30 Eb (InSe)/eV 0.419 0.350 0.342 0.338 0.337 Eb (InTe)/eV 0.294 0.244 0.237 0.235 0.234 
下载: 导出CSV
表 2 不同原子层厚度InSe以及InTe放置在50个原子层厚度h-BN衬底上时的激子结合能
Table 2. The exciton binding energies for different atomic thicknesses of InSe and InTe on h-BN substrate with 50 atomic layer thickness.
n (InSe/InTe) 1 5 10 20 30 Eb (InSe)/eV 0.419 0.350 0.342 0.338 0.337 Eb (InTe)/eV 0.294 0.244 0.237 0.235 0.234
下载: 导出CSV
-
[1] Dai M, Gao C, Nie Q, Wang Q J, Lin, Y, F, Chu J, Li W 2022 Adv. Mater. Technol. 7 2200321
Google Scholar
[2] Bandurin D A, Tyurnina A V, Yu G L, Mishchenko A, Zolyomi V, Morozov S V, Kumar R K, Gorbachev R V, Kudrynskyi Z R, Pezzini S, Kovalyuk Z D, Zeitler U, Novoselov K S, Patanè A, Eaves L, Grigorieva I V, Fal’ko V I, Geim A K, Cao Y 2017 Nat. Nanotechnol. 12 223
Google Scholar
[3] Li Z Y, Cheng H Y, Kung S H, Yao H C, Inbaraj C R P, Sankar R, Ou M N, Chen Y F, Lee C C, Lin K H 2023 Nanomaterials 13 750
Google Scholar
[4] Feng W, Zhou X, Tian W Q, Zheng W, Hu P 2015 Phys. Chem. Chem. Phys. 17 3653
Google Scholar
[5] Mudd G W, Svatek S A, Hague L, Makarovsky O, Kudrynskyi Z R, Mellor C J, Beton P H, Eaves L, Novoselov K S, Kovalyuk Z D, Vdovin E E, Marsden A J, Wilson N R, Patane A 2015 Adv. Mater. 27 3760
Google Scholar
[6] 张芳, 贾利群, 孙现亭, 戴宪起, 黄奇祥, 李伟 2020 69 157302
Google Scholar
Zhang F, Jia L Q, Sun X T, Dai X Q, Huang Q X, Li W 2020 Acta Phys. Sin. 69 157302
Google Scholar
[7] Mudd G W, Svatek S A, Ren T, Patanè A, Makarovsky O, Eaves L, Beton P H, Kovalyuk Z D, Lashkarev G V, Kudrynskyi Z R, Dmitriev A I 2013 Adv. Mater. 25 5714
Google Scholar
[8] Henck H, Pierucci D, Zribi J, Bisti F, Papalazarou E, Girard J C, Chaste J., Bertran F, Fèvre P L, Sirotti F, Perfetti L, Giorgetti C, Shukla A, Rault J E, Ouerghi A 2019 Phys. Rev. Mater. 3 034004
Google Scholar
[9] Lei S, Ge L, Najmaei S, George A, Kappera R, Lou J, Chhowalla M, Yamaguchi H, Gupta G, Vajtai R, Mohite A D, Ajayan P M 2014 ACS Nano 8 1263
Google Scholar
[10] Zhou J, Shi J, Zeng Q, Chen Y, Niu L, Liu F, Yu T, Suenaga Kazu, Liu X, Lin J, Liu Z 2018 2D Mater. 5 025019
Google Scholar
[11] Sucharitakul S, Goble N J, Kumar U R, Sankar R, Bogorad Z A, Chou F, Chen Y T, Gao Xuan P A 2015 Nano Lett. 156 3815
Google Scholar
[12] Lauth J, Gorris F E S, Khoshkhoo M S, Chassé T, Friedrich W, Lebedeva V, Meyer A, Klinke C, Kornowski A, Scheele M, Weller H 2016 Chem. Mater. 28 1728
Google Scholar
[13] 范人杰, 江先燕, 陶奇睿, 梅期才, 唐颖菲, 陈志权, 苏贤礼, 唐新峰 2021 70 137102
Google Scholar
Fan R J, Jiang X Y, Tao Q R, Mei Q C, Tang Y F, Chen Z Q, Su X L, Tang X F 2021 Acta Phys. Sin. 70 137102
Google Scholar
[14] Pal S, Bose D N 1996 Solid State Commun. 97 725
Google Scholar
[15] Zólyomi V, Drummond N D, Falko V I 2014 Phys. Rev. B 89 205416
Google Scholar
[16] Jain M, Chelikowsky J R, Louie S, G, 2011 Phys. Rev. Lett. 107 216806
Google Scholar
[17] Ma H, Feng J, Jin F, Wei M, Liu C, Ma Y 2018 Nanoscale 10 15624
Google Scholar
[18] Yi Z, Wu M, Pang Y, Jia R, Xu R R 2021 Appl. Surf. Sci. 567 150842
Google Scholar
[19] Klots A R, Newaz A K M, Wang B, Prasai D, Krzyzanowska H, Lin Junhao, Caudel D, Ghimire N J, Yan J, Ivanov B L, Velizhanin K A, Burger A, Mandrus D G, Tolk N H, Pantelides S T, Bolotin K I 2014 Sci. Rep. 4 6608
Google Scholar
[20] Thygesen K S 2017 2D Mater. 4 022004
Google Scholar
[21] Jiang Y, Chen S, Zheng W, Zheng B, Pan A 2021 Light-Sci. Appl. 10 72
Google Scholar
[22] Qiu, D. Y. Jornada F da H, Louie S G 2013 Phys. Rev. Lett. 111 216805
Google Scholar
[23] Komsa, H P, Krasheninnikov A V 2012 Phys. Rev. B 86 241201(R
Google Scholar
[24] Shi H L, Pan H, Zhang Y W, Yakobson B I 2013 Phys. Rev. B 87 155304
Google Scholar
[25] Qiu D Y, Felipe H da J, Louie, S G 2017 Nano Lett. 17 4706
Google Scholar
[26] Raja A, Chaves A, Yu J, Arefe G, Hill Heather M, Rigosi Albert F, Berkelbach Timothy C, Nagler P, Schüller C, Korn T, Nuckolls C, Hone J, Brus Louis E, Heinz Tony F, Reichman David R, Chernikov A 2017 Nat. Commun. 8 15251
Google Scholar
[27] Ugeda Miguel M, Bradley Aaron J, Shi S F, Jornada Felipe H da, Zhang Y, Qiu Diana Y, Ruan W, Mo S K, Hussain Z, Shen Z X, Wang F, Louie Steven G, Crommie Michael F 2014 Nat. Mater. 13 1091
Google Scholar
[28] Enkovaara J, Rostgaard C, Mortensen J J, Chen J, Dułak M, Ferrighi L, Gavnholt J, Glinsvad C, Haikola V, Hansen H A, Kristoffersen H H, Kuisma M, Larsen A H, Lehtovaara L, Ljungberg M, Lopez-Acevedo O, Moses P G, Ojanen J, Olsen T, Petzold V, Romero N A, Stausholm-Møller J, Strange M, Tritsaris G A, Vanin M, Walter M, Hammer B, Häkkinen H, Madsen G K H, Nieminen R M, Nørskov J K, Puska M, Rantala T T, Schiøtz J, Thygesen K S, Jacobsen K W 2010 J. Phys. Condens. Mater. 22 253202
Google Scholar
[29] Blöchl P E 1994 Phys. Rev. B 50 17953
Google Scholar
[30] Perdew J P, Burke K 1996 Phys. Rev. Lett. 77 3865
Google Scholar
[31] Babaie-Kafaki S, Aminifard Z 2019 Numer. Algorithms 82 1345
Google Scholar
[32] Hüser F, Olsen T, Thygesen K S 2013 Phys. Rev. B 87 235132
Google Scholar
[33] Yan J, Mortensen J J, Jacobsen K W, Thygesen K S 2011 Phys. Rev. B 83 245122
Google Scholar
[34] Yi Z, Ma Y, Zheng Y, Duan Y, Li H 2019 Adv. Mater. Interfaces 6 1801175
Google Scholar
[35] Rozzi C A, Varsano D, Marini A, Gross E K U, Rubio A 2006 Phys. Rev. B 73 205119
Google Scholar
[36] Smail-Beigi S 2006 Phys. Rev. B 73 233103
Google Scholar
[37] Rasmussen F A, Schmidt P S, Winther K T, Thygesen K S 2016 Phys. Rev. B 94 155406
Google Scholar
[38] Olsen T 2016 Phys. Rev. B 94 235106
Google Scholar
[39] Tiago M L, Ismail-Beigi S, Louie S G 2004 Phys. Rev. B 69 125212
Google Scholar
[40] Klimeš J, Kaltak M, Kresse G 2014 Phys. Rev. B 90 075125
Google Scholar
[41] Haastrup S, Strange Mikke, Pandey M, Deilmann T, Schmidt Per S, Hinsche Nicki F, Gjerding Morten N, Torelli D, Larsen Peter M, Riis-Jensen Anders C 2018 2D Mater. 5 042002
Google Scholar
[42] Onida G, Reining L and Rubio A 2002 Rev. Mod. Phys. 74 601
Google Scholar
[43] 隋国民, 严桂俊, 杨光, 张宝, 冯亚青 2022 71 208801
Google Scholar
Sui G M, Yan G J, Yang G, Zhang B, Feng Y Q 2022 Acta Phys. Sin. 71 208801
Google Scholar
[44] Andersen K, Latini S, Thygesen K S 2015 Nano Lett. 15 4616
Google Scholar
[45] Pandey T, Parker David S, Lindsay L 2017 Nanotechnology 28 455706
Google Scholar
[46] Pang Y, Wu M, Zhang J, Yi Z 2021 J. Phys. Chem. C 125 3027
Google Scholar
下载:
计量
- 文章访问数: 4331
- PDF下载量: 111
- 被引次数: 0
