汇聚激波诱导具有正弦扰动双层重气柱界面的演化机理

党子涵; 郑纯; 张焕好; 陈志华

doi:10.7498/aps.71.20221012

摘要

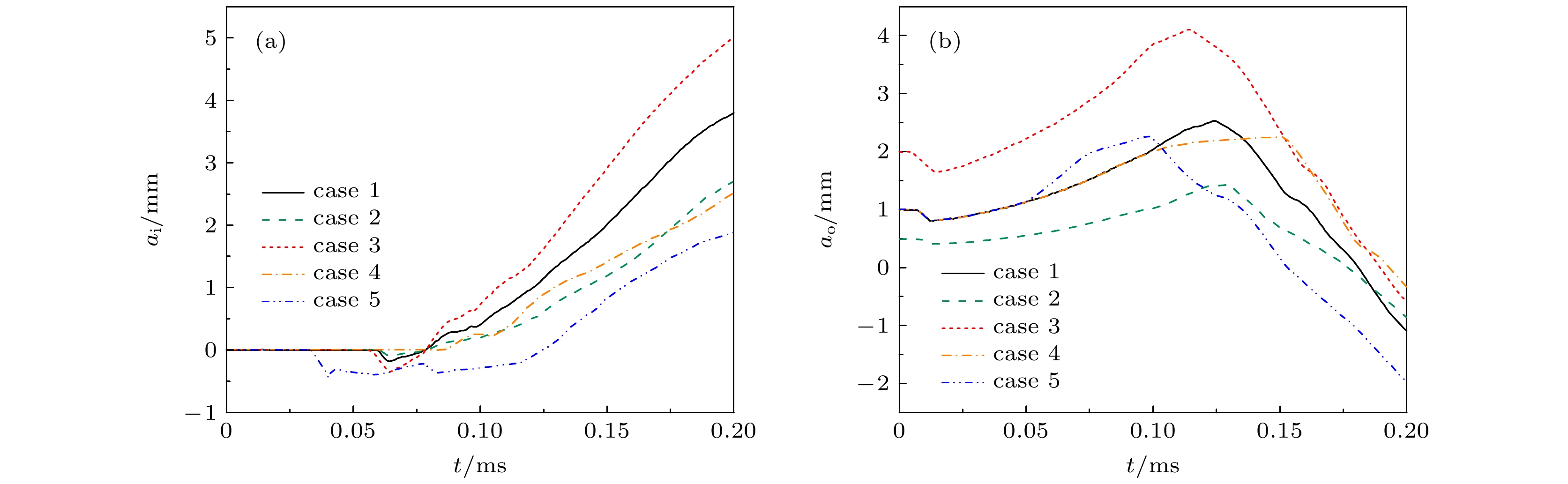
基于可压缩多组分Navier-Stokes方程, 结合5阶WENO (weighted essentially non-oscillatory)格式以及结构化自适应网格加密技术, 数值研究了汇聚激波冲击不同初始扰动幅值和气层厚度的双层SF₆重气柱界面不稳定性演化过程, 揭示了界面与激波结构相互作用及演变机理, 定量分析了环量、混合率及湍动能的变化规律, 并对涡量进行动模态分解. 结果表明: 初始扰动幅值较大的条件下, 气层内界面内外均形成马赫反射结构并在中心发生多次激波聚焦, 激波穿透外界面后环量增速更大, 内界面“尖钉”“气泡”更早发展, 内外界面幅值与混合率增速更大. 气层厚度较大时, 透射激波在重气柱内移动时相位发生改变, 使得内界面波峰向外发展而波谷向内发展. 气层厚度较小时, 内界面生成“尖钉”“气泡”较晚且不明显. 通过动模态分解可以发现: 耦合效应弱时, 低频弱增长的动模态决定了主干结构, 低频弱增长的动模态决定了主干结构上正负涡量的交换, 而高频弱增长的动模态决定了界面上正负涡量的快速交换.

关键词:

Abstract

Based on Navier-Stokes equations, combining the fifth-order weighted essentially non-oscillatory scheme with the adaptive structured grid refinement technique, the interactions between converging shock and annular SF₆ layers with different initial perturbation amplitudes and thickness are numerically investigated. The evolution mechanism of shock structure and interface are revealed in detail, and the variations of the circulation, mixing rate and turbulent kinetic energy are quantitatively analyzed. The dynamic mode decomposition method is used to analyze the dynamic characteristics of the vorticity. The results show that in the case with large initial perturbation amplitude, the transmitted shock wave forms Mach reflection structures both inside and outside of the inner interface of SF₆ layer, and multiple shock focusing phenomena occur in the center. After the transmitted shock wave penetrates the outer interface, the circulation increases faster, and the “spike” and “bubble” structure on inner interface develop faster, so that the amplitude of the inner and outer interfaces and the gas mixing rate increase. As for the case with larger thickness of the gas layer, the phase of the transmitted shock wave changes inside the layer, which forms “bubble” at the crest of the inner interface and “spike” at the trough. When the thickness of the gas layer decreases, the crest of the inner interface does not move inside after being impacted, and “spike” and “bubble” are generated in the late stage. The dynamic modes show that the main structure of vorticity and the exchange of positive and negative vorticity on the main structure are determined by the modes with weak growth and low frequency, but the modes with weak growth and high frequency determine rapid exchange of positive and negative vorticity at the interface in the cases with weak coupling effect.

Keywords:

作者及机构信息

1.
南京理工大学, 瞬态物理国家重点实验室, 南京　210094

2.
南京理工大学机械工程学院, 南京　210094

通信作者: 郑纯, Chun9211@njust.edu.cn ; 张焕好, zhanghuanhao@njust.edu.cn ;

基金项目: 国家自然科学基金(批准号: 12072162, 12102196)、江苏省自然科学基金(批准号: BK20210322)和中国博士后科学基金(批准号: 2022M711642)资助的课题.

Authors and contacts

1.
National Key Laboratory of Transient Physics, Nanjing University of Science and Technology, Nanjing 210094, China

2.
School of Mechanical Engineering, Nanjing University of Science and Technology, Nanjing 210094, China

Corresponding author: Zheng Chun, Chun9211@njust.edu.cn ; Zhang Huan-Hao, zhanghuanhao@njust.edu.cn ;

Funds: Project supported by the National Natural Science Foundation of China (Grant Nos. 12072162, 12102196), the Natural Science Foundation of Jiangsu Province, China (Grants No. BK20210322), and the China Postdoctoral Science Foundation (Grant No. 2022M711642).

文章全文 : translate this paragraph

参考文献

[1]	Yang J, Kubota T, Zukoski E E 1993 AIAA J. 31 854 Google Scholar
[2]	Cao L, Fei W L, Grosshans H, Cao N 2017 Appl. Sci. 7 880 Google Scholar
[3]	Lindl J D, McCrory R L, Campbell E M 1992 Phys. Today 45 32 Google Scholar
[4]	Richtmyer R D 1960 Commun. Pure Appl. Math. 13 297 Google Scholar
[5]	Fraley G 1986 Phys. Fluids 29 376 Google Scholar
[6]	Haehn N, Ranjan D, Weber C, Oakley J G, Anderson M H, Bonazza R 2010 Phys. Scr. T142 014067 Google Scholar
[7]	Haehn N, Weber C, Oakley J, Anderson M, Ranjan D, Bonazza R 2012 Shock Waves 22 47 Google Scholar
[8]	Luo X S, Wang M H, Si T, Zhai Z G 2015 J. Fluid Mech. 773 366 Google Scholar
[9]	沙莎, 陈志华, 薛大文, 张辉 2014 63 085205 Google Scholar Sha S, Chen Z H, Xue D W, Zhang H 2014 Acta Phys. Sin. 63 085205 Google Scholar
[10]	Mikaelian K O 1990 Phys. Rev. A 42 3400 Google Scholar
[11]	Lombardini M, Pullin D I 2009 Phys. Fluids 21 114103 Google Scholar
[12]	Si T, Long T, Zhai Z G, Luo X S 2015 J. Fluid Mech. 784 225 Google Scholar
[13]	Ding J C, Si T, Yang J M, Lu X Y, Zhai Z G, Luo X S 2017 Phys. Rev. Lett. 119 014501 Google Scholar
[14]	Ding J C, Li J M, Sun R, Zhai Z G, Luo X S 2019 J. Fluid Mech. 878 277 Google Scholar
[15]	Mikaelian K O 1995 Phys. Fluids 7 888 Google Scholar
[16]	Sun R, Ding J C, Zhai Z G, Si T, Luo X S 2020 J. Fluid Mech. 902 A3 Google Scholar
[17]	Li J M, Ding J C, Si T, Luo X S 2020 J. Fluid Mech. 884 R2 Google Scholar
[18]	徐建于, 黄生洪 2019 力学学报 51 998 Google Scholar Xu J Y, Huang S H 2019 Chin. J. Theor. Appl. Mech. 51 998 Google Scholar
[19]	梁煜, 关奔, 翟志刚, 罗喜胜 2017 66 064701 Google Scholar Liang Y, Guan B, Zhai Z G, Luo X S 2017 Acta Phys. Sin. 66 064701 Google Scholar
[20]	Zhou Z B, Ding J C, Zhai Z G, Cheng W, Luo X S 2020 Acta Mech. Sin. 36 356 Google Scholar
[21]	Tang J G, Zhang F, Luo X S, Zhai Z G 2020 Acta Mech. Sin. 37 434 Google Scholar
[22]	何惠琴, 翟志刚, 司廷, 罗喜胜 2016 计算物理 33 66 Google Scholar He H Q, Zhai Z G, Si T, Luo X S 2016 Chin. J. Comput. Phys. 33 66 Google Scholar
[23]	Fu Y W, Yu C P, Li X L 2020 AIP Adv. 10 105302 Google Scholar
[24]	Lombardini M, Hill D J, Pullin D I, Meiron D I 2011 J. Fluid Mech. 670 439 Google Scholar
[25]	Hill D J, Pullin D I 2004 J. Comput. Phys. 194 435 Google Scholar
[26]	Pantano C, Deiterding R, Hill D J, Pullin D I 2007 J. Comput. Phys. 221 63 Google Scholar
[27]	Henry D, Movahed P, Johnsen E 2015 Shock Waves 25 329 Google Scholar

施引文献

图 1 数值结果与文献^[14]中外界面(OI)、内界面(II)与激波(shock)位置的对比

Fig. 1. Comparison of variations of displacements of outer and inner interfaces (OI and II) and shock waves of experimental^[14] and numerical results.

下载: 全尺寸图片幻灯片

图 2 网格无关性检验

Fig. 2. Verification of the mesh resolution.

下载: 全尺寸图片幻灯片

图 3 计算模型示意图 (is, 初始激波; R, 外界面位置; R₀, 外界面平均半径; r₀, 内界面半径; a₀: 初始扰动幅值)

Fig. 3. Illustration of computational model (is, initial shock; R, location of outer interface; R₀, mean radius of outer interface; r₀, initial radius of inner interface; a₀, initial amplitude).

下载: 全尺寸图片幻灯片

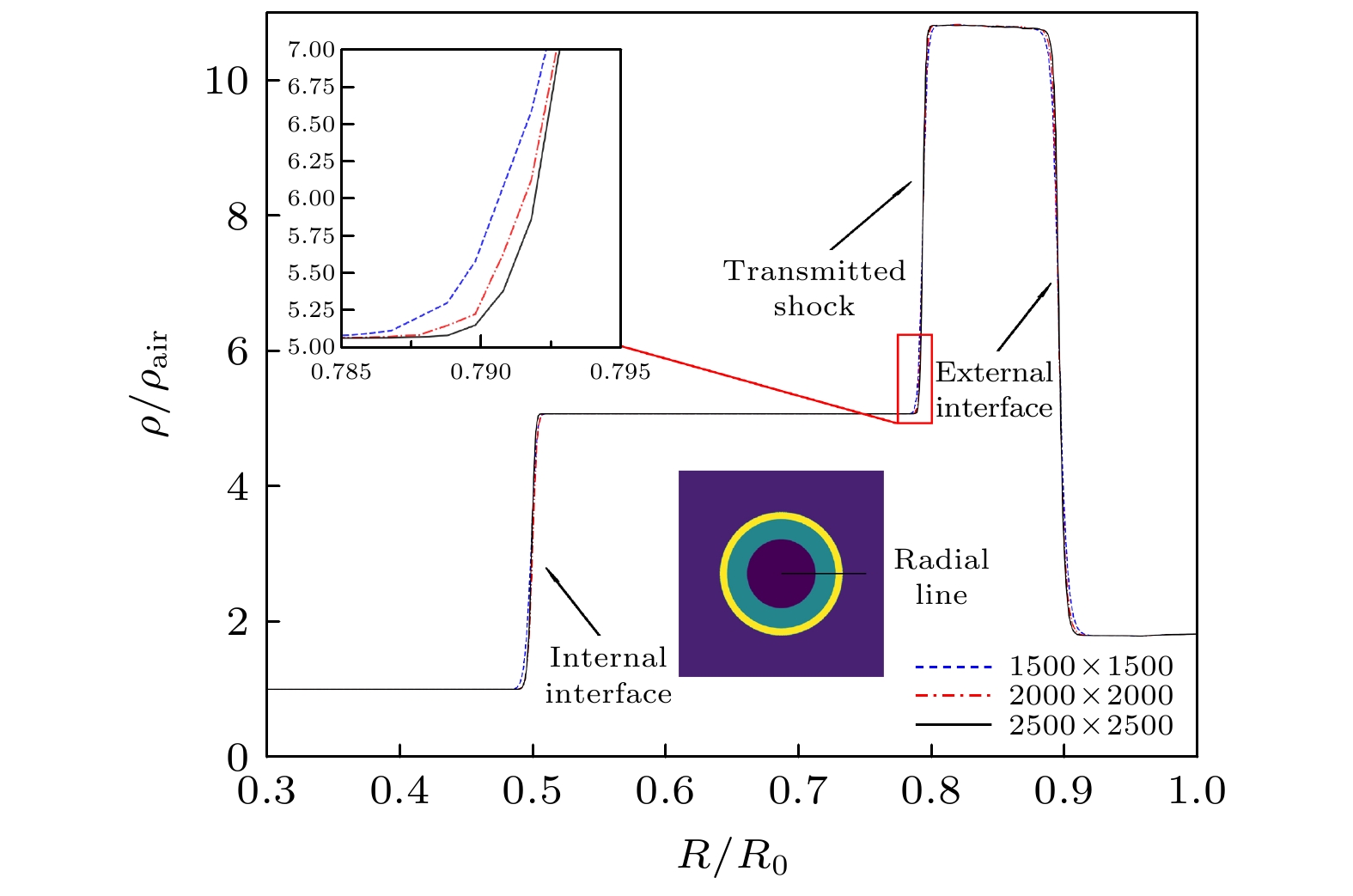
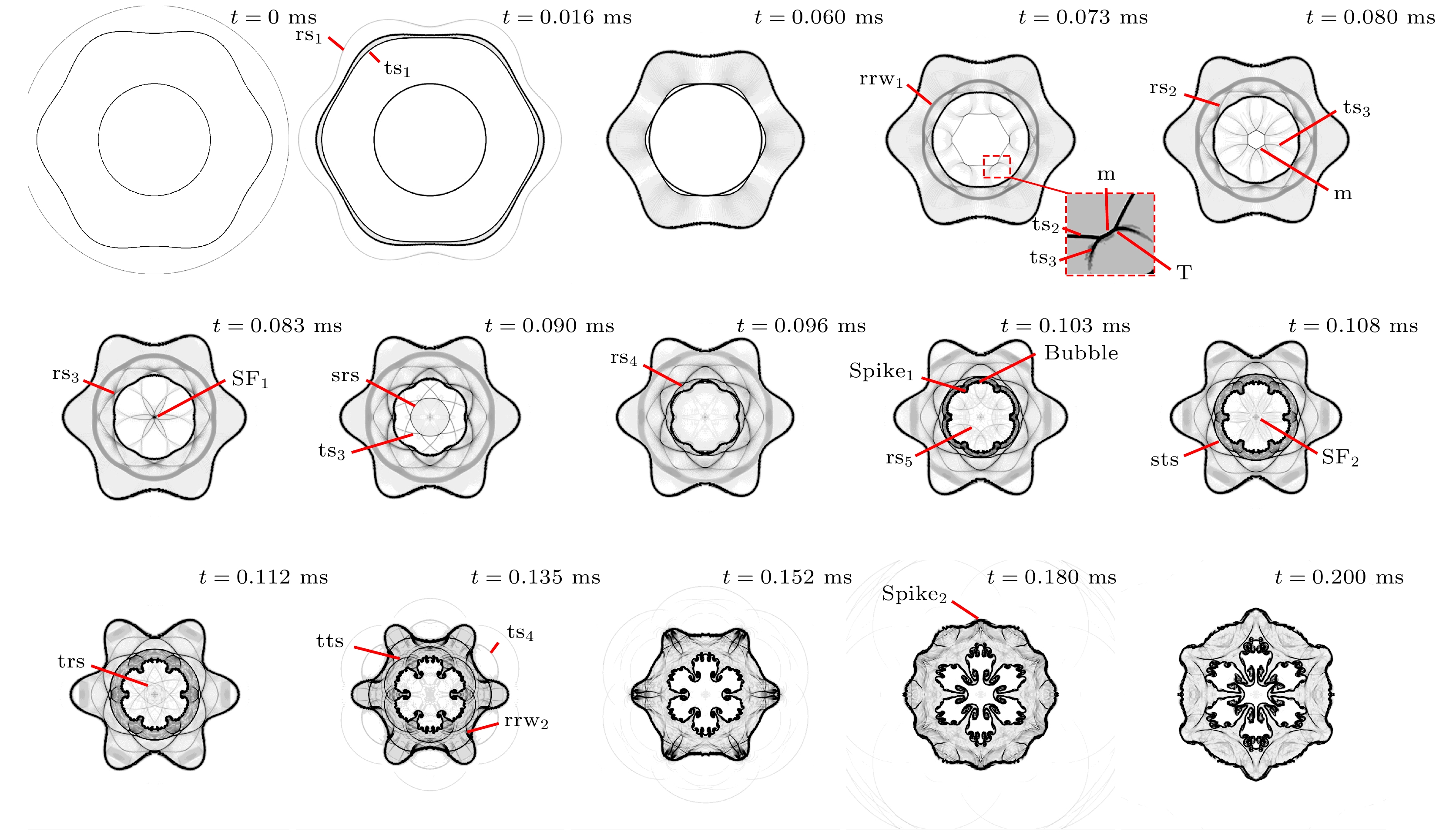
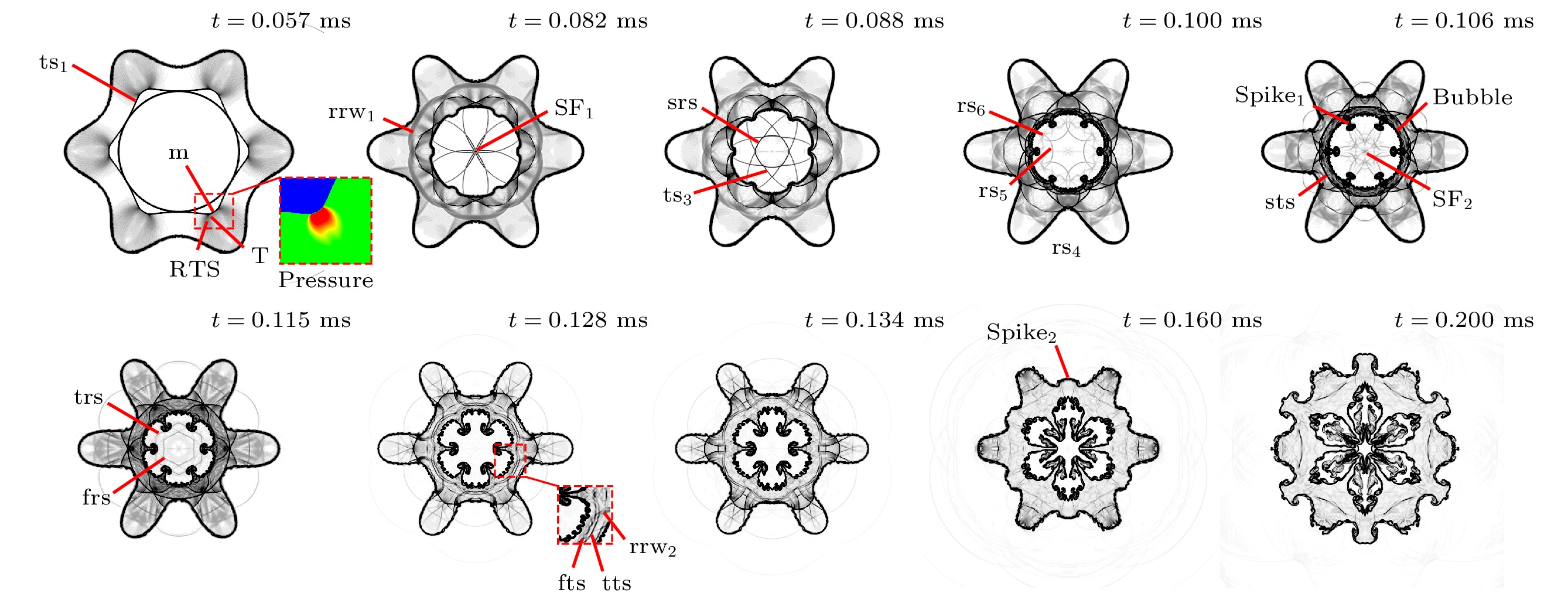
图 4 case 1的界面与激波结构演变过程示意图(ts, 透射激波; rs, 反射激波; rrw, 反射稀疏波; m, 马赫杆; T, 三波点; SF, 激波聚焦; srs, 二次反射激波; spike, “尖钉”结构; bubble, “气泡”结构; sts, 二次透射激波; trs, 三次反射激波; tts, 三次透射激波; 下文符号含义相同)

Fig. 4. Evolution of the interface and shock wave structures of case 1 (ts, transmitted shock; rs, reflected shock; rrw, reflected rarefaction wave; m, Mach stem; T, triple point; SF, shock focusing; srs, the second reflected shock; spike, “spike” structure; bubble; “bubble” structure; sts, the second transmitted shock; trs, the third reflected shock; tts, the third transmitted shock. The meaning of these abbreviations is similar hereinafter).

下载: 全尺寸图片幻灯片

图 5 case 1中不同时刻流场涡量分布图

Fig. 5. Distribution of vorticity at different times of case 1.

下载: 全尺寸图片幻灯片

图 6 case 2的界面与激波结构演变过程

Fig. 6. Evolution of the interface and shock wave structures of case 2.

下载: 全尺寸图片幻灯片

图 7 case 3的界面与激波结构演变过程(frs. 四次反射激波; fts, 四次透射激波)

Fig. 7. Evolution of the interface and shock wave structures of case 3 (frs, the forth reflected shock; fts, the forth transmitted shock).

下载: 全尺寸图片幻灯片

图 8 case 4的界面与激波结构演变过程

Fig. 8. Evolution of the interface and shock wave structures of case 4.

下载: 全尺寸图片幻灯片

图 9 case 5的界面与激波结构演变过程

Fig. 9. Evolution of the interface and shock wave structures of case 5.

下载: 全尺寸图片幻灯片

图 10 case 1中不同时刻压力沿径向分布图

Fig. 10. Variations of pressure along the radial of case 1.

下载: 全尺寸图片幻灯片

图 11 直角坐标下t = 0.1 ms时case 1的组分分布图

Fig. 11. Illustration of the fraction of SF₆ for case 1 in Cartesian coordinate system at t = 0.1 ms.

下载: 全尺寸图片幻灯片

图 12 cases 1—5中(a)内界面与(b)外界面扰动幅值演化过程

Fig. 12. Evolution of the amplitude of (a) inner interface and (b) outer interface of cases 1–5.

下载: 全尺寸图片幻灯片

图 13 cases 1—5中(a) 环量绝对值$ \left|{ \varGamma }\right| $与(b)混合率随时间变化情况

Fig. 13. Evolution of (a) absolute value of circulation $ \left|{ \varGamma }\right| $ and (b) mixing rate of cases 1–5.

下载: 全尺寸图片幻灯片

图 14 cases 1—5中湍动能随时间分布图　(a) case 1; (b) case 2; (c) case 3; (d) case 4; (e) case 5

Fig. 14. Distributions of turbulent kinetic energy (TKE) of (a) case 1, (b) case 2, (c) case 3, (d) case 4 and (e) case 5.

下载: 全尺寸图片幻灯片

图 15 cases 1—5中DMD频谱分布图　(a) case 1; (b) case 2; (c) case 3; (d) case 4; (e) case 5

Fig. 15. Distributions of the frequency spectrum of the DMD modes of (a) case 1, (b) case 2, (c) case 3, (d) case 4, and (e) case 5.

下载: 全尺寸图片幻灯片

图 16 (a)—(e) cases 1—5中涡量的DMD模态的实数部分　(a1)—(e1) DM₁; (a2)—(e2) DM₂; (a3)—(e3) DM₃; (a4)—(e4) DM₄

Fig. 16. (a)–(e) Representation of DMD modes with their real parts using contours of vorticity of cases 1–5: (a1)–(e1) DM₁; (a2)–(e2) DM₂; (a3)–(e3) DM₃; (a4)–(e4) DM₄.

下载: 全尺寸图片幻灯片

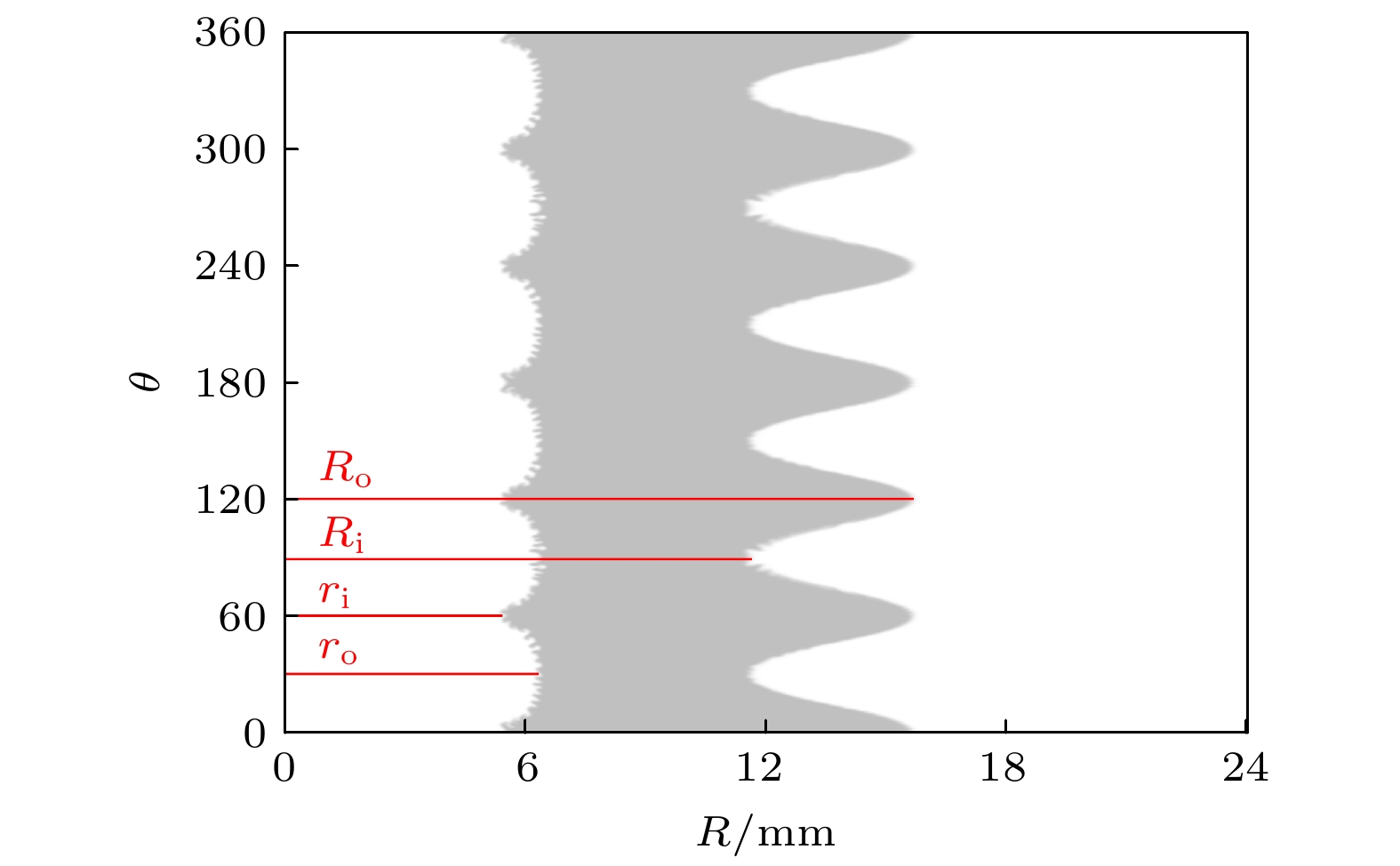
表 1 不同双层重气柱几何参数表

Table 1. Structural parameters of cylinder of different cases.

Case R₀/mm r₀/mm a₀/mm n λ/mm a₀/λ

Case 1 20 10 1 6 20.94 0.048
Case 2 20 10 0.5 6 20.94 0.024
Case 3 20 10 2 6 20.94 0.096
Case 4 20 5 1 6 20.94 0.048
Case 5 20 15 1 6 20.94 0.048

下载: 导出CSV

表 2 气体参数表

Table 2. Parameters of gases.

Gas γ M/(g·mol^–1) ρ/(kg·m^–3)

Air 1.399 28.967 1.23
SF₆ 1.103 128.491 5.45

下载: 导出CSV

map

[1]	Yang J, Kubota T, Zukoski E E 1993 AIAA J. 31 854 Google Scholar
[2]	Cao L, Fei W L, Grosshans H, Cao N 2017 Appl. Sci. 7 880 Google Scholar
[3]	Lindl J D, McCrory R L, Campbell E M 1992 Phys. Today 45 32 Google Scholar
[4]	Richtmyer R D 1960 Commun. Pure Appl. Math. 13 297 Google Scholar
[5]	Fraley G 1986 Phys. Fluids 29 376 Google Scholar
[6]	Haehn N, Ranjan D, Weber C, Oakley J G, Anderson M H, Bonazza R 2010 Phys. Scr. T142 014067 Google Scholar
[7]	Haehn N, Weber C, Oakley J, Anderson M, Ranjan D, Bonazza R 2012 Shock Waves 22 47 Google Scholar
[8]	Luo X S, Wang M H, Si T, Zhai Z G 2015 J. Fluid Mech. 773 366 Google Scholar
[9]	沙莎, 陈志华, 薛大文, 张辉 2014 63 085205 Google Scholar Sha S, Chen Z H, Xue D W, Zhang H 2014 Acta Phys. Sin. 63 085205 Google Scholar
[10]	Mikaelian K O 1990 Phys. Rev. A 42 3400 Google Scholar
[11]	Lombardini M, Pullin D I 2009 Phys. Fluids 21 114103 Google Scholar
[12]	Si T, Long T, Zhai Z G, Luo X S 2015 J. Fluid Mech. 784 225 Google Scholar
[13]	Ding J C, Si T, Yang J M, Lu X Y, Zhai Z G, Luo X S 2017 Phys. Rev. Lett. 119 014501 Google Scholar
[14]	Ding J C, Li J M, Sun R, Zhai Z G, Luo X S 2019 J. Fluid Mech. 878 277 Google Scholar
[15]	Mikaelian K O 1995 Phys. Fluids 7 888 Google Scholar
[16]	Sun R, Ding J C, Zhai Z G, Si T, Luo X S 2020 J. Fluid Mech. 902 A3 Google Scholar
[17]	Li J M, Ding J C, Si T, Luo X S 2020 J. Fluid Mech. 884 R2 Google Scholar
[18]	徐建于, 黄生洪 2019 力学学报 51 998 Google Scholar Xu J Y, Huang S H 2019 Chin. J. Theor. Appl. Mech. 51 998 Google Scholar
[19]	梁煜, 关奔, 翟志刚, 罗喜胜 2017 66 064701 Google Scholar Liang Y, Guan B, Zhai Z G, Luo X S 2017 Acta Phys. Sin. 66 064701 Google Scholar
[20]	Zhou Z B, Ding J C, Zhai Z G, Cheng W, Luo X S 2020 Acta Mech. Sin. 36 356 Google Scholar
[21]	Tang J G, Zhang F, Luo X S, Zhai Z G 2020 Acta Mech. Sin. 37 434 Google Scholar
[22]	何惠琴, 翟志刚, 司廷, 罗喜胜 2016 计算物理 33 66 Google Scholar He H Q, Zhai Z G, Si T, Luo X S 2016 Chin. J. Comput. Phys. 33 66 Google Scholar
[23]	Fu Y W, Yu C P, Li X L 2020 AIP Adv. 10 105302 Google Scholar
[24]	Lombardini M, Hill D J, Pullin D I, Meiron D I 2011 J. Fluid Mech. 670 439 Google Scholar
[25]	Hill D J, Pullin D I 2004 J. Comput. Phys. 194 435 Google Scholar
[26]	Pantano C, Deiterding R, Hill D J, Pullin D I 2007 J. Comput. Phys. 221 63 Google Scholar
[27]	Henry D, Movahed P, Johnsen E 2015 Shock Waves 25 329 Google Scholar

[1]	张升博, 张焕好, 张军, 毛勇建, 陈志华, 石启陈, 郑纯. 激波与轻质气柱作用过程的磁场抑制特性. , 2024, 73(8): 084701. doi: 10.7498/aps.73.20231916
[2]	孙贝贝, 叶文华, 张维岩. 密度扰动的类Richtmyer-Meshkov不稳定性增长及其与无扰动界面耦合的数值模拟. , 2023, 72(19): 194701. doi: 10.7498/aps.72.20230928
[3]	张升博, 张焕好, 陈志华, 郑纯. 不同界面组分分布对Richtmyer-Meshkov不稳定性的影响. , 2023, 72(10): 105202. doi: 10.7498/aps.72.20222090
[4]	袁永腾, 涂绍勇, 尹传盛, 李纪伟, 戴振生, 杨正华, 侯立飞, 詹夏宇, 晏骥, 董云松, 蒲昱东, 邹士阳, 杨家敏, 缪文勇. 冲击波波后辐射效应对Richtmyer-Meshkov不稳定性增长影响的实验研究. , 2021, 70(20): 205203. doi: 10.7498/aps.70.20210653
[5]	沙莎, 张焕好, 陈志华, 郑纯, 吴威涛, 石启陈. 纵向磁场抑制Richtmyer-Meshkov不稳定性机理. , 2020, 69(18): 184701. doi: 10.7498/aps.69.20200363
[6]	董国丹, 郭则庆, 秦建华, 张焕好, 姜孝海, 陈志华, 沙莎. 不同磁场构型下Richtmyer-Meshkov不稳定性的数值研究及动态模态分解. , 2019, 68(16): 165201. doi: 10.7498/aps.68.20190410
[7]	李冬冬, 王革, 张斌. 激波作用不同椭圆氦气柱过程中流动混合研究. , 2018, 67(18): 184702. doi: 10.7498/aps.67.20180879
[8]	董国丹, 张焕好, 林震亚, 秦建华, 陈志华, 郭则庆, 沙莎. 磁控条件下激波冲击三角形气柱过程的数值研究. , 2018, 67(20): 204701. doi: 10.7498/aps.67.20181127
[9]	殷建伟, 潘昊, 吴子辉, 郝鹏程, 段卓平, 胡晓棉. 爆轰驱动Cu界面的Richtmyer-Meshkov扰动增长稳定性. , 2017, 66(20): 204701. doi: 10.7498/aps.66.204701
[10]	梁煜, 关奔, 翟志刚, 罗喜胜. 激波汇聚效应对球形气泡演化影响的数值研究. , 2017, 66(6): 064701. doi: 10.7498/aps.66.064701
[11]	李俊涛, 孙宇涛, 胡晓棉, 任玉新. 激波冲击V形界面重气体导致的壁面与旋涡作用及其对湍流混合的影响. , 2017, 66(23): 235201. doi: 10.7498/aps.66.235201
[12]	李俊涛, 孙宇涛, 潘建华, 任玉新. 冲击加载下V形界面的失稳与湍流混合. , 2016, 65(24): 245202. doi: 10.7498/aps.65.245202
[13]	沙莎, 陈志华, 张庆兵. 激波与SF6球形气泡相互作用的数值研究. , 2015, 64(1): 015201. doi: 10.7498/aps.64.015201
[14]	沙莎, 陈志华, 薛大文, 张辉. 激波与SF6梯形气柱相互作用的数值模拟. , 2014, 63(8): 085205. doi: 10.7498/aps.63.085205
[15]	霍新贺, 王立锋, 陶烨晟, 李英骏. 非理想流体中Rayleigh-Taylor和Richtmyer-Meshkov不稳定性气泡速度研究. , 2013, 62(14): 144705. doi: 10.7498/aps.62.144705
[16]	沙莎, 陈志华, 薛大文. 激波冲击R22重气柱所导致的射流与混合研究. , 2013, 62(14): 144701. doi: 10.7498/aps.62.144701
[17]	陶烨晟, 王立锋, 叶文华, 张广财, 张建成, 李英骏. 任意Atwood数Rayleigh-Taylor和 Richtmyer-Meshkov 不稳定性气泡速度研究. , 2012, 61(7): 075207. doi: 10.7498/aps.61.075207
[18]	邹　秀, 宫　野, 刘金远, 宫继全. 外加磁场、电流及弧柱半径对电弧螺旋不稳定性的影响. , 2004, 53(3): 824-828. doi: 10.7498/aps.53.824
[19]	刘金远, 宫野, 王晓刚, 马腾才, 吕文彦. 等离子体放电柱磁螺旋不稳定性的线性理论. , 2000, 49(3): 502-507. doi: 10.7498/aps.49.502
[20]	顾永年, 邱乃贤. 椭圆截面等离子体柱的扭曲不稳定性. , 1980, 29(11): 1367-1377. doi: 10.7498/aps.29.1367

计量

文章访问数: 3262
PDF下载量: 36
被引次数: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

搜索

留言板

汇聚激波诱导具有正弦扰动双层重气柱界面的演化机理