-
基于倒Y型四能级系统, 理论研究了探测光吸收谱线的线宽窄化极限. 发现得益于中间激发态与另一超精细基态之间施加的第三束控制光, 线宽窄化极限的限制条件转变为两个基态能级之间的相干衰减率, 而非基态与高激发态之间. 与传统的梯型结构相比, 吸收光谱线宽的窄化极限能够提高2个数量级. 研究表明, 通过适当调节这束控制光的拉比频率和失谐量, 可以获得兼具超窄线宽和高对比度的吸收光谱信号. 数值计算结果与理论分析完全相符. 此外, 还讨论了吸收谱线对光场的响应规律和多普勒效应的影响. 对原子热运动的研究发现, 倒Y模型由于缺少三光子作用的过程而无法完全消除多普勒增宽的影响. 借助传播光场的优化设计可以减小多普勒效应的影响, 在有限温度下获得较窄的吸收谱线. 本文的研究成果对高分辨光谱学的实验发展具有重要的指导意义.Depending on a four-level inverted-Y atomic system, we demonstrate the limitation of linewidth-narrowing for the probe absorption spectrum in the electromagnetic induced absorption platform. Thanks to the use of an auxiliary control field which couples one hyperfine ground state and one middle-excited state we show that the linewidth limitation can be constrained by a coherence decay rate between two hyperfine ground states, rather than by the decay rate between the ground and the excited states as in previous Ladder schemes. That fact makes the theoretically-predicted absorption linewidth at least two orders of magnitude narrower. By using a suitable adjustment for the control-field amplitude and the detuning we numerically show that an extremely-narrowed probe absorption spectrum accompanied by a higher spectra contrast can be obtained, which confirms well with our theoretical predictions. We study the transient time response to the absorption spectrum and show that a relatively longer response time arises due to the small coherence decay rate between two hyperfine ground states. Furthermore, we reduce the influence on linewidth-narrowing from the Doppler effect via an optimized design of lasers, and reveal that no Doppler-free effect exists due to the lack of three-photon process. Our results may pave a route to the development of high-resolution spectroscopy in current experiments.
-
Keywords:
- spectrum linewidth /
- inverted-Y type atomic system /
- coherence decay rate /
- Doppler broadening
[1] Hartmann J M, Sironneau V, Boulet C, Svensson T, Hodges J T, Xu C T 2013 Phys. Rev. A 87 032510
Google Scholar
[2] Thomas T D, Kukk E, Ueda K, Ouchi T, Sakai K, Carroll T X, Nicolas C, Travnikova O, Miron C 2011 Phys. Rev. Lett. 106 193009
Google Scholar
[3] Lukin M D, Fleischhauer M, Zibrov A S, Robinson H G, Velichansky V L, Hollberg L, Scully M O 1997 Phys. Rev. Lett. 79 2959
Google Scholar
[4] Lambo R, Xu C Y, Pratt S T, Xu H, Zappala J C, Bailey K G, Lu Z T, Mueller P, O’Connor T P, Kamorzin B B, Bezrukov D S, Xie Y Q, Buchachenko A A, Singh J T 2021 Phys. Rev. A 104 062809
Google Scholar
[5] Budker D, Yashchuk V, Zolotorev M 1998 Phys. Rev. Lett. 81 5788
Google Scholar
[6] Iftiquar S M, Karve G R, Natarajan V 2008 Phys. Rev. A 77 063807
Google Scholar
[7] Tay J W, Farr W G, Ledingham P M, Korystov D, Longdell J J 2013 Phys. Rev. A 87 063824
Google Scholar
[8] Narducci L M, Scully M O, Oppo G L, Ru P, Tredicce J R 1990 Phys. Rev. A 42 1630
Google Scholar
[9] Gauthier D J, Zhu Y, Mossberg T W 1991 Phys. Rev. Lett. 66 2460
Google Scholar
[10] Zhu Y, Wasserlauf T N 1996 Phys. Rev. A 54 3653
Google Scholar
[11] Rapol U D, Wasan A, Natarajan V 2003 Phys. Rev. A 67 053802
Google Scholar
[12] Iftiquar S M, Natarajan V 2009 Phys. Rev. A 79 013808
Google Scholar
[13] Ye C Y, Zibrov A S, Rostovtsev Y, Scully M O 2002 Phys. Rev. A 65 043805
Google Scholar
[14] Goren C, Wilson-Gordon A D, Rosenbluh M, Friedmann H 2004 Phys. Rev. A 69 063802
Google Scholar
[15] Yang L J, Zhang L S, Zhuang Z H, Guo Q L, Fu G S 2008 Chin. Phys. B 17 2147
Google Scholar
[16] Mondal S, Ghosh A, Islam K, Bandyopadhyay A 2019 Laser Phys. 29 075204
Google Scholar
[17] Mu Y, Qin L, Shi Z Y, Huang G X 2021 Phys. Rev. A 103 043709
Google Scholar
[18] Hou B P, Wang S J, Yu W L, Sun W L 2004 Phys. Rev. A 69 053805
Google Scholar
[19] Dutta B K, Mahapatra P K 2008 J. Phys. B 41 055501
Google Scholar
[20] Qi J B 2010 Phys. Scr. 81 015402
Google Scholar
[21] Ghosh A, Islam K, Bhattacharyya D, Bandyopadhyay A 2016 J. Phys. B 49 195401
Google Scholar
[22] Liao K Y, Tu H T, Yang S Z, Chen C J, Liu X H, Liang J, Zhang X D, Yan H, Zhu S L 2020 Phys. Rev. A 101 053432
Google Scholar
[23] Naweed A, Farca G, Shopova S I, Rosenberger A T 2005 Phys. Rev. A 71 043804
Google Scholar
[24] Stassi R, Macrì V, Kockum A F, Stefano O D, Miranowicz A, Savasta S, Nori F 2017 Phys. Rev. A 96 023818
Google Scholar
[25] Adhikari P, Hafezi M, Taylor J M 2013 Phys. Rev. Lett. 110 060503
Google Scholar
[26] Chai X, Ropagnol X, Raeis-Zadeh S M, Reid M, Safavi-Naeini S, Ozaki T 2018 Phys. Rev. Lett. 121 143901
Google Scholar
[27] Prehn A, Ibrügger M, Rempe G, Zeppenfeld M 2021 Phys. Rev. Lett. 127 173602
Google Scholar
[28] Gustin C, Hanschke L, Boos K, Müller J R A, Kremser M, Finley J J, Hughes S, Müller K 2021 Phys. Rev. Res. 3 013044
Google Scholar
[29] Rose W, Haas H, Chen A Q, Jeon N, Lauhon L J, Cory D G, Budakian R 2018 Phys. Rev. X 8 011030
[30] Yan D, Liu Y M, Bao Q Q, Fu C B, Wu J H 2012 Phys. Rev. A 86 023828
Google Scholar
[31] Li Y, Xiao M 1995 Phys. Rev. A 51 4959
Google Scholar
[32] Giner L, Veissier L, Sparkes B, Sheremet A S, Nicolas A, Mishina O S, Scherman M, Burks S, Shomroni I, Kupriyanov D V, Lam P K, Giacobino E, Laurat J 2013 Phys. Rev. A 87 013823
Google Scholar
[33] Zhu C J, Tan C H, Huang G X 2013 Phys. Rev. A 87 043813
Google Scholar
[34] Anisimov P M, Dowling J P, Sanders B C 2011 Phys. Rev. Lett. 107 163604
Google Scholar
[35] Sheng D, Pérez Galván A, Orozco L A 2008 Phys. Rev. A 78 062506
Google Scholar
[36] Bharti V, Wasan A 2012 J. Phys. B 45 185501
Google Scholar
[37] 周炳琨 2009 激光原理 (北京: 国防工业出版社) 第129页
Zhou B K 2009 Laser Principle (Beijing: National Defense Industry Press) p129 (in Chinese)
[38] Berman P R, Salomaa R 1982 Phys. Rev. A 25 2667
Google Scholar
[39] Schmidt-Eberle S, Stolz T, Rempe G, Dürr S 2020 Phys. Rev. A 101 013421
Google Scholar
[40] Stiesdal N, Busche H, Kumlin J, Kleinbeck K, Büchler H P, Hofferberth S 2020 Phys. Rev. Res. 2 043339
Google Scholar
[41] Pack M V, Camacho R M, Howell J C 2007 Phys. Rev. A 76 013801
Google Scholar
[42] Feng L, Li P X, Zhang M Z, Wang T, Xiao Y H 2014 Phys. Rev. A 89 013815
Google Scholar
[43] Van Dyke J S, Kandel Y P, Qiao H F, Nichol J M, Economou S E, Barnes E 2021 Phys. Rev. B 103 245303
Google Scholar
[44] Blok M S, Ramasesh V V, Schuster T, O’Brien K, Kreikebaum J M, Dahlen D, Morvan A, Yoshida B, Yao N Y, Siddiqi I 2021 Phys. Rev. X 11 021010
[45] Zhang Y, Qiao J B, Yin L J, He L 2018 Phys. Rev. B 98 045413
Google Scholar
[46] de Boo G G, Yin C M, Rančić M, Johnson B C, McCallum J C, Sellars M J, Rogge S 2020 Phys. Rev. B 102 155309
Google Scholar
[47] Longhi S 2008 Phys. Rev. A 77 015807
Google Scholar
[48] Yang Z J, Lustig E, Harari G, Plotnik Y, Lumer Y, Bandres M A, Segev M 2020 Phys. Rev. X 10 011059
[49] Bai S Y, Bao Q Q, Tian X D, Liu Y M, Wu J H 2018 J. Phys. B 51 075502
Google Scholar
[50] Chen T L, Chang S Y, Huang Y J, Shukla K, Huang Y C, Suen T H, Kuan T Y, Shy J T, Liu Y W 2020 Phys. Rev. A 101 052507
Google Scholar
[51] Tauschinsky A, Newell R, van Linden van den Heuvell H B, Spreeuw R J C 2013 Phys. Rev. A 87 042522
Google Scholar
[52] Kou J, Wan R G, Kang Z H, Wang H H, Jiang L, Zhang X J, Jiang Y, Gao J Y 2010 J. Opt. Soc. Am. B 27 002035
Google Scholar
[53] Ryabtsev I I, Beterov I I, Tretyakov D B, Entin V M, Yakshina E A 2011 Phys. Rev. A 84 053409
Google Scholar
-
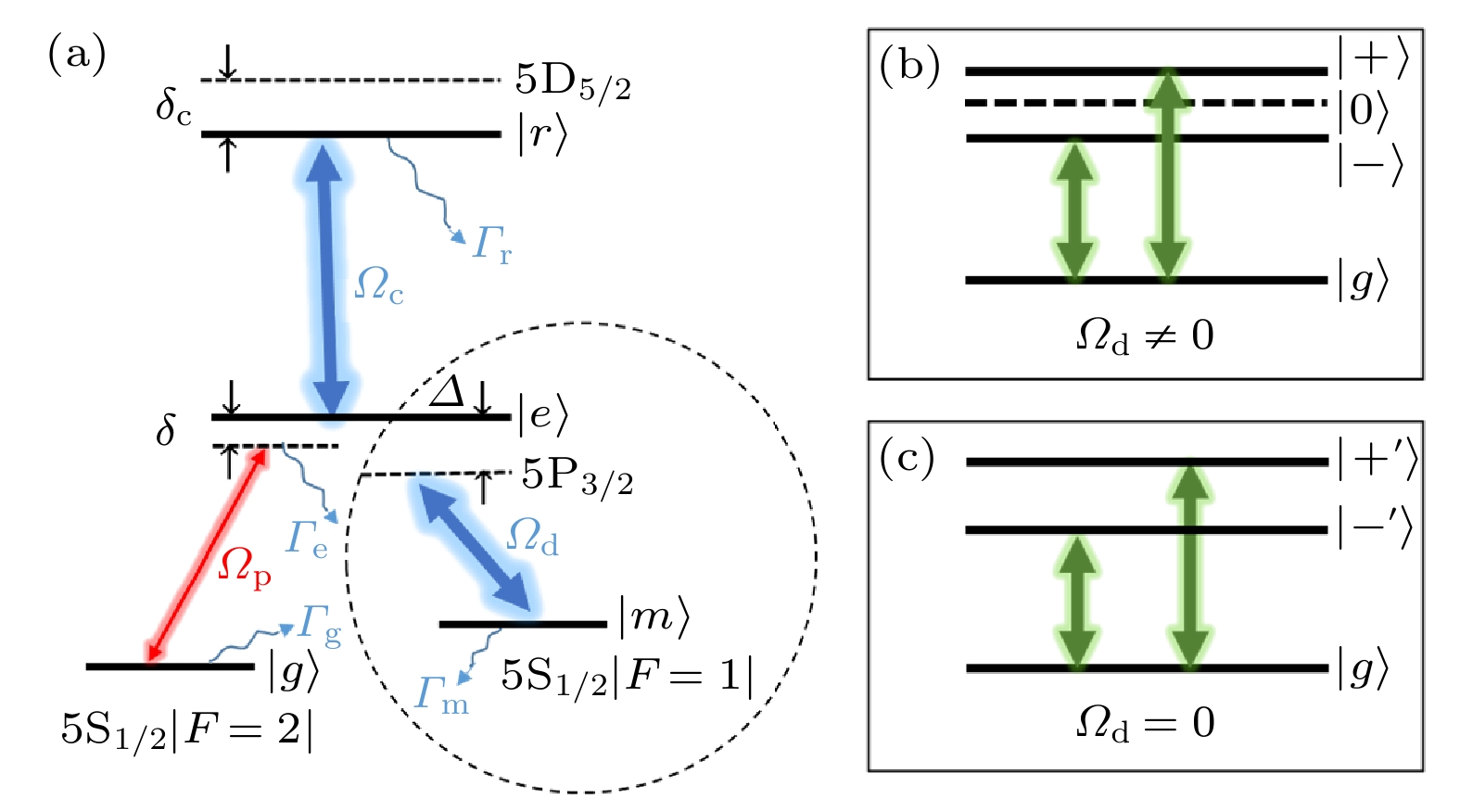
图 1 (a) 倒Y型四能级原子系统的裸态能级示意图; (b) 考虑
$ {\varOmega }_{\mathrm{d}}\ne 0 $ 时, 在满足$\varDelta +{\delta }_{\mathrm{c}}=0$ 的情况下缀饰态能级$ \left|\pm \rangle\right. $ 与基态$ \left|g\rangle\right. $ 发生耦合, 而能级$ \left|0\rangle\right. $ 由于不包含裸态$ \left|e\rangle\right. $ , 故不与$ \left|g\rangle\right. $ 耦合; (c) 反映了不存在控制光$ {\varOmega }_{\mathrm{d}} $ 的情况下, 系统约化为三能级梯型结构所对应的缀饰态能级$\left|{\pm' }\rangle\right.$ 与基态$ \left|g\rangle\right. $ 之间的耦合Fig. 1. (a) Schematic of an inverted-Y type four-level atomic system coupling with three light fields
$ {\varOmega }_{\mathrm{p}}, {\varOmega }_{\mathrm{c}}, {\varOmega }_{\mathrm{d}} $ ; (b) for$ {\varOmega }_{\mathrm{d}}\ne 0 $ and$\varDelta +{\delta }_{\mathrm{c}}=0$ , the ground state$ \left|g\rangle\right. $ only couples with$ \left|\pm \rangle\right. $ (dressed states); (c) While$ {\varOmega }_{\mathrm{d}}=0 $ , the system reduces to a three-level ladder structure where$ \left|g\rangle\right. $ couples with the other two dressed states$ \left|{\pm'}\rangle\right. $ .
图 2 (a)
$ {\delta =\delta }_{+} $ 处吸收谱线的线宽$ {w}_{+D} $ 与$ {\varOmega }_{\mathrm{d}} $ 的关系. 红色虚线是根据(6)式得到的理论结果, 蓝色实线是数值计算的结果. (b)$ {\delta =\delta }_{+} $ 处吸收谱线的对比度$ {\eta }_{\mathrm{c}\mathrm{o}\mathrm{n}\mathrm{t}\mathrm{r}\mathrm{a}\mathrm{s}\mathrm{t}} $ 与$ {\varOmega }_{\mathrm{d}} $ 的依赖关系. 计算所取参数为$ {\varOmega }_{\mathrm{p}}=0.03 $ ,$ {\varOmega }_{\mathrm{c}}=0.3 $ ,$\varDelta =15$ ,$ {\delta }_{\mathrm{c}}=-15 $ . 箭头所指位置表示对比度$ {\eta }_{\mathrm{c}\mathrm{o}\mathrm{n}\mathrm{t}\mathrm{r}\mathrm{a}\mathrm{s}\mathrm{t}} $ 达到0.99对应的$ {\varOmega }_{\mathrm{d}} $ 和$ {w}_{+D} $ 的取值Fig. 2. (a) Absorption linewidth
$ {w}_{+D} $ vs the coupling-field Rabi frequency$ {\varOmega }_{\mathrm{d}} $ . Theoretical (Eq. (6)) and numerical results are plotted by red-dashed and blue-solid curves, respectively. (b) Spectrum contrast$ {\eta }_{\mathrm{c}\mathrm{o}\mathrm{n}\mathrm{t}\mathrm{r}\mathrm{a}\mathrm{s}\mathrm{t}} $ vs$ {\varOmega }_{\mathrm{d}} $ for$ {\delta =\delta }_{+} $ . Arrow shows the location of (${{\varOmega }_{\mathrm{d}}, w}_{+D}, {\eta }_{\mathrm{c}\mathrm{o}\mathrm{n}\mathrm{t}\mathrm{r}\mathrm{a}\mathrm{s}\mathrm{t}})= (1.1{\varGamma }_{e}, $ $ 23\text{ KHz}, 0.99)$ . Simulation parameters are$ {\varOmega }_{\mathrm{p}}=0.03 $ ,$ {\varOmega }_{\mathrm{c}}= $ $ 0.3 $ ,$\varDelta =15$ and$ {\delta }_{\mathrm{c}}=-15 $ .
图 3 三能级梯型系统中, 在
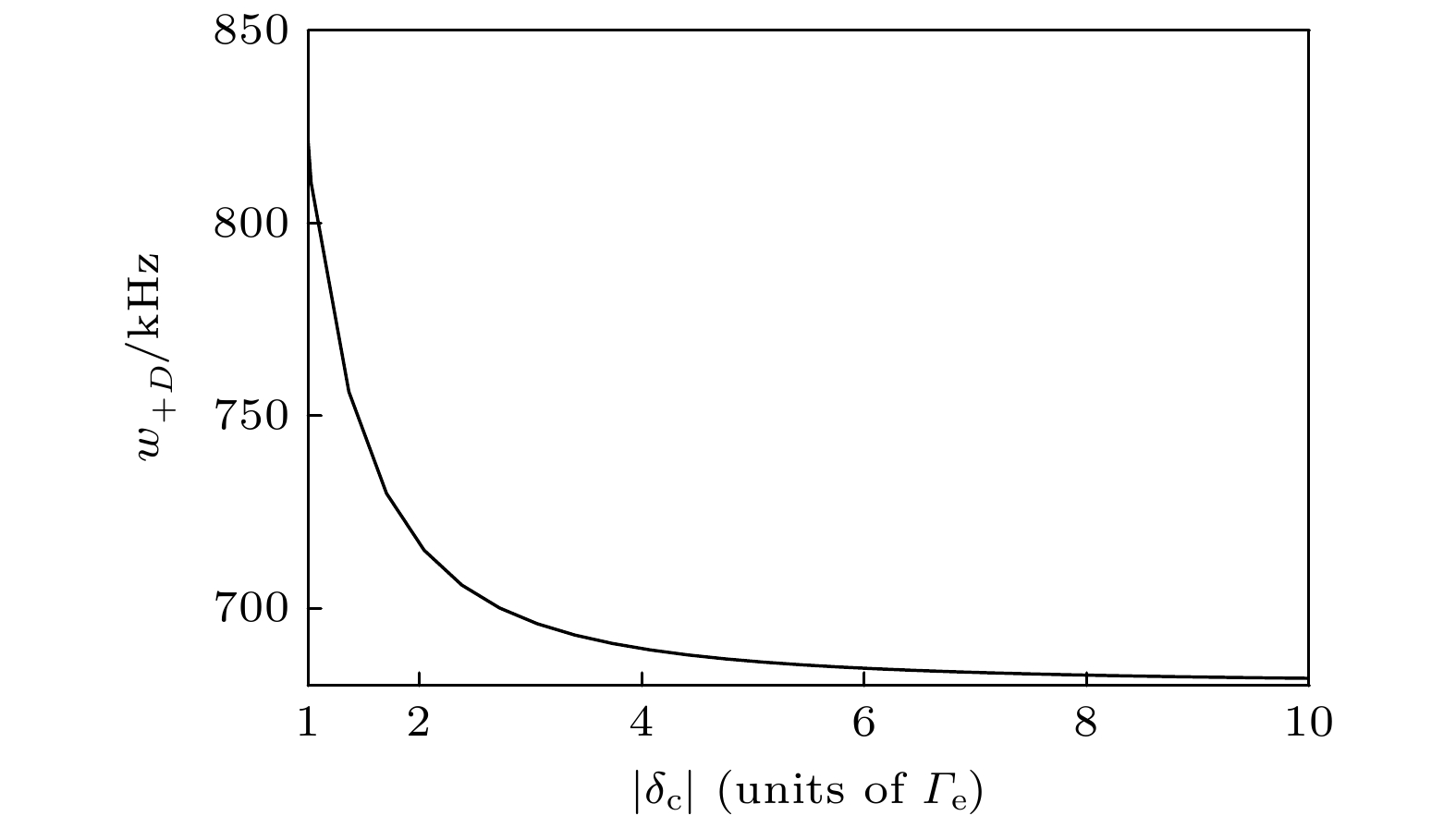
$\delta ={{\delta' _{+}}}$ 位置处吸收谱线线宽${{w'_{+D}}}$ 与控制光失谐量$ \left|{\delta }_{\mathrm{c}}\right| $ 的依赖关系. 这里取$ {\varOmega }_{\mathrm{p}}=0.03 $ ,$ {\varOmega }_{\mathrm{c}}=0.3 $ Fig. 3. In a three-level Ladder system the spectrum linewidth
${w'_{+D}}$ vs detuning$ \left|{\delta }_{\mathrm{c}}\right| $ . Here$ {\varOmega }_{\mathrm{p}}=0.03 $ ,$ {\varOmega }_{\mathrm{c}}=0.3 $ .
图 4
$ \left(\mathrm{a}\right){\varOmega }_{\mathrm{d}}=0.5 $ 和$ \left(\mathrm{b}\right){\varOmega }_{\mathrm{d}}=1.1 $ 两种情况下,$ \delta ={\delta }_{+} $ 处吸收谱线宽度$ {w}_{+D} $ (蓝色实线)和对比度$ {\eta }_{\mathrm{c}\mathrm{o}\mathrm{n}\mathrm{t}\mathrm{r}\mathrm{a}\mathrm{s}\mathrm{t}} $ (红色虚线)随失谐量$\varDelta$ 的变化关系. 其他参数和图2相同Fig. 4. Dependence of spectrum linewidth
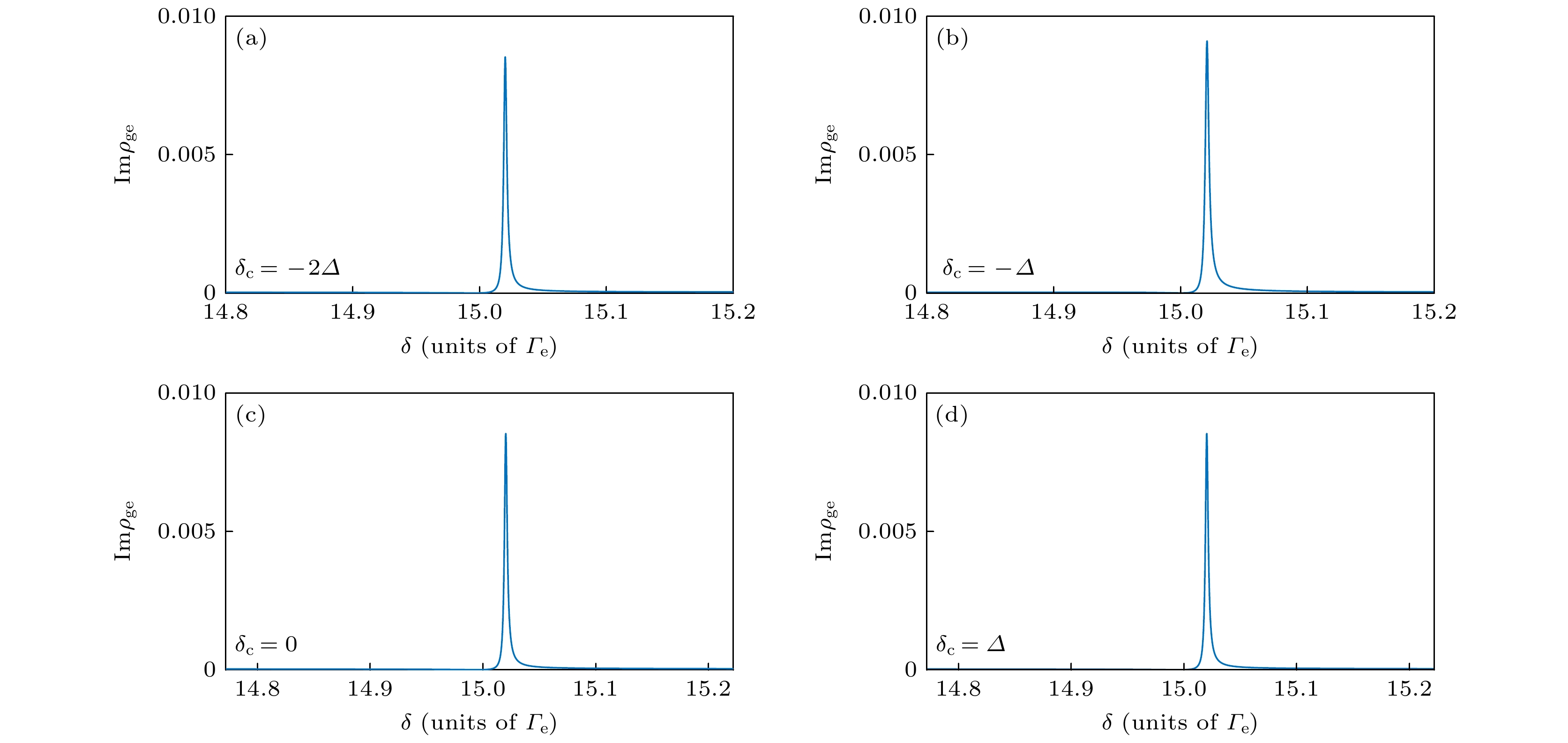
$ {w}_{+D} $ (blue-solid) and contrast$ {\eta }_{contrast} $ (red-dashed) on the detuning$\varDelta$ under (a)$ {\varOmega }_{\mathrm{d}}= $ $ 0.5 $ and (b)$ {\varOmega }_{\mathrm{d}}=1.1 $ . Other parameters are same as in Fig. 2.
图 5 (a)—(d)不同的
$ {\delta }_{\mathrm{c}} $ 取值下,$ \delta ={\delta }_{+} $ 位置附近的吸收谱线. 这里取$ {\varOmega }_{\mathrm{d}}=1.1 $ ,$\varDelta =15$ , 其他参数和图2相同Fig. 5. (a)–(d) Absorption spectrum around
$ \delta ={\delta }_{+} $ for${\delta }_{\mathrm{c}}=(-2\varDelta , -\varDelta , 0, \varDelta )$ . Here we choose$ {\varOmega }_{\mathrm{d}}=1.1 $ and$\varDelta =15$ . Other parameters have been described in Fig. 2.
图 6 考虑控制光场
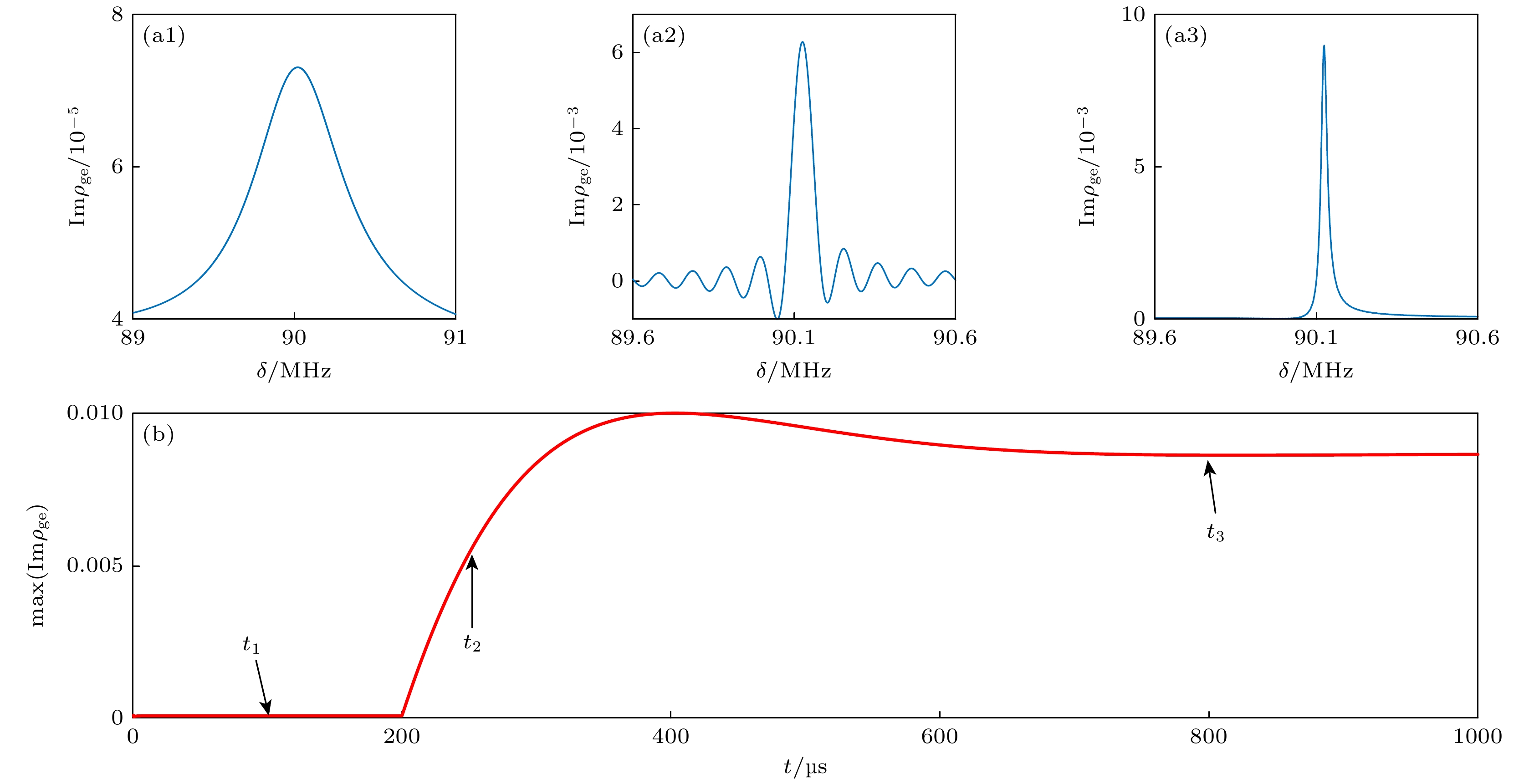
$ {\varOmega }_{\mathrm{d}}\left(t\right) $ 在$ t=200\text{ μ}\mathrm{s} $ 时开启, (a1)—(a3)当$ t=({t}_{1}, {t}_{2}, {t}_{3})=(100, \mathrm{250, 800})\text{ μ}\mathrm{s} $ 时$ \delta ={\delta }_{+} $ 位置处的吸收谱线; (b) 该处吸收谱线的峰值高度M$ \mathrm{a}\mathrm{x}\left(\mathrm{I}\mathrm{m}{\rho }_{\mathrm{g}\mathrm{e}}\right) $ 随时间$ t $ 的变化. 当$ t < 200\text{ μ}\mathrm{s} $ 时,$ {\varOmega }_{\mathrm{d}}\left(t\right)=0 $ ; 当$t\geqslant 200\text{ μ}\mathrm{s}$ 时,${\varOmega }_{\mathrm{d}}\left(t\right)=6.6\text{ MHz}$ Fig. 6. In the case of a time-dependent control field which is
$ {\varOmega }_{\mathrm{d}}\left(t\right)=0 $ for$ t < 200\text{ μ}\mathrm{s} $ and${\varOmega }_{\mathrm{d}}\left(t\right)=6.6\text{ MHz}$ for$t\geqslant 200\text{ μ}\mathrm{s}$ . (a1)–(a3) The absorption spectrum at$\delta ={\delta }_{+}\approx 90.12\text{ MHz}$ when$ t=({t}_{1}, {t}_{2}, {t}_{3})=(100, \mathrm{250, 800})\text{ μ}\mathrm{s}, $ respectively. (b) Time-dependent peak absorption$ \mathrm{M}\mathrm{a}\mathrm{x}\left(\mathrm{I}\mathrm{m}{\rho }_{\mathrm{g}\mathrm{e}}\right(t\left)\right) $ as a function of time t. The control field is turned on at$ t=200\text{ μ}\mathrm{s} $ .
图 7 不同的温度
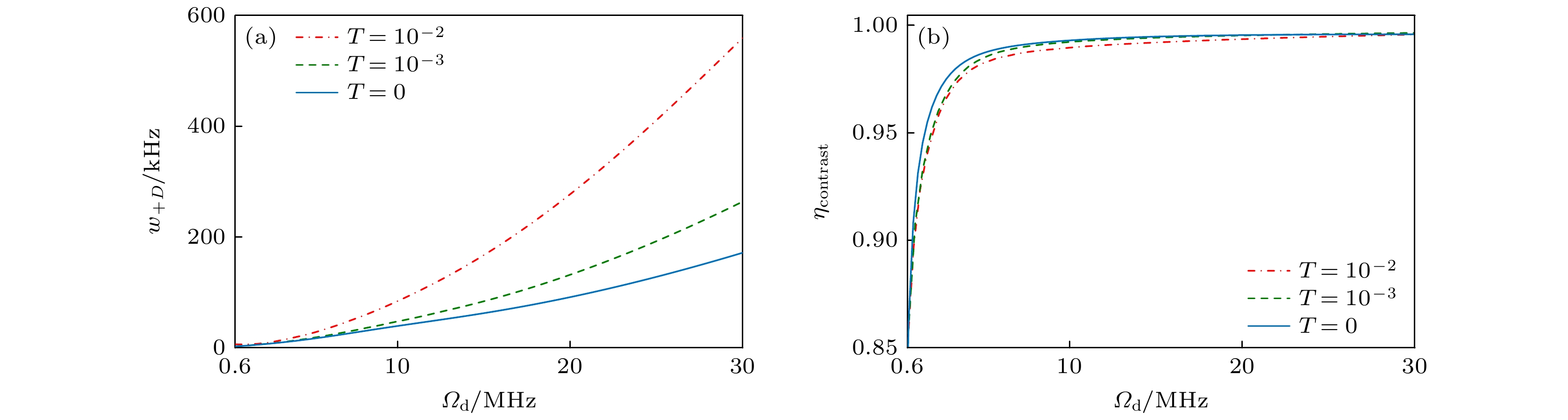
$T=\left({10}^{-2}, {10}^{-3}, 0\right)\text{ K}$ 下, (a)$ {w}_{+D} $ 和(b)$ {\eta }_{\mathrm{c}\mathrm{o}\mathrm{n}\mathrm{t}\mathrm{r}\mathrm{a}\mathrm{s}\mathrm{t}} $ 与$ {\varOmega }_{\mathrm{d}} $ 的依赖关系. 选取的参数是$\varDelta =90$ MHz,${\delta }_{\mathrm{c}}=-\varDelta$ , 其他和图2相同Fig. 7. Under different temperatures
$ T=\left({10}^{-2}, {10}^{-3}, 0\right) $ K, (a)$ {w}_{+D} $ and (b)$ {\eta }_{\mathrm{c}\mathrm{o}\mathrm{n}\mathrm{t}\mathrm{r}\mathrm{a}\mathrm{s}\mathrm{t}} $ vs the control-field Rabi frequency$ {\varOmega }_{\mathrm{d}} $ . Here$\varDelta =90$ MHz and${\delta }_{\mathrm{c}}=-\varDelta$ and others are the same as in Fig. 2. -
[1] Hartmann J M, Sironneau V, Boulet C, Svensson T, Hodges J T, Xu C T 2013 Phys. Rev. A 87 032510
Google Scholar
[2] Thomas T D, Kukk E, Ueda K, Ouchi T, Sakai K, Carroll T X, Nicolas C, Travnikova O, Miron C 2011 Phys. Rev. Lett. 106 193009
Google Scholar
[3] Lukin M D, Fleischhauer M, Zibrov A S, Robinson H G, Velichansky V L, Hollberg L, Scully M O 1997 Phys. Rev. Lett. 79 2959
Google Scholar
[4] Lambo R, Xu C Y, Pratt S T, Xu H, Zappala J C, Bailey K G, Lu Z T, Mueller P, O’Connor T P, Kamorzin B B, Bezrukov D S, Xie Y Q, Buchachenko A A, Singh J T 2021 Phys. Rev. A 104 062809
Google Scholar
[5] Budker D, Yashchuk V, Zolotorev M 1998 Phys. Rev. Lett. 81 5788
Google Scholar
[6] Iftiquar S M, Karve G R, Natarajan V 2008 Phys. Rev. A 77 063807
Google Scholar
[7] Tay J W, Farr W G, Ledingham P M, Korystov D, Longdell J J 2013 Phys. Rev. A 87 063824
Google Scholar
[8] Narducci L M, Scully M O, Oppo G L, Ru P, Tredicce J R 1990 Phys. Rev. A 42 1630
Google Scholar
[9] Gauthier D J, Zhu Y, Mossberg T W 1991 Phys. Rev. Lett. 66 2460
Google Scholar
[10] Zhu Y, Wasserlauf T N 1996 Phys. Rev. A 54 3653
Google Scholar
[11] Rapol U D, Wasan A, Natarajan V 2003 Phys. Rev. A 67 053802
Google Scholar
[12] Iftiquar S M, Natarajan V 2009 Phys. Rev. A 79 013808
Google Scholar
[13] Ye C Y, Zibrov A S, Rostovtsev Y, Scully M O 2002 Phys. Rev. A 65 043805
Google Scholar
[14] Goren C, Wilson-Gordon A D, Rosenbluh M, Friedmann H 2004 Phys. Rev. A 69 063802
Google Scholar
[15] Yang L J, Zhang L S, Zhuang Z H, Guo Q L, Fu G S 2008 Chin. Phys. B 17 2147
Google Scholar
[16] Mondal S, Ghosh A, Islam K, Bandyopadhyay A 2019 Laser Phys. 29 075204
Google Scholar
[17] Mu Y, Qin L, Shi Z Y, Huang G X 2021 Phys. Rev. A 103 043709
Google Scholar
[18] Hou B P, Wang S J, Yu W L, Sun W L 2004 Phys. Rev. A 69 053805
Google Scholar
[19] Dutta B K, Mahapatra P K 2008 J. Phys. B 41 055501
Google Scholar
[20] Qi J B 2010 Phys. Scr. 81 015402
Google Scholar
[21] Ghosh A, Islam K, Bhattacharyya D, Bandyopadhyay A 2016 J. Phys. B 49 195401
Google Scholar
[22] Liao K Y, Tu H T, Yang S Z, Chen C J, Liu X H, Liang J, Zhang X D, Yan H, Zhu S L 2020 Phys. Rev. A 101 053432
Google Scholar
[23] Naweed A, Farca G, Shopova S I, Rosenberger A T 2005 Phys. Rev. A 71 043804
Google Scholar
[24] Stassi R, Macrì V, Kockum A F, Stefano O D, Miranowicz A, Savasta S, Nori F 2017 Phys. Rev. A 96 023818
Google Scholar
[25] Adhikari P, Hafezi M, Taylor J M 2013 Phys. Rev. Lett. 110 060503
Google Scholar
[26] Chai X, Ropagnol X, Raeis-Zadeh S M, Reid M, Safavi-Naeini S, Ozaki T 2018 Phys. Rev. Lett. 121 143901
Google Scholar
[27] Prehn A, Ibrügger M, Rempe G, Zeppenfeld M 2021 Phys. Rev. Lett. 127 173602
Google Scholar
[28] Gustin C, Hanschke L, Boos K, Müller J R A, Kremser M, Finley J J, Hughes S, Müller K 2021 Phys. Rev. Res. 3 013044
Google Scholar
[29] Rose W, Haas H, Chen A Q, Jeon N, Lauhon L J, Cory D G, Budakian R 2018 Phys. Rev. X 8 011030
[30] Yan D, Liu Y M, Bao Q Q, Fu C B, Wu J H 2012 Phys. Rev. A 86 023828
Google Scholar
[31] Li Y, Xiao M 1995 Phys. Rev. A 51 4959
Google Scholar
[32] Giner L, Veissier L, Sparkes B, Sheremet A S, Nicolas A, Mishina O S, Scherman M, Burks S, Shomroni I, Kupriyanov D V, Lam P K, Giacobino E, Laurat J 2013 Phys. Rev. A 87 013823
Google Scholar
[33] Zhu C J, Tan C H, Huang G X 2013 Phys. Rev. A 87 043813
Google Scholar
[34] Anisimov P M, Dowling J P, Sanders B C 2011 Phys. Rev. Lett. 107 163604
Google Scholar
[35] Sheng D, Pérez Galván A, Orozco L A 2008 Phys. Rev. A 78 062506
Google Scholar
[36] Bharti V, Wasan A 2012 J. Phys. B 45 185501
Google Scholar
[37] 周炳琨 2009 激光原理 (北京: 国防工业出版社) 第129页
Zhou B K 2009 Laser Principle (Beijing: National Defense Industry Press) p129 (in Chinese)
[38] Berman P R, Salomaa R 1982 Phys. Rev. A 25 2667
Google Scholar
[39] Schmidt-Eberle S, Stolz T, Rempe G, Dürr S 2020 Phys. Rev. A 101 013421
Google Scholar
[40] Stiesdal N, Busche H, Kumlin J, Kleinbeck K, Büchler H P, Hofferberth S 2020 Phys. Rev. Res. 2 043339
Google Scholar
[41] Pack M V, Camacho R M, Howell J C 2007 Phys. Rev. A 76 013801
Google Scholar
[42] Feng L, Li P X, Zhang M Z, Wang T, Xiao Y H 2014 Phys. Rev. A 89 013815
Google Scholar
[43] Van Dyke J S, Kandel Y P, Qiao H F, Nichol J M, Economou S E, Barnes E 2021 Phys. Rev. B 103 245303
Google Scholar
[44] Blok M S, Ramasesh V V, Schuster T, O’Brien K, Kreikebaum J M, Dahlen D, Morvan A, Yoshida B, Yao N Y, Siddiqi I 2021 Phys. Rev. X 11 021010
[45] Zhang Y, Qiao J B, Yin L J, He L 2018 Phys. Rev. B 98 045413
Google Scholar
[46] de Boo G G, Yin C M, Rančić M, Johnson B C, McCallum J C, Sellars M J, Rogge S 2020 Phys. Rev. B 102 155309
Google Scholar
[47] Longhi S 2008 Phys. Rev. A 77 015807
Google Scholar
[48] Yang Z J, Lustig E, Harari G, Plotnik Y, Lumer Y, Bandres M A, Segev M 2020 Phys. Rev. X 10 011059
[49] Bai S Y, Bao Q Q, Tian X D, Liu Y M, Wu J H 2018 J. Phys. B 51 075502
Google Scholar
[50] Chen T L, Chang S Y, Huang Y J, Shukla K, Huang Y C, Suen T H, Kuan T Y, Shy J T, Liu Y W 2020 Phys. Rev. A 101 052507
Google Scholar
[51] Tauschinsky A, Newell R, van Linden van den Heuvell H B, Spreeuw R J C 2013 Phys. Rev. A 87 042522
Google Scholar
[52] Kou J, Wan R G, Kang Z H, Wang H H, Jiang L, Zhang X J, Jiang Y, Gao J Y 2010 J. Opt. Soc. Am. B 27 002035
Google Scholar
[53] Ryabtsev I I, Beterov I I, Tretyakov D B, Entin V M, Yakshina E A 2011 Phys. Rev. A 84 053409
Google Scholar
下载:
计量
- 文章访问数: 4028
- PDF下载量: 56
- 被引次数: 0
