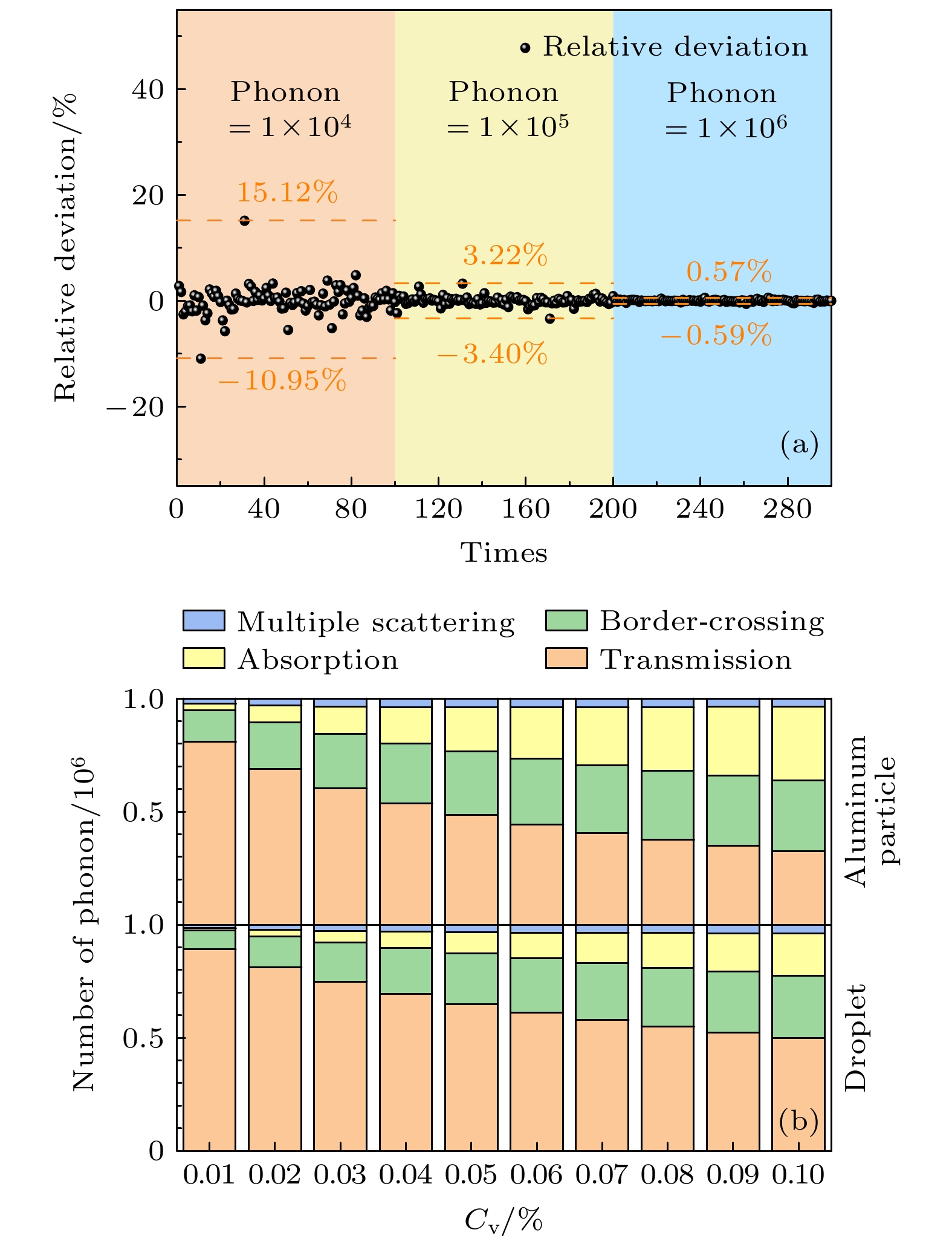
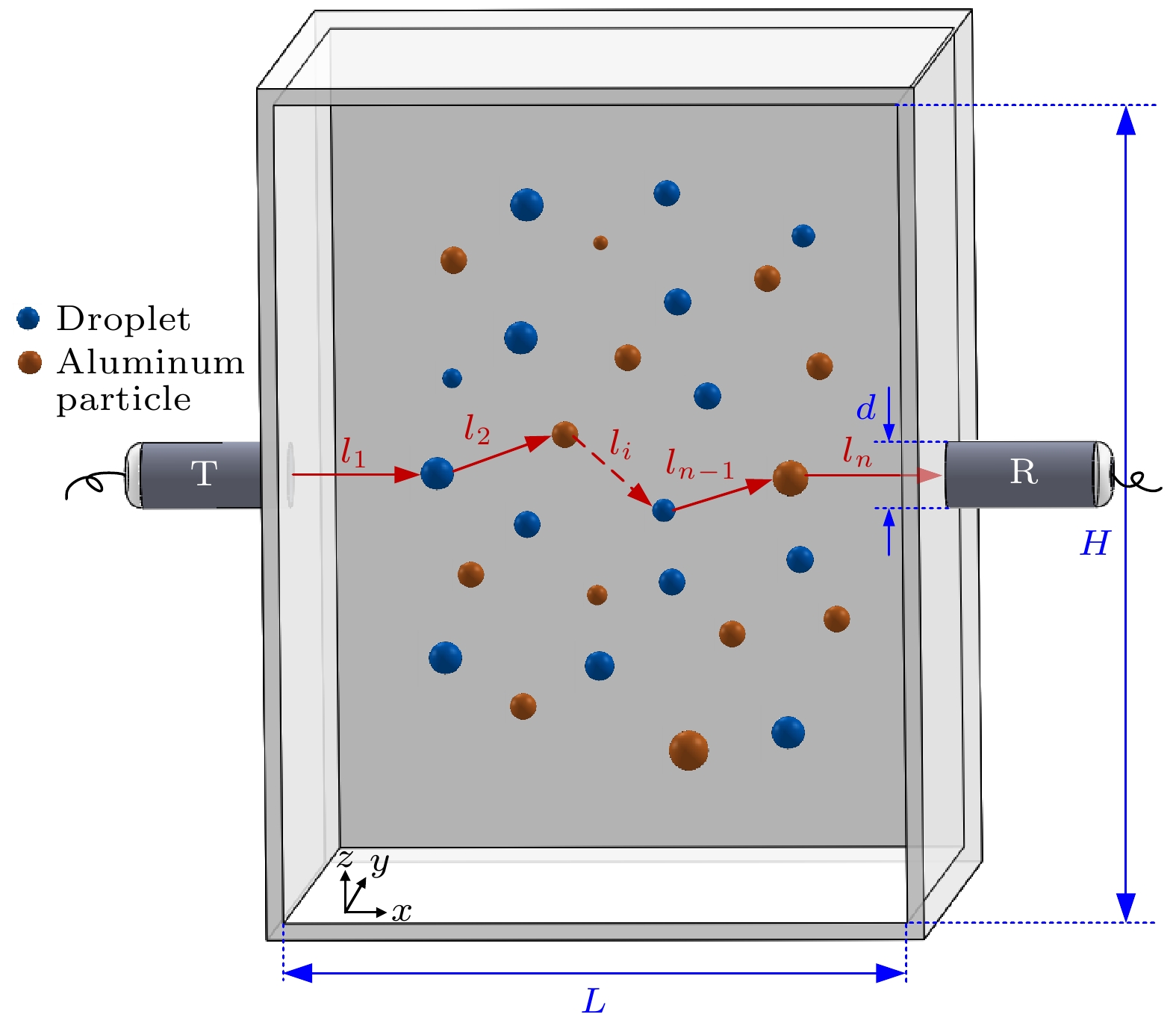
-
从单个固体和液滴颗粒的声吸收和散射特性计算入手, 基于概率统计的蒙特卡罗方法(MCM), 将声波以离散化的声子加以处理, 通过追踪其运动历程并进行事件统计, 建立一种气体介质中球形混合颗粒的声衰减预测模型. 对空气中铝粉颗粒和亚微米级水滴的声衰减分别计算和验证后, 将模型推广至含有混合颗粒的三相体系, 对铝粉和液滴构成的单、多分散混合颗粒体系进行数值研究. 结果表明: 两类颗粒的声吸收特性差异明显, 其散射声压均随颗粒无因次尺寸kR的增加呈现从后向散射占主要地位逐渐过渡到前向增强的趋势. 气液固混合颗粒三相体系中, 颗粒类型对于声衰减影响明显、且随浓度的增加不同颗粒的衰减贡献不再遵循随混合比的线性递变关系; 对于多分散体系而言, 声衰减谱受平均粒径影响较大, 对于粒径分布宽度参数则不敏感. 模型可进一步结合数学反演形成混合颗粒体系测量的理论基础.From the perspective of calculating ultrasonic absorption and scattering properties of individual solid particle and droplet, the ultrasonic wave is treated as discrete phonons. And by tracking their motion process and event statistics, a new prediction model of ultrasonic attenuation of spherical mixed particles in gaseous medium is established with Monte Carlo method. Considering the difference in physical properties between solid particles and liquid particles, the ultrasonic absorption characteristics of the two kinds of particles are obviously different, and when dimensionless particle size kR ≤ 1, the backscattering of particles is uniform and dominant, then the ultrasonic scattering pressures gradually transit from the dominant position of backscattering to the trend of forward enhancement with the increase of dimensionless particle size. The numerical simulation results for the system with a single particle type are compared with those from various standard models such as classical ECAH model and McC model, showing that they are in good agreement. Similarly, the results are then compared with experimental results, which accord with each other in general. After calculating and verifying the ultrasonic attenuation of aluminum particles and submicron droplets respectively in air, the method is extended to the three-phase monodisperse and polydisperse mixed particle system composed of aluminum particles and liquid droplets. In the three-phase system of gas-liquid-solid mixed particles, the particle type has a significant influence on ultrasonic attenuation, and the attenuation contribution of different particles against mixing ratio does not follow the linear gradient with the increase of volume concentration. For a polydisperse system, the ultrasonic attenuation spectrum is greatly affected by the average particle size, but it is insensitive to the width of particle size distribution. The numerical results also show that both the particle type and particle distribution size should be carefully take into account in the polydisperse system. Moreover, the MCM model can be further extended to non-spherical particles and combined with mathematical inversion to form the theoretical basis for the measurement of mixed particle system.
-
Keywords:
- Monte Carlo method /
- mixed particle three-phase system /
- polydisperse /
- ultrasonic attenuation
[1] 黄婷 2018 博士学位论文(成都: 西南石油大学)
Huang T 2018 Ph. D. Dissertation (Chengdu: Southwest Petroleum University) (in Chinese)
[2] 葛春亮, 蒋楠, 刘文榉, 厉雄峰, 李晨朗 2021 热力发电 50 103
Google Scholar
Ge C L, Jing N, Liu W J, Li X F, Li C L 2021 Therm. Power Gener. 50 103
Google Scholar
[3] 黄正梁, 王超, 李少硕, 杨遥, 孙婧元, 王靖岱, 阳永荣 2020 化工学报 71 274
Huang Z L, Wang C, Li S S, Yang Y, Sun J Y, Wang J D, Yang Y R 2020 J. Chem. Ind. Eng. 71 274
[4] 陶芳芳, 宁尚雷, 靳海波 2020 过程工程学报 20 371
Google Scholar
Tao F F, Ning S L, Jin H B 2020 Chin. J. Process Eng. 20 371
Google Scholar
[5] 万梓傲, 童永霞, 陈思井, 周金荣 2020 舰船电子工程 40 174
Google Scholar
Wan Z A, Tong Y X, Chen S J, Zhou J R 2020 Ship Electron. Eng. 40 174
Google Scholar
[6] Epstein P S, Carhart R R 1953 J. Acoust. Soc. Am. 25 553
Google Scholar
[7] Allegra J R, Hawley S A 1972 J. Acoust. Soc. Am. 51 1545
Google Scholar
[8] Mcclements D J, Hemar Y, Herrmann N 1999 J. Acoust. Soc. Am. 105 915
Google Scholar
[9] Mcclements D J, Coupland J N 1996 Colloids Surf., A 117 161
Google Scholar
[10] Mcclements D J 1992 J. Acoust. Soc. Am. 91 849
Google Scholar
[11] Parker N G, Povey M J W 2012 Food Hydrocolloids Oxford 26 99
Google Scholar
[12] Liu L 2009 Chem. Eng. Sci. 64 5036
Google Scholar
[13] Dong L L, Su M X, Xue M H, Cai X S, Shang Z T 2007 AIP Conf. Proc. 914 654
Google Scholar
[14] Wang Q, Attenborough K, Woodhead S 2000 J. Sound Vib. 236 781
Google Scholar
[15] Wang Q, Attenborough K, Woodhead S 2001 Proc. Inst. Mech. Eng. Part E 215 133
Google Scholar
[16] 杜娜, 苏明旭 2019 应用声学 38 980
Google Scholar
Du N, Su M X 2019 J. Appl. Acoust. 38 980
Google Scholar
[17] 侯森, 胡长青, 赵梅 2021 70 044301
Google Scholar
Hou S, Hu C Q, Zhao M 2021 Acta Phys. Sin. 70 044301
Google Scholar
[18] 陈时, 张迪, 王成会, 张引红 2019 68 074301
Google Scholar
Chen S, Zhang D, Wang C H, Zhang Y H 2019 Acta Phys. Sin. 68 074301
Google Scholar
[19] 郭盼盼, 苏明旭, 陈丽, 蔡小舒 2014 过程工程学报 14 562
Guo P P, Su M X, Chen L, Cai X S 2014 Chin. J. Process Eng. 14 562
[20] 李运思, 苏明旭, 杨荟楠, 凡凤仙, 蔡小舒 2017 声学学报 42 586
Google Scholar
Li Y S, Su M X, Yang H N, Fan F X, Cai X S 2017 Acta. Acust. 42 586
Google Scholar
[21] Huang B F, Fan F X, Li Y S, Su M X 2019 Ultrason. 94 218
Google Scholar
[22] 冷坤, 章曦, 武文远, 龚艳春, 杨云涛 2018 物理与工程 28 74
Google Scholar
Leng K, Zhang X, Wu W Y, Gong Y C, Yang Y T 2018 Phys. Eng. 28 74
Google Scholar
[23] 王敏, 申玉清, 陈震宇, 徐鹏 2021 计算物理 38 623
Google Scholar
Wang M, Shen Y Q, Chen Z Y, Xu P 2021 Chin. J. Comput. Phys. 38 623
Google Scholar
[24] Hay A E, Mercer D G 1985 J. Acoust. Soc. Am. 78 1761
Google Scholar
[25] Faran J J 1951 J. Acoust. Soc. Am. 23 405
Google Scholar
[26] 冯若 1999 超声手册 (南京: 南京大学出版社) 第66页
Feng R 1999 Ultrasonic Handbook (Nanjing: Nanjing University Press) p66 (in Chinese)
[27] Wang M, Zheng D, Dong J, Hu J 2021 IEEE Trans. Instrum. Meas. 70 1
Google Scholar
-
图 1 声子在混合颗粒体系中传播过程
Fig. 1. Schematic of a phonon propagation through a mixed particle system.
图 3 液滴和铝粉的声散射及吸收系数比
Fig. 3. Ratio of scattering and absorption coefficients of droplet and aluminum particle.
图 5 不同条件声子统计结果 (a) 不同声子数目时相对偏差; (b) 不同体积浓度时声子去向
Fig. 5. Statistic of phonons under different conditions: (a) Relative deviations at different number of phonons; (b) phonon events at different particle volume concentrations.
图 6 模型验证及物性敏感性 (a) 声衰减系数随颗粒半径变化; (b) 声衰减系数随颗粒物性参数变化
Fig. 6. Model validation and the sensitivity of physical parameters: (a) Ultrasonic attenuation coefficient varies with the particle radius; (b) ultrasonic attenuation coefficient varies with the particle parameters.
图 7 实验结果对比 (a) 铝粉颗粒; (b) 液滴颗粒 (R1和R2分别为文献中超声和图像法测得液滴半径)
Fig. 7. Comparison with experimental results: (a) Aluminum particle; (b) droplet (R1 and R2 are droplet radius measured by ultrasonic and image method in the reference respectively).
图 8 单分散三相混合体系声衰减
Fig. 8. Ultrasonic attenuation coefficient in monodisperse mixed system.
图 10 多分散三相混合体系声衰减 (a) 随频率变化; (b) 随浓度变化
Fig. 10. Ultrasonic attenuation coefficient in polydisperse mixed system: (a) Curves with frequency; (b) curves with volume concentration.
表 1 数值计算中介质和颗粒物性参数(25 ℃)
Table 1. Parameters in the numerical simulation (25 ℃)
密度
ρ/(kg·m–3)声速
c/(m·s–1)剪切模量
G/Pa比热容
cp/(J·kg–1·K–1)黏度
μ/(Pa·s)热膨胀
系数 β/K–1声吸收
系数 aw/(Np·m–1)空气 1.21 334.3 — 1004 1.81 × 10–4 3.661 × 10–4 1.7 × 10–11 液滴 1000 1497 — 4178.5 9.03 × 10–4 2.57 × 10–4 2.2 × 10–14 铝粉 3800 5000 2.63 × 1010 769 — 2.65 × 10–5 1.3 × 10–9 
下载: 导出CSV
-
[1] 黄婷 2018 博士学位论文(成都: 西南石油大学)
Huang T 2018 Ph. D. Dissertation (Chengdu: Southwest Petroleum University) (in Chinese)
[2] 葛春亮, 蒋楠, 刘文榉, 厉雄峰, 李晨朗 2021 热力发电 50 103
Google Scholar
Ge C L, Jing N, Liu W J, Li X F, Li C L 2021 Therm. Power Gener. 50 103
Google Scholar
[3] 黄正梁, 王超, 李少硕, 杨遥, 孙婧元, 王靖岱, 阳永荣 2020 化工学报 71 274
Huang Z L, Wang C, Li S S, Yang Y, Sun J Y, Wang J D, Yang Y R 2020 J. Chem. Ind. Eng. 71 274
[4] 陶芳芳, 宁尚雷, 靳海波 2020 过程工程学报 20 371
Google Scholar
Tao F F, Ning S L, Jin H B 2020 Chin. J. Process Eng. 20 371
Google Scholar
[5] 万梓傲, 童永霞, 陈思井, 周金荣 2020 舰船电子工程 40 174
Google Scholar
Wan Z A, Tong Y X, Chen S J, Zhou J R 2020 Ship Electron. Eng. 40 174
Google Scholar
[6] Epstein P S, Carhart R R 1953 J. Acoust. Soc. Am. 25 553
Google Scholar
[7] Allegra J R, Hawley S A 1972 J. Acoust. Soc. Am. 51 1545
Google Scholar
[8] Mcclements D J, Hemar Y, Herrmann N 1999 J. Acoust. Soc. Am. 105 915
Google Scholar
[9] Mcclements D J, Coupland J N 1996 Colloids Surf., A 117 161
Google Scholar
[10] Mcclements D J 1992 J. Acoust. Soc. Am. 91 849
Google Scholar
[11] Parker N G, Povey M J W 2012 Food Hydrocolloids Oxford 26 99
Google Scholar
[12] Liu L 2009 Chem. Eng. Sci. 64 5036
Google Scholar
[13] Dong L L, Su M X, Xue M H, Cai X S, Shang Z T 2007 AIP Conf. Proc. 914 654
Google Scholar
[14] Wang Q, Attenborough K, Woodhead S 2000 J. Sound Vib. 236 781
Google Scholar
[15] Wang Q, Attenborough K, Woodhead S 2001 Proc. Inst. Mech. Eng. Part E 215 133
Google Scholar
[16] 杜娜, 苏明旭 2019 应用声学 38 980
Google Scholar
Du N, Su M X 2019 J. Appl. Acoust. 38 980
Google Scholar
[17] 侯森, 胡长青, 赵梅 2021 70 044301
Google Scholar
Hou S, Hu C Q, Zhao M 2021 Acta Phys. Sin. 70 044301
Google Scholar
[18] 陈时, 张迪, 王成会, 张引红 2019 68 074301
Google Scholar
Chen S, Zhang D, Wang C H, Zhang Y H 2019 Acta Phys. Sin. 68 074301
Google Scholar
[19] 郭盼盼, 苏明旭, 陈丽, 蔡小舒 2014 过程工程学报 14 562
Guo P P, Su M X, Chen L, Cai X S 2014 Chin. J. Process Eng. 14 562
[20] 李运思, 苏明旭, 杨荟楠, 凡凤仙, 蔡小舒 2017 声学学报 42 586
Google Scholar
Li Y S, Su M X, Yang H N, Fan F X, Cai X S 2017 Acta. Acust. 42 586
Google Scholar
[21] Huang B F, Fan F X, Li Y S, Su M X 2019 Ultrason. 94 218
Google Scholar
[22] 冷坤, 章曦, 武文远, 龚艳春, 杨云涛 2018 物理与工程 28 74
Google Scholar
Leng K, Zhang X, Wu W Y, Gong Y C, Yang Y T 2018 Phys. Eng. 28 74
Google Scholar
[23] 王敏, 申玉清, 陈震宇, 徐鹏 2021 计算物理 38 623
Google Scholar
Wang M, Shen Y Q, Chen Z Y, Xu P 2021 Chin. J. Comput. Phys. 38 623
Google Scholar
[24] Hay A E, Mercer D G 1985 J. Acoust. Soc. Am. 78 1761
Google Scholar
[25] Faran J J 1951 J. Acoust. Soc. Am. 23 405
Google Scholar
[26] 冯若 1999 超声手册 (南京: 南京大学出版社) 第66页
Feng R 1999 Ultrasonic Handbook (Nanjing: Nanjing University Press) p66 (in Chinese)
[27] Wang M, Zheng D, Dong J, Hu J 2021 IEEE Trans. Instrum. Meas. 70 1
Google Scholar
下载:
计量
- 文章访问数: 4105
- PDF下载量: 64
- 被引次数: 0
