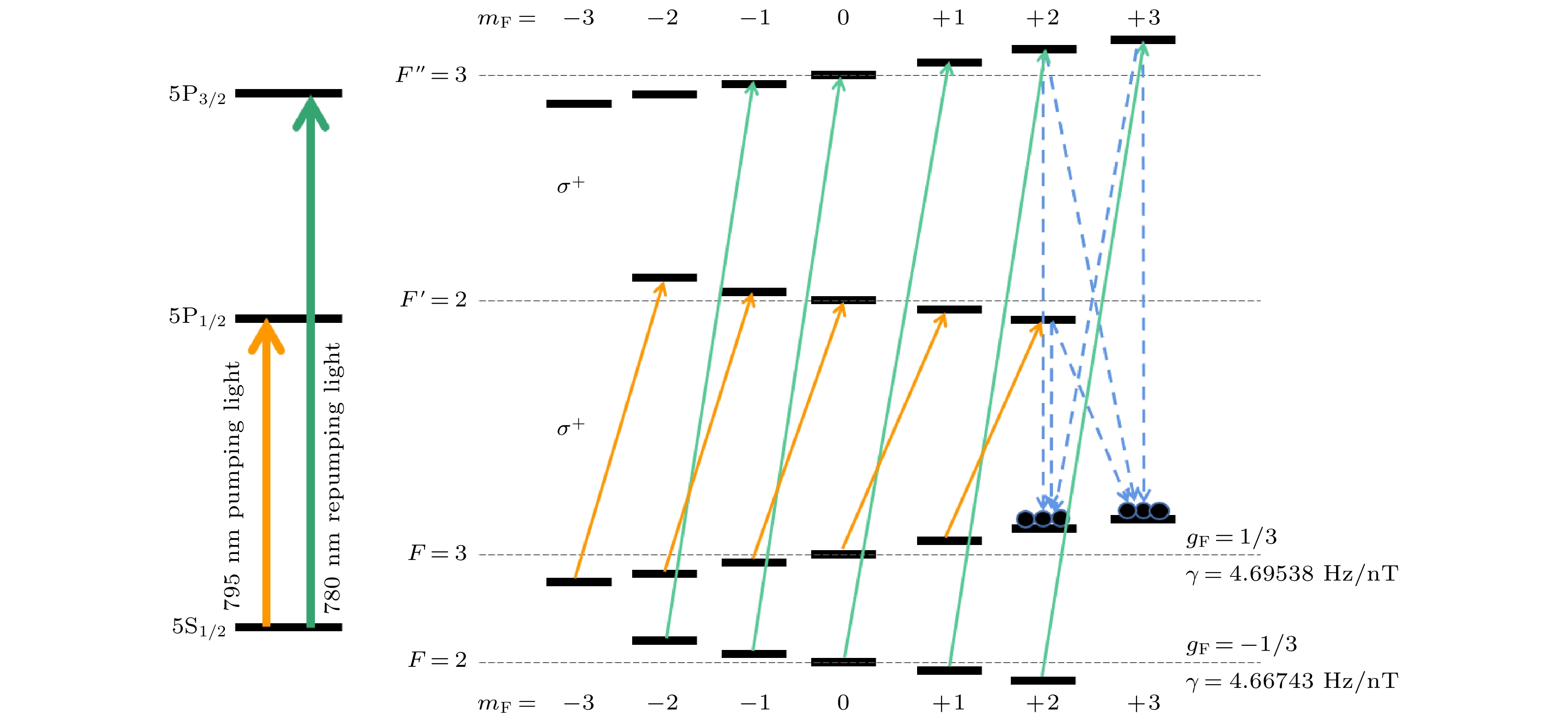
-
在光泵原子磁强计的实验装置中, 窄线宽及高信噪比的磁共振信号是实现高灵敏度磁强计的充要条件. 本文的实验中利用795 nm波长窄线宽单频连续极化光(同时也是探测光)对比研究了不同类型铷原子气室、不同温度下典型的磁共振信号, 在镀石蜡的铷原子气室中获得最优化的磁共振信号. 通过引入铷原子D2线780 nm波长窄线宽单频连续反抽运光, 研究了激光功率对磁共振信号信噪比和线宽的影响. 实验表明, 780 nm波长窄线宽单频连续反抽运光的引入使得铷-85原子磁共振信号的信号幅值有明显提高并且线宽没有明显展宽. 引入780 nm波长窄线宽单频连续反抽运光后, 闭环锁定的铷-85原子磁强计在约1.2 kHz频率带宽范围内灵敏度约为26.4 pT/Hz1/2, 相比仅有795 nm波长窄线宽单频连续极化光(同时也是探测光)存在时提高了近1个数量级. 同时本实验利用增强后的铷原子磁共振信号对一种商用的磁通门磁强计在弱磁场测量时的准确度和偏差进行了校准.For the experimental implementation of an optically pumped atomic magnetometer, the magnetic resonance signal with a narrow linewidth and a high signal-to-noise ratio (SNR) is required for achieving a high sensitivity. Using 795-nm laser as both the pumping and the probe laser, we compare the magnetic resonance signals from different rubidium atomic vapor cells and investigate the variations of magnetic resonance signals with temperature. Optimized magnetic resonance signal is achieved with a paraffin-coated rubidium atomic vapor cell. Then the 780-nm laser at rubidium D2 line is introduced as a repumping laser, and we explore the changes of linewidth and SNR of the magnetic resonance signal under different power of the pumping laser and the repumping laser. Owing to the 780-nm repumping laser beam, the signal amplitude of rubidium-85 magnetic resonance signal is improved remarkably because more rubidium-85 atoms are spin- polarized by the 795-nm pumping laser beam. At the same time, the linewidth of rubidium-85 magnetic resonance signal is roughly not broadened anymore. We realize a closed-loop optically pumped rubidium-85 atomic magnetometer with a bandwidth of ~1.2 kHz, and the sensitivity is calibrated to be ~245.5 pT/Hz1/2 only with the 795-nm pumping laser beam. Owing to the employment of the 780-nm repumping laser beam, the sensitivity is improved to be ~26.4 pT/Hz1/2 which is improved roughly by one order of magnitude. We also calibrate the measurement accuracy and deviation of a commercial fluxgate magnetometer by using the enhanced rubidium magnetic resonance signal.
-
Keywords:
- optically pumped rubidium atomic magnetometer /
- linewidth of magnetic resonance signal /
- signal-to-noise ratio /
- repumping light /
- fluxgate magnetometer’s calibration
[1] Labyt E, Corsi M C, Fourcault W, Laloy A P, Bertrand F, Lenouvel F, Cauffet G, Prado L P, Berger F, Morales S 2019 IEEE Trans. Medical Imaging 38 90
Google Scholar
[2] Tralshawala N, Claycomb J R, Miller J H 1997 Appl. Phys. Lett. 71 1573
Google Scholar
[3] Bonavolonta C, Valentino M, Peluso G, Barone A 2007 IEEE Trans. Appl. Superconductivity 17 772
Google Scholar
[4] Sarma B S P, Verma B K, Satyanarayana S V 1999 Geophysics 64 1735
Google Scholar
[5] Smith E J, Dougherty M K, Russell C T, Southwood D J 2001 J. Geophys. Research: Space Phys. 106 30129
Google Scholar
[6] Savukov I M, Seltzer S J, Romalis M V 2005 Phys. Rev. Lett. 95 063004
Google Scholar
[7] Shah V, Knappe S, Schwindt P D D, Kitching J 2007 Nature Photonics 1 649
Google Scholar
[8] Boto E, Holmes N, Leggett J, Roberts G, Shah V, Meyer S S, Munoz L D, Mullinger K J, Tierney T M, Barnes G R, Bowtell R, Brookes M J 2018 Nature 555 657
Google Scholar
[9] Dang H B, Maloof A C, Romalis M V 2010 Appl. Phys. Lett. 97 151110
Google Scholar
[10] Ledbetter M P, Budker D 2013 Phys. Today 66 44
Google Scholar
[11] Valentina T, Kamal 2013 The Sci. World J. 2013 1
Google Scholar
[12] Zhao Q, Fan B L, Wang S G, Wang L J 2019 J. Magnetism & Magnetic Materials 481 257
Google Scholar
[13] Budker D, Romalis M 2007 Nature Phys. 3 227
Google Scholar
[14] Budker D, Kimball D F J 2013 Optical Magnetometry (New York: Cambridge University Press) p60
[15] 汪之国, 罗晖, 樊振方, 谢元平 2016 65 210702
Google Scholar
Wang Z G, Luo H, Fan Z F, Xie Y P 2016 Acta Phys. Sin. 65 210702
Google Scholar
[16] 李曙光, 周翔, 曹晓超, 盛继腾, 徐云飞, 王兆英, 林强 2010 59 877
Google Scholar
Li S G, Zhou X, Cao X C, Sheng J T, Xu Y F, Wang Z Y, Lin Q 2010 Acta Phys. Sin. 59 877
Google Scholar
[17] Dong H F, Fang J C, Zhou B Q, Tang X B, Qin J 2012 Eur. Phys. J. Appl. Phys. 57 21004
Google Scholar
[18] Zhang R, Xiao W, Ding Y D, Feng Y L, Peng X, Shen L, Sun C, W T, Wu Y L, Yang Y C, Zheng Z Y, Zhang X Z, Chen J B, Guo H 2020 Sci. Adv. 6 eaba8792
Google Scholar
[19] 孙伟民, 刘双强, 赵文辉, 张军海 2015 光学原子磁力仪 (哈尔滨: 哈尔滨工程大学出版社) 第42页
Sun W M, Liu S Q, Zhao W H, Zhang J H 2015 Optical Atomic Magnetometer (Harbin: Harbin Engineering University Press) p42 (in Chinese)
[20] 顾源, 石荣晔, 王延辉 2014 63 110701
Google Scholar
Gu Y, Shi R Y, Wang Y H 2014 Acta Phys. Sin. 63 110701
Google Scholar
[21] Yang H, Wang Y, Zhao N 2020 Eur. Phys. J. D 74 225
Google Scholar
[22] Bloch F 1946 Phys. Rev. 70 460
Google Scholar
[23] Seltzer S J 2008 Ph. D. Dissertation (Princeton: Princeton University)
[24] Keaveney J 2013 Ph. D. Dissertation (Durham: Durham University)
[25] Wang Z, Peng X, Zhang R, Luo H, Guo H 2020 Phys. Rev. Lett. 124 193002
Google Scholar
-
图 1 铷-85原子的相关能级图及有关的跃迁
Fig. 1. Relevant energy levels of rubidium-85 atoms and the related transitions.
图 2 实验装置图 ECDL, 外腔半导体激光器; DBR, 分布布拉格反射激光器; APP, 整形棱镜对; ISO, 光隔离器; λ/2, 1/2波片; PBS, 偏振分光棱镜; HR, 高反镜; NDF, 衰减片; DM1, 双色镜(780 nm高透, 795 nm高反); DM2, 双色镜(795 nm高透, 780 nm高反); LB, 挡光板; BE, 扩束望远镜; PD, 光电探测器; Dump, 遮光器; Lock-in, 锁相放大器; PID, 比例积分微分放大器; SAS, 饱和吸收光谱装置; PS, 偏振光谱装置; FFT, 快速傅里叶变换动态信号分析仪
Fig. 2. Schematic diagram of the experimental setup. ECDL, external-cavity diode laser; DBR, distributed-Bragg-reflector type diode laser; APP, anamorphic prism pairs; ISO, optical isolator; λ/2, half-wave plate; PBS, polarization beam splitter cube; HR, high-reflectivity mirror; NDF, neutral density filter; DM1, two-color mirror (high-transmittance@780-nm, high-reflection@795-nm); DM2, two-color mirror (high-transmittance@795-nm, high-reflection@780-nm); LB, light barrier; BE, the beam expanding telescope; PD, photodetector; Dump, laser dump; Lock-in, lock-in amplifier; PID, the proportional-integrational-differential amplifier; SAS, the saturation absorption spectroscopic device; PS, the polarization spectroscopic device; FFT, the Fast-Fourier-Transformation dynamic signal analyzer.
图 3 不同原子气室下的磁共振信号 温度40 ℃, 铷-85 (F = 3)对应的旋磁比为4.69538 Hz/nT, 轴向直流偏置磁场~8.87 μT, 795 nm波长窄线宽单频连续极化光(同时也是探测光)功率~200 μW, 光斑高斯直径~7.3 mm, 频率共振于铷-85原子
$ (F\;=\;3) $ −$(F′\;=\;2)$ 超精细跃迁. 图(a)为充有20 Torr He和10 Torr Ne的自然丰度铷原子气室, 铷-85原子对应的磁共振信号半高全宽~6.1 kHz; 图(b)为不缓冲气体、气室内壁镀石蜡的自然丰度铷原子气室, 磁共振信号半高全宽~3.3 kHzFig. 3. Magnetic resonance signal at different rubidium vapor cells at temperature 40 ℃. The magnetogyric ratio of the 85Rb (F = 3) is 4.69538 Hz/nT, the static magnetic field is ~8.87 μT, the 795-nm pumping laser beam’s power is ~200 μW, the Gaussian diameter is ~7.3 mm and the frequency is locked to the 85Rb
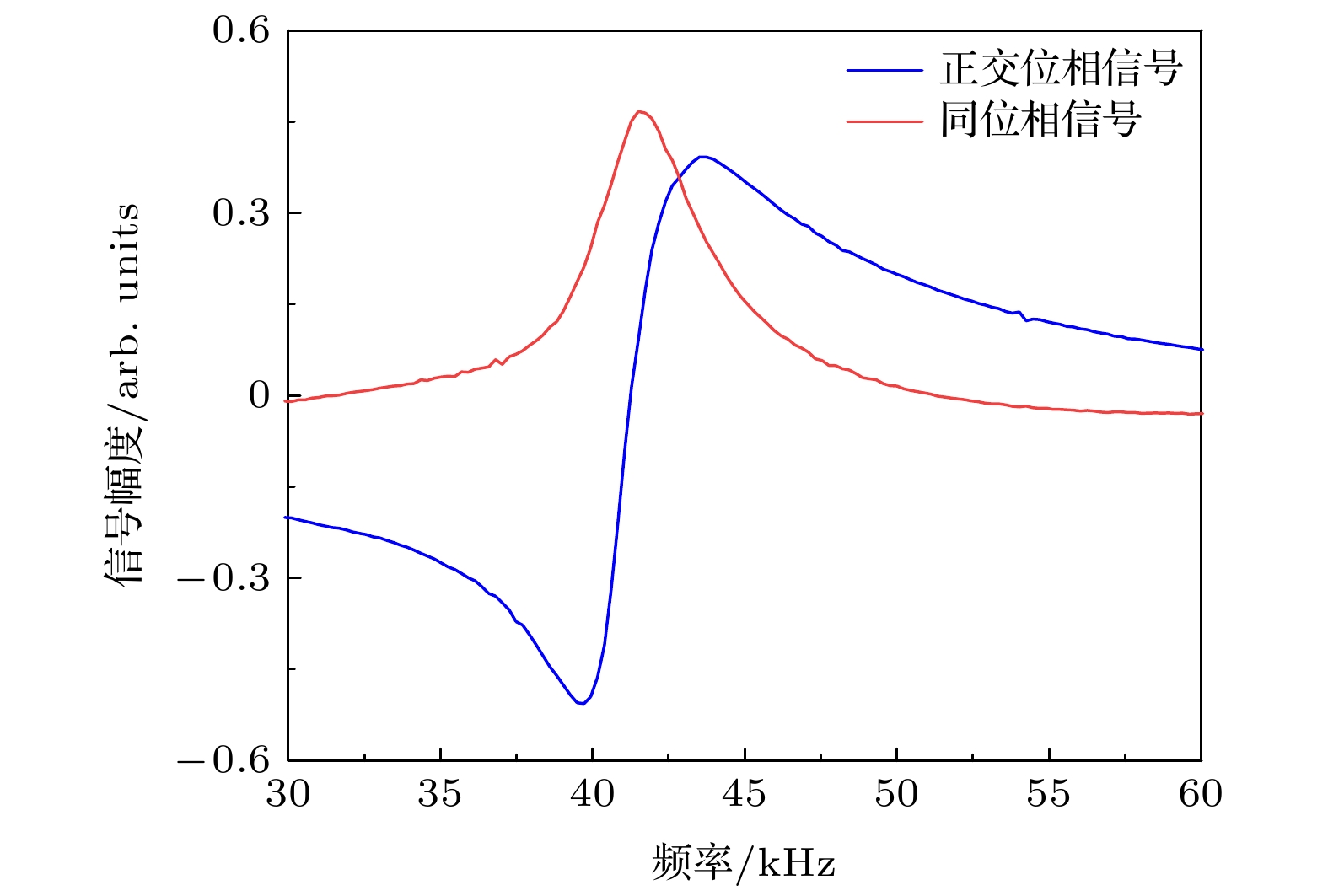
$ (F\;=\;3) $ −$(F′\;=\;2)$ transition. Fig.3(a) shows the 85Rb + 87Rb vapor cell filled with 20 Torr of Helium (He) and 10 Torr of Neon (Ne) as the buffer gases, the magnetic resonance signal’s line width(FWHM) is ~6.1 kHz. Fig.3 (b) shows the 85Rb + 87Rb vapor cell with paraffin-coating (without the buffer gas), the magnetic resonance signal’s linewidth (FWHM) is ~3.3 kHz.
图 4 不同温度(27−45 ℃)下的磁共振信号 27, 35, 40, 45 ℃温度下磁共振信号的半高全宽分别为~2.2, ~2.7, ~3.3, ~4.0 kHz
Fig. 4. The magnetic resonance signals at different temperatures (27−45 ℃): The linewidth (FWHM) of the magnetic resonance signals are ~2.2 kHz@27 ℃, ~2.7 kHz@35 ℃, ~3.3 kHz@40 ℃, and 4.0 kHz@45 ℃, respectively.
图 5 锁相放大器调制解调信号, 温度45 ℃, 795 nm波长窄线宽单频连续极化光(同时也是探测光)光强200 μW, 轴向直流偏置磁场~8.87 μT, 光斑高斯直径~7.3 mm, 频率共振于铷-85原子
$ (F\;=\;3) $ −$(F′\;=\;2)$ 超精细跃迁. 解调后同位相信号的半高全宽为~4.0 kHzFig. 5. The modulation and demodulation signal of the lock-in amplifier: the red curve is the demodulated in-phase signal. The blue curve is the out-of-phase gradient after demodulation. The 795-nm pumping laser beam’s power is ~200 μW, the static magnetic field is ~8.87 μT, the Gaussian diameter is ~7.3 mm and the frequency is locked to the 85Rb
$ (F\;=\;3) $ −$(F′\;=\;2)$ transition line. The linewidth (FWHM) of the magnetic resonance signal is ~4.0 kHz.
图 6 不同780 nm波长窄线宽单频连续反抽运光和795 nm波长窄线宽单频连续极化光(同时也是探测光)功率下磁共振信号的线宽、信号幅值的变化情况
Fig. 6. The variation of linewidth (FWHM) and signal amplitude of magnetic resonance signal under different power of pumping and repumping laser beam.
图 7 有无780 nm波长窄线宽单频连续反抽运光情况下磁场灵敏度, 标定场频率63 Hz, 磁场强度4.7 nT(a)在仅有795 nm波长窄线宽单频连续极化光(同时也是探测光)(功率200 μW, 共振于铷-85原子D1线
$ (F\;=\;3) $ −$(F′\;=\;2)$ 超精细跃迁)情况下的磁场灵敏度; (b)加入780 nm波长窄线宽单频连续反抽运光(功率300 μW, 共振于铷-85原子D2线$ (F\;=\;2) $ −$(F′′\;=\;3)$ 超精细跃迁)情况下的磁场灵敏度Fig. 7. The sensitivity of the magnetometer: The calibration field frequency is 63 Hz and the magnetic field strength is 4.7 nT: (a) The magnetic field sensitivity in the presence of pumping laser beam (the frequency is resonant with
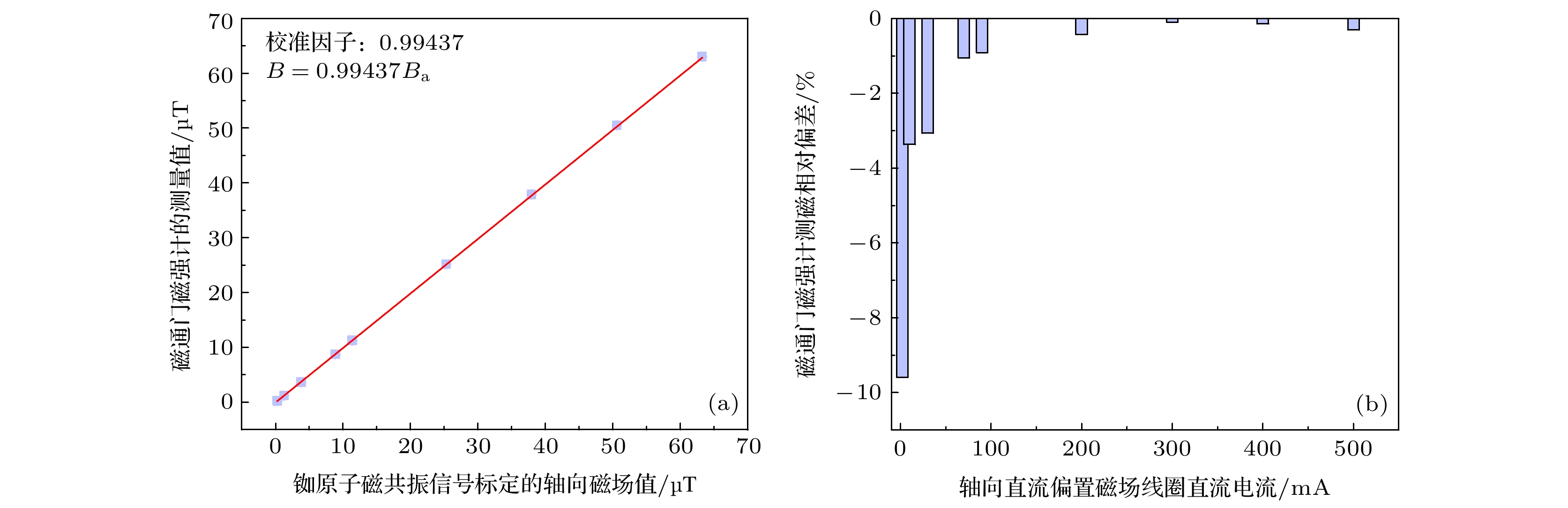
$ (F\;=\;3) $ −$(F′\;=\;2)$ hyperfine transition line of 85Rb D1 line with the power of 200 μW); (b) the magnetic field sensitivity with the addition of repumping laser beam (the frequency is resonant with$ (F\;=\;2) $ −$(F′′\;=\;3)$ hyperfine transition line of 85Rb D2 line with the power of 300 μW).
图 8 铷原子磁共振信号标定的磁场值
Fig. 8. Magnetic field values calibrated by rubidium atomic magnetic resonance signals.
图 9 磁通门磁强计测量磁场值与磁共振信号测量磁场值的关系 (a)蓝红点线为磁通门磁强计测量磁场值相对于铷原子光泵磁强计测量磁场值的对比; (b) 磁通门磁强计测量磁场值相对磁共振信号测量磁场值的相对偏差
Fig. 9. The relationship of the magnetic field measured by fluxgate magnetometer and magnetic resonance signal: (a) The blue and red dot lines are the comparison of the magnetic field measured by the fluxgate magnetometer and the rubidium atom optical pump magnetometer; (b) shows the relative deviation of the magnetic field measured by fluxhgate magnetometer compared with magnetic resonance signal.
表 1 磁通门磁强计与铷原子磁共振信号标定磁场的相对误差值
Table 1. Relative error value of magnetic field calibration between fluxgate magnetometer and rubidium atomic magnetic resonance signal.
直流偏置磁场线圈电流/mA 2 10 30 70 90 200 300 400 500 铷原子磁共振信号标定磁场的相对误差/nT ± 0.7 ± 1.2 ± 1.7 ± 2.1 ± 2.1 ± 3.1 ± 3.3 ± 3.7 ± 4.6 磁通门磁强计标定磁场的相对误差/nT ± 1 ± 2 ± 2 ± 4 ± 5 ± 6 ± 9 ± 13 ± 12 
下载: 导出CSV
-
[1] Labyt E, Corsi M C, Fourcault W, Laloy A P, Bertrand F, Lenouvel F, Cauffet G, Prado L P, Berger F, Morales S 2019 IEEE Trans. Medical Imaging 38 90
Google Scholar
[2] Tralshawala N, Claycomb J R, Miller J H 1997 Appl. Phys. Lett. 71 1573
Google Scholar
[3] Bonavolonta C, Valentino M, Peluso G, Barone A 2007 IEEE Trans. Appl. Superconductivity 17 772
Google Scholar
[4] Sarma B S P, Verma B K, Satyanarayana S V 1999 Geophysics 64 1735
Google Scholar
[5] Smith E J, Dougherty M K, Russell C T, Southwood D J 2001 J. Geophys. Research: Space Phys. 106 30129
Google Scholar
[6] Savukov I M, Seltzer S J, Romalis M V 2005 Phys. Rev. Lett. 95 063004
Google Scholar
[7] Shah V, Knappe S, Schwindt P D D, Kitching J 2007 Nature Photonics 1 649
Google Scholar
[8] Boto E, Holmes N, Leggett J, Roberts G, Shah V, Meyer S S, Munoz L D, Mullinger K J, Tierney T M, Barnes G R, Bowtell R, Brookes M J 2018 Nature 555 657
Google Scholar
[9] Dang H B, Maloof A C, Romalis M V 2010 Appl. Phys. Lett. 97 151110
Google Scholar
[10] Ledbetter M P, Budker D 2013 Phys. Today 66 44
Google Scholar
[11] Valentina T, Kamal 2013 The Sci. World J. 2013 1
Google Scholar
[12] Zhao Q, Fan B L, Wang S G, Wang L J 2019 J. Magnetism & Magnetic Materials 481 257
Google Scholar
[13] Budker D, Romalis M 2007 Nature Phys. 3 227
Google Scholar
[14] Budker D, Kimball D F J 2013 Optical Magnetometry (New York: Cambridge University Press) p60
[15] 汪之国, 罗晖, 樊振方, 谢元平 2016 65 210702
Google Scholar
Wang Z G, Luo H, Fan Z F, Xie Y P 2016 Acta Phys. Sin. 65 210702
Google Scholar
[16] 李曙光, 周翔, 曹晓超, 盛继腾, 徐云飞, 王兆英, 林强 2010 59 877
Google Scholar
Li S G, Zhou X, Cao X C, Sheng J T, Xu Y F, Wang Z Y, Lin Q 2010 Acta Phys. Sin. 59 877
Google Scholar
[17] Dong H F, Fang J C, Zhou B Q, Tang X B, Qin J 2012 Eur. Phys. J. Appl. Phys. 57 21004
Google Scholar
[18] Zhang R, Xiao W, Ding Y D, Feng Y L, Peng X, Shen L, Sun C, W T, Wu Y L, Yang Y C, Zheng Z Y, Zhang X Z, Chen J B, Guo H 2020 Sci. Adv. 6 eaba8792
Google Scholar
[19] 孙伟民, 刘双强, 赵文辉, 张军海 2015 光学原子磁力仪 (哈尔滨: 哈尔滨工程大学出版社) 第42页
Sun W M, Liu S Q, Zhao W H, Zhang J H 2015 Optical Atomic Magnetometer (Harbin: Harbin Engineering University Press) p42 (in Chinese)
[20] 顾源, 石荣晔, 王延辉 2014 63 110701
Google Scholar
Gu Y, Shi R Y, Wang Y H 2014 Acta Phys. Sin. 63 110701
Google Scholar
[21] Yang H, Wang Y, Zhao N 2020 Eur. Phys. J. D 74 225
Google Scholar
[22] Bloch F 1946 Phys. Rev. 70 460
Google Scholar
[23] Seltzer S J 2008 Ph. D. Dissertation (Princeton: Princeton University)
[24] Keaveney J 2013 Ph. D. Dissertation (Durham: Durham University)
[25] Wang Z, Peng X, Zhang R, Luo H, Guo H 2020 Phys. Rev. Lett. 124 193002
Google Scholar
下载:
计量
- 文章访问数: 6548
- PDF下载量: 200
- 被引次数: 0
