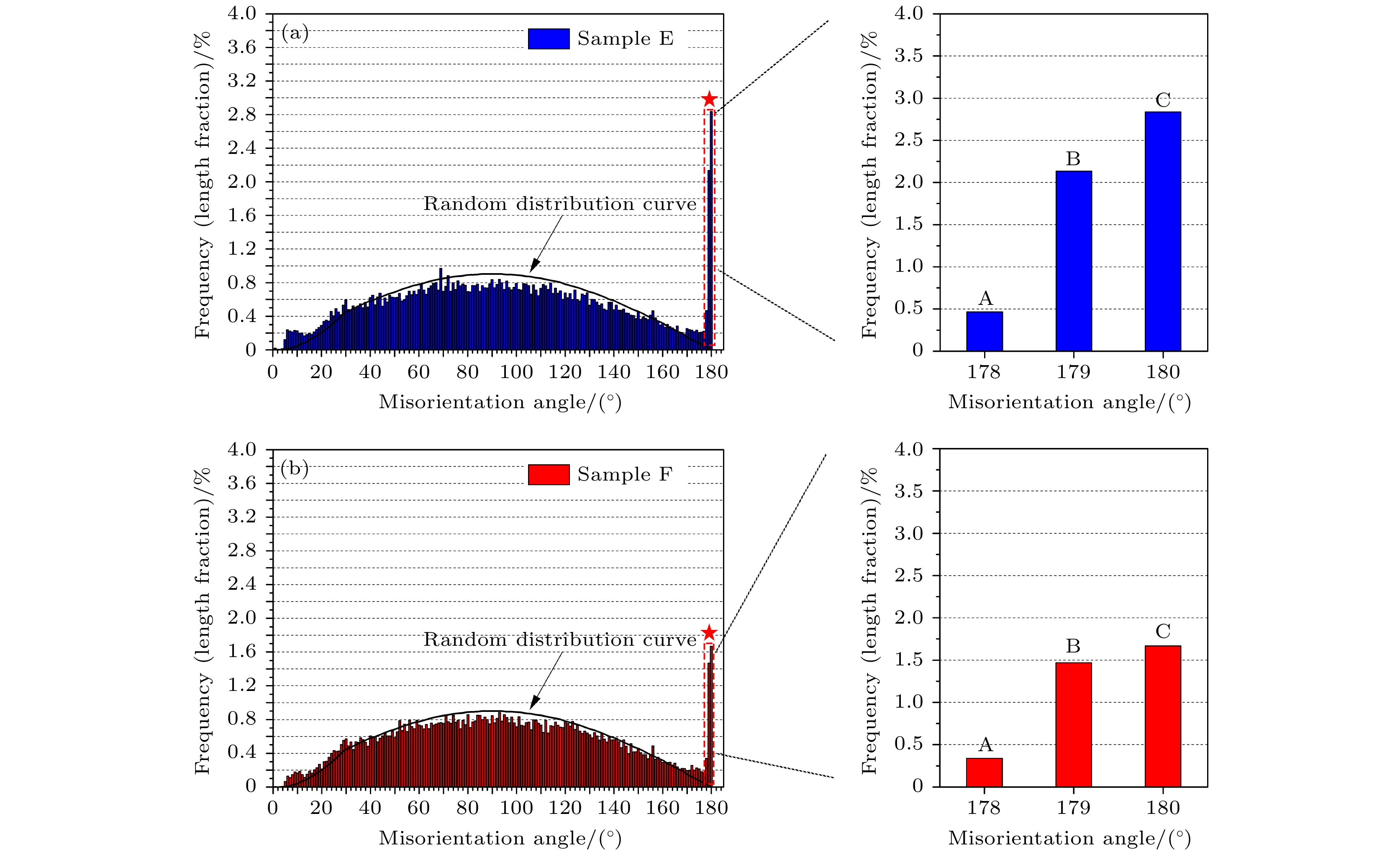
-
晶界的结构对氮化硅陶瓷材料的物理和化学性能、特别是高温力学性能有重要影响. 本文利用基于电子背散射衍射技术、体视学及统计学的五参数分析法研究了国产和国外产热等静压烧结的商用氮化硅轴承球样品的晶界特征分布. 结果表明, 两个样品的晶界取向差分布均在约180°处出现异常, 相关晶界占总晶界的比例明显高于随机分布, 其取向差主要包括[0 1 –1 0]/180°和[–1 2 –1 0]/180°, 分别对应Σ2和Σ3晶界. 两个样品中的Σ2晶界的界面匹配基本一致, 均以{0 0 0 1}/{0 0 0 1}基面/基面匹配为主, 但二者Σ3晶界的界面匹配存在很大差异, 表现为国产样品以{–1 2 –1 0}/{–1 2 –1 0}柱面匹配为主, 而国外产样品以{1 0 –1 0}/{1 0 –1 0}柱面匹配为主; 具有{–1 2 –1 0}/{–1 2 –1 0}, {0 0 0 1}/{0 0 0 1}和{1 0 –1 0}/{1 0 –1 0}三种界面匹配特征的晶界, 其面重合点密度分别为2.45 /nm2, 7.95 /nm2和9.10 /nm2, 晶界的结构有序度依次升高. 分析指出, 具有{1 0 –1 0}/{1 0 –1 0}界面匹配特征的Σ3晶界以及具有{0 0 0 1}/{0 0 0 1}界面匹配特征的Σ2晶界是氮化硅陶瓷材料中的一类特殊晶界.The structure of grain boundary has an essential influence on the physical and mechanical properties, especially the high-temperature mechanical properties of silicon nitride ceramics. In the present work, the five-parameter analysis method which is established based on electron backscatter diffraction (EBSD), and stereology and statistics are used to study the grain boundary character distributions in the two commercial silicon nitride ceramic ball samples. These two samples are both fabricated by hot isostatic pressing but made in China and abroad, respectively. The results indicate that the misorientation distributions of the two samples radically deviate from the random distribution at a rotation angle of 180°, showing that the frequency of the relevant grain boundaries is dramatically higher than that of the randomly distributed ones. These grain boundaries are mainly those possessing the misorientations of [0 1 –1 0]/180° and [–1 2 –1 0]/180°, corresponding to Σ2 and Σ3 boundaries, respectively. The grain boundary inter-connections (GBICs) of the Σ2 boundaries are basically the same in the two samples, and they are dominated mainly by the {0 0 0 1}/{0 0 0 1} basal-to-basal inter-connection. However, the GBICs of Σ3 boundaries are quite different in the two samples. they primarily the {–1 2 –1 0}/{–1 2 –1 0} prismatic-to-prismatic inter-connection for the Σ3 boundaries in the sample made in China, but it is mainly the {1 0 –1 0}/{1 0 –1 0} prismatic-to-prismatic inter-connection for the Σ3 boundary in the sample made abroad. Crystallographic analysis shows that the planar coincidence site density (PCSD) for the {–1 2 –1 0}/{–1 2 –1 0}, {0 0 0 1}/{0 0 0 1} and {1 0 –1 0}/{1 0 –1 0} GBIC are 2.45 /nm2, 7.95 /nm2 and 9.10 /nm2, respectively, implying that the degree of the structural ordering increases in turn. Further discussion emphasizes that the grain boundaries possessing {1 0 –1 0}/{1 0 –1 0} GBIC and {0 0 0 1}/{0 0 0 1} GBIC should be one type of special boundaries due to their high degrees of structural ordering.
-
Keywords:
- silicon nitride ceramic /
- electron backscatter diffraction /
- grain boundary character distribution /
- grain boundary inter-connection
[1] Krstic Z, Krstic V D 2012 J. Mater. Sci. 47 535
[2] Zhang Y, Yu X, Gu H, Yao D X, Zuo K H, Xia Y F, Yin J W, Liang H Q, Zeng Y P 2020 Ceram. Int. 47 5656
[3] Santos C, Ribeiro S, Strecker K, Rodrigues J D, Silvaet C R M 2007 J. Mater. Process. Technol. 184 108
Google Scholar
[4] Guo G F, Li J B, Yang X Z, Lin H, Liang L, He M S, Tong X G, Yang J 2007 Acta Mater. 54 2311
[5] Blugan G, Wittig D, Kuebler J 2009 Corros. Sci. 51 547
Google Scholar
[6] Fox K M, Hellmann J R 2010 Int. J. Appl. Ceram. Technol. 5 138
[7] Vieillard C 2016 Int. J. Fatigue 96 283
[8] Schilm J, Herrmann M, Michael G 2003 J. Eur. Ceram. Soc. 23 577
Google Scholar
[9] Tian X H, Zhao J, Wang Y T, Gong F, Qin W Z, Pan H L 2015 Ceram. Int. 43 3381
[10] Guo S Q, Hirosaki N, Nishimura T, Yamamoto Y, Mitomo M 2010 J. Am. Ceram. Soc. 86 1900
[11] Lu H H, Huang J L 2001 Ceram. Int. 27 621
Google Scholar
[12] Ahmad S, Ludwig T, Herrmann M, Mahmoud M M, Lippmann W, Seifert H J 2015 J. Eur. Ceram. Soc. 35 2261
Google Scholar
[13] Kmra B, Bps A 2019 J. Alloy. Compd. 779 590
Google Scholar
[14] Basu B, Vleugels J, Kalin M, Biest O V D 2003 Mater. Sci. Eng., A 359 228
Google Scholar
[15] Guan X J, Shi F, Ji H M, Li X W 2019 Mater. Sci. Eng., A 765 138299
Google Scholar
[16] Wang H, Guo K, Liu X Q, Hong C F, Wang W G, Dai P Q 2019 Mater. Charact. 149 105
Google Scholar
[17] Tokita S, Kokawa H, Kodama S, Sato Y S, Sano Y, Li Z G, Feng K, Wu Y X 2020 Mater. Today Commun. 25 101572
Google Scholar
[18] Wang W G, Fang X Y 2008 Mater. Sci. Eng., A 491 199
Google Scholar
[19] Kim C S, Hu Y, Rohrer G S, Randle V 2005 Scr. Mater. 52 633
Google Scholar
[20] Flávia D C G, Luiz M B D A, Cilene L, Leonardo S A, Jean D, Luiz H D A 2020 J. Mater. Res. Technol. 9 1801
Google Scholar
[21] Lai Y F, Zhao S L, Luo T T, Xu Q F, Liu C Y, Liu K, Li Q Z, Yang M J, Zhang S, Han M X, Goto T, Tu R 2020 Ceram. Int. 46 27000
Google Scholar
[22] Beladi H, Ghaderi A, Rohrer G S 2019 Philos. Mag. 100 1
[23] Wang W G, Cai C H, Rohrer G S, Gu X F, Lin Y, Chen S, Dai P Q 2018 Mater. Charact. 144 411
Google Scholar
[24] Lu X F, Gao X, Ren J Q, Li C X, Guo X, Yan X B, La P Q 2018 Comput. Mater. Sci. 151 296
Google Scholar
[25] Qiu J J, Zhang M, Liu X Y, Zhang X X, Tan Z L 2020 Mater. Sci. Eng., A 797 139985
Google Scholar
[26] Rohrer G S, El Dasher B S, Miller H M, Rollett A D, Saylor D M 2004 Mater. Res. Soc. Symp. Proc. 819 265
[27] Wang W G, Chen S, Rohrer G S, Chen W Z 2017 Scr. Mater. 128 18
Google Scholar
[28] Wang W G, Cui Y K, Rohrer G S, Cai C H, Chen S, Gu X F, Lin Y 2019 Scr. Mater. 170 62
Google Scholar
[29] Jeyaraam R, Vedantam S, Sarma V S 2019 Mater. Charact. 152 276
Google Scholar
[30] Fang X Y, Wang W G, Cai Z X, Qin C X, Zhou B X 2010 Mater. Sci. Eng., A 527 1571
Google Scholar
[31] Pellan M, Lay S, Missiaen J M, Norgren S, Angseryd J, Coronel E, Persson T 2017 Powder Metall. 60 208
Google Scholar
[32] Li B, Li G Q, Chen J H, Chen H Y, Xing X M, Hou X M, Li Y 2018 Ceram. Int. 44 9395
Google Scholar
-
图 1 电子背散射衍射(EBSD)全欧拉角去噪前(a)和(b)及去噪后(c)和(d)显微组织 (a), (c)样品E; (b), (d) 样品F
Fig. 1. All Euler microstructures before (a) and (b) and after (c) and (d) noise reduction, obtained by electron backscatter diffraction (EBSD): (a), (c) Sample E; (b), (d) sample F.
图 2 经50 ℃氢氟酸(HF)浸泡240 min后样品E(a)和F(b)的二次电子显微组织(SEM)
Fig. 2. The secondary electron microscopy (SEM) of sample E (a) and F (b) after soaking in hydrofluoric acid at 50 ℃ for 240 min.
图 3 取向成像显微图(a)和(b)及Si3N4/ Si3N4晶界网络(c)和(d) (a), (c) 样品E; (b)和(d) 样品F
Fig. 3. Orientation imaging microscopy (OIM) (a) and (b), and Si3N4/Si3N4 grain boundary networks (c) and (d): (a), (c) Sample E; (b), (d) sample F.
图 4 φ2 = 40°截面的取向分布函数图 (a) 样品E; (b) 样品F
Fig. 4. φ2 = 40° section of orientation distribution function (ODF): (a) Sample E; (b) sample F.
图 5 Si3N4/Si3N4晶界取向差分布图 (a) 样品E; (b) 样品F
Fig. 5. Misorientation distributions of Si3N4/Si3N4 grain boundaries of sample E (a) and F (b).
图 6 Si3N4/ Si3N4晶界随旋转轴分布 (a) 样品E; (b) 样品F
Fig. 6. The frequencies of the gain boundaries with varied rotation axis in sample E (a) and F(b).
图 7 以[0 1 –1 0]((a)和(c))及[–1 2 –1 0] ((b)和(d))为旋转轴的Si3N4/Si3N4晶界取向差分布 (a), (b) 样品E; (c), (d) 样品F
Fig. 7. Misorientation distributions of Si3N4/Si3N4 grain boundaries possessing rotation axis [0 1 –1 0] (a) and (c) and [–1 2 –1 0] (b) and (d): (a), (b) Sample E; (c), (d) sample F.
图 8 两Si3N4晶粒呈[0 1 –1 0]/180°(Σ2)取向差关系对应的重合原子示意图 (a) 2个等价N1原子重合图; (b) 6个等价N2原子重合图; (c) 6个等价Si原子重合图. 其中蓝色圆圈和红色圆圈分别代表旋转前后的原子, 绿色圆点代表重合原子. 每个示意图均包含为50个Si3N4晶胞
Fig. 8. Schematic illustrations for the coincident atoms when two Si3N4 crystals have a misorientation of [0 1 –1 0]/180°(Σ2): (a) Two identical N1 atoms; (b) 6 identical N2 atoms; (c) 6 identical Si atoms. Blue and red circles stand for the atoms before and after rotation, respectively. Green dots represent the coincident atoms. Each plot contains 50 unit cells of Si3N4.
图 9 两Si3N4晶粒呈[–1 2 –1 0]/180°(Σ3)取向差关系对应的重合原子示意图 (a) 2个等价N1原子重合图; (b) 6个等价N2原子重合图; (c) 6个等价Si原子重合图. 其中蓝色圆圈和红色圆圈分别代表旋转前后的原子, 绿色圆点代表重合原子. 每个示意图均包含为50个Si3N4晶胞
Fig. 9. Schematic illustrations for the coincident atoms when two Si3N4 crystals have a misorientation of [–1 2 –1 0]/180°(Σ3): (a) Two identical N1 atoms; (b) 6 identical N2 atoms; (c) 6 identical Si atoms. Blue and red circles stand for the atoms before and after rotation, respectively. Green dots represent the coincident atoms. Each plot contains 50 unit cells of Si3N4.
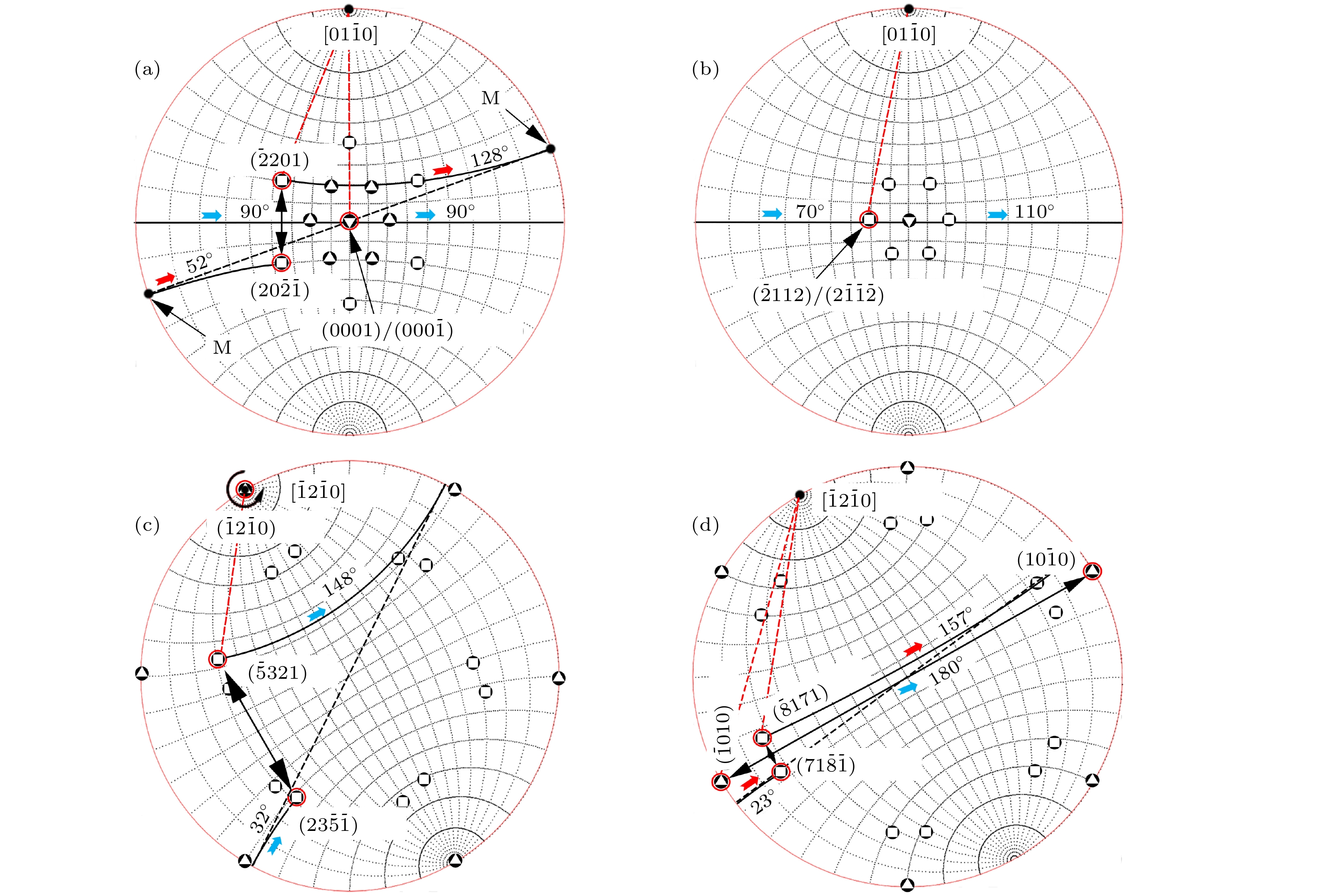
图 10 样品E((a)和(c))和F((b)和(d))[0 1 –1 0]/180°((a)和(b))以及[–1 2 –1 0]/180°((c)和(d))晶界的晶界面分布FPA图(投影在(0 0 0 1)内) (a)和(b)中的
, 
和 
为分布强点等同位置; (c)和(d)中的 和 分别为分布强点和次强点等同位置Fig. 10. (0 0 0 1) projections of grain boundary plane distributions of [0 1 –1 0]/180°((a) and (b))and [–1 2 –1 0]/180°((c) and (d)) grain boundaries in sample E ((a) and (c)) and sample F ((b) and(d)).
, and in Figure (a) and (b) stand for the identical positions of the strongest distribution, while and in Figure (c) and (d) represent the identical positions of the strongest and the second strongest distribution, respectively.
图 11 样品E((a)和(b))和F((c)和(d))各自的Si3N4/ Si3N4全部晶界的晶界面分布FPA图((a)和(c))及其分布强点和次强点位置示意图((b)和(d))(投影在(0 0 0 1)内).
, 为分布最强点等同位置, 为分布次强点等同位置Fig. 11. (0 0 0 1) projections of grain boundary plane distributions of all grain boundaries insample E ((a) and (b)) and F ((c) and (d)).
and stand for the identical positions of the strongest distributions, while represents the identical positions of the second strongest distribution.
图 12 对应于图10的样品E((a)和(c))和F((b)和(d))[0 1 –1 0]/180°((a)和(b))以及[–1 2 –1 0]/180°((c)和(d))晶界的晶界面分布示意图(投影在(0 0 0 1)内)
Fig. 12. Schematic illustrations of grain boundary plane distributions of [0 1 –1 0]/180° ((a) and (b)) and [–1 2 –1 0]/180° ((c) and (d)) grain boundaries in sample E ((a) and (c)) and F ((b) and (d)).
表 1 样品E和F中[0 1 –1 0]/180°和[–1 2 –1 0]/180°晶界的GBIC及对应的PCSD值
Table 1. The GBICs and PCSD values of [0 1 –1 0]/180° and [–1 2 –1 0]/180° grain boundaries observed in sample E and F
Sample E Sample F Misorientation
(u v t w/θ)GBICs PCSD
/(/nm2)Misorientation
(u v t w/θ)GBICs PCSD
/(/nm2)[0 1 –1 0]/180° {–2 2 0 1}/{–2 2 0 1} 1.49 [0 1 –1 0]/180° {–2 1 1 2}/{–2 1 1 2} 3.5 (Σ2) {–2 1 1 2}/{–2 1 1 2} 3.5 (Σ2) {0 0 0 1}/{0 0 0 1} 7.95 {0 0 0 1}/{0 0 0 1} 7.95 [–1 2 –1 0]/180° {–5 3 2 1}/{2 3 –5 –1} 0.93 [–1 2 –1 0]/180° {–8 1 7 1}/{7 1 –8 –1} 1.63 (Σ3) {–1 2 –1 0}/{–1 2 –1 0} 2.45 (Σ3) {1 0 –1 0}/{1 0 –1 0} 9.1 
下载: 导出CSV
-
[1] Krstic Z, Krstic V D 2012 J. Mater. Sci. 47 535
[2] Zhang Y, Yu X, Gu H, Yao D X, Zuo K H, Xia Y F, Yin J W, Liang H Q, Zeng Y P 2020 Ceram. Int. 47 5656
[3] Santos C, Ribeiro S, Strecker K, Rodrigues J D, Silvaet C R M 2007 J. Mater. Process. Technol. 184 108
Google Scholar
[4] Guo G F, Li J B, Yang X Z, Lin H, Liang L, He M S, Tong X G, Yang J 2007 Acta Mater. 54 2311
[5] Blugan G, Wittig D, Kuebler J 2009 Corros. Sci. 51 547
Google Scholar
[6] Fox K M, Hellmann J R 2010 Int. J. Appl. Ceram. Technol. 5 138
[7] Vieillard C 2016 Int. J. Fatigue 96 283
[8] Schilm J, Herrmann M, Michael G 2003 J. Eur. Ceram. Soc. 23 577
Google Scholar
[9] Tian X H, Zhao J, Wang Y T, Gong F, Qin W Z, Pan H L 2015 Ceram. Int. 43 3381
[10] Guo S Q, Hirosaki N, Nishimura T, Yamamoto Y, Mitomo M 2010 J. Am. Ceram. Soc. 86 1900
[11] Lu H H, Huang J L 2001 Ceram. Int. 27 621
Google Scholar
[12] Ahmad S, Ludwig T, Herrmann M, Mahmoud M M, Lippmann W, Seifert H J 2015 J. Eur. Ceram. Soc. 35 2261
Google Scholar
[13] Kmra B, Bps A 2019 J. Alloy. Compd. 779 590
Google Scholar
[14] Basu B, Vleugels J, Kalin M, Biest O V D 2003 Mater. Sci. Eng., A 359 228
Google Scholar
[15] Guan X J, Shi F, Ji H M, Li X W 2019 Mater. Sci. Eng., A 765 138299
Google Scholar
[16] Wang H, Guo K, Liu X Q, Hong C F, Wang W G, Dai P Q 2019 Mater. Charact. 149 105
Google Scholar
[17] Tokita S, Kokawa H, Kodama S, Sato Y S, Sano Y, Li Z G, Feng K, Wu Y X 2020 Mater. Today Commun. 25 101572
Google Scholar
[18] Wang W G, Fang X Y 2008 Mater. Sci. Eng., A 491 199
Google Scholar
[19] Kim C S, Hu Y, Rohrer G S, Randle V 2005 Scr. Mater. 52 633
Google Scholar
[20] Flávia D C G, Luiz M B D A, Cilene L, Leonardo S A, Jean D, Luiz H D A 2020 J. Mater. Res. Technol. 9 1801
Google Scholar
[21] Lai Y F, Zhao S L, Luo T T, Xu Q F, Liu C Y, Liu K, Li Q Z, Yang M J, Zhang S, Han M X, Goto T, Tu R 2020 Ceram. Int. 46 27000
Google Scholar
[22] Beladi H, Ghaderi A, Rohrer G S 2019 Philos. Mag. 100 1
[23] Wang W G, Cai C H, Rohrer G S, Gu X F, Lin Y, Chen S, Dai P Q 2018 Mater. Charact. 144 411
Google Scholar
[24] Lu X F, Gao X, Ren J Q, Li C X, Guo X, Yan X B, La P Q 2018 Comput. Mater. Sci. 151 296
Google Scholar
[25] Qiu J J, Zhang M, Liu X Y, Zhang X X, Tan Z L 2020 Mater. Sci. Eng., A 797 139985
Google Scholar
[26] Rohrer G S, El Dasher B S, Miller H M, Rollett A D, Saylor D M 2004 Mater. Res. Soc. Symp. Proc. 819 265
[27] Wang W G, Chen S, Rohrer G S, Chen W Z 2017 Scr. Mater. 128 18
Google Scholar
[28] Wang W G, Cui Y K, Rohrer G S, Cai C H, Chen S, Gu X F, Lin Y 2019 Scr. Mater. 170 62
Google Scholar
[29] Jeyaraam R, Vedantam S, Sarma V S 2019 Mater. Charact. 152 276
Google Scholar
[30] Fang X Y, Wang W G, Cai Z X, Qin C X, Zhou B X 2010 Mater. Sci. Eng., A 527 1571
Google Scholar
[31] Pellan M, Lay S, Missiaen J M, Norgren S, Angseryd J, Coronel E, Persson T 2017 Powder Metall. 60 208
Google Scholar
[32] Li B, Li G Q, Chen J H, Chen H Y, Xing X M, Hou X M, Li Y 2018 Ceram. Int. 44 9395
Google Scholar
下载:
计量
- 文章访问数: 5831
- PDF下载量: 137
- 被引次数: 0
