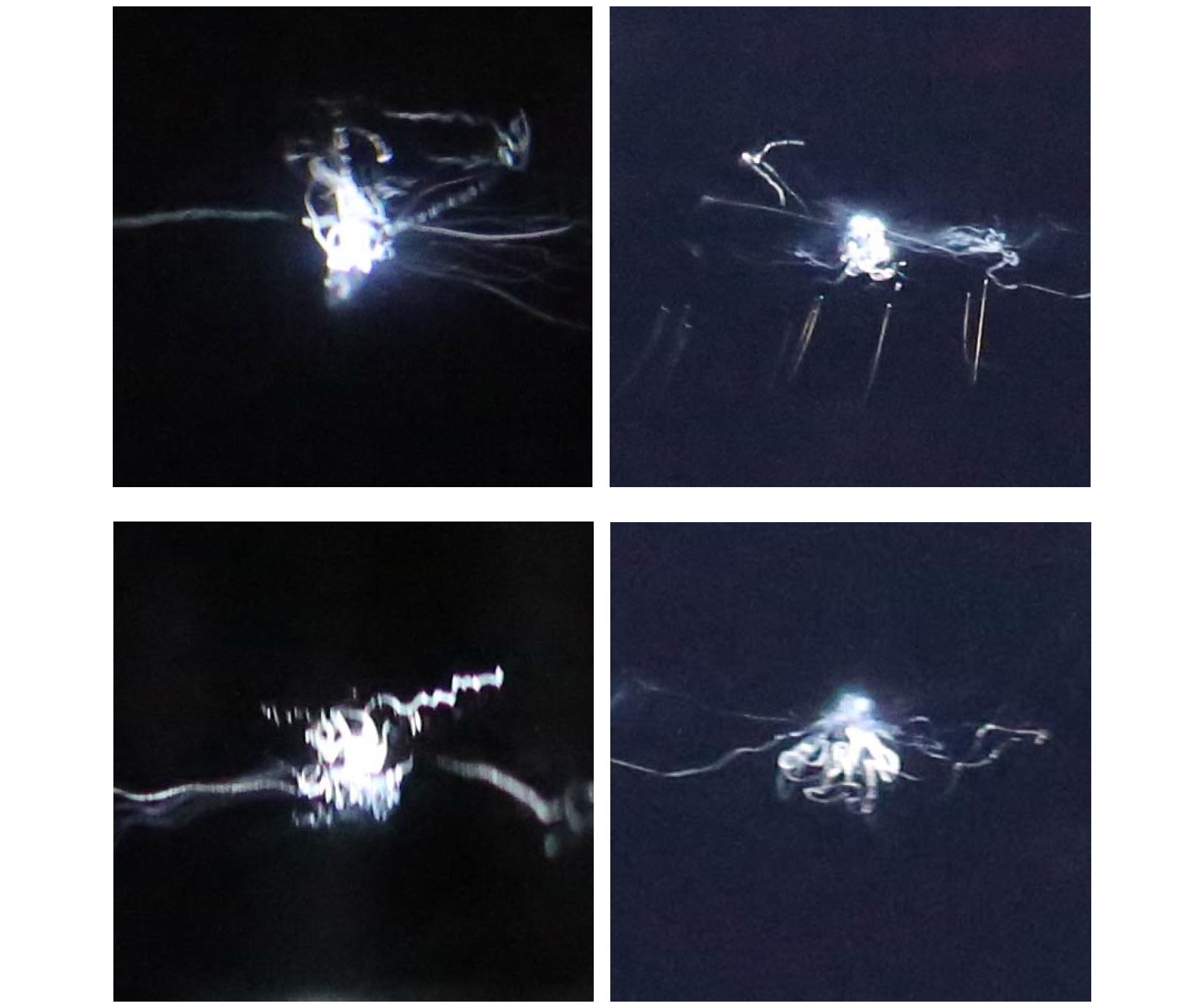
-
声空化实验中经常观察到由许多空化气泡组成的各种泡群结构. 本文利用气泡群及群内任一气泡的 Rayleigh-Plesset 方程并结合van der Waals型过程方程, 研究了不同类型气泡组成的混合泡群中的气泡半径、泡内温度和群内压力脉冲变化规律, 得到以下结果: 相同尺寸相同气体气泡和相同尺寸不同气体气泡组成的两种泡群中气泡所含的不同气体对泡内温度有较明显的影响, 但对气泡半径变化和群内压力脉冲峰值的影响较小; 不同尺寸相同气体气泡和不同尺寸不同气体气泡组成的两种混合泡群中, 随着大气泡数的增多, 大小气泡内温度开始快速下降, 之后大泡内温度逐渐趋近于纯大气泡组成泡群的泡内温度, 小泡内温度逐渐趋近于许多大泡辐射作用下的单一小气泡泡内温度; 气泡崩溃时产生的压力脉冲峰值, 先急剧减小到拐点, 之后平稳增加并逐渐趋近于纯氩气大气泡和纯氦气大气泡组成泡群内的压力脉冲峰值; 群内大气泡数占比对泡群空化特性有重要影响, 只有大气泡数占比达到一定值后泡群中才能出现不同尺寸气泡同时崩溃的现象.In this paper, we observe and record the phenomenon of multi-bubble sonoluminescence in phosphoric acid solution which helium is injected into. It is found that a large number of cavitation bubbles are produced in the solution, which can form bubble groups with different shapes and structures, among them the spherical bubble group is a typical one. By the Rayleigh-Plesset equation of bubble group and any bubble in the group with the combination of van der Waals process equation, we study the variations of the bubble radius, temperature of the bubble, pressure pulse in the groups which are composed, respectively, of the bubbles with the same size and the same gas, the bubbles with the same size and different gases, the bubbles with different sizes and the same gas and the bubbles with different sizes and different gases. The results show that for the bubble group composed of the bubbles with the same size and the same gas and the bubble group composed of the bubbles with the same size and different gases, the gas contained in the bubble has a significant effect on the temperature inside the bubble, but has little effect on the bubble radius and the pressure pulse peak within the cluster. Bubble collapse depth of gas with low molecular weight is deeper than that of gas with high molecular weight, but the radius change of rebound stage is smaller than that of the latter. For the bubble group composed of the bubbles with different sizes and the same gas and the bubble group composed of the bubbles with different sizes and different gases, when the total number of bubbles in a bubble group is constant, for the case where there is only one large bubble in the group, the temperature in the large bubble is the highest, which can be higher than the temperature in a single bubble with the same size and the same gas; with the increase of the number of large bubbles in the group, the temperatures in the large and small bubbles both decrease rapidly: the temperature in the large bubbles approaches to the temperature in the bubbles of the bubble group composed of large bubbles with the same gas and the same size, and the temperature in the small bubble gradually approaches to the temperature in small bubble with the same gas under the radiation of many large bubbles. With the increase of the number of large bubbles, the peak value of the pressure pulse in the bubble group first decreases sharply to the inflection point, and then increases steadily to the peak value of the pressure pulse in the bubble group composed of large bubbles with the same gas and the same size. The proportion of large bubbles number in the bubble group has an important influence on the cavitation characteristics of the bubble group, only when the proportion of large bubbles reaches a certain value, can the bubbles of different sizes in the bubble group collapse at the same time, and this conclusion improve and perfect the previous conclusion. The results of this paper will provide some theoretical guidance and help to further explore the cavitation effect and luminescent mechanism of the bubble group.
-
Keywords:
- spherical bubble group /
- different types of bubbles /
- expansion and collapse /
- bubble temperature /
- pressure in bubble group
[1] Yasui K, Iida Y, Tuziuti T, Kozuka T, Towata A 2008 Phys. Rev. E 77 016609
Google Scholar
[2] Doinikov A A, Zavtrak S T 1998 J. Acoust. Soc. Am. 99 3849
[3] Mettin R, Luther S, Ohl C D 1999 Ultrason. Sonochem. 6 25
Google Scholar
[4] An Y 2011 Phys. Rev. E 83 066313
Google Scholar
[5] Nasibullaeva E S, Akhatov I S 2005 Acoust. Phys. 51 705
Google Scholar
[6] 沈壮志, 吴胜举 2012 61 244301
Google Scholar
Shen Z Z, Wu S J 2012 Acta Phys. Sin. 61 244301
Google Scholar
[7] 王成会, 莫润阳, 胡静, 陈时 2015 64 234301
Google Scholar
Wang C H, Mo R Y, Hu J, Chen S 2015 Acta Phys. Sin. 64 234301
Google Scholar
[8] 张鹏利, 林书玉, 朱华泽, 张涛 2019 68 134301
Google Scholar
Zhang P L, Lin S Y, Zhu H Z, Zhang T 2019 Acta Phys. Sin. 68 134301
Google Scholar
[9] 苗博雅, 安宇 2015 64 204301
Google Scholar
Miao B Y, An Y 2015 Acta Phys. Sin. 64 204301
Google Scholar
[10] Zhang Y Y, Chen W Z, Zhang L L, Wang X, Chen Z 2019 Chin. Phys. Lett. 36 034301
Google Scholar
[11] 清河美, 那仁满都拉 2019 68 234302
Google Scholar
Qinghim, Naranmandula 2019 Acta Phys. Sin. 68 234302
Google Scholar
[12] Shen Z Z 2020 Chin. Phys. B 29 014304
Google Scholar
[13] Sugita N, Ando K, Sugiura T 2017 Ultrason. 77 160
Google Scholar
[14] Zhang L L, Chen W Z, Zhang Y Y, Wu Y R, Wang X, Zhao G Y 2020 Chin. Phys. B 29 034303
Google Scholar
[15] Liang J F, An Y, Chen W Z 2019 Chin. Phys. Lett. 36 107801
Google Scholar
[16] Nasibullaeva E S, Akhatov I S 2013 J. Acoust. Soc. Am. 133 3727
Google Scholar
[17] Yasui K 2001 Phys. Rev. E 63 035301
Google Scholar
[18] Storey B D, Szeri A J 2002 Phys. Rev. Lett. 88 074301
Google Scholar
[19] Lohse D, Breener M P, Dupont T F, Hilgenfeldt S, Johnston B 1997 Phys. Rev. Lett. 78 1359
Google Scholar
[20] 陈伟中 2014 声空化物理 (北京: 科学出版社) 第297—298 页
Chen W Z 2014 Sound Cavitation Physics (Beijing: Science Press) pp297–298 (in Chinese)
[21] Hilgenfeldt S, Grossmann S, Lohse D 1999 Phys. Fluids 11 1318
Google Scholar
[22] Moshaii A, Faraji M, Tajik-Nezhad S 2010 Ultrason. Sonochem. 18 1148
Google Scholar
[23] Faraji M, Moshaii A 2012 Phys. Lett. A 376 2703
Google Scholar
[24] Didenko Y T, McNamara III W B, Suslick K S 2000 Phys. Rev. Lett. 84 777
Google Scholar
[25] Xu H, Suslick K S 2010 Phys. Rev. Lett. 104 244301
Google Scholar
[26] Cairós C, Mettin R 2017 Phys. Rev. Lett. 118 064301
Google Scholar
[27] Ida M, Naoe T, Futakawa M 2007 Phys. Rev. E 76 046309
Google Scholar
-
图 1 实验观察到的注入氦气磷酸溶液中的泡群结构
Fig. 1. Experimental observation of bubble structure in injected helium phosphoric acid solution.
图 2 相同尺寸相同气体气泡组成的四种泡群的声空化特性 (a) 气泡半径变化; (b) 气泡径向速度变化; (c) 气泡崩溃及回弹阶段的半径放大图; (d) 泡群半径变化; (e) 泡群径向速度变化; (f) 气泡内温度变化; (g) 泡群内压力变化
Fig. 2. Acoustic cavitation characteristics of four kinds of bubble groups composed of same size and same gas bubbles: (a) Change of bubbles radius; (b) change of bubbles radial velocity; (c) magnified figure of bubbles radius at the stage of collapse and rebound; (d) change of bubble group radius; (e) change of bubble groups radial velocity; (f) change of temperature in bubbles; (g) change of pressure in bubble groups.
图 3 相同尺寸不同气体气泡组成的两种混合泡群的声空化特性 (a) 气泡半径变化; (b) 气泡径向速度变化; (c) 气泡崩溃及回弹阶段的半径放大图; (d) 泡群半径变化; (e) 泡群径向速度变化; (f) 气泡内温度变化; (g) 泡群内压力变化
Fig. 3. Acoustic cavitation characteristics of two kinds of mixed bubble groups composed of same size and different gas bubbles: (a) Change of bubbles radius; (b) change of bubbles radial velocity; (c) magnified figure of bubbles radius at the stage of collapse and rebound; (d) change of bubble group radius; (e) change of bubble groups radial velocity; (f) change of temperature in bubbles; (g) change of pressure in bubble groups.
图 4 不同尺寸相同气体气泡组成的两种混合泡群的声空化特性 (a) 大泡内最高温度随大气泡数的变化; (b) 小泡内最高温度随大气泡数的变化; (c) 泡群内压力脉冲峰值随大气泡数的变化
Fig. 4. Acoustic cavitation characteristics of two kinds of mixed bubble groups composed of different size and same gas bubbles: (a) The change of the maximum temperature in the large bubble with the number of large bubbles; (b) the change of the maximum temperature in the small bubble with the number of large bubbles; (c) the change of the peak of pressure pulse with the number of large bubbles in a bubble groups.
图 5 氦气泡群中, 大(红实线)、小(黑虚线)气泡的半径变化及对应的群内压力脉冲变化 (a1), (a2)
$ N_{1} = 1 $ ; (b1), (b2)$ N_{1} = 24 $ ; (c1), (c2)$ N_{1} = 100 $ ; (d1), (d2)$ N_{1} = 400 $ ; (e1), (e2)$ N_{1} = 800 $ Fig. 5. Radius change of large (red solid line) and small (black dotted line) bubbles in helium bubble group and corresponding pressure pulse change in the group: (a1), (a2)
$ N_{1} = 1 $ ; (b1), (b2)$ N_{1} = 24 $ ; (c1), (c2)$ N_{1} = 100 $ ; (d1), (d2)$ N_{1} = 400 $ ; (e1), (e2)$ N_{1} = 800 $ 
图 6 不同尺寸不同气体气泡组成的两种混合泡群的声空化特性 (a) 大泡内最高温度随大气泡数的变化; (b) 小泡内最高温度随大气泡数的变化; (c) 泡群内压力脉冲峰值随大气泡数的变化
Fig. 6. Acoustic cavitation characteristics of two kinds of mixed bubble groups composed of different size and different gas bubbles: (a) The change of the maximum temperature in the large bubble with the number of large bubbles; (b) the change of the maximum temperature in the small bubble with the number of large bubbles; (c) the change of the peak of pressure pulse with the number of large bubbles in a bubble groups.
相关参数 值 液体密度 $\rho$/kg·m–3 1575 表面张力 $\sigma$/N·m–1 0.07987 黏滞系数 $\eta$/Pa·s 0.05111 环境压强 $P_{0}$/atm 1 声速 $c$/m·s–1 1671 环境温度 $T_{0}$/K 273.15 蒸气压 $P_{\rm v}$/Pa 763 
下载: 导出CSV
-
[1] Yasui K, Iida Y, Tuziuti T, Kozuka T, Towata A 2008 Phys. Rev. E 77 016609
Google Scholar
[2] Doinikov A A, Zavtrak S T 1998 J. Acoust. Soc. Am. 99 3849
[3] Mettin R, Luther S, Ohl C D 1999 Ultrason. Sonochem. 6 25
Google Scholar
[4] An Y 2011 Phys. Rev. E 83 066313
Google Scholar
[5] Nasibullaeva E S, Akhatov I S 2005 Acoust. Phys. 51 705
Google Scholar
[6] 沈壮志, 吴胜举 2012 61 244301
Google Scholar
Shen Z Z, Wu S J 2012 Acta Phys. Sin. 61 244301
Google Scholar
[7] 王成会, 莫润阳, 胡静, 陈时 2015 64 234301
Google Scholar
Wang C H, Mo R Y, Hu J, Chen S 2015 Acta Phys. Sin. 64 234301
Google Scholar
[8] 张鹏利, 林书玉, 朱华泽, 张涛 2019 68 134301
Google Scholar
Zhang P L, Lin S Y, Zhu H Z, Zhang T 2019 Acta Phys. Sin. 68 134301
Google Scholar
[9] 苗博雅, 安宇 2015 64 204301
Google Scholar
Miao B Y, An Y 2015 Acta Phys. Sin. 64 204301
Google Scholar
[10] Zhang Y Y, Chen W Z, Zhang L L, Wang X, Chen Z 2019 Chin. Phys. Lett. 36 034301
Google Scholar
[11] 清河美, 那仁满都拉 2019 68 234302
Google Scholar
Qinghim, Naranmandula 2019 Acta Phys. Sin. 68 234302
Google Scholar
[12] Shen Z Z 2020 Chin. Phys. B 29 014304
Google Scholar
[13] Sugita N, Ando K, Sugiura T 2017 Ultrason. 77 160
Google Scholar
[14] Zhang L L, Chen W Z, Zhang Y Y, Wu Y R, Wang X, Zhao G Y 2020 Chin. Phys. B 29 034303
Google Scholar
[15] Liang J F, An Y, Chen W Z 2019 Chin. Phys. Lett. 36 107801
Google Scholar
[16] Nasibullaeva E S, Akhatov I S 2013 J. Acoust. Soc. Am. 133 3727
Google Scholar
[17] Yasui K 2001 Phys. Rev. E 63 035301
Google Scholar
[18] Storey B D, Szeri A J 2002 Phys. Rev. Lett. 88 074301
Google Scholar
[19] Lohse D, Breener M P, Dupont T F, Hilgenfeldt S, Johnston B 1997 Phys. Rev. Lett. 78 1359
Google Scholar
[20] 陈伟中 2014 声空化物理 (北京: 科学出版社) 第297—298 页
Chen W Z 2014 Sound Cavitation Physics (Beijing: Science Press) pp297–298 (in Chinese)
[21] Hilgenfeldt S, Grossmann S, Lohse D 1999 Phys. Fluids 11 1318
Google Scholar
[22] Moshaii A, Faraji M, Tajik-Nezhad S 2010 Ultrason. Sonochem. 18 1148
Google Scholar
[23] Faraji M, Moshaii A 2012 Phys. Lett. A 376 2703
Google Scholar
[24] Didenko Y T, McNamara III W B, Suslick K S 2000 Phys. Rev. Lett. 84 777
Google Scholar
[25] Xu H, Suslick K S 2010 Phys. Rev. Lett. 104 244301
Google Scholar
[26] Cairós C, Mettin R 2017 Phys. Rev. Lett. 118 064301
Google Scholar
[27] Ida M, Naoe T, Futakawa M 2007 Phys. Rev. E 76 046309
Google Scholar
下载:
计量
- 文章访问数: 6453
- PDF下载量: 100
- 被引次数: 0
