相干合成涡旋光束的螺旋谱分析及应用研究

彭一鸣; 薛煜; 肖光宗; 于涛; 谢文科; 夏辉; 刘爽; 陈欣; 陈芳琳; 孙学成

doi:10.7498/aps.68.20190880

摘要

应用螺旋谱分析理论, 推导了相干合成涡旋光束螺旋谱分量的位置和大小, 数值分析验证了理论推导的正确性. 基于上述谱分析理论, 可将螺旋谱分析结果作为相干合成涡旋光束质量评价函数并指导相干合成参数优化. 结果表明: 随着子光束数量和束腰半径的增加、组束环半径的减少可提高目标合成拓扑荷的模式纯度, 同时获得高质量涡旋光束. 这与采用桶中功率等传统评价函数得到的结论具有一致性.

关键词:

The vortex beam is a ring-shaped beam whose center intensity or axial intensity is zero in the propagation direction and whose phase has a spiral rising or falling gradient distribution, which is also called a dark hollow beam. Vortex beams have important applications in free-space optical communication, optical micromanipulation, quantum information processing, optical measurement, super-resolution imaging, laser processing, and material processing. In recent years, with the in-depth research on vortex beams, the application requirements for high-power vortex beams also increase. High-power and high-quality vortex beam can be obtained by coherent combining technology. However, the spiral spectrum characteristics of the vortex beam generated by coherent combining technology need further exploring. In this paper, based on the theory of spectral analysis, we derive the position and magnitude of the spiral phase spectral component of the coherent synthetic vortex beam. The numerical results verify the correctness of the theoretical derivation. Based on the above spectral analysis theory, the mode purity of the target synthesis topology charge can be used as the evaluation function to evaluate quality and optimize the parameters for the coherent synthetic vortex beam, and then to quantitatively guide the coherent synthesis of the vortex beam. The results show that with the increase of the number of sub-beams and the radius of the beam waist of the source plane, the reduction of the radius of the bundle ring and the mode purity of the target synthesis topology charge can be improved, and then we can obtain the high-quality vortex beam. This is consistent with the conclusion obtained by using traditional evaluation functions such as power in the bucket. The spiral spectrum analysis of the coherent synthetic vortex beam not only makes up for the lack of evaluation of the spiral phase synthesis effect by the traditional evaluation function, but also has certain reference significance for understanding the nature of the coherent synthesis technique.

Keywords:

作者及机构信息

1.
中南大学物理与电子学院, 长沙　410083

2.
国防科技大学前沿交叉学科学院, 长沙　410073

通信作者: 谢文科, wenkexiedan@163.com

基金项目: 装备预研领域基金(批准号: 6140415020311)、高能激光技术湖南省重点实验室开放基金(批准号: GNJGJS04)和湖南省光电惯性工程技术研究中心开放基金(批准号: HN-NUDT1908)资助的课题

Authors and contacts

1.
School of Physics and Electronics, Central South University, Changsha 410083, China

2.
College of Advanced Interdisciplinary Studies, National University of Defense Technology, Changsha 410073, China

Corresponding author: Xie Wen-Ke, wenkexiedan@163.com

Funds: Project supported by the Equipment Pre-research Field Fund, China (Grant No. 6140415020311), the Hunan Provincial Key Laboratory of High Energy Laser Technology Fund, China (Grant No. GNJGJS04), and the Hunan Engineering Research Center of Optoelectronic Inertial Technology, China (Grant No. HN-NUDT1908)

文章全文 : translate this paragraph

参考文献

[1]	Liu P S, Yang H J, Rong J, Wang G, Yan Y M 2010 Opt. Laser Technol. 42 99 Google Scholar
[2]	Wang J, Yang J Y, Fazal I M, Ahmed N, Yan Y, Huang H, Ren Y X, Yue Y, Dolinar S, Tur M, Willner A E 2012 Nat. Photonics 6 488 Google Scholar
[3]	Zhu J, Zhu K C, Tang H Q, Xia H 2017 J. Mod. Opt. 64 1915
[4]	Cheng S B, Tao S H 2016 J. Optics-Uk 18 105603 Google Scholar
[5]	Cheng S B, Tao S H, Zhou C H, Wu L 2015 J. Optics-Uk 17 105613 Google Scholar
[6]	Xiao G Z, Yang K Y, Luo H, Chen X L, Xiong W 2016 IEEE Photonics J. 8 6100108 Google Scholar
[7]	Vaziri A, Pan J W, Jennewein T, Weihs G, Zeilinger A 2003 Phys. Rev. Lett. 91 227902 Google Scholar
[8]	Lavery M P J, Speirits F C, Barnett S M, Padgett M J 2013 Science 341 537 Google Scholar
[9]	Tamburini F, Anzolin G, Umbriaco G, Bianchini A, Barbieri C 2006 Phys. Rev. Lett. 97 163903 Google Scholar
[10]	Allegre O J, Jin Y, Perrie W, Ouyang J, Fearon E, Edwardson S P, Dearden G 2013 Opt. Express 21 21198 Google Scholar
[11]	Cheng S B, Tao S H, Zhang X Y, Ma W Z 2016 IEEE Photonics J. 8 6100407 Google Scholar
[12]	Tao S H, Yu W X 2015 Opt. Express 23 1052 Google Scholar
[13]	Zhu K C, Li S X, Tang Y, Yu Y, Tang H Q 2012 J. Opt. Soc. Am. A 29 251 Google Scholar
[14]	齐晓庆, 高春清, 刘义东 2010 59 264 Google Scholar Qi X Q, Gao C Q, Liu Y D 2010 Acta Phys. Sin. 59 264 Google Scholar
[15]	Algorri J F, Urruchi V, Garcia-Camara B, Sanchez-Pena J M 2014 IEEE Electron Device Lett. 35 856 Google Scholar
[16]	Kumar A, Vaity P, Bhatt J, Singh R P 2013 J. Mod. Opt. 60 1696 Google Scholar
[17]	Brzobohaty O, Cizmar T, Zemanek P 2008 Opt. Express 16 12688 Google Scholar
[18]	朱开成, 唐慧琴, 郑小娟, 唐英 2014 63 104210 Google Scholar Zhu K C, Tang H Q, Zheng X J, Tang Y 2014 Acta Phys. Sin. 63 104210 Google Scholar
[19]	Yu T, Xia H, Fan Z H, Xie W K, Zhang P, Liu J S, Chen X, Chu X X 2019 Opt. Commun. 436 14 Google Scholar
[20]	Xie W K, Zhang P, Wang H, Chu X X 2018 Opt. Commun. 427 288 Google Scholar
[21]	Fu Y Q, Feng G Y, Zhang D Y, Chen J G, Zhou S H 2010 Optik 121 452 Google Scholar
[22]	Xiong W, Xiao G Z, Han X, Zhou J H, Chen X L, Luo H 2017 Opt. Express 25 9449 Google Scholar
[23]	Ishaaya A A, Eckhouse V, Shimshi L, Davidson N, Friesem A A 2005 Opt. Express 13 2722 Google Scholar
[24]	于涛, 夏辉, 樊志华, 谢文科, 张盼, 刘俊圣, 陈欣 2018 67 134203 Google Scholar Yu T, Xia H, Fan Z H, Xie W K, Zhang P, Liu J S, Chen X 2018 Acta Phys. Sin. 67 134203 Google Scholar

施引文献

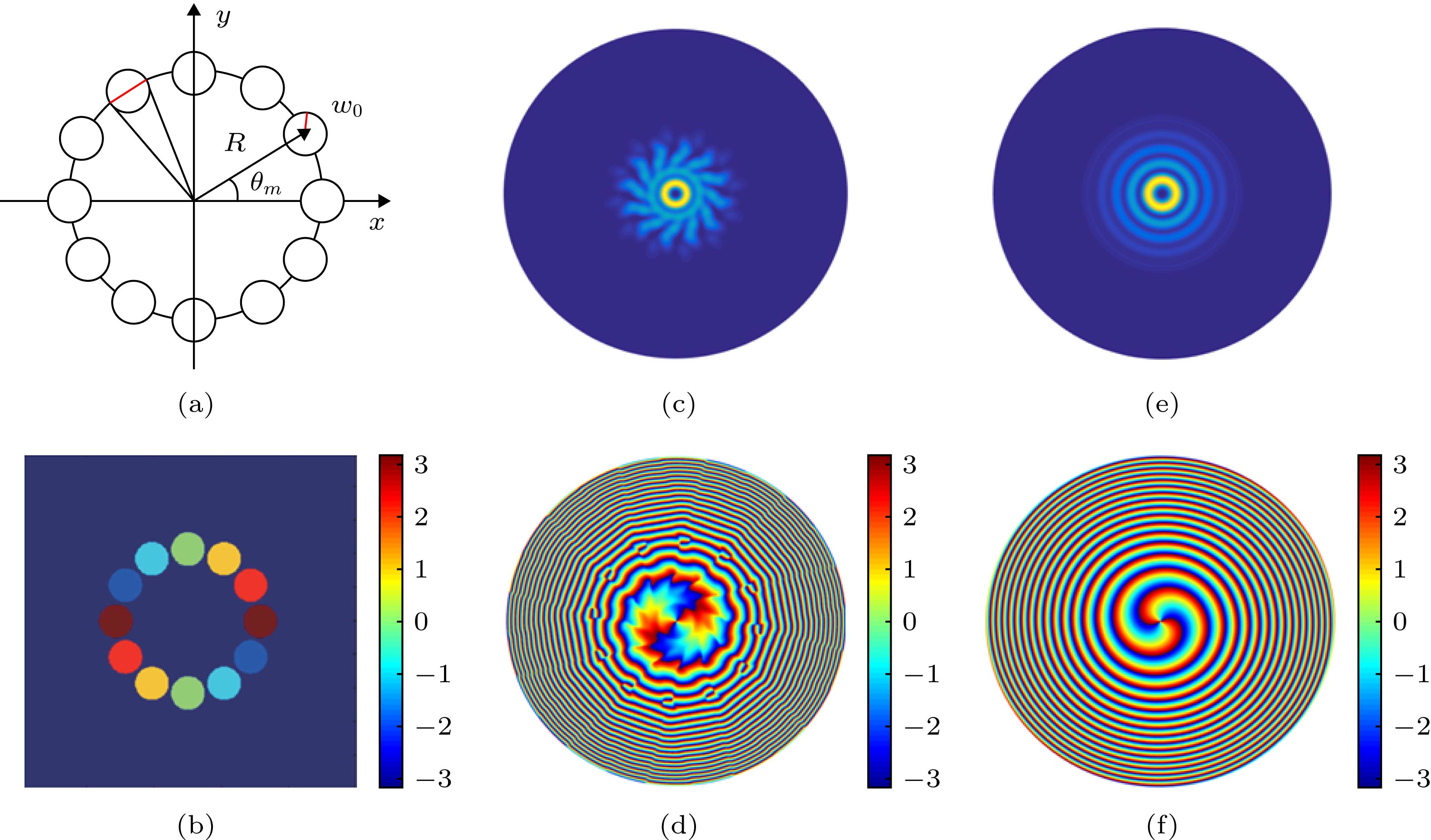
图 1 M = 12, n = 2, R = 1.2 mm, w₀ = 0.24 mm时的高斯光束阵列　(a)源平面空间分布; (b)源平面相位分布; (c)传输 2 m后合成涡旋光束强度分布; (d)传输2 m后合成涡旋光束相位分布; (e)标准2阶BG涡旋光束强度分布; (f)标准2阶BG涡旋光束相位分布

Fig. 1. Gaussian beam array with M = 12, n = 2, R = 1.2 mm, w₀ = 0.24 mm: (a) Source plane spatial distribution; (b) source plane phase distribution; (c) light field distribution of synthetic vortex beam after 2 m transmission; (d) phase distribution of synthetic vortex beam after 2 m transmission; (e) light field distribution of standard 2nd order BG vortex beam; (f) phase distribution of standard 2nd order BG vortex beam.

下载: 全尺寸图片幻灯片

图 2 z = 10 m处相干合成涡旋光束的(a)强度分布和(b)光束相位分布; 螺旋谐波重建的(c)强度分布和(d)相位分布

Fig. 2. Target plane at z = 10 m: (a) Light field distribution of coherent synthetic vortex beam; (b) phase distribution of coherent synthetic vortex beam; (c) light field distribution of spiral harmonic reconstruction light field; (d) phase distribution of spiral harmonic reconstruction light field.

下载: 全尺寸图片幻灯片

图 3 相干合成涡旋光束螺旋谱分布及大小(其中n = 1, z = 10 m, w₀ = 0.2 mm, R = 2.1 mm)　(a) M = 8; (b) M = 12; (c) M = 16

Fig. 3. Coherent synthetic vortex beam spiral spectrum distribution and size (n = 1, z = 10 m, w₀ = 0.2 mm, R = 2.1 mm): (a) M = 8; (b) M = 12; (c) M = 16.

下载: 全尺寸图片幻灯片

图 4 相干合成BG涡旋光束(n = 1, z = 10 m, w₀ = 0.2 mm, R = 2.1 mm)　(a) M = 8时强度分布; (b) M = 16时强度分布; (c) M = 8时相位分布; (d) M = 16时相位分布; (e) M = 8时螺旋谱分布; (f) M = 16时螺旋谱分布

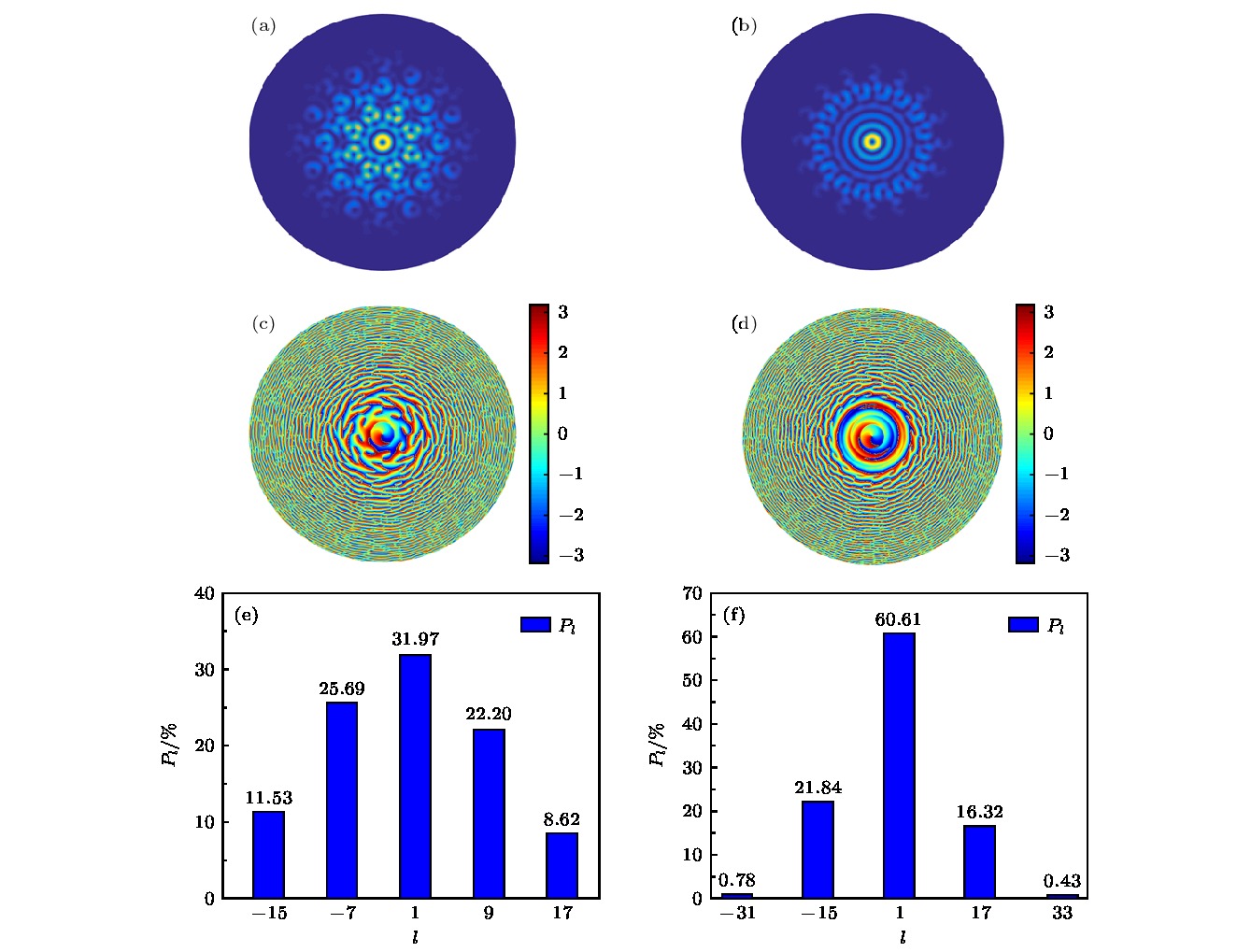
Fig. 4. Coherently synthesized BG vortex beam (n = 1, z = 10 m, w₀ = 0.2 mm, R = 2.1 mm): (a) M = 8, light intensity distribution; (b) M =16, light intensity distribution; (c) M = 8, phase distribution; (d) M = 16, phase distribution; (e) M = 8, spiral distribution; (f) M = 16, spiral distribution.

下载: 全尺寸图片幻灯片

图 5 不同阶合成涡旋光束拓扑荷模式纯度P_l随子光束数量M的变化趋势(w₀ = 0.2 mm, R = 2.1 mm, z = 10 m)

Fig. 5. Variation trend of the spectral purity P_l of the different order synthetic vortex beams with the number of sub-beams M (w₀ = 0.2 mm, R = 2.1 mm, z = 10 m).

下载: 全尺寸图片幻灯片

图 6 相干合成BG涡旋光束(n = 1, z = 10 m, M = 12, R = 2.1 mm)　(a) w₀ = 0.15 mm时强度分布; (b) w₀ = 0.3 mm时强度分布; (c) w₀ = 0.15 mm时相位分布; (d) w₀ = 0.3 mm时相位分布; (e) w₀ = 0.15 mm时螺旋谱分布; (f) w₀ = 0.3 mm时螺旋谱分布

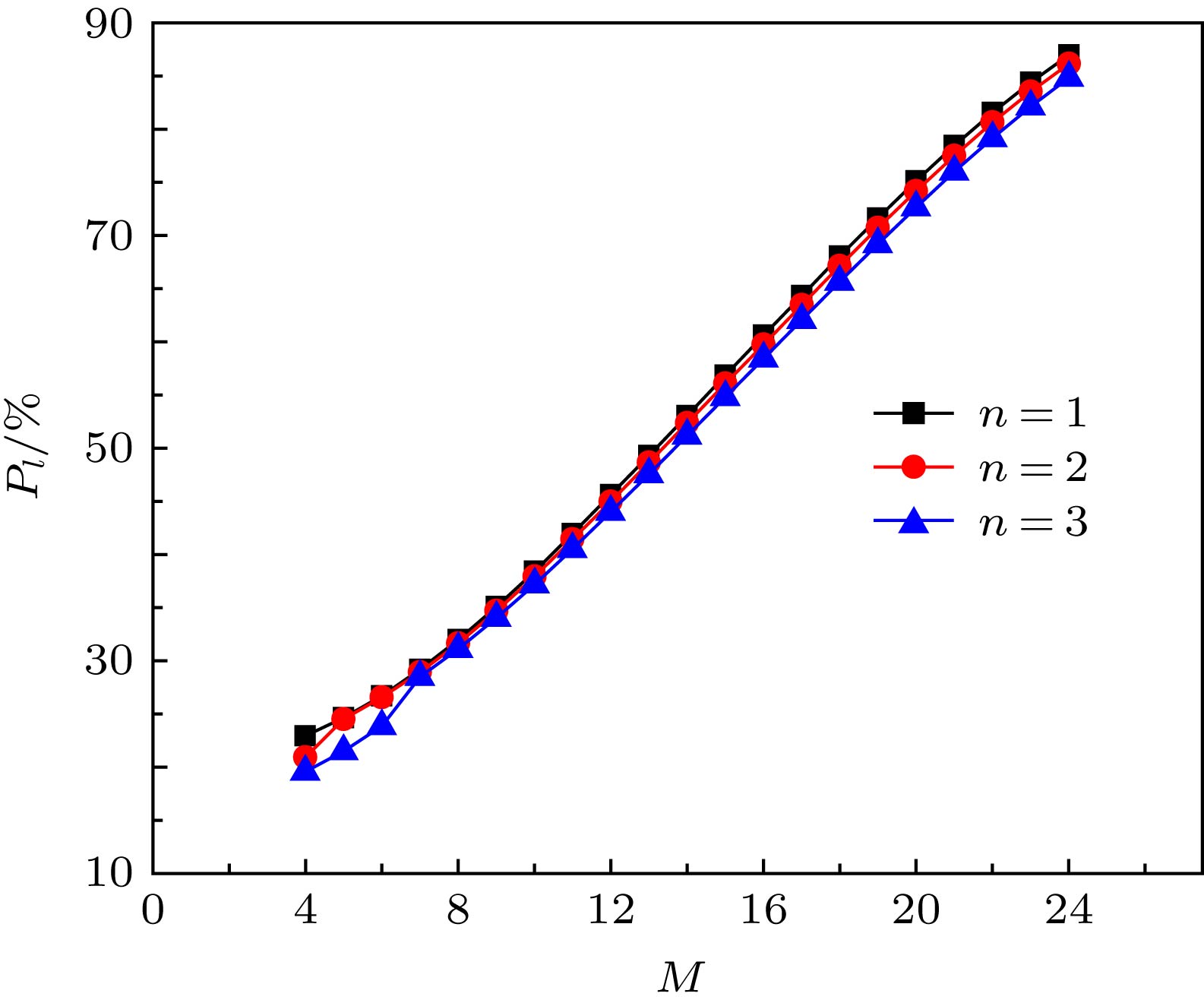
Fig. 6. Coherently synthesized BG vortex beam (n = 1, z = 10 m, M = 12, R = 2.1 mm): (a) w₀ = 0.15 mm, light intensity distribution; (b) w₀ = 0.3 mm, light intensity distribution; (c) w₀ = 0.15 mm, phase distribution; (d) w₀ = 0.3 mm, phase distribution; (e) w₀ = 0.15 mm, spiral distribution; (f) w₀ = 0.3 mm, spiral distribution.

下载: 全尺寸图片幻灯片

图 7 不同阶合成涡旋光束拓扑荷模式纯度P_l随子光束束腰半径w₀的变化(M = 12, R = 2.1 mm, z = 10 m)

Fig. 7. Variation trend of the spectral purity P_l of the different order synthetic vortex beams with sub beam waist radius w₀ (M = 12, R = 2.1 mm, z = 10 m).

下载: 全尺寸图片幻灯片

图 8 相干合成BG涡旋光束(n = 1, z = 10 m, M = 12, w₀ = 0.2 mm)　(a) R = 1 mm时强度分布; (b) R = 2.2 mm时强度分布; (c) R = 1 mm时相位分布; (d) R = 2.2 mm时相位分布; (e) R = 1 mm时螺旋谱分布; (f) R = 2.2 mm时螺旋谱分布

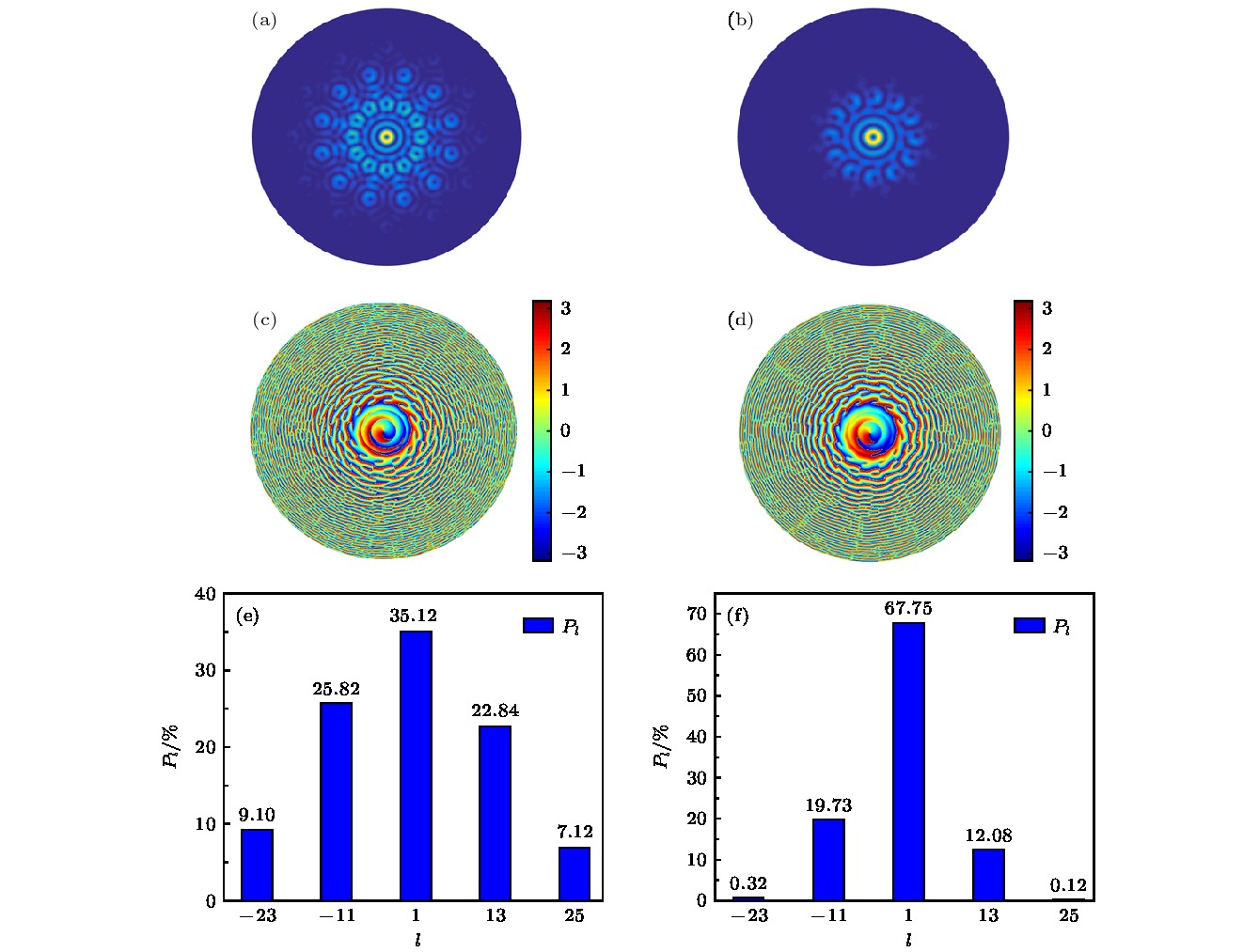
Fig. 8. Coherently synthesized BG vortex beam (n = 1, z = 10 m, M = 12, w₀ = 0.2 mm): (a) R = 1 mm, light intensity distribution; (b) R = 2.2 mm, light intensity distribution; (c) R = 1 mm, phase distribution; (d) R = 2.2 mm, phase distribution; (e) R = 1 mm, spiral distribution; (f) R = 2.2 mm, spiral distribution.

下载: 全尺寸图片幻灯片

图 9 不同阶合成涡旋光束拓扑荷模式纯度P_l随组束环半径R的变化(M = 12, w₀ = 0.2 mm, z = 10 m)

Fig. 9. Variation trend of the spectral purity P_l of the different order synthetic vortex beams with beam ring radius R (M = 12, w₀ = 0.2 mm, z = 10 m).

下载: 全尺寸图片幻灯片

map

[1]	Liu P S, Yang H J, Rong J, Wang G, Yan Y M 2010 Opt. Laser Technol. 42 99 Google Scholar
[2]	Wang J, Yang J Y, Fazal I M, Ahmed N, Yan Y, Huang H, Ren Y X, Yue Y, Dolinar S, Tur M, Willner A E 2012 Nat. Photonics 6 488 Google Scholar
[3]	Zhu J, Zhu K C, Tang H Q, Xia H 2017 J. Mod. Opt. 64 1915
[4]	Cheng S B, Tao S H 2016 J. Optics-Uk 18 105603 Google Scholar
[5]	Cheng S B, Tao S H, Zhou C H, Wu L 2015 J. Optics-Uk 17 105613 Google Scholar
[6]	Xiao G Z, Yang K Y, Luo H, Chen X L, Xiong W 2016 IEEE Photonics J. 8 6100108 Google Scholar
[7]	Vaziri A, Pan J W, Jennewein T, Weihs G, Zeilinger A 2003 Phys. Rev. Lett. 91 227902 Google Scholar
[8]	Lavery M P J, Speirits F C, Barnett S M, Padgett M J 2013 Science 341 537 Google Scholar
[9]	Tamburini F, Anzolin G, Umbriaco G, Bianchini A, Barbieri C 2006 Phys. Rev. Lett. 97 163903 Google Scholar
[10]	Allegre O J, Jin Y, Perrie W, Ouyang J, Fearon E, Edwardson S P, Dearden G 2013 Opt. Express 21 21198 Google Scholar
[11]	Cheng S B, Tao S H, Zhang X Y, Ma W Z 2016 IEEE Photonics J. 8 6100407 Google Scholar
[12]	Tao S H, Yu W X 2015 Opt. Express 23 1052 Google Scholar
[13]	Zhu K C, Li S X, Tang Y, Yu Y, Tang H Q 2012 J. Opt. Soc. Am. A 29 251 Google Scholar
[14]	齐晓庆, 高春清, 刘义东 2010 59 264 Google Scholar Qi X Q, Gao C Q, Liu Y D 2010 Acta Phys. Sin. 59 264 Google Scholar
[15]	Algorri J F, Urruchi V, Garcia-Camara B, Sanchez-Pena J M 2014 IEEE Electron Device Lett. 35 856 Google Scholar
[16]	Kumar A, Vaity P, Bhatt J, Singh R P 2013 J. Mod. Opt. 60 1696 Google Scholar
[17]	Brzobohaty O, Cizmar T, Zemanek P 2008 Opt. Express 16 12688 Google Scholar
[18]	朱开成, 唐慧琴, 郑小娟, 唐英 2014 63 104210 Google Scholar Zhu K C, Tang H Q, Zheng X J, Tang Y 2014 Acta Phys. Sin. 63 104210 Google Scholar
[19]	Yu T, Xia H, Fan Z H, Xie W K, Zhang P, Liu J S, Chen X, Chu X X 2019 Opt. Commun. 436 14 Google Scholar
[20]	Xie W K, Zhang P, Wang H, Chu X X 2018 Opt. Commun. 427 288 Google Scholar
[21]	Fu Y Q, Feng G Y, Zhang D Y, Chen J G, Zhou S H 2010 Optik 121 452 Google Scholar
[22]	Xiong W, Xiao G Z, Han X, Zhou J H, Chen X L, Luo H 2017 Opt. Express 25 9449 Google Scholar
[23]	Ishaaya A A, Eckhouse V, Shimshi L, Davidson N, Friesem A A 2005 Opt. Express 13 2722 Google Scholar
[24]	于涛, 夏辉, 樊志华, 谢文科, 张盼, 刘俊圣, 陈欣 2018 67 134203 Google Scholar Yu T, Xia H, Fan Z H, Xie W K, Zhang P, Liu J S, Chen X 2018 Acta Phys. Sin. 67 134203 Google Scholar

[1]	海迪且木⋅阿布都吾甫尔, 谭乐韬, 于涛, 谢文科, 刘静, 邵铮铮. 基于相干合成涡旋光束的离轴入射转速测量. , 2024, 73(16): 168701. doi: 10.7498/aps.73.20240655
[2]	朱雪松, 刘星雨, 张岩. 涡旋光束在双拉盖尔-高斯旋转腔中的非互易传输. , 2022, 71(15): 150701. doi: 10.7498/aps.71.20220191
[3]	钟哲强, 母杰, 王逍, 张彬. 基于紧聚焦方式的阵列光束相干合成特性分析. , 2020, 69(9): 094204. doi: 10.7498/aps.69.20200034
[4]	吴文兵, 圣宗强, 吴宏伟. 平板式螺旋相位板的设计与应用. , 2019, 68(5): 054102. doi: 10.7498/aps.68.20181677
[5]	于涛, 夏辉, 樊志华, 谢文科, 张盼, 刘俊圣, 陈欣. 贝塞尔-高斯涡旋光束相干合成研究. , 2018, 67(13): 134203. doi: 10.7498/aps.67.20180325
[6]	何杰铃, 魏凌, 杨金生, 李喜琪, 何益, 张雨东. 光瞳半径对纯位相调制激光束整形系统的影响. , 2016, 65(4): 048701. doi: 10.7498/aps.65.048701
[7]	施建珍, 杨深, 邹亚琪, 纪宪明, 印建平. 用四台阶相位板产生涡旋光束. , 2015, 64(18): 184202. doi: 10.7498/aps.64.184202
[8]	王亚东, 甘雪涛, 俱沛, 庞燕, 袁林光, 赵建林. 利用非传统螺旋相位调控高阶涡旋光束的拓扑结构. , 2015, 64(3): 034204. doi: 10.7498/aps.64.034204
[9]	王林, 袁操今, 聂守平, 李重光, 张慧力, 赵应春, 张秀英, 冯少彤. 数字全息术测定涡旋光束拓扑电荷数. , 2014, 63(24): 244202. doi: 10.7498/aps.63.244202
[10]	黄素娟, 谷婷婷, 缪庄, 贺超, 王廷云. 多环涡旋光束的实验研究. , 2014, 63(24): 244103. doi: 10.7498/aps.63.244103
[11]	谭毅, 李新阳. 光束相干合成中填充因子对远场光强分布的影响. , 2014, 63(9): 094202. doi: 10.7498/aps.63.094202
[12]	耿超, 罗文, 谭毅, 刘红梅, 牟进博, 李新阳. 基于自适应桶中功率评价函数的光纤放大器相干合成实验研究. , 2013, 62(22): 224202. doi: 10.7498/aps.62.224202
[13]	丁攀峰, 蒲继雄. 离轴拉盖尔-高斯涡旋光束传输中的光斑演变. , 2012, 61(6): 064103. doi: 10.7498/aps.61.064103
[14]	李建龙, 冯国英, 周寿桓, 李玮. 单口径相干合成系统激光光束的M2因子研究. , 2012, 61(9): 094206. doi: 10.7498/aps.61.094206
[15]	冯博, 甘雪涛, 刘圣, 赵建林. 光波场中多边位错向螺旋位错的转化. , 2011, 60(9): 094203. doi: 10.7498/aps.60.094203
[16]	丁攀峰, 蒲继雄. 拉盖尔高斯涡旋光束的传输. , 2011, 60(9): 094204. doi: 10.7498/aps.60.094204
[17]	连天虹, 王石语, 过振, 李兵斌, 蔡德芳, 文建国. 用于激光雷达的相干合成光束研究. , 2011, 60(12): 124208. doi: 10.7498/aps.60.124208
[18]	李阳月, 陈子阳, 刘辉, 蒲继雄. 涡旋光束的产生与干涉. , 2010, 59(3): 1740-1748. doi: 10.7498/aps.59.1740
[19]	韩伟涛, 侯蓝田, 耿鹏程. 双包层多芯光子晶体光纤自相干合成的数值分析与实验. , 2010, 59(10): 7091-7095. doi: 10.7498/aps.59.7091
[20]	戚巽骏, 林斌, 曹向群, 陈钰清. 基于调制传递函数的光学低通滤波器评价模型与实验研究. , 2008, 57(5): 2854-2859. doi: 10.7498/aps.57.2854

计量

文章访问数: 10769
PDF下载量: 185
被引次数: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

搜索

留言板

相干合成涡旋光束的螺旋谱分析及应用研究