-
应用基于B样条基组的相对论耦合簇理论方法, 计算了212Fr原子的nS (n = 7—12), nP (n = 7—12)和nD (n = 6—11)态的磁偶极超精细结构常数. 与精确实验值的比较说明这套理论方法能精确计算出磁偶极超精细结构常数, 其中7P态的磁偶极超精细常数的理论值与实验值之间的差异小于1%. 在忽略场移效应对Fr原子7P态超精细结构常数的影响下, 通过结合实验值进一步定出了207−213,220−228Fr核磁偶极矩
$\mu $ , 这些值与已有的测量值具有非常好的一致性. 本文报道了12S, nP (n = 9—12)和 nD (n = 10—11)态的磁偶极超精细结构常数.-
关键词:
- 磁偶极超精细结构常数 /
- B样条 /
- 耦合簇理论方法 /
- 磁偶极矩
As the heaviest atom in alkali-metal elements, Fr atom has been regarded as a candidate for the search of the permanent electric dipole moment of the electron and of parity-nonconservation effects. Accurate knowledge of Fr atomic properties is of great interest. In this work, we use a relativistic coupled-cluster method to calculate the magnetic dipole hyperfine structure constants for nS (n = 7－12), nP (n = 7－12) and nD (n = 6－11) states of 212Fr. A finite B-spline basis set is used to expand the Dirac radial function, including completely the single and double excitation in correlation calculation. Our results are compared with available theoretical and experimental values. The comparison shows that our method can offer accurate calculation of magnetic dipole hyperfine structure constant. For 7P state the differences between our results and experimental values are within 1%. The magnetic dipole hyperfine structure constants for 12S, nP (n = 9－12) and nD (n = 10－11) states are reported for the first time, which are very useful as benchmarks for experimental measurements and calculations by other theoretical methods of these quantities. In the relativistic coupled-cluster theoretical framework, we study the electron correlation effect on hyperfine-structure constant A for the S, P, and D states of Fr. We observe that the electron correlation effect is very important for hyperfine-structure constant properties. The D state has a considerable correlation effect. At the same time, we also investigate contribution trends of individual electron correlation effects involving direct, core-polarization and pair-correlation ones in S, P, and D Rydberg series. It is found that the dominant contributions for the S1/2, P1/2,3/2 and nD3/2 (n = 7－11) states are to from the direct effect; however, the dominant contributions for the 6D3/2, and nD5/2 (n = 6－11) states are due to the pair-correlation and the core-polarization, respectively. For D5/2 states, there is very strong cancellation among these individual correlation effects. The knowledge of these correlation trends is useful for studying the permanent electric dipole moment and parity-nonconservation effect of Fr in future. Moreover, the magnetic dipole moment$ {\mu}$ for each of isotopes 207−213,220−228Fr is determined by combining with experimental values for magnetic dipole hyperfine structure constant of 7P state. For each of isotope 207−213Fr, our magnetic dipole moment$ {\mu}$ is perfectly consistent with the experimental value, and our uncertainties are twice smaller than those in the experiments . For each of isotope 220−228Fr, our magnetic dipole moment$ {\mu}$ has a larger uncertainty, but is still in agreement with the experimental magnetic dipole moment$ {\mu}$ .-
Keywords:
- hyperfine-structure constant A /
- B-spline /
- coupled-cluster method /
- magnetic dipole moment
[1] Grant I P 2007 Relativistic Quantum Theory of Atoms and Molecules (New York: Springer) pp533−577
[2] Fischer C F, Brage T, Jönsson P 1997 Computational Atomic Structure: An MCHF Approach (UK: Institute of Physics) pp1−67
[3] Jönsson P, Gaigalas G, Bieroń J, Fishcher C F, Grant I 2013 Computer Physics Communications. 184 2197
Google Scholar
[4] Jönsson P, He X, Fishcher C F, Grant I 2007 Computer Physics Communications. 177 597
Google Scholar
[5] Dzuba V A, Flambaum V V, Kozlov M G 1996 Phys. Rev. A 54 3948
Google Scholar
[6] Dzuba V A, Johnson W R 1998 Phys. Rev. A 57 2459
Google Scholar
[7] Angstmann E J, Dzuba V A, Flambaum V V 2004 Phys. Rev. A 70 014102
Google Scholar
[8] Dinh T H, Dzuba V A, Flambaum V V, Ginges J S M 2008 Phys. Rev. A 78 054501
Google Scholar
[9] Kozlov M G, Porsev S G, Johnson W R 2001 Phys. Rev. A 64 052107
Google Scholar
[10] Pal R, Safronova M S, Johnson W R, Derevianko A, Porsev S G 2007 Phys. Rev. A 75 042515
Google Scholar
[11] Blundell S A, Johnson W R, Liu Z W, Sapirstein 1989 Phys. Rev. A 40 2233
Google Scholar
[12] Eliav E, Vikas M J, Ishikawa Y, Kaldor U 2005 Chem. Phys. 311 163
Google Scholar
[13] Mani B K, Angom D 2011 Phys. Rev. A 83 012501
Google Scholar
[14] Kallay M, Nataraj H S, Sahoo B K, Das B P, Visscher L 2011 Phys. Rev. A 83 030503
Google Scholar
[15] Nandy D K, Singh Y, Sahoo B K 2014 Phys. Rev. A 89 062509
Google Scholar
[16] Borschevsky A, Eliav E, Vilkas M J, Ishikawa Y, Kaldor U 2007 Phys. Rev. A 75 042514
Google Scholar
[17] Eliav E, Kaldor U, Ishikawa Y 1996 Phys. Rev. A 53 3050
Google Scholar
[18] Chaudhuri R K, Chattopadhyay S, Mahapatra U S 2013 J. Phys. Chem. A 117 12616
Google Scholar
[19] Tang Y B, Lou B Q, Shi T Y 2017 Phys. Rev. A 96 022513
Google Scholar
[20] Tang Y B, Gao N N, Lou B Q, Shi T Y 2018 Phys. Rev. A 98 062511
Google Scholar
[21] Byrnes T M R, Dzuba V A, Flambaum F F, Murray D W 1999 Phys. Rev. A 59 3082
Google Scholar
[22] Mukherjee D, Sahoo B K, Nataraj H S, Das B P 2009 J. Phys. Chem. A 113 12549
Google Scholar
[23] Sakemi Y, Harada K, Hayamizu T, Itoh M, Kawamura H, Liu S, Nataraj H S, Oikawa A, Saito M, Sato T 2011 J. Phys. Conf. Ser. 302 012051
Google Scholar
[24] Sahoo B K, Aoki T, Das B P, Sakemi Y 2016 Phys. Rev. A 93 032520
Google Scholar
[25] Atutov S N, Calabrese R, Corradi L, Dainelli A, Mauro C D, Khanbekyan A, Mariotti E, Minguzzi P, Moi L, Sanguinetti S, Stancari G, Tomassetti L 2008 Proc. SPIE 7027 70270C
[26] Ekström C, Ingelman S, Wannberg G, Skarestad M 1978 Physica Scripta 18 51
Google Scholar
[27] Coc A, Thibault C, Touchard F, Duong H T, Juncar P, Liberman S, Pinard J, Lermé J, Vialle J L, Büttgenbach S, Mueller A C, Pesnelle A, the ISOLDE Collaboration 1985 Phys. Lett. B 163 66
Google Scholar
[28] Coc A, Thibault C, Touchard F, Duong H T, Juncar P, Liberman S, Pinard J, Carre M, Lermé J, Vialle J L, Büttgenbach S, Mueller A C, Pesnelle A, the ISOLDE Collaboration 1987 Nucl. Phys. A 468 1
Google Scholar
[29] Arnold E, Borchers W, Duong H T, Juncar P, Lermé J, Lievens P, Neu W, Neugart R, Pellerin M, Pinard J, Vialle J L, Wendt K, the ISOLDE Collaboration 1990 J. Phys. B 23 3511
Google Scholar
[30] Arnold E, Borchers W, Carré M, Duong H T, Juncar P, Lermé J, Liberman S, Neu W, Neugart R, Otten W, Pellerin M, Pinard J, Pesnelle A, Vialle J L, Wendt K, the ISOLDE Collaboration 1989 J. Phys. B 22 L391
Google Scholar
[31] Bauche J, Duong H T, Juncar P, Liberman S, Pinard J, Coc A, Thibault C, Touchard F, Lermé J, Vialle J L, Büttgenbach S, Mueller A C, Pesnelle A, the ISOLDE Collaboration 1986 J. Phys. B 19 L593
Google Scholar
[32] Grossman J S, Orozco L A, Simsarian J E, Sprouse G D, Zhao W Z 1999 Phys. Rev. Lett. 83 935
Google Scholar
[33] Sansonetti J E 2007 J. Phys. Chem. Ref. Data 36 497
Google Scholar
[34] Gomez E, Aubin S, Orozco L A, Sprouse G D, Iskrenova-Tchoukova E, Safronova M S 2008 Phys. Rev. Lett. 100 172502
Google Scholar
[35] Dzuba V A, Flambaum V V, Sushkov O P 1984 J. Phys. B: At. Mol. Phys. 17 1953
Google Scholar
[36] Owusu A, Dougherty R W, Gowri G, Das T P 1997 Phys. Rev. A 56 305
Google Scholar
[37] Safronova M S, Johnson W R, Derevianko A 1999 Phys. Rev. A 60 4476
Google Scholar
[38] Sahoo B K, Nandy D K, Das B P, Sakemi Y 2015 Phys. Rev. A 91 042507
Google Scholar
[39] Duong H T, Juncar P, Liberman S, Mueller A C, Neugart R, Otten E W, Peuse B, Pinard J, Stoke H H, Thibault C, Touchard F, Vialle J L, Wendt K, the ISOLDE Collaboration 1987 Europhys. Lett. 3 175
Google Scholar
[40] Barber Z W, Stalnaker J E, Lemke N D, Poli N, Oates C W, Fortier T M, Diddams S A, Hollberg L, Hoyt C W, Taichenachev A V, Yudin V I 2008 Phys. Rev. Lett. 100 103002
Google Scholar
[41] Kien F L, Balykin V I, Hakuta K 2005 J. Phys. Soc. Jpn. 74 910
Google Scholar
[42] Ingvar L 1978 Int. J. Quantum Chem. 12 33
[43] Sinha D, Mukhopadhyay S, Mukherjee D 1986 Chem. Phys. Lett. 129 369
Google Scholar
[44] Blundell S A, Johnson W R, Sapiratein J 1991 Phys. Rev. A 43 3407
Google Scholar
[45] Porsev S G, Beloy K, Derevianko A 2010 Phys. Rev. D 82 036008
Google Scholar
[46] Sahoo B K, Sur C, Beier T, Das B P, Chaudhuri R K, Mukherjee D 2007 Phys. Rev. A 75 042504
Google Scholar
[47] Safronova M S, Safronova U I 2011 Phys. Rev. A 83 052508
Google Scholar
-
图 1 212Fr原子S1/2, P1/2, P3/2, D3/2和D5/2态磁偶极超精细结构常数中的电子关联效应
Fig. 1. Electron correlation effects in hyperfine-structure constant A for S1/2, P1/2, P3/2, D3/2 and D5/2 states of 212Fr.
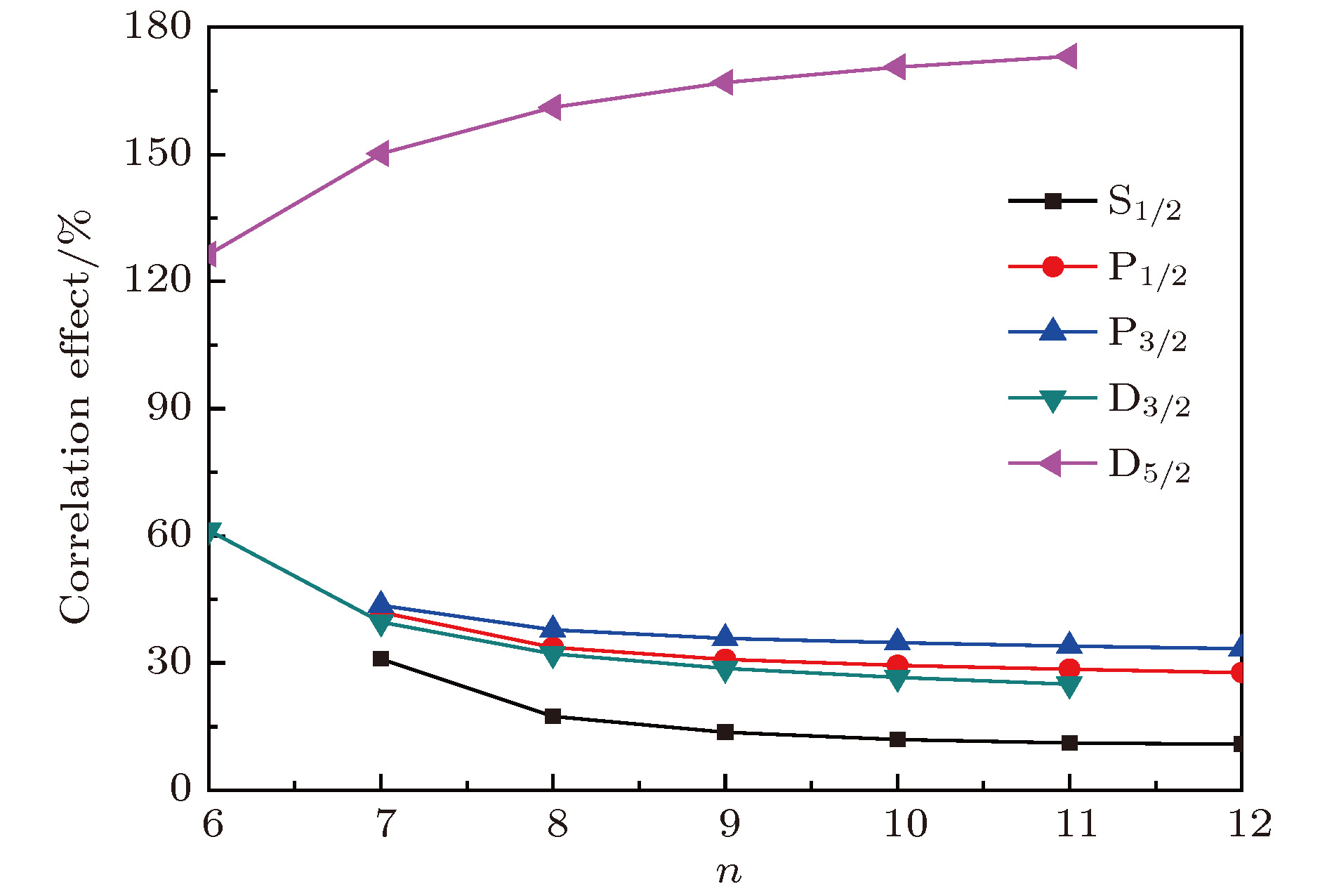
图 2 直接效应ADF、核极化效应ACP、对关联效应APC, 以及相对于CCSD的3种效应的总和AT = ADF + ACP + APC, 针对主量子数n的S, P和D态的结果A的比率 (a) ADF/A; (b) ACP/A; (c) APC/A; (d) AT/A
Fig. 2. Ratios of direct effect ADF, core polarization effect ACP, pair correlation effect APC, and the total of the three effects AT = ADF + APC + ACP to the CCSD, results A for S, P and D states against the principal quantum number n: (a) ADF/A; (b) ACP/A; (c) APC/A; (d) AT/A.
表 1 212Fr原子S态的超精细结构常数A (单位: MHz)
Table 1. Hyperfine-structure constant A for the S states of 212Fr in MHz.
下载: 导出CSV
表 2 212Fr原子P态的超精细结构常数A (单位: MHz)
Table 2. Hyperfine-structure constant A for the P states of 212Fr in MHz.
能级 ${A_{{\rm{DF}}}}$ ${A_{{\rm{CCSD}}}}$ $\varDelta /$% Ref.[38] 实验值 7P1/2 642.48 1198.10 41.96 1181(9) 1189.1(4.6)[28] 1187.1(6.8)[39] 1192.0(2)[32] 8P1/2 228.04 372.04 33.66 371(5) 373.0(1)[39] 9P1/2 106.78 167.21 30.88 10P1/2 58.35 89.53 29.46 11P1/2 35.26 53.38 28.51 12P1/2 22.88 34.24 27.68 7P3/2 51.05 97.88 43.55 96(3) 97.2(1)[27] 97.2(1)[39] 8P3/2 18.67 32.51 37.82 32(3) 32.8(1)[39] 9P3/2 8.89 15.00 35.83 10P3/2 4.91 8.15 34.75 11P3/2 3.00 4.92 34.01 12P3/2 1.97 3.20 33.33
下载: 导出CSV
表 3 212Fr原子D态的超精细结构常数A (单位: MHz)
Table 3. Hyperfine-structure constant A for the D states of 212Fr in MHz.
能级 ${A_{{\rm{DF}}}}$ ${A_{{\rm{CCSD}}}}$ $\varDelta / $% Ref.[38] 实验值 6D3/2 33.25 92.91 61.27 79(5) 7D3/2 16.82 30.17 39.65 29(3) 8D3/2 8.65 13.81 32.20 13(1) 13.0(6)[29] 9D3/2 4.93 7.50 28.75 7(1) 7.1(7)[29] 10D3/2 3.06 4.52 26.64 11D3/2 2.03 2.93 24.97 6D5/2 13.14 –53.92 126.38 –54(5) 7D5/2 6.32 –13.64 150.21 –15(3) 8D5/2 3.20 –5.67 161.12 –6(1) –7.1(6)[29] 9D5/2 1.81 –2.96 166.96 –3.3(6) –3.6(4)[29] 10D5/2 1.12 –1.72 170.59 11D5/2 0.74 –1.10 173.16
下载: 导出CSV
表 4 Fr原子同位素的磁偶极矩
$\mu$ Table 4. Magnetic dipole moment
$\mu$ of Fr isotope.同位素 核自旋 7P1/2 7P3/2 ${\mu}$ ${A_{{\rm{expt}}.}}$[33] ${{\mu} _{1/2}}$ ${A_{{\rm{expt}}.}}$[33] ${{\mu} _{3/2}}$ ${{\mu} _{{\rm{present}}}}$ ${{\mu} _{{\rm{expt}}{\rm{.}}}}$[33] 207Fr 9/2 90.7(6) 3.85(3) 3.85(3) 3.89(9) 208Fr 7 874.8(3) 4.723(2) 72.4(5) 4.784(33) 4.753(33) 4.75(10) 209Fr 9/2 1127.9(2) 3.914(1) 93.3(5) 3.963(21) 3.939(22) 3.95(8) 210Fr 6 946.3(3) 4.379(1) 78.0(2) 4.418(11) 4.399(20) 4.40(9) 211Fr 9/2 1142.1(2) 3.964(1) 94.9(3) 4.031(13) 3.998(34) 4.00(8) 212Fr 5 1187(7) 4.577(26) 97.2(1) 4.588(5) 4.583(30) 4.62(9) 213Fr 9/2 1150(8) 3.991(28) 95.3(3) 4.047(13) 4.019(30) 4.02(8) 220Fr 1 –73.2(5) –0.691(5) –0.691(5) –0.67(1) 221Fr 5/2 808(12) 1.558(23) 65.5(6) 1.545(14) 1.552(25) 1.58(3) 222Fr 2 33(1) 0.623(19) 0.623(19) 0.63(1) 223Fr 3/2 83.3(9) 1.179(13) 1.179(13) 1.17(2) 224Fr 1 42.1(7) 0.397(7) 0.397(7) 0.40(1) 225Fr 3/2 77(3) 1.090(42) 1.090(42) 1.07(2) 226Fr 1 7(1) 0.066(9) 0.066(9) 0.071(2) 227Fr 1/2 316(2) 1.491(9) 1.491(9) 1.50(3) 228Fr 2 –41(2) –0.77(4) –0.77(4) –0.76(2)
下载: 导出CSV
-
[1] Grant I P 2007 Relativistic Quantum Theory of Atoms and Molecules (New York: Springer) pp533−577
[2] Fischer C F, Brage T, Jönsson P 1997 Computational Atomic Structure: An MCHF Approach (UK: Institute of Physics) pp1−67
[3] Jönsson P, Gaigalas G, Bieroń J, Fishcher C F, Grant I 2013 Computer Physics Communications. 184 2197
Google Scholar
[4] Jönsson P, He X, Fishcher C F, Grant I 2007 Computer Physics Communications. 177 597
Google Scholar
[5] Dzuba V A, Flambaum V V, Kozlov M G 1996 Phys. Rev. A 54 3948
Google Scholar
[6] Dzuba V A, Johnson W R 1998 Phys. Rev. A 57 2459
Google Scholar
[7] Angstmann E J, Dzuba V A, Flambaum V V 2004 Phys. Rev. A 70 014102
Google Scholar
[8] Dinh T H, Dzuba V A, Flambaum V V, Ginges J S M 2008 Phys. Rev. A 78 054501
Google Scholar
[9] Kozlov M G, Porsev S G, Johnson W R 2001 Phys. Rev. A 64 052107
Google Scholar
[10] Pal R, Safronova M S, Johnson W R, Derevianko A, Porsev S G 2007 Phys. Rev. A 75 042515
Google Scholar
[11] Blundell S A, Johnson W R, Liu Z W, Sapirstein 1989 Phys. Rev. A 40 2233
Google Scholar
[12] Eliav E, Vikas M J, Ishikawa Y, Kaldor U 2005 Chem. Phys. 311 163
Google Scholar
[13] Mani B K, Angom D 2011 Phys. Rev. A 83 012501
Google Scholar
[14] Kallay M, Nataraj H S, Sahoo B K, Das B P, Visscher L 2011 Phys. Rev. A 83 030503
Google Scholar
[15] Nandy D K, Singh Y, Sahoo B K 2014 Phys. Rev. A 89 062509
Google Scholar
[16] Borschevsky A, Eliav E, Vilkas M J, Ishikawa Y, Kaldor U 2007 Phys. Rev. A 75 042514
Google Scholar
[17] Eliav E, Kaldor U, Ishikawa Y 1996 Phys. Rev. A 53 3050
Google Scholar
[18] Chaudhuri R K, Chattopadhyay S, Mahapatra U S 2013 J. Phys. Chem. A 117 12616
Google Scholar
[19] Tang Y B, Lou B Q, Shi T Y 2017 Phys. Rev. A 96 022513
Google Scholar
[20] Tang Y B, Gao N N, Lou B Q, Shi T Y 2018 Phys. Rev. A 98 062511
Google Scholar
[21] Byrnes T M R, Dzuba V A, Flambaum F F, Murray D W 1999 Phys. Rev. A 59 3082
Google Scholar
[22] Mukherjee D, Sahoo B K, Nataraj H S, Das B P 2009 J. Phys. Chem. A 113 12549
Google Scholar
[23] Sakemi Y, Harada K, Hayamizu T, Itoh M, Kawamura H, Liu S, Nataraj H S, Oikawa A, Saito M, Sato T 2011 J. Phys. Conf. Ser. 302 012051
Google Scholar
[24] Sahoo B K, Aoki T, Das B P, Sakemi Y 2016 Phys. Rev. A 93 032520
Google Scholar
[25] Atutov S N, Calabrese R, Corradi L, Dainelli A, Mauro C D, Khanbekyan A, Mariotti E, Minguzzi P, Moi L, Sanguinetti S, Stancari G, Tomassetti L 2008 Proc. SPIE 7027 70270C
[26] Ekström C, Ingelman S, Wannberg G, Skarestad M 1978 Physica Scripta 18 51
Google Scholar
[27] Coc A, Thibault C, Touchard F, Duong H T, Juncar P, Liberman S, Pinard J, Lermé J, Vialle J L, Büttgenbach S, Mueller A C, Pesnelle A, the ISOLDE Collaboration 1985 Phys. Lett. B 163 66
Google Scholar
[28] Coc A, Thibault C, Touchard F, Duong H T, Juncar P, Liberman S, Pinard J, Carre M, Lermé J, Vialle J L, Büttgenbach S, Mueller A C, Pesnelle A, the ISOLDE Collaboration 1987 Nucl. Phys. A 468 1
Google Scholar
[29] Arnold E, Borchers W, Duong H T, Juncar P, Lermé J, Lievens P, Neu W, Neugart R, Pellerin M, Pinard J, Vialle J L, Wendt K, the ISOLDE Collaboration 1990 J. Phys. B 23 3511
Google Scholar
[30] Arnold E, Borchers W, Carré M, Duong H T, Juncar P, Lermé J, Liberman S, Neu W, Neugart R, Otten W, Pellerin M, Pinard J, Pesnelle A, Vialle J L, Wendt K, the ISOLDE Collaboration 1989 J. Phys. B 22 L391
Google Scholar
[31] Bauche J, Duong H T, Juncar P, Liberman S, Pinard J, Coc A, Thibault C, Touchard F, Lermé J, Vialle J L, Büttgenbach S, Mueller A C, Pesnelle A, the ISOLDE Collaboration 1986 J. Phys. B 19 L593
Google Scholar
[32] Grossman J S, Orozco L A, Simsarian J E, Sprouse G D, Zhao W Z 1999 Phys. Rev. Lett. 83 935
Google Scholar
[33] Sansonetti J E 2007 J. Phys. Chem. Ref. Data 36 497
Google Scholar
[34] Gomez E, Aubin S, Orozco L A, Sprouse G D, Iskrenova-Tchoukova E, Safronova M S 2008 Phys. Rev. Lett. 100 172502
Google Scholar
[35] Dzuba V A, Flambaum V V, Sushkov O P 1984 J. Phys. B: At. Mol. Phys. 17 1953
Google Scholar
[36] Owusu A, Dougherty R W, Gowri G, Das T P 1997 Phys. Rev. A 56 305
Google Scholar
[37] Safronova M S, Johnson W R, Derevianko A 1999 Phys. Rev. A 60 4476
Google Scholar
[38] Sahoo B K, Nandy D K, Das B P, Sakemi Y 2015 Phys. Rev. A 91 042507
Google Scholar
[39] Duong H T, Juncar P, Liberman S, Mueller A C, Neugart R, Otten E W, Peuse B, Pinard J, Stoke H H, Thibault C, Touchard F, Vialle J L, Wendt K, the ISOLDE Collaboration 1987 Europhys. Lett. 3 175
Google Scholar
[40] Barber Z W, Stalnaker J E, Lemke N D, Poli N, Oates C W, Fortier T M, Diddams S A, Hollberg L, Hoyt C W, Taichenachev A V, Yudin V I 2008 Phys. Rev. Lett. 100 103002
Google Scholar
[41] Kien F L, Balykin V I, Hakuta K 2005 J. Phys. Soc. Jpn. 74 910
Google Scholar
[42] Ingvar L 1978 Int. J. Quantum Chem. 12 33
[43] Sinha D, Mukhopadhyay S, Mukherjee D 1986 Chem. Phys. Lett. 129 369
Google Scholar
[44] Blundell S A, Johnson W R, Sapiratein J 1991 Phys. Rev. A 43 3407
Google Scholar
[45] Porsev S G, Beloy K, Derevianko A 2010 Phys. Rev. D 82 036008
Google Scholar
[46] Sahoo B K, Sur C, Beier T, Das B P, Chaudhuri R K, Mukherjee D 2007 Phys. Rev. A 75 042504
Google Scholar
[47] Safronova M S, Safronova U I 2011 Phys. Rev. A 83 052508
Google Scholar
下载:
计量
- 文章访问数: 10199
- PDF下载量: 95
- 被引次数: 0
