-
近年来, 碱土金属原子和类碱土金属原子体系的研究成为冷原子物理的研究热点之一. 特别是最近在173Yb原子中发现的轨道Feshbach共振, 使得研究有强相互作用的碱土金属和类碱土金属原子系统成为可能, 极大扩展了此类原子体系的研究范围. 本文介绍了173Yb费米气体在轨道Feshbach共振附近的杂质态问题. 在此问题中, 位于
$ ^{3}{\rm P}_0 $ 态的杂质原子与处于基态的背景费米海相互作用, 并在费米海表面产生分子态或极化子态. 本文使用试探波函数的研究方法, 首先对分子态和吸引极化子态进行介绍, 并重点描述了分子态与吸引极化子态间的转变. 其次归纳总结了排斥极化子态的相关性质, 如有效质量、衰变率等. 然后考虑双费米面情况, 介绍在闭通道中引入另外一个费米面对系统产生的影响. 最后简要介绍二维173Yb费米气体中的杂质态问题.-
关键词:
- 类碱土金属原子 /
- 轨道Feshbach共振 /
- 分子态 /
- 极化子态
In recent years, alkaline-earth and alkaline-earth-like atoms have attracted much research interest in the field of ultracold atom. Especially, the recently discovered orbital Feshbach resonance makes it possible to investigate a strongly interacting gas of alkaline-earth or alkaline-earth-like atoms, which has greatly enriched the scope of quantum simulation in these systems. This paper focuses on the impurity problem in a Fermi gas of 173Yb atoms near orbital Feshbach resonance. In this problem, the impurity atom in 3P0 state will interact with the background Fermi sea in the ground state and the molecule or polaron state will be produced out of the Fermi sea. By using the Chevy-like ansatz, we investigate the properties of the molecule and attractive polaron states firstly and a transition between these two states will be found. Then, some properties of the repulsive polaron state will be introduced, such as the effective mass and the decay rate. Furthermore, the effect of an additional Fermi sea will be considered in this system. Finally, we will discuss the impurity problem in a two-dimensional system.-
Keywords:
- alkaline-earth-like atom /
- orbital Feshbach resonance /
- the molecule state /
- the polaron state
[1] Takamoto M, Hong F L, Higashi R, Katori H 2005 Nature 435 321
Google Scholar
[2] Ludlow A D, Boyd M M, Zelevinsky T, Foreman S M, Blatt S, Notcutt M, Ido T, Ye J 2006 Phys. Rev. Lett. 96 033003
Google Scholar
[3] Bloom B J, Nicholson T L, Williams J R, Campbell S L, Bishof M, Zhang X, Zhang W, Bromley S L, Ye J 2014 Nature 506 71
Google Scholar
[4] Wu C, Hu J P, Zhang S C 2003 Phys. Rev. Lett. 91 186402
Google Scholar
[5] Fukuhara T, Takasu Y, Kumakura M, Takahashi Y 2007 Phys. Rev. Lett. 98 030401
Google Scholar
[6] Gorshkov A V, Rey A M, Daley A J, Boyd M M, Ye J, Zoller P, Lukin M D 2009 Phys. Rev. Lett. 102 110503
Google Scholar
[7] Zhang X, Bishof M, Bromley S L, Kraus C V, Safronova M S, Zoller P, Rey A M, Ye J 2014 Science 345 6203
[8] Cappellini G, Mancini M, Pagano G, Lombardi P, Livi L, Siciliani de Cumis M, Cancio P, Pizzocaro M, Calonico D, Levi F, Sias C, Catani J, Inguscio M, Fallani L 2014 Phys. Rev. Lett. 113 120402
[9] Scazza F, Hofrichter C, Höfer M, De Groot P C, Bloch I, Fölling S 2014 Nat. Phys. 10 779
[10] Enomoto K, Kasa K, Kitagawa M, Takahashi Y 2008 Phys. Rev. Lett. 101 203201
Google Scholar
[11] Blatt S, Nicholson T L, Bloom B J, Williams J R, Thomsen J W, Julienne P S, Ye J 2011 Phys. Rev. Lett. 107 073202
Google Scholar
[12] Yan M, DeSalvo B J, Ramachandhran B, Pu H, Killian T C 2013 Phys. Rev. Lett. 110 123201
Google Scholar
[13] Zhang R, Cheng Y, Zhai H, Zhang P 2015 Phys. Rev. Lett. 115 135301
Google Scholar
[14] Pagano G, Mancini M, Cappellini G, Livi L, Sias C, Catani J, Inguscio M, Fallani L 2015 Phys. Rev. Lett. 115 265301
Google Scholar
[15] Höfer M, Riegger L, Scazza F, Hofrichter C, Fernandes D R, Parish M M, Levinsen J, Bloch I, Fölling S 2015 Phys. Rev. Lett. 115 265302
Google Scholar
[16] Chin C, Grimm R, Julienne P, Tiesinga E 2010 Rev. Mod. Phys. 82 1225
Google Scholar
[17] Porsev S G, Derevianko A, Fortson E N 2004 Phys. Rev. A 69 021403(R)
Google Scholar
[18] Santra R, Christ K V, Greene C H 2004 Phys. Rev. A 69 042510
Google Scholar
[19] Xu J, Zhang R, Cheng Y, Zhang P, Qi R, Zhai H 2016 Phys. Rev. A 94 033609
Google Scholar
[20] Iskin M 2016 Phys. Rev. A 94 011604(R)
Google Scholar
[21] Isaev L, Schachenmayer J, Rey A M 2016 Phys. Rev. Lett. 117 135302
Google Scholar
[22] He L, Wang J, Peng S G, Liu X J, Hu H 2016 Phys. Rev. A 94 043624
Google Scholar
[23] Chen J G, Deng T S, Yi W, Zhang W 2016 Phys. Rev. A 94 053627
Google Scholar
[24] Iskin M 2017 Phys. Rev. A 95 013618
Google Scholar
[25] Zhang Y C, Ding S, Zhang S 2017 Phys. Rev. A 95 041603(R)
Google Scholar
[26] Wang S, Pan J S, Cui X, Zhang W, Yi W 2017 Phys. Rev. A 95 043634
Google Scholar
[27] Deng T S, Zhang W, Yi W 2017 Phys. Rev. A 96 050701(R)
Google Scholar
[28] Zhou X, Pan J S, Liu Z X, Zhang W, Yi W, Chen G, Jia S 2017 Phys. Rev. Lett. 119 185701
Google Scholar
[29] Xu J, Qi R 2018 Eur. Phys. J. D 72 65
Google Scholar
[30] Chen J G, Shi Y R, Zhang X, Zhang W 2018 Frontiers of Physics 4 136702
[31] Schirotzek A, Wu C H, Sommer A, Zwierlein M W 2009 Phys. Rev. Lett. 102 230402
Google Scholar
[32] Kohstall C, Zaccanti M, Jag M, Trenkwalder A, Massignan P, Bruun G M, Schreck F, Grimm R 2012 Nature 485 615
Google Scholar
[33] Koschorreck M, Pertot D, Vogt E, Fröhlich B, Feld M, Köhl M 2012 Nature 485 619
Google Scholar
[34] Nascimbène S, Navon N, Jiang K J, Tarruell L, Teichmann M, McKeever J, Chevy F, Salomon C 2009 Phys. Rev. Lett. 103 170402
Google Scholar
[35] Massignan P, Zaccanti M, Bruun G M 2014 Rep. Prog. Phys. 77 034401
Google Scholar
[36] Cui X, Zhai H 2010 Phys. Rev. A 81 041602(R)
Google Scholar
[37] Pilati S, Bertaina G, Giorgini S, Troyer M 2010 Phys. Rev. Lett. 105 030405
Google Scholar
[38] Mathy C J M, Parish M M, Huse D A 2011 Phys. Rev. Lett. 106 166404
Google Scholar
[39] Yi W, Zhang W 2012 Phys. Rev. Lett. 109 140402
Google Scholar
[40] Zhou L, Cui X, Yi W 2014 Phys. Rev. Lett. 112 195301
Google Scholar
[41] Nishida Y 2015 Phys. Rev. Lett. 114 115302
Google Scholar
[42] Yi W, Cui X 2015 Phys. Rev. A 92 013620
Google Scholar
[43] Landau L D 1933 Phys. Z. Sowjetunion 3 644
[44] Pekar S I 1946 Zh. Eksp. Teor. Fiz. 16 335
[45] Fröhlich H, Pelzer H, Zienau S 1950 Philos. Mag. 41 221
Google Scholar
[46] Fröhlich H 1954 Adv. Phys. 3 325
Google Scholar
[47] Feynman R P 1955 Phys. Rev. 97 660
Google Scholar
[48] Bardeen J, Baym G, Pines D 1967 Phys. Rev. 156 207
Google Scholar
[49] Li J, Schneider W D, Berndt R, Delley B 1998 Phys. Rev. Lett. 80 2893
Google Scholar
[50] Chikkatur A P, Görlitz A, Stamper-Kurn D M, Inouye S, Gupta S, Ketterle W 2000 Phys. Rev. Lett. 85 483
Google Scholar
[51] Catani J, Lamporesi G, Naik D, Gring M, Inguscio M, Minardi F, Kantian A, Giamarchi T 2012 Phys. Rev. A 85 023623
Google Scholar
[52] Spethmann N, Kindermann F, John S, Weber C, Meschede D, Widera A 2012 Phys. Rev. Lett. 109 235301
Google Scholar
[53] Scelle R, Rentrop T, Trautmann A, Schuster T, Oberthaler M K 2013 Phys. Rev. Lett. 111 070401
Google Scholar
[54] Cucchietti F M, Timmermans E 2006 Phys. Rev. Lett. 96 210401
Google Scholar
[55] Tempere J, Casteels W, Oberthaler M K, Knoop S, Timmermans E, Devreese J T 2009 Phys. Rev. B 80 184504
Google Scholar
[56] Rath S P, Schmidt R 2013 Phys. Rev. A 88 053632
Google Scholar
[57] Qiu X, Cui X, Yi W 2016 Phys. Rev. A 94 051604(R)
Google Scholar
[58] Sun M, Cui X 2017 Phys. Rev. A 96 022707
Google Scholar
[59] Bai X D, Wang J, Liu X J, Xiong J, Deng F G, Hu H 2018 Phys. Rev. A 98 023627
Google Scholar
[60] Hu H, Mulkerin B C, Wang J, Liu X J 2018 Phys. Rev. A 98 013626
Google Scholar
[61] Chevy F 2006 Phys. Rev. A 74 063628
Google Scholar
[62] Combescot R, Recati A, Lobo C, Chevy F 2007 Phys. Rev. Lett. 98 180402
Google Scholar
[63] Schmidt R, Enss T 2011 Phys. Rev. A 83 063620
Google Scholar
[64] Massignan P, Bruun G M 2011 Eur. Phys. J. D 65 83
Google Scholar
[65] Deng T S, Lu Z C, Shi Y R, Chen J G, Zhang W, Yi W 2018 Phys. Rev. A 97 013635
Google Scholar
[66] Scazza F, Valtolina G, Massignan P, Recati A, Amico A, Burchianti A, Fort C, Inguscio M, Zaccanti M, Roati G 2017 Phys. Rev. Lett. 118 083602
Google Scholar
[67] Dyke P, Kuhnle E D, Whitlock S, Hu H, Mark M, Hoinka S, Lingham M, Hannaford P, Vale C J 2011 Phys. Rev. Lett. 106 105304
Google Scholar
-
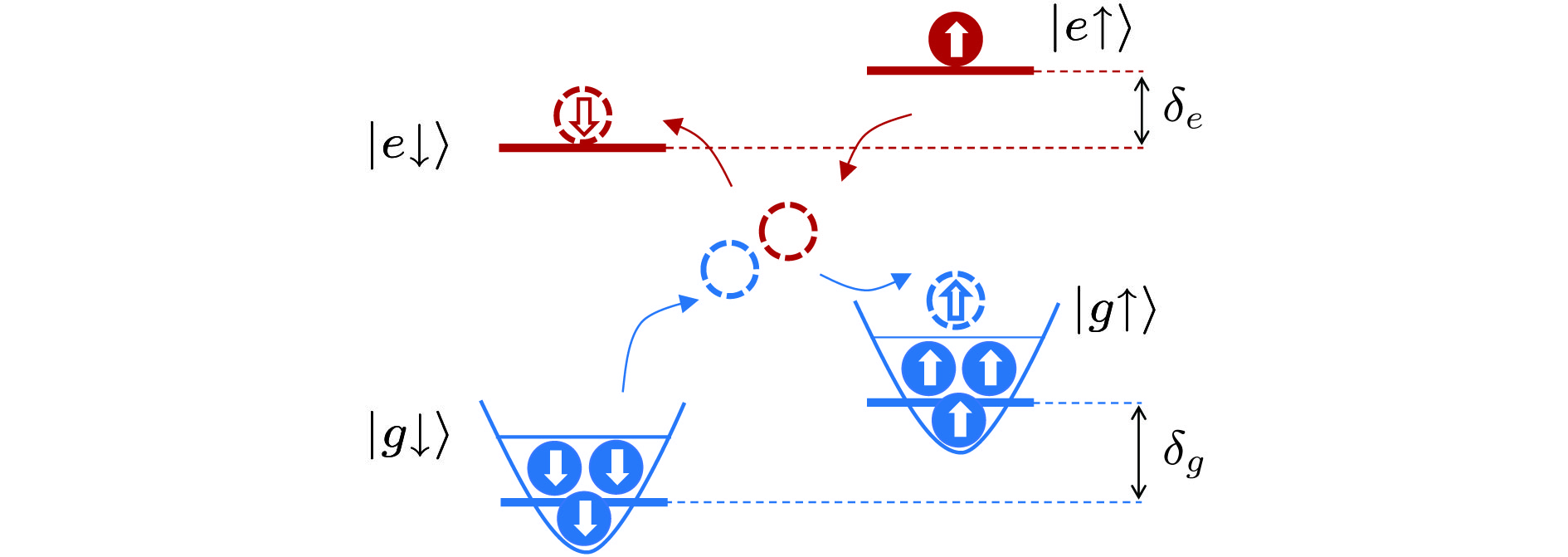
图 1 类碱土金属原子中的OFR能级图. 杂质原子处于
$ | e\uparrow \rangle $ 态, 费米海处于$ | g\downarrow \rangle $ 态. 通过相互作用, 杂质原子和费米海中原子会被散射到闭通道的两个态上.$ \delta_g $ 和$ \delta_e $ 是两个能级的塞曼移动Fig. 1. Level diagram of an OFR. An impurity atom in the
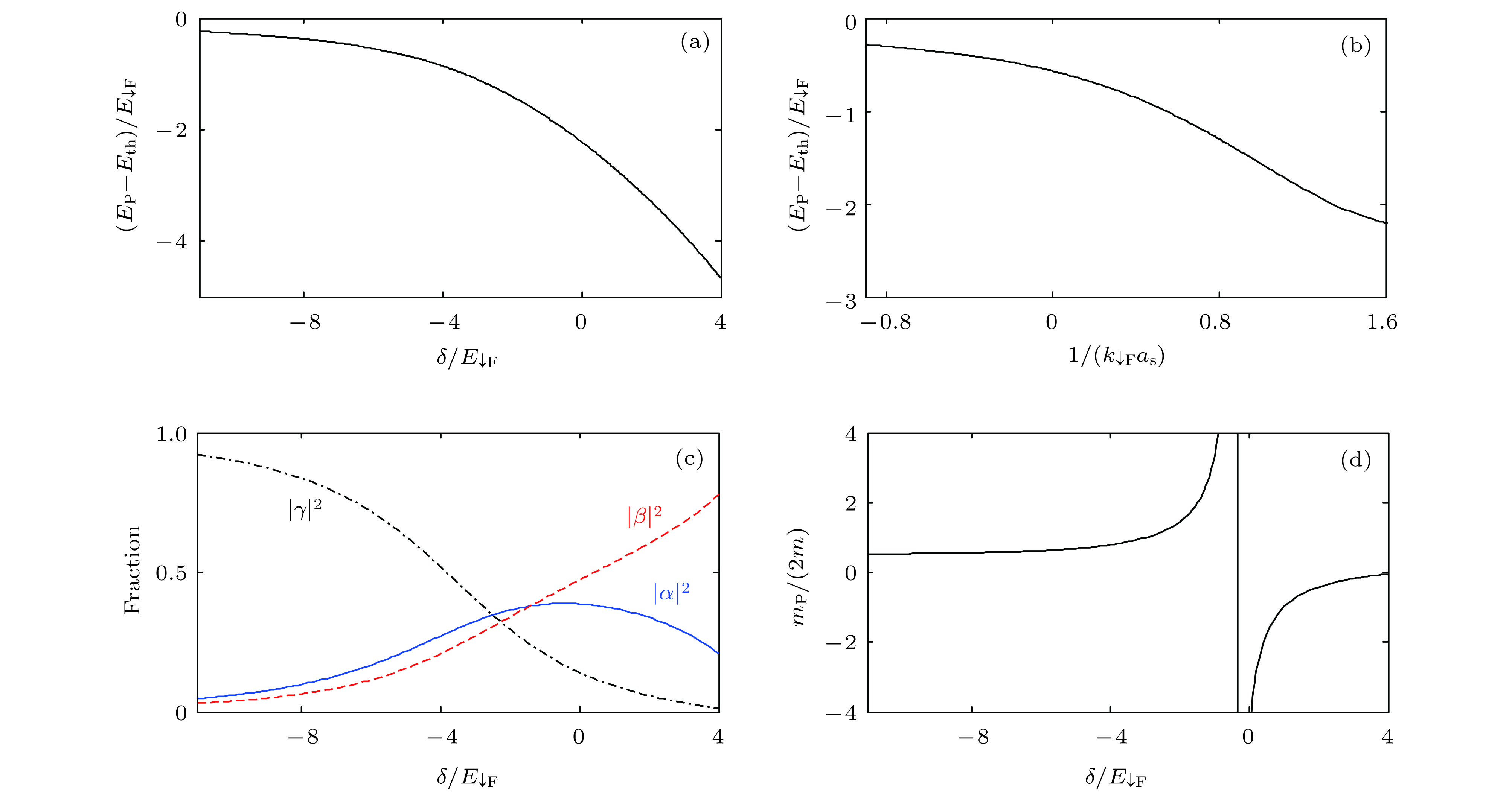
$ |e\uparrow\rangle $ state is immersed in a Fermi gas of alkaline-earth-like atoms in the$ |g\downarrow\rangle $ state.$ \delta_g $ and$ \delta_e $ are the Zeeman shifts of the$ |g\rangle $ and$ |e\rangle $ manifolds, respectively.
图 2 (a), (b) 分子态的能量随
$ \delta $ 和$ 1/(k_{\downarrow {\rm F}}a_{\rm s}) $ 的变化; (c) 分子态的波函数分布情况; (d)$ E_{\rm M} $ 接近阈值能量$ E_{\rm F} $ 的分子态, 在$ { Q}\neq 0 $ 时的有效质量. 引自参考文献[23]Fig. 2. (a), (b) The eigenenergy in the molecular state varies with
$ \delta $ and$ 1/(k_{\downarrow {\rm F}}a_{\rm s}) $ ; (c) the wave functions' distribution in the open and closed channels; (d) the effective mass for the molecular state whose energy is closed to the threshold energy when$ { Q}\neq 0 $ . Reproduced from Ref. [23].
图 3 (a), (b) 吸引极化子态的能量随
$ \delta $ 和$ 1/(k_{\downarrow {\rm F}}a_{\rm s}) $ 的变化; (c) 吸引极化子态的波函数分布情况; (d) 吸引极化子态在$ { Q}\neq 0 $ 时的有效质量, 图中发散点为$ \delta/E_{\downarrow {\rm F}}\approx -0.35 $ , 对应于$ 1/(k_{\downarrow {\rm F}}a_{\rm s})\approx 1.40 $ . 图中参数与图2中一致. 引自参考文献[23]Fig. 3. (a), (b) The eigenenergy in the attractive polaron state varies with
$ \delta $ and$ 1/(k_{\downarrow {\rm F}}a_{\rm s}) $ ; (c) the wave functions' distribution in the open and closed channels; (d) the effective mass for the attractive polaron state when$ { Q}\neq 0 $ . Parameters here are the same as those in Fig. 2. Reproduced from Ref. [23].
图 4 (a), (b) 分子态(黑色实线)和吸引极化子态(蓝色虚线)的本征能量随
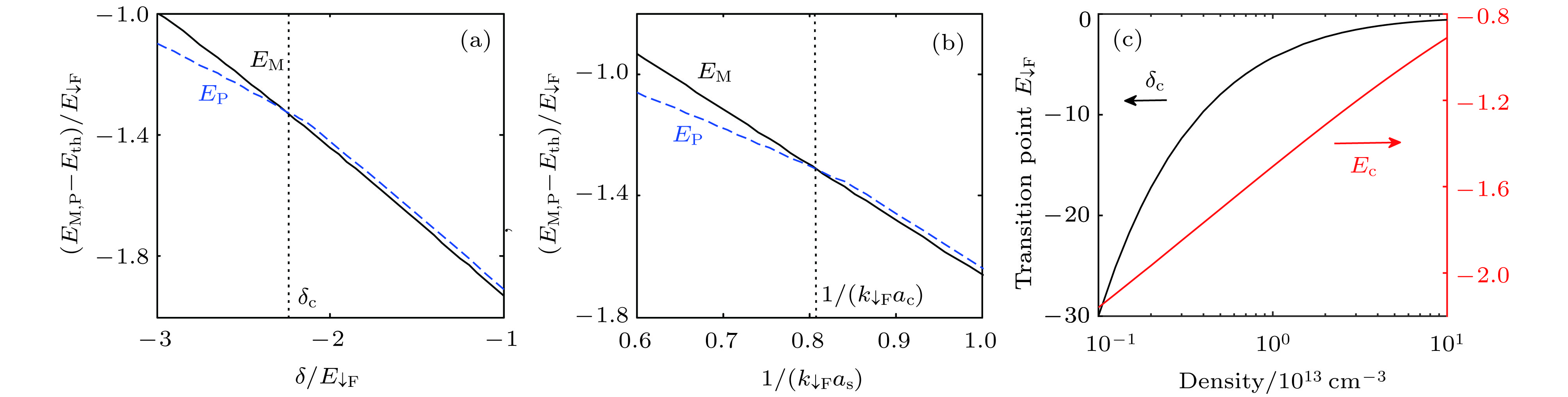
$ \delta $ 和$ 1/(k_{\downarrow {\rm F}}a_{\rm s}) $ 的变化, 吸引极化子态到分子态的转变发生在$ \delta_{\rm c}/E_{\downarrow {\rm F}} \approx -2.3 $ 和$ 1/(k_{\downarrow {\rm F}} a_{\rm c})\approx 0.81 $ 处; (c) 转变点$ \delta_{\rm c} $ (黑色实线)和对应的转变能量$ E_{\rm c} $ (红色实线)随粒子数密度的变化. 引自参考文献[23]Fig. 4. (a), (b) The eigenenergy of the molecule (black solid) and polaron (blue dashed) states vary with
$ \delta $ and$ 1/(k_{\downarrow {\rm F}}a_{\rm s}) $ . The transition point is around$ \delta_c/E_{\downarrow {\rm F}} \approx -2.3 $ and$ 1/(k_{\downarrow {\rm F}} a_{\rm c})\approx 0.81 $ ; (c) the transition point$ \delta_{\rm c} $ (black solid) and the corresponding energy$ E_{\rm c} $ (red solid) vary with particle density. Reproduced from Ref. [23].
图 5 极化子态的谱函数
$ A({ Q}=0, E_{\rm P}) $ 随$ \delta $ 和能量变化的彩色图. 图中红色实线表示分子态能量, 由(8)式计算得到. 黑色点划线为吸引极化子态能量, 由(11)式计算得到. 两条线相交于$ \delta_{\rm c}/E_{\downarrow {\rm F}}\approx -2.3 $ 处. 图中蓝色虚线代表排斥极化子能量, 由(15)式得到. 当$ \delta $ 为较大负值时, 排斥极化子与图中由浅黄色区域代表的粒子-空穴连续区域重合. 当$ \delta $ 大于零时, 由于存在闭通道散射, 排斥极化子分支会变得模糊. 引自参考文献[30]Fig. 5. The spectral function of the polaron state as a function of
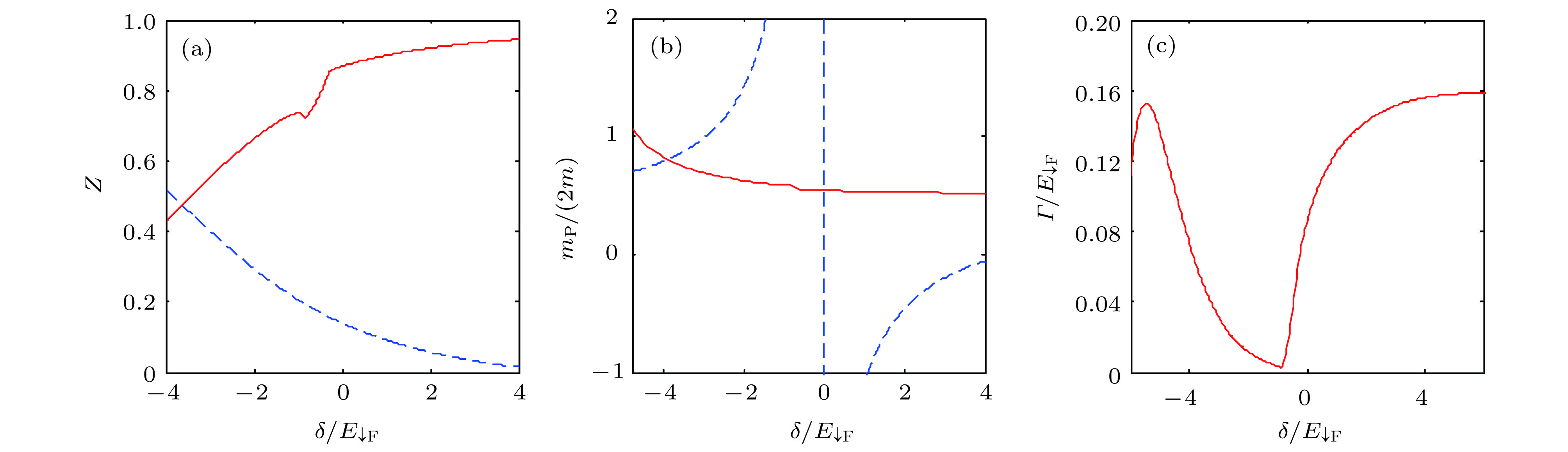
$ \delta $ and energy for$ { Q}=0 $ . The red solid line represents the energy of molecule state, which is given by Eq. (8), and the black dashed-dotted line is the energy of attractive polaron state given by Eq. (11). The value of the crossing point for these two lines is$ \delta_{\rm c}/E_{\downarrow {\rm F}}\approx -2.3 $ . The blue dashed line denotes the repulsive polaron energy given by Eq. (15). At large negative$ \delta $ , the branch of repulsive polaron merges into the molecule-hole continuum, which is represented by the broad light yellow area. When$ \delta $ is a positive value, because of the closed channel scattering continuum, the repulsive polaron branch will be blurred. Reproduced from Ref. [30].
图 6 (a), (b) 极化子态的准粒子残余和有效质量随
$ \delta $ 的变化, 其中红色实线对应排斥极化子态, 蓝色虚线对应吸引极化子态; (c) 排斥极化子态的衰变率随$ \delta $ 的变化. 引自参考文献[65]Fig. 6. (a), (b) Quasiparticle residues
$ Z_\pm $ and effective masses for the attractive (blue dashed) and repulsive (red solid) polarons as functions of$ \delta $ ; (c) the decay rate$ \varGamma $ varies with$ \delta $ . Reproduced from Ref. [65].
图 7 双费米面情况下, 类碱土金属原子的OFR能级图. 杂质原子处于
$ | e\uparrow \rangle $ 态, 费米海处于$ | g\downarrow \rangle $ 态和$ | g\uparrow \rangle $ 态. 通过相互作用, 杂质原子和费米海中原子会被散射到闭通道的两个态上.$ \delta_g $ 和$ \delta_e $ 是两个能级的塞曼移动Fig. 7. Level diagram of an OFR, which has two fermi seas. An impurity atom in the
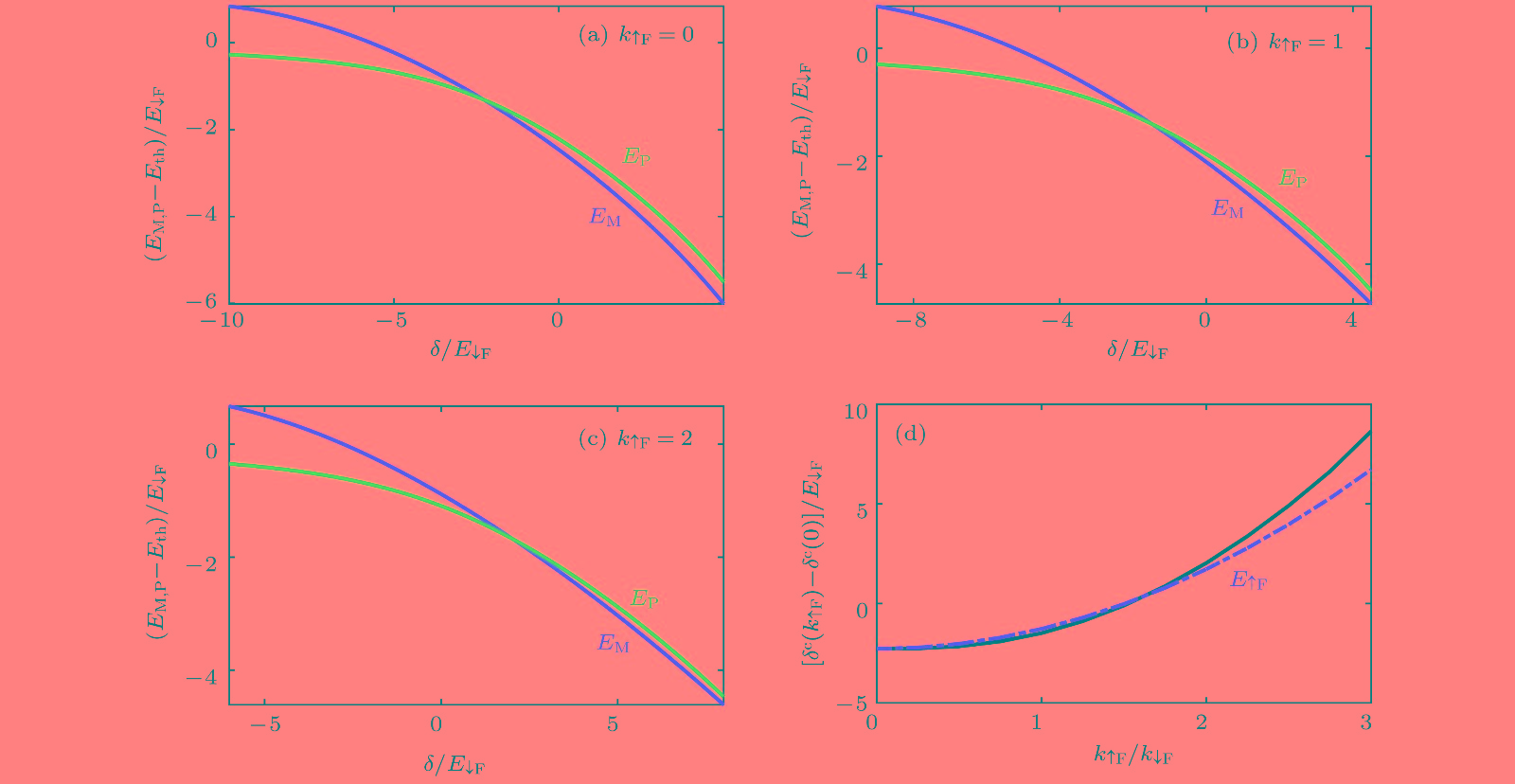
$ |e\uparrow\rangle $ state is immersed in a Fermi gas of alkaline-earth-like atoms in the$ |g\downarrow\rangle $ and$ | g\uparrow \rangle $ states.$ \delta_g $ and$ \delta_e $ are the Zeeman shifts of the$ |g\rangle $ and$ |e\rangle $ manifolds, respectively.
图 8 质心动量为零,
$ k_{\uparrow {\rm F}}=0 $ ((a)),$ k_{\uparrow {\rm F}}=1 $ ((b)),$ k_{\uparrow {\rm F}}=2 $ ((c))时, 分子态和吸引极化子态的本征能量随$ \delta $ 的变化; (d) 吸引极化子态到分子态的转变点随$ k_{\uparrow {\rm F}} $ 的变化, 其中, 黑色实线为计算得到的转变点, 红色点划线为费米能级$ E_{\uparrow {\rm F}}=k_{\uparrow {\rm F}}^2 $ . 引自参考文献[30]Fig. 8. The eigen energies of molecule and attractive polaron states vary with
$ \delta $ when$ { Q}=0 $ and (a)$ k_{\uparrow {\rm F}}=0 $ , (b)$ k_{\uparrow {\rm F}}=1 $ , (c)$ k_{\uparrow {\rm F}}=2 $ ; (d) the transition point$ \delta_{\rm c} $ varies with$ k_{\uparrow {\rm F}} $ , where the black solid line represents$ \delta_{\rm c} $ and the red dashed-dotted line is the Fermi level$ E_{\uparrow F}=k_{\uparrow {\rm F}}^2 $ . Reproduced from Ref. [30].
图 9 (a), (c) 分子态的波函数分布情况; (b), (d) 吸引极化子态的波函数分布, 此时质心动量为零. (a)和(b)中,
$ k_{\uparrow {\rm F}}=1 $ , (c)和(d)中,$ k_{\uparrow {\rm F}}=2 $ . 引自参考文献[30]Fig. 9. The fractions of wave functions for molecule ((a), (c)) and attractive polaron states ((b), (d)) with zero center-of-mass momentum. The parameter in this figure is
$ k_{\uparrow {\rm F}}=1 $ for (a) and (b),$ k_{\uparrow {\rm F}}=2 $ for (c) and (d). Reproduced from Ref. [30].
图 10 (a), (b) 在
$ k_{\uparrow {\rm F}} $ 等于不同值时, 排斥极化子态的准粒子残余和有效质量随$ \delta $ 的变化情况. 图(a)中的插图描绘了吸引极化子态的准粒子残余随$ \delta $ 的变化情况. 图中, 当$ k_{\uparrow {\rm F}}=0 $ 时出现的非解析行为发生在$ \delta/E_{\downarrow {\rm F}}=-0.87 $ 处; (c) 排斥极化子态的衰变率$ \varGamma $ 在$ k_{\uparrow {\rm F}} $ 等于不同值时随$ \delta $ 的变化情况. 引自参考文献[30]Fig. 10. (a), (b) The quasiparticle residue
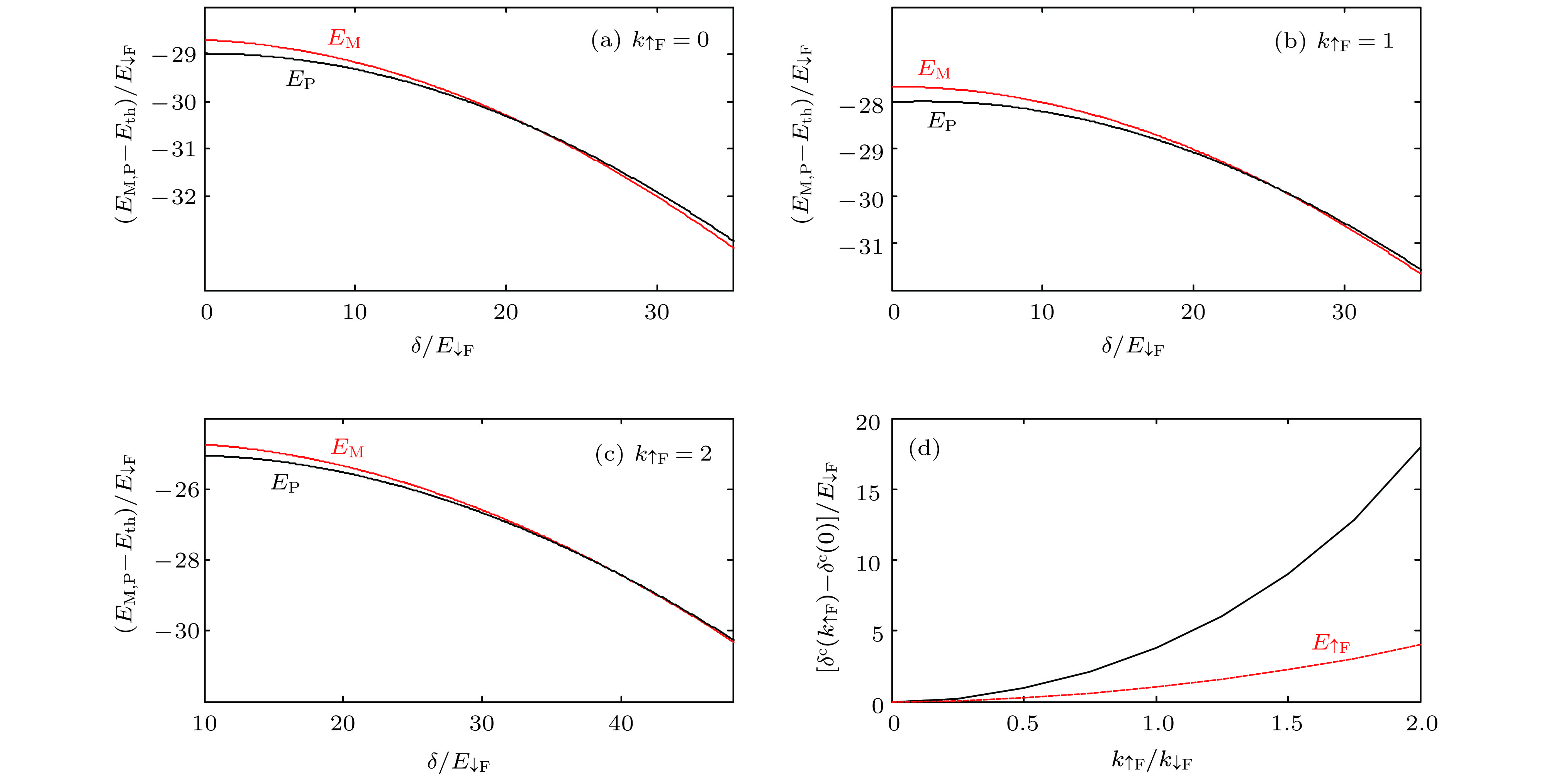
$ Z_+ $ and the effective mass$ m_{{\rm P}+} $ vary with$ \delta $ for different values of$ k_{\uparrow {\rm F}} $ . The obvious kink structure when$ k_{\uparrow {\rm F}}=0 $ appears near$ \delta/E_{\downarrow {\rm F}}=-0.87 $ . The inset of (a) is the residues for attractive polarons; (c) the decay rates vary with$ \delta $ for different values of$ k_{\uparrow {\rm F}} $ . Reproduced from Ref. [30].
图 11 (a)-(c) 二维系统中, 质心动量为零,
$ k_{\uparrow {\rm F}}=0 $ ((a)),$ k_{\uparrow {\rm F}}=1 $ ((b)),$ k_{\uparrow {\rm F}}=2 $ ((c))时, 分子态和吸引极化子态的本征能量随$ \delta $ 的变化; (d) 吸引极化子态到分子态的转变点随$ k_{\uparrow {\rm F}} $ 的变化, 其中黑色实线为计算得到的转变点, 红色虚线为费米能级$ E_{\uparrow {\rm F}}=k_{\uparrow {\rm F}}^2 $ Fig. 11. The eigen energies of molecule and attractive polaron states vary with
$ \delta $ when$ { Q}=0 $ in a two-dimensional system and (a)$ k_{\uparrow {\rm F}}=0 $ , (b)$ k_{\uparrow {\rm F}}=1 $ , (c)$ k_{\uparrow {\rm F}}=2 $ ; (d) the transition point$ \delta_{\rm c} $ varies with$ k_{\uparrow {\rm F}} $ , where the black solid line represents$ \delta_{\rm c} $ and the red dashed line is the Fermi level$ E_{\uparrow {\rm F}}=k_{\uparrow {\rm F}}^2 $ .
图 12 二维系统中
$ k_{\uparrow {\rm F}} $ 等于不同值时排斥极化子态的衰变率$ \varGamma $ 随$ \delta $ 的变化情况Fig. 12. The decay rates of the repulsive polaron state in a a two-dimentional system vary with
$ \delta $ for different values of$ k_{\uparrow {\rm F}} $ . -
[1] Takamoto M, Hong F L, Higashi R, Katori H 2005 Nature 435 321
Google Scholar
[2] Ludlow A D, Boyd M M, Zelevinsky T, Foreman S M, Blatt S, Notcutt M, Ido T, Ye J 2006 Phys. Rev. Lett. 96 033003
Google Scholar
[3] Bloom B J, Nicholson T L, Williams J R, Campbell S L, Bishof M, Zhang X, Zhang W, Bromley S L, Ye J 2014 Nature 506 71
Google Scholar
[4] Wu C, Hu J P, Zhang S C 2003 Phys. Rev. Lett. 91 186402
Google Scholar
[5] Fukuhara T, Takasu Y, Kumakura M, Takahashi Y 2007 Phys. Rev. Lett. 98 030401
Google Scholar
[6] Gorshkov A V, Rey A M, Daley A J, Boyd M M, Ye J, Zoller P, Lukin M D 2009 Phys. Rev. Lett. 102 110503
Google Scholar
[7] Zhang X, Bishof M, Bromley S L, Kraus C V, Safronova M S, Zoller P, Rey A M, Ye J 2014 Science 345 6203
[8] Cappellini G, Mancini M, Pagano G, Lombardi P, Livi L, Siciliani de Cumis M, Cancio P, Pizzocaro M, Calonico D, Levi F, Sias C, Catani J, Inguscio M, Fallani L 2014 Phys. Rev. Lett. 113 120402
[9] Scazza F, Hofrichter C, Höfer M, De Groot P C, Bloch I, Fölling S 2014 Nat. Phys. 10 779
[10] Enomoto K, Kasa K, Kitagawa M, Takahashi Y 2008 Phys. Rev. Lett. 101 203201
Google Scholar
[11] Blatt S, Nicholson T L, Bloom B J, Williams J R, Thomsen J W, Julienne P S, Ye J 2011 Phys. Rev. Lett. 107 073202
Google Scholar
[12] Yan M, DeSalvo B J, Ramachandhran B, Pu H, Killian T C 2013 Phys. Rev. Lett. 110 123201
Google Scholar
[13] Zhang R, Cheng Y, Zhai H, Zhang P 2015 Phys. Rev. Lett. 115 135301
Google Scholar
[14] Pagano G, Mancini M, Cappellini G, Livi L, Sias C, Catani J, Inguscio M, Fallani L 2015 Phys. Rev. Lett. 115 265301
Google Scholar
[15] Höfer M, Riegger L, Scazza F, Hofrichter C, Fernandes D R, Parish M M, Levinsen J, Bloch I, Fölling S 2015 Phys. Rev. Lett. 115 265302
Google Scholar
[16] Chin C, Grimm R, Julienne P, Tiesinga E 2010 Rev. Mod. Phys. 82 1225
Google Scholar
[17] Porsev S G, Derevianko A, Fortson E N 2004 Phys. Rev. A 69 021403(R)
Google Scholar
[18] Santra R, Christ K V, Greene C H 2004 Phys. Rev. A 69 042510
Google Scholar
[19] Xu J, Zhang R, Cheng Y, Zhang P, Qi R, Zhai H 2016 Phys. Rev. A 94 033609
Google Scholar
[20] Iskin M 2016 Phys. Rev. A 94 011604(R)
Google Scholar
[21] Isaev L, Schachenmayer J, Rey A M 2016 Phys. Rev. Lett. 117 135302
Google Scholar
[22] He L, Wang J, Peng S G, Liu X J, Hu H 2016 Phys. Rev. A 94 043624
Google Scholar
[23] Chen J G, Deng T S, Yi W, Zhang W 2016 Phys. Rev. A 94 053627
Google Scholar
[24] Iskin M 2017 Phys. Rev. A 95 013618
Google Scholar
[25] Zhang Y C, Ding S, Zhang S 2017 Phys. Rev. A 95 041603(R)
Google Scholar
[26] Wang S, Pan J S, Cui X, Zhang W, Yi W 2017 Phys. Rev. A 95 043634
Google Scholar
[27] Deng T S, Zhang W, Yi W 2017 Phys. Rev. A 96 050701(R)
Google Scholar
[28] Zhou X, Pan J S, Liu Z X, Zhang W, Yi W, Chen G, Jia S 2017 Phys. Rev. Lett. 119 185701
Google Scholar
[29] Xu J, Qi R 2018 Eur. Phys. J. D 72 65
Google Scholar
[30] Chen J G, Shi Y R, Zhang X, Zhang W 2018 Frontiers of Physics 4 136702
[31] Schirotzek A, Wu C H, Sommer A, Zwierlein M W 2009 Phys. Rev. Lett. 102 230402
Google Scholar
[32] Kohstall C, Zaccanti M, Jag M, Trenkwalder A, Massignan P, Bruun G M, Schreck F, Grimm R 2012 Nature 485 615
Google Scholar
[33] Koschorreck M, Pertot D, Vogt E, Fröhlich B, Feld M, Köhl M 2012 Nature 485 619
Google Scholar
[34] Nascimbène S, Navon N, Jiang K J, Tarruell L, Teichmann M, McKeever J, Chevy F, Salomon C 2009 Phys. Rev. Lett. 103 170402
Google Scholar
[35] Massignan P, Zaccanti M, Bruun G M 2014 Rep. Prog. Phys. 77 034401
Google Scholar
[36] Cui X, Zhai H 2010 Phys. Rev. A 81 041602(R)
Google Scholar
[37] Pilati S, Bertaina G, Giorgini S, Troyer M 2010 Phys. Rev. Lett. 105 030405
Google Scholar
[38] Mathy C J M, Parish M M, Huse D A 2011 Phys. Rev. Lett. 106 166404
Google Scholar
[39] Yi W, Zhang W 2012 Phys. Rev. Lett. 109 140402
Google Scholar
[40] Zhou L, Cui X, Yi W 2014 Phys. Rev. Lett. 112 195301
Google Scholar
[41] Nishida Y 2015 Phys. Rev. Lett. 114 115302
Google Scholar
[42] Yi W, Cui X 2015 Phys. Rev. A 92 013620
Google Scholar
[43] Landau L D 1933 Phys. Z. Sowjetunion 3 644
[44] Pekar S I 1946 Zh. Eksp. Teor. Fiz. 16 335
[45] Fröhlich H, Pelzer H, Zienau S 1950 Philos. Mag. 41 221
Google Scholar
[46] Fröhlich H 1954 Adv. Phys. 3 325
Google Scholar
[47] Feynman R P 1955 Phys. Rev. 97 660
Google Scholar
[48] Bardeen J, Baym G, Pines D 1967 Phys. Rev. 156 207
Google Scholar
[49] Li J, Schneider W D, Berndt R, Delley B 1998 Phys. Rev. Lett. 80 2893
Google Scholar
[50] Chikkatur A P, Görlitz A, Stamper-Kurn D M, Inouye S, Gupta S, Ketterle W 2000 Phys. Rev. Lett. 85 483
Google Scholar
[51] Catani J, Lamporesi G, Naik D, Gring M, Inguscio M, Minardi F, Kantian A, Giamarchi T 2012 Phys. Rev. A 85 023623
Google Scholar
[52] Spethmann N, Kindermann F, John S, Weber C, Meschede D, Widera A 2012 Phys. Rev. Lett. 109 235301
Google Scholar
[53] Scelle R, Rentrop T, Trautmann A, Schuster T, Oberthaler M K 2013 Phys. Rev. Lett. 111 070401
Google Scholar
[54] Cucchietti F M, Timmermans E 2006 Phys. Rev. Lett. 96 210401
Google Scholar
[55] Tempere J, Casteels W, Oberthaler M K, Knoop S, Timmermans E, Devreese J T 2009 Phys. Rev. B 80 184504
Google Scholar
[56] Rath S P, Schmidt R 2013 Phys. Rev. A 88 053632
Google Scholar
[57] Qiu X, Cui X, Yi W 2016 Phys. Rev. A 94 051604(R)
Google Scholar
[58] Sun M, Cui X 2017 Phys. Rev. A 96 022707
Google Scholar
[59] Bai X D, Wang J, Liu X J, Xiong J, Deng F G, Hu H 2018 Phys. Rev. A 98 023627
Google Scholar
[60] Hu H, Mulkerin B C, Wang J, Liu X J 2018 Phys. Rev. A 98 013626
Google Scholar
[61] Chevy F 2006 Phys. Rev. A 74 063628
Google Scholar
[62] Combescot R, Recati A, Lobo C, Chevy F 2007 Phys. Rev. Lett. 98 180402
Google Scholar
[63] Schmidt R, Enss T 2011 Phys. Rev. A 83 063620
Google Scholar
[64] Massignan P, Bruun G M 2011 Eur. Phys. J. D 65 83
Google Scholar
[65] Deng T S, Lu Z C, Shi Y R, Chen J G, Zhang W, Yi W 2018 Phys. Rev. A 97 013635
Google Scholar
[66] Scazza F, Valtolina G, Massignan P, Recati A, Amico A, Burchianti A, Fort C, Inguscio M, Zaccanti M, Roati G 2017 Phys. Rev. Lett. 118 083602
Google Scholar
[67] Dyke P, Kuhnle E D, Whitlock S, Hu H, Mark M, Hoinka S, Lingham M, Hannaford P, Vale C J 2011 Phys. Rev. Lett. 106 105304
Google Scholar
下载:
计量
- 文章访问数: 7858
- PDF下载量: 102
- 被引次数: 0
