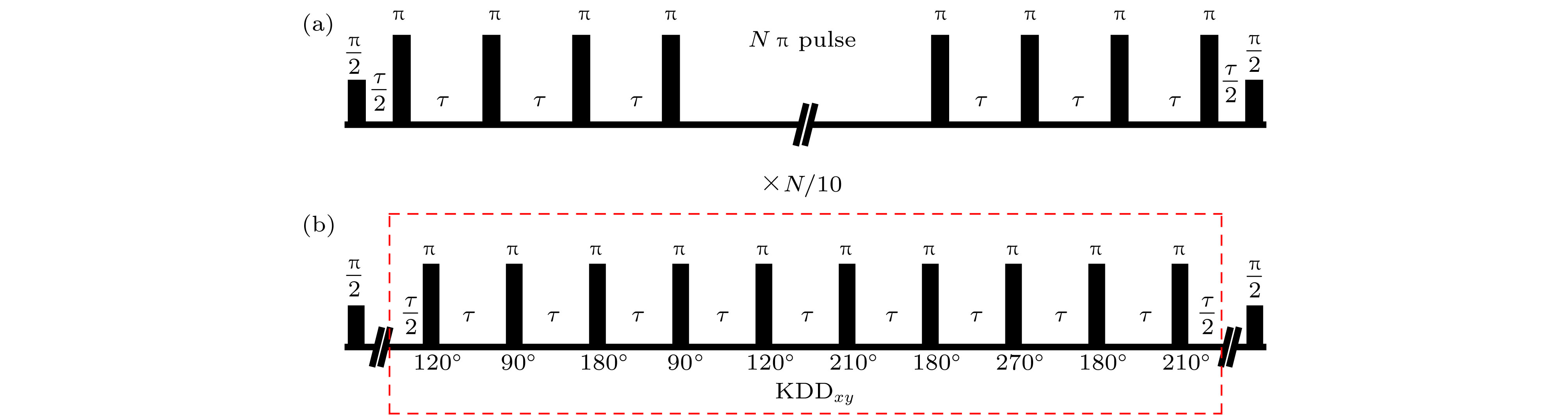
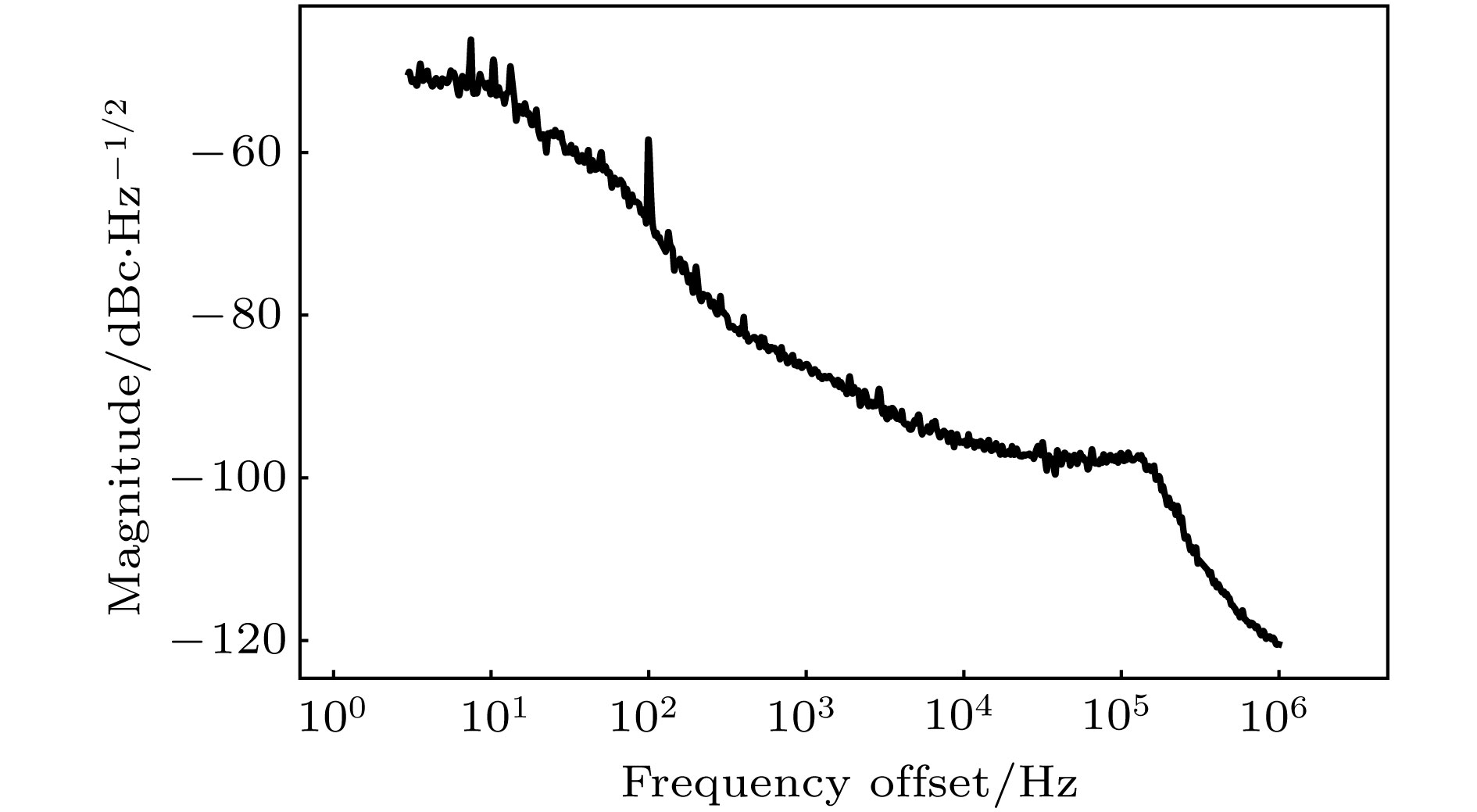
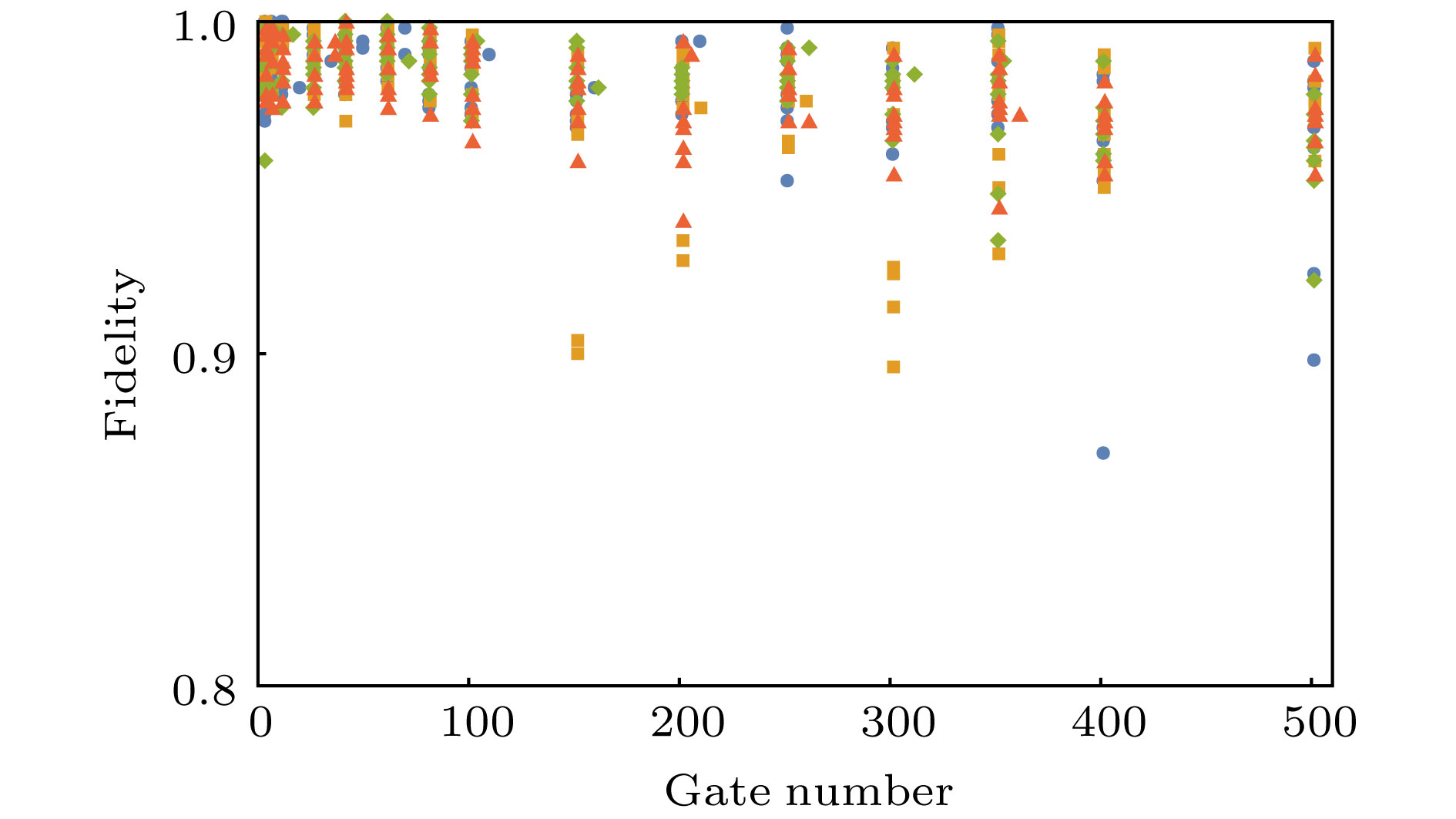
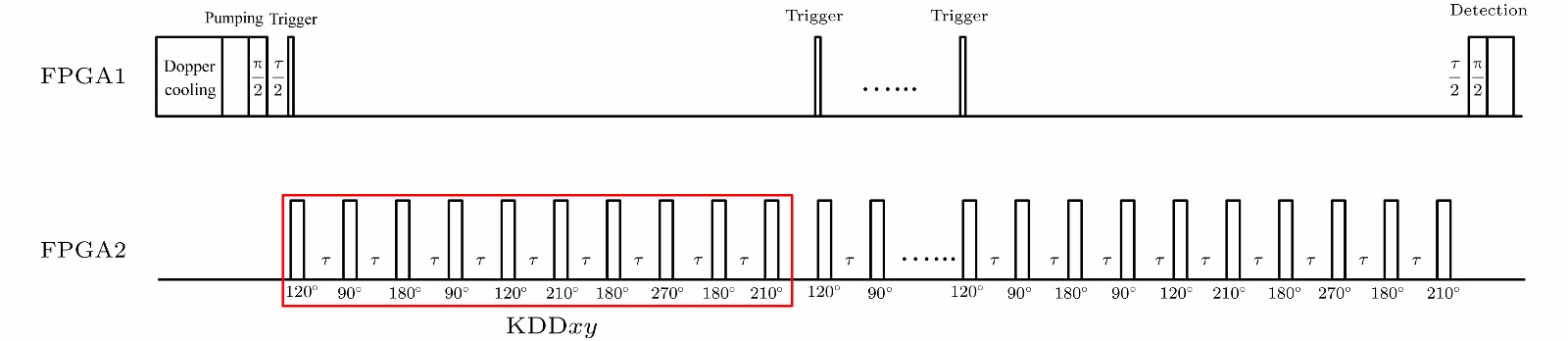
-
能够长时间储存量子信息的量子存储设备是实现大规模量子计算和量子通信的基本要素. 与其他量子计算平台相比, 囚禁离子系统的优势之一在于具有很长的相干时间. 此前, 基于囚禁离子的单量子比特相干时间不到1 min. 研究发现, 在囚禁离子系统中, 限制量子比特相干时间的主要因素是运动能级加热和环境噪声, 其中后者包含环境磁场涨落和微波相位噪声. 在同时囚禁171Yb+离子和138Ba+离子的混合囚禁系统中, 通过实施协同冷却和动力学解耦, 可以实现相干时间超过10 min的单离子量子比特. 这一技术有望用于实现量子密码学和搭建混合量子计算平台.Quantum memory device capable of storing quantum information for a long period of time is one of the fundamental ingredients to realize large-scale quantum computation and quantum communication. Comparing with other quantum computation platforms, one of the advantages of the trapped-ion system is the long intrinsic coherence time. Before our work, the longest single-qubit coherence time in trapped-ion systems has been achieved to be less than 1 minute. It is discovered that the main limitation for the coherence time is the motional mode heating and the environment noise that includes the contributions from the magnetic field fluctuation and the phase noise of the microwaves. In a hybrid trapping system simultaneously trapping 171Yb+ and 138Ba+ ions, single-qubit quantum memories with coherence time longer than 10 minutes can be realized by applying sympathetic cooling and dynamical decoupling. This technique may have some value as the building blocks for quantum cryptography protocols and hybrid quantum computation platforms.
-
Keywords:
- quantum memory /
- trapped ions /
- sympathetic cooling /
- dynamical decoupling
[1] Feynman R P 1982 Int. J. Theor. Phys. 21 467
Google Scholar
[2] Ladd T D, Jelezko F, Laflamme R, Nakamura Y, Monroe C, O’Brien J L 2010 Nature 464 45
Google Scholar
[3] Duan L M, Monroe C 2010 Rev. Mod. Phys. 82 1209
Google Scholar
[4] Wiesner S 1983 ACM SIGACT News 15 78
[5] Pastawski F, Yao N Y, Jiang L, Lukin M D, Cirac J I 2012 Proc. Natl. Acad. Sci. U.S.A. 109 16079
Google Scholar
[6] Nickerson N H, Fitzsimons J F, Benjamin S C 2014 Phys. Rev. X 4 041041
Google Scholar
[7] Kielpinski D, Monroe C, Wineland D J 2002 Nature 417 709
Google Scholar
[8] Blinov B B, Moehring D L, Duan L M, Monroe C 2004 Nature 428 153
Google Scholar
[9] Moehring D L, Maunz P, Olmschenk S, Younge K C, Matsukevich D N, Duan L M, Monroe C 2007 Nature 449 68
Google Scholar
[10] Monroe C, Kim J 2013 Science 339 1164
Google Scholar
[11] Bollinger J, Heizen D, Itano W, et al. 1991 IEEE Trans. Instrum. Meas. 40 126
Google Scholar
[12] Fisk P, Sellars M, Lawn M, et al. 1995 IEEE Trans. Instrum. Meas. 44 113
Google Scholar
[13] Langer C, Ozeri R, Jost J, et al. 2005 Phys. Rev. Lett. 95 060502
Google Scholar
[14] Häffner H, Schmidt-Kaler F, Hänsel W, et al. 2005 Appl. Phys. B 81 151
Google Scholar
[15] Harty T P, Allcock D T C, Ballance C J, et al. 2014 Phys. Rev. Lett. 113 220501
Google Scholar
[16] Epstein R J, Seidelin S, Leibfried D, et al. 2007 Phys. Rev. A 76 033411
Google Scholar
[17] Wesenberg J, Epstein R, Leibfried D, et al. 2007 Phys. Rev. A 76 053416
Google Scholar
[18] Hite D A, Colombe Y, Wilson A C, et al. 2012 Phys. Rev. Lett. 109 103001
Google Scholar
[19] Deslauriers L, Olmschenk S, Stick D, et al. 2006 Phys. Rev. Lett. 97 103007
Google Scholar
[20] Biercuk M J, Uys H, VanDevender A P, et al. 2009 Nature 458 996
Google Scholar
[21] Souza A M, Álvarez G A, Suter D 2011 Phys. Rev. Lett. 106 240501
Google Scholar
[22] Peng X H, Suter D, Lidar D A 2011 J. Phys. B 44 154003
Google Scholar
[23] Saeedi K, Simmons S, Salvail J Z, et al. 2013 Science 342 830
Google Scholar
[24] Zhong M J, Hedges M P, Ahlefeldt R L, et al. 2015 Nature 517 177
Google Scholar
[25] Khodjasteh K, Sastrawan J, Hayes D, et al. 2013 Nat. Commun. 4 2045
Google Scholar
[26] Soare A, Ball H, Hayes D, et al. 2014 Nat. Phys. 10 825
Google Scholar
[27] Cywiński L, Lutchyn R M, Nave C P, Das Sarma S 2008 Phys. Rev. B 77 174509
Google Scholar
[28] Uhrig G S 2008 New J. Phys. 10 083024
Google Scholar
[29] Pasini S, Uhrig G S 2010 Phys. Rev. A 81 012309
Google Scholar
[30] Viola L, Lloyd S 1998 Phys. Rev. A 58 2733
Google Scholar
[31] Hahn E L 1950 Phys. Rev. 94 580
[32] Carr H Y, Purcell E M 1954 Phys. Rev. 94 630
Google Scholar
[33] Meiboom S, Gill D 1958 Rev. Sci. Instrum. 29 688
Google Scholar
[34] Uhrig G S 2007 Phys. Rev. Lett. 98 100504
Google Scholar
[35] Brown K R, Harrow A W, Chuang I L 2004 Phys. Rev. A 70 052318
Google Scholar
[36] Ryan C A, Hodges J S, Cory D G 2010 Phys. Rev. Lett. 105 200402
Google Scholar
[37] Kotler S, Akerman N, Glickman Y, Ozeri R 2013 Phys. Rev. Lett. 110 110503
Google Scholar
[38] Kotler S, Akerman N, Glickman Y, Keselman A, Ozeri R 2011 Nature 473 61
Google Scholar
[39] Knill E, Leibfried D, Reichle R, et al. 2008 Phys. Rev. A 77 012307
Google Scholar
[40] Wang Y, Um M, Zhang J H, et al. 2017 Nature Photon. 11 646
Google Scholar
[41] Kielpinski D, Kafri D, Wooley M J, et al. 2012 Phys. Rev. Lett. 108 130504
Google Scholar
[42] Daniilidis N, Gorman D J, Tian L, Häffner H 2013 New J. Phys. 15 073017
Google Scholar
-
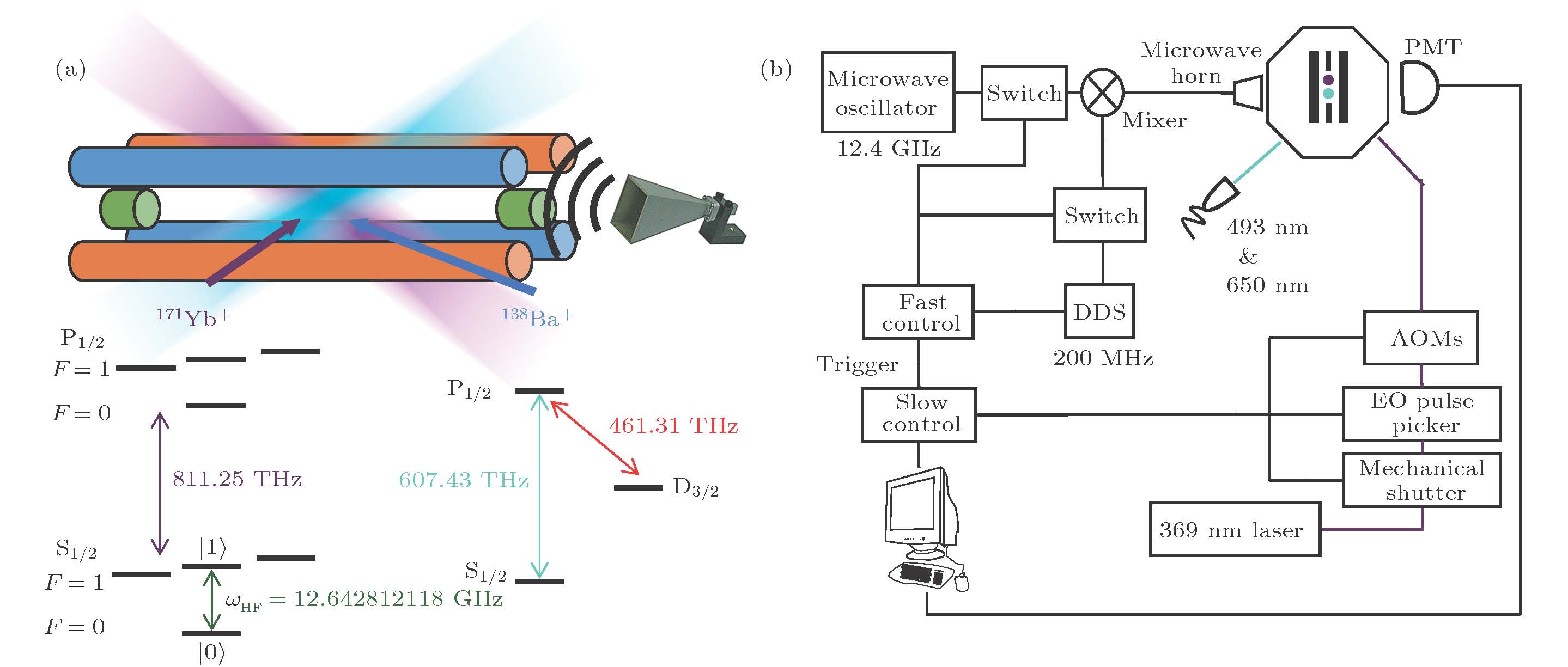
图 1 实验装置和控制系统 (a) 同时囚禁
${{}^{171}{\rm Yb}^+ }$ 离子和$ ^{138}{\rm Ba}^+ $ 离子的混合囚禁系统及相关能谱图; (b) 微波和激光信号的控制系统Fig. 1. Experimental setup and control system: (a) Hybrid trapping system that traps
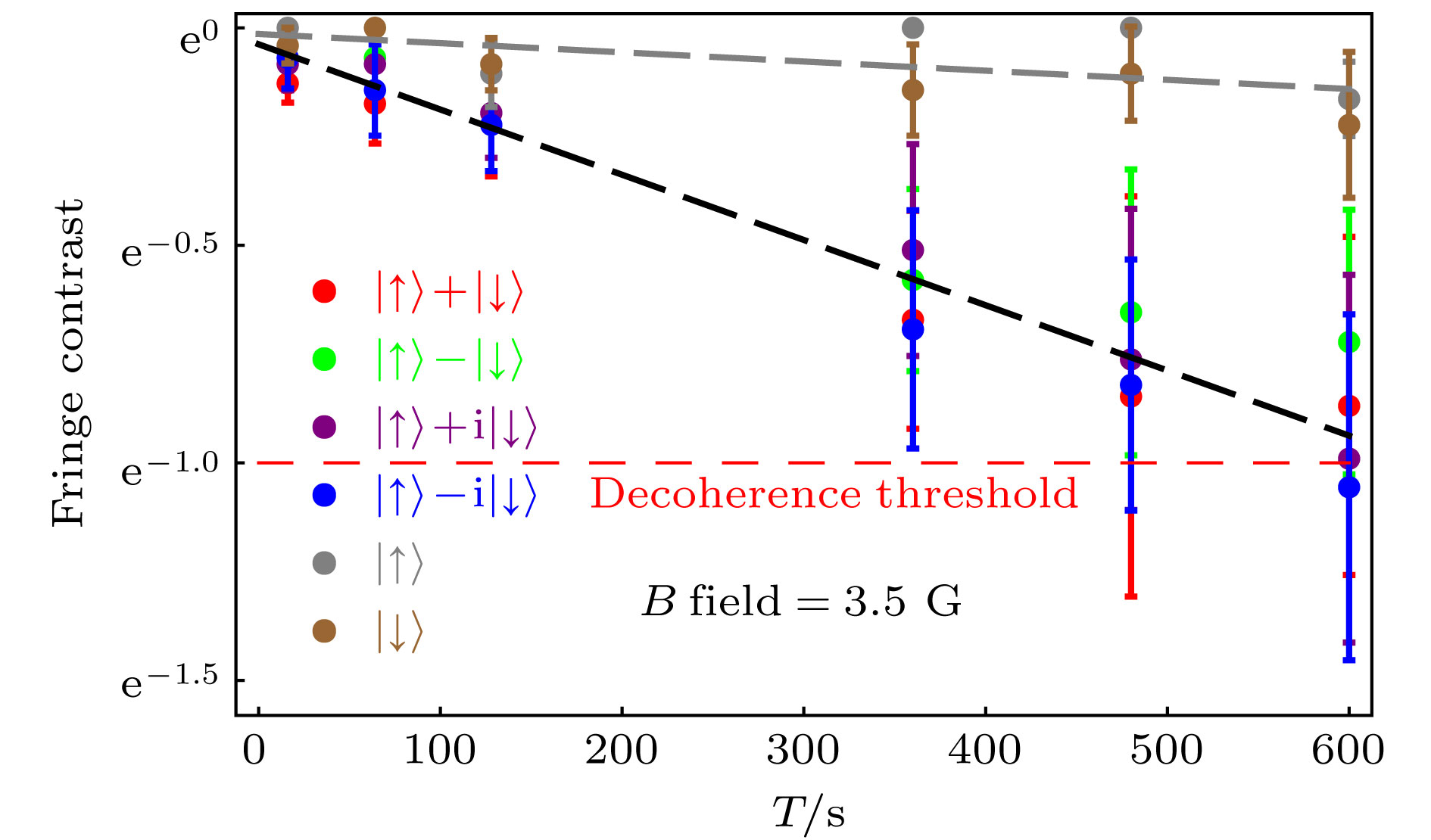
$ ^{171}{\rm Yb}^+ $ and$ ^{138}{\rm Ba}^+ $ simultaneously; (b) control system for generating laser and microwave signals.
图 2 动力学解耦脉冲序列 (a) CPMG方案; (b)
$ {\rm KDD}_{xy} $ 方案Fig. 2. Pulse sequence for dyanmical decoupling: (a) CPMG protocol; (b)
${\rm{KD}}{{\rm{D}}_{{xy}}}$ protocol.
图 4 (a) 执行包含
$ N $ 个脉冲的动力学解耦序列, 不同的总演化时间$ T $ 对应的条纹对比度; (b) 通过分析图(a)中的数据得到的环境噪声谱Fig. 4. (a) Ramsey contrasts depending on the total evolution time T for various numbers of pulses N in the dynamical decoupling sequence; (b) the noise spectra analized from the measured data in Fig. (a).
图 5 序列保真度随序列长度的变化
Fig. 5. Sequence fidelity pl as a function of the sequence length l.
图 6 测量单量子比特相干时间的脉冲序列
Fig. 6. Pulse sequences for measuring the single-qubit coherent time.
图 7 单量子比特六个不同初态的相干时间, 其中
$\left| \uparrow \right\rangle $ 和$\left| \downarrow \right\rangle $ , 对应的相干时间是(4740$ \pm $ 1760) s; 其他四个初态对应的相干时间为(667$ \pm $ 17) s; 图中的误差线代表标准差Fig. 7. Single-qubit coherece time for six different initial states. For
$\left| \uparrow \right\rangle $ and$\left| \downarrow \right\rangle $ , the coherence time is (4740$ \pm $ 1760) s. For the other four initial states, the coherence time is (667$ \pm $ 17) s. The error bars are the standard deviation. -
[1] Feynman R P 1982 Int. J. Theor. Phys. 21 467
Google Scholar
[2] Ladd T D, Jelezko F, Laflamme R, Nakamura Y, Monroe C, O’Brien J L 2010 Nature 464 45
Google Scholar
[3] Duan L M, Monroe C 2010 Rev. Mod. Phys. 82 1209
Google Scholar
[4] Wiesner S 1983 ACM SIGACT News 15 78
[5] Pastawski F, Yao N Y, Jiang L, Lukin M D, Cirac J I 2012 Proc. Natl. Acad. Sci. U.S.A. 109 16079
Google Scholar
[6] Nickerson N H, Fitzsimons J F, Benjamin S C 2014 Phys. Rev. X 4 041041
Google Scholar
[7] Kielpinski D, Monroe C, Wineland D J 2002 Nature 417 709
Google Scholar
[8] Blinov B B, Moehring D L, Duan L M, Monroe C 2004 Nature 428 153
Google Scholar
[9] Moehring D L, Maunz P, Olmschenk S, Younge K C, Matsukevich D N, Duan L M, Monroe C 2007 Nature 449 68
Google Scholar
[10] Monroe C, Kim J 2013 Science 339 1164
Google Scholar
[11] Bollinger J, Heizen D, Itano W, et al. 1991 IEEE Trans. Instrum. Meas. 40 126
Google Scholar
[12] Fisk P, Sellars M, Lawn M, et al. 1995 IEEE Trans. Instrum. Meas. 44 113
Google Scholar
[13] Langer C, Ozeri R, Jost J, et al. 2005 Phys. Rev. Lett. 95 060502
Google Scholar
[14] Häffner H, Schmidt-Kaler F, Hänsel W, et al. 2005 Appl. Phys. B 81 151
Google Scholar
[15] Harty T P, Allcock D T C, Ballance C J, et al. 2014 Phys. Rev. Lett. 113 220501
Google Scholar
[16] Epstein R J, Seidelin S, Leibfried D, et al. 2007 Phys. Rev. A 76 033411
Google Scholar
[17] Wesenberg J, Epstein R, Leibfried D, et al. 2007 Phys. Rev. A 76 053416
Google Scholar
[18] Hite D A, Colombe Y, Wilson A C, et al. 2012 Phys. Rev. Lett. 109 103001
Google Scholar
[19] Deslauriers L, Olmschenk S, Stick D, et al. 2006 Phys. Rev. Lett. 97 103007
Google Scholar
[20] Biercuk M J, Uys H, VanDevender A P, et al. 2009 Nature 458 996
Google Scholar
[21] Souza A M, Álvarez G A, Suter D 2011 Phys. Rev. Lett. 106 240501
Google Scholar
[22] Peng X H, Suter D, Lidar D A 2011 J. Phys. B 44 154003
Google Scholar
[23] Saeedi K, Simmons S, Salvail J Z, et al. 2013 Science 342 830
Google Scholar
[24] Zhong M J, Hedges M P, Ahlefeldt R L, et al. 2015 Nature 517 177
Google Scholar
[25] Khodjasteh K, Sastrawan J, Hayes D, et al. 2013 Nat. Commun. 4 2045
Google Scholar
[26] Soare A, Ball H, Hayes D, et al. 2014 Nat. Phys. 10 825
Google Scholar
[27] Cywiński L, Lutchyn R M, Nave C P, Das Sarma S 2008 Phys. Rev. B 77 174509
Google Scholar
[28] Uhrig G S 2008 New J. Phys. 10 083024
Google Scholar
[29] Pasini S, Uhrig G S 2010 Phys. Rev. A 81 012309
Google Scholar
[30] Viola L, Lloyd S 1998 Phys. Rev. A 58 2733
Google Scholar
[31] Hahn E L 1950 Phys. Rev. 94 580
[32] Carr H Y, Purcell E M 1954 Phys. Rev. 94 630
Google Scholar
[33] Meiboom S, Gill D 1958 Rev. Sci. Instrum. 29 688
Google Scholar
[34] Uhrig G S 2007 Phys. Rev. Lett. 98 100504
Google Scholar
[35] Brown K R, Harrow A W, Chuang I L 2004 Phys. Rev. A 70 052318
Google Scholar
[36] Ryan C A, Hodges J S, Cory D G 2010 Phys. Rev. Lett. 105 200402
Google Scholar
[37] Kotler S, Akerman N, Glickman Y, Ozeri R 2013 Phys. Rev. Lett. 110 110503
Google Scholar
[38] Kotler S, Akerman N, Glickman Y, Keselman A, Ozeri R 2011 Nature 473 61
Google Scholar
[39] Knill E, Leibfried D, Reichle R, et al. 2008 Phys. Rev. A 77 012307
Google Scholar
[40] Wang Y, Um M, Zhang J H, et al. 2017 Nature Photon. 11 646
Google Scholar
[41] Kielpinski D, Kafri D, Wooley M J, et al. 2012 Phys. Rev. Lett. 108 130504
Google Scholar
[42] Daniilidis N, Gorman D J, Tian L, Häffner H 2013 New J. Phys. 15 073017
Google Scholar
下载:
计量
- 文章访问数: 9208
- PDF下载量: 165
- 被引次数: 0
