-
本文从量子几何的角度研究多能级系统布居转移的优化控制. 首先, 建立基于动力学量子几何张量对受激拉曼绝热通道(STIRAP)方案进行优化设计的一般理论框架. 然后, 以具有单光子失谐的$ {{\Lambda }} $型三能级系统和三脚架型四能级系统为例, 分别计算了体系的动力学量子几何张量和非绝热跃迁率, 研究系统的布居转移动力学. 此外, 还讨论了拉比脉冲工作时间、幅度涨落以及单光子失谐等参数对转移过程的影响, 揭示了系统的绝热共振转移现象. 研究发现, 利用动力学量子几何张量优化的STIRAP方案比传统的STIRAP方案具有更快更高效的布居转移.The optimal control of population transfer for multi-level systems is investigated from the perspective of quantum geometry. Firstly, the general theoretical framework of optimizing the STIRAP scheme based on the dynamical quantum geometric tensor is given, and then the dynamical quantum geometric tensor and the nonadiabatic transition rate are calculated by taking the detuned $ {{\Lambda }} $-type three-level system and tripod-type four-level system for example. Secondly, the transfer dynamics of the particle population of the system are investigated in detail. For a three-level system, the optimal STIRAP scheme has an efficiency of over 98% in transferring the population to the state $ \left|3\right.\rangle $, while the transfer efficiency of traditional STIRAP is about 72%. The superposition states with arbitrary proportions can be efficiently prepared for a four-level system due to the decoupling of the degenerate dark states. Finally, the influences of system parameters, such as the operating time of the Rabi pulses, the amplitude fluctuation and the single-photon detuning, on the transfer process are discussed. Especially, the phenomena of the adiabatic resonance transfer are revealed. Choosing the pulse parameters in the resonance window can reduce the infidelity of the population transfer to below 10–3. It is found that the optimal STIRAP scheme by the dynamical quantum geometric tensor provides faster and more efficient transfer than the traditional STIRAP scheme.
-
Keywords:
- stimulated Raman adiabatic passage /
- dynamical quantum geometric tensor /
- adiabatic population transfer /
- $ {{\Lambda }} $-type three-level system /
- tripod-type four-level system /
- dark states
[1] Born M, Fock V 1928 Zeitschrift für Physik 51 165
 Google Scholar
Google Scholar
[2] Wu Z, Yang H 2005 Phys. Rev. A 72 012114
 Google Scholar
Google Scholar
[3] Holonomy S B 1983 Phys. Rev. Lett. 51 2167
 Google Scholar
Google Scholar
[4] Xiao D, Chang M C, Niu Q 2010 Rev. Mod. Phys. 82 1959
 Google Scholar
Google Scholar
[5] Vitanov N V, Rangelov A A, Shore B W, Bergmann K 2017 Rev. Mod. Phys. 89 015006
 Google Scholar
Google Scholar
[6] Shore B W, Bergmann K, Oreg J, Rosenwaks S 1991 Phys. Rev. A 44 7442
 Google Scholar
Google Scholar
[7] Shore B W 2013 Acta Phys. Slovaca 63 361
 Google Scholar
Google Scholar
[8] 孙晓鹏, 冯志芳, 李卫东, 贾锁堂 2007 56 5727
 Google Scholar
Google Scholar
Sun X P, Feng Z F, Li W D, Jia S T 2007 Acta Phys. Sin. 56 5727
 Google Scholar
Google Scholar
[9] 孟少英, 吴炜, 刘彬 2009 58 6902
 Google Scholar
Google Scholar
Meng S Y, Wu W, Liu B 2009 Acta Phys. Sin. 58 6902
 Google Scholar
Google Scholar
[10] 李冠强, 彭娉 2011 60 110304
 Google Scholar
Google Scholar
Li G Q, Peng P 2011 Acta Phys. Sin. 60 110304
 Google Scholar
Google Scholar
[11] 李冠强, 彭娉, 曹振洲, 薛具奎 2012 61 090301
 Google Scholar
Google Scholar
Li G Q, Peng P, Cao Z Z, Xue J K 2012 Acta Phys. Sin. 61 090301
 Google Scholar
Google Scholar
[12] Bergmann K, Vitanov N V, Shore B W 2015 J. Chem. Phys. 142 170901
 Google Scholar
Google Scholar
[13] Fewell M P, Shore B W, Bergmann K 1997 Austra. J. Phys 50 281
 Google Scholar
Google Scholar
[14] Guéry-Odelin D, Ruschhaupt A, Kiely A, Torrontegui E, Martínez-Garaot S, Muga J G 2019 Rev. Mod. Phys. 91 045001
 Google Scholar
Google Scholar
[15] Hatomura T 2024 J. Phys. B: At. Mol. Opt. Phys. 57 102001
 Google Scholar
Google Scholar
[16] Chen X, Lizuain I, Ruschhaupt A, Guéry-Odelin D, Muga J G 2010 Phys. Rev. Letts. 105 123003
 Google Scholar
Google Scholar
[17] Minář Jí, Söyler Ş G, Rotondo P, Lesanovsky I 2017 New J. Phys. 19 063033
 Google Scholar
Google Scholar
[18] Ban Y, Chen X, Sherman E Y, Muga J G 2012 Phys. Rev. Letts. 109 206602
 Google Scholar
Google Scholar
[19] Opatrný T, Saberi H, Brion E, Mølmer K 2016 Phys. Rev. A 93 023815
 Google Scholar
Google Scholar
[20] Tian L 2012 Phys. Rev. Lett. 108 153604
 Google Scholar
Google Scholar
[21] Barrett S, Hammerer K, Harrison S, Northup T E, Osborne T J 2013 Phys. Rev. Lett. 110 090501
 Google Scholar
Google Scholar
[22] Yu X M, Zhou K, Zhang H Y, Li S X, Huang Z, Wen J, Zhang R, Yu Y 2025 Phys. Rev. A 111 012623
 Google Scholar
Google Scholar
[23] Masuda S 2012 Phys. Rev. A 86 063624
 Google Scholar
Google Scholar
[24] Masuda S, Güngördü U, Chen X, Ohmi T, Nakahara M 2016 Phys. Rev. A 93 013626
 Google Scholar
Google Scholar
[25] Demirplak M, Rice S A 2003 J. Phys. Chem. A 107 9937
 Google Scholar
Google Scholar
[26] Berry M V 2009 J. Phys. A: Math. Theor. 42 365303
 Google Scholar
Google Scholar
[27] Lewis H R, Riesenfeld W B 1969 J. Math. Phys. 10 1458
 Google Scholar
Google Scholar
[28] Chen X, Torrontegui E, Muga J G 2011 Phys. Rev. A 83 062116
 Google Scholar
Google Scholar
[29] Masuda S, Rice S A 2015 J. Phys. Chem. A 119 3479
 Google Scholar
Google Scholar
[30] Masuda S, Nakamura K 2008 Phys. Rev. A 78 062108
 Google Scholar
Google Scholar
[31] Torosov B T, Della Valle G, Longhi S 2014 Phys. Rev. A 89 063412
 Google Scholar
Google Scholar
[32] Torosov B T, Della Valle G, Longhi S 2013 Phys. Rev. A 87 052502
 Google Scholar
Google Scholar
[33] Li G Q, Chen G D, Peng P, Qi W 2017 Euro. Phys. J. D 71 14
 Google Scholar
Google Scholar
[34] Li K Z, Tian J Z, Xiao L T 2024 Phys. Rev. A 109 022443
 Google Scholar
Google Scholar
[35] Chen J F 2022 Phys. Rev. Res. 4 023252
 Google Scholar
Google Scholar
[36] Sun C P 1988 J. Phys. A: Math. Gen. 21 1595
 Google Scholar
Google Scholar
[37] Rigolin G, Ortiz G, Ponce V H 2008 Phys. Rev. A 78 052508
 Google Scholar
Google Scholar
[38] Chen J F, Sun C P, Dong H 2019 Phys. Rev. E 100 062140
 Google Scholar
Google Scholar
[39] Oh S, Shim Y P, Fei J, et al. 2013 Phys. Rev. A 87 022332
 Google Scholar
Google Scholar
[40] Gaubatz U, Rudecki P, Schiemann S, Bergmann K 1990 J. Chem. Phys. 92 5363
 Google Scholar
Google Scholar
[41] Unanyan R G, Shore B W, Bergmann K 2001 Phys. Rev. A 63 043401
 Google Scholar
Google Scholar
[42] Vitanov N V 1998 Phys. Rev. A 58 2295
 Google Scholar
Google Scholar
[43] Vitanov N V, Halfmann T, Shore B W, Bergmann K 2001 Ann. Rev. Phys. Chem. 52 763
 Google Scholar
Google Scholar
[44] Unanyan R, Fleischhauer M, Shore B W, Bergmann K 1998 Opt. Commun. 155 144
 Google Scholar
Google Scholar
[45] Madasu C S, Rathod K D, Kwong C C, Wilkowski D 2024 Phys. Rev. Appl. 21 L051001
 Google Scholar
Google Scholar
[46] Shi Z C, Wang J H, Zhang C, Song J, Xia Y 2024 Phys. Rev. A 109 022441
 Google Scholar
Google Scholar
[47] Jin Z Y, Jing J 2025 Phys. Rev. A 111 022628
 Google Scholar
Google Scholar
[48] Li G Q, Guo H, Zhang Y Q, Yang B, Peng P 2025 Commun. Theor. Phys. 77 015103
 Google Scholar
Google Scholar
-
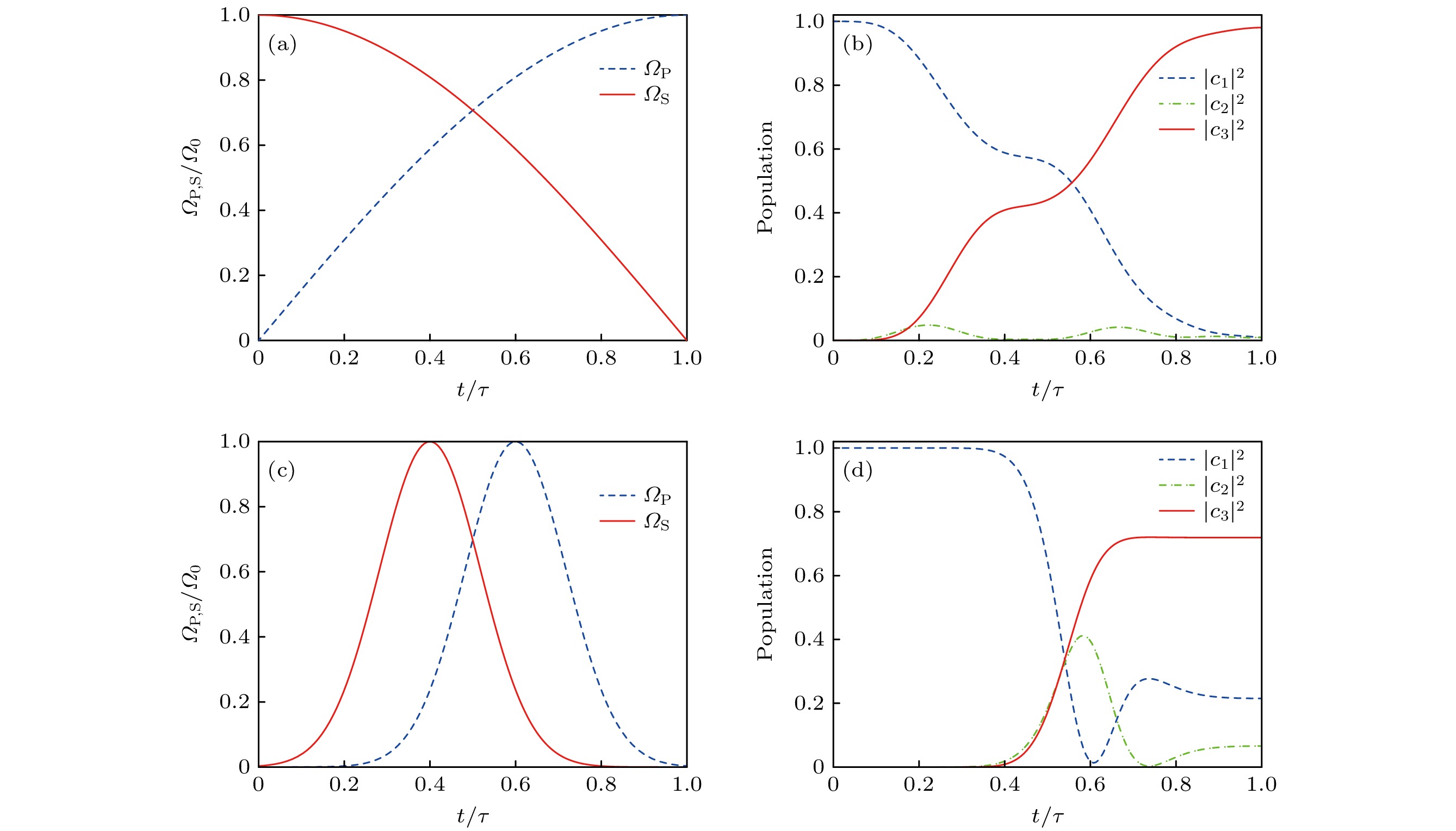
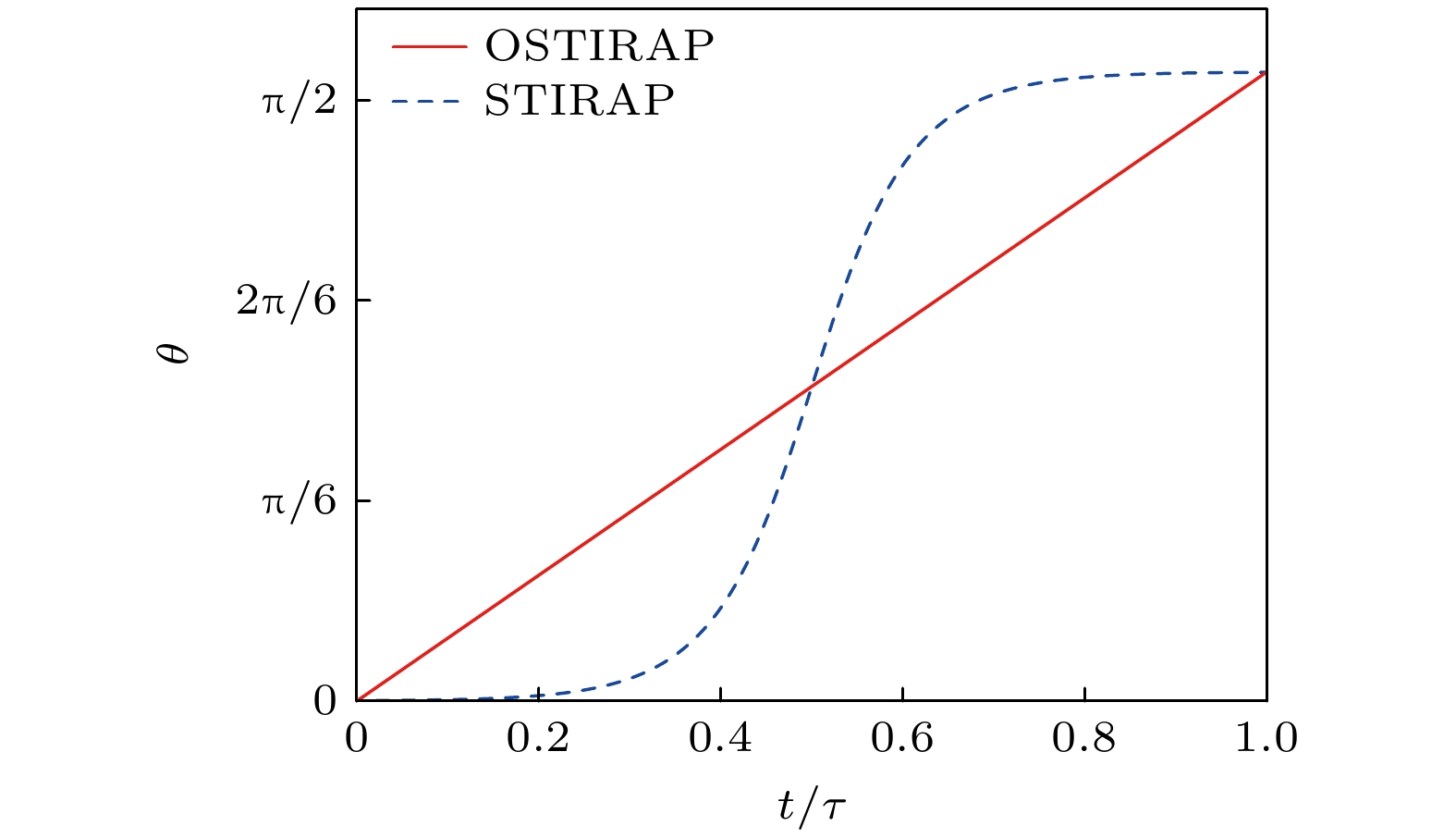
图 2 三能级系统拉比脉冲结构与粒子数布居的演化结果 (a)优化STIRAP脉冲结构; (b)优化STIRAP粒子数布居的演化结果; (c)传统STIRAP脉冲结构; (d)传统STIRAP粒子数布居的演化结果. 脉冲工作时间$ \tau =4{\mathrm{ }}\;{\text{μ}}{\mathrm{s}} $, 脉冲峰值$ {\varOmega }_{0}=30.79\;{\mathrm{ }}{\mathrm{M}}{\mathrm{H}}{\mathrm{z}} $, 失谐量$ \varDelta =2{\mathrm{\pi }}\;{\mathrm{M}}{\mathrm{H}}{\mathrm{z}} $
Fig. 2. Rabi pulse’s structures and the evolution results of the populations for the three-level system: (a) Pulse structures for the optimal STIRAP; (b) evolution of the populations for the optimal STIRAP; (c) pulse structures for the standard STIRAP; (d) evolution of the populations for the standard STIRAP. The pulse operating time $ \tau =4\;{\mathrm{ }}{\text{μ}}{\mathrm{s}} $, the pulse peak $ {\varOmega }_{0}=30.79{\mathrm{ }}\;{\mathrm{M}}{\mathrm{H}}{\mathrm{z}} $, and the detuning $ \varDelta =2{\mathrm{\pi }}\;{\mathrm{ }}{\mathrm{M}}{\mathrm{H}}{\mathrm{z}} $
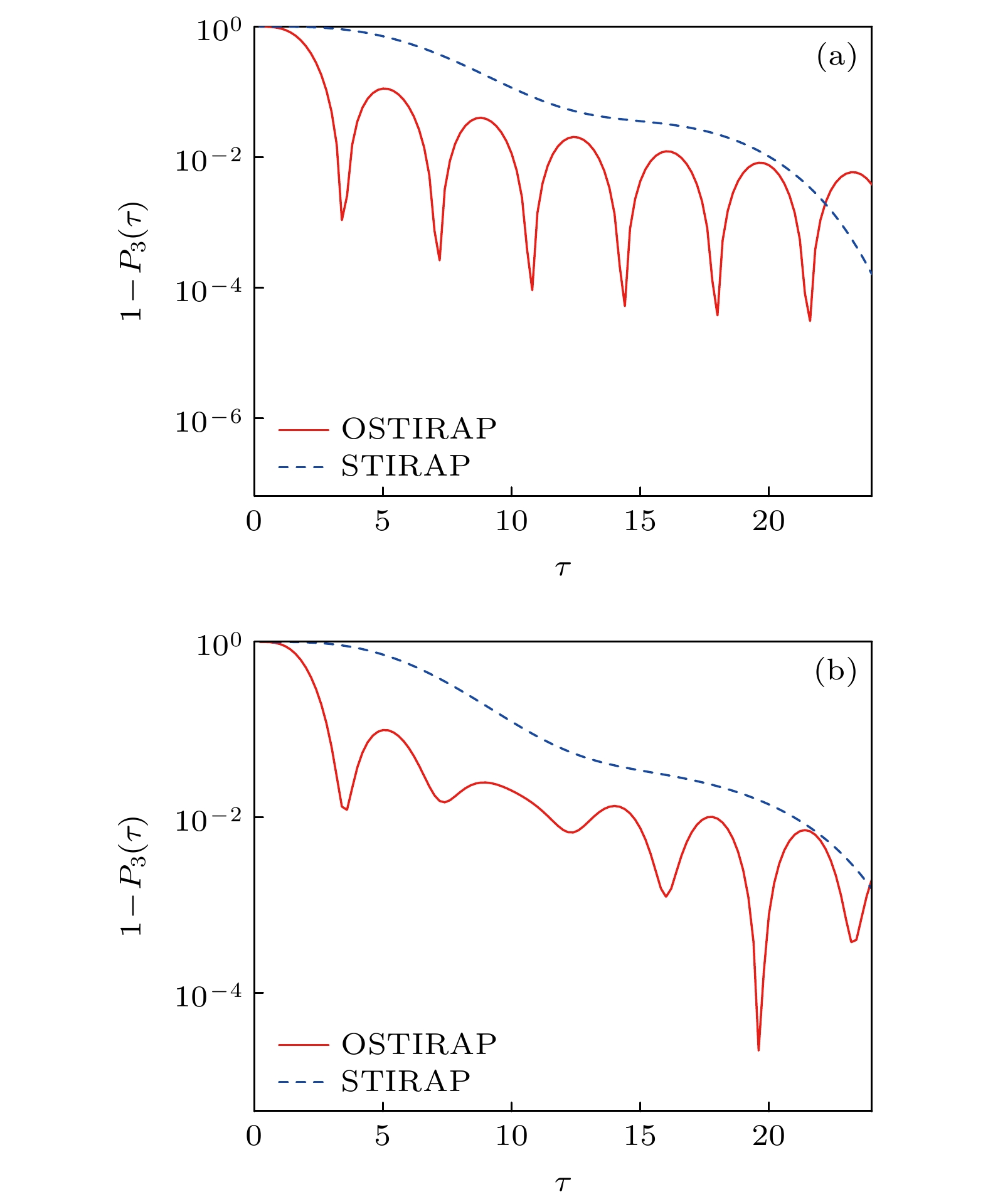
图 4 三能级系统失真度随工作时间的变化 (a)不存在失谐的情况($ \varDelta =0 $); (b)存在失谐的情况($ \varDelta =2{\mathrm{\pi }}\;{\mathrm{ }}{\mathrm{M}}{\mathrm{H}}{\mathrm{z}}) $. 红色实线表示优化STIRAP, 蓝色虚线表示传统STIRAP; 脉冲峰值$ {\varOmega }_{0}=35{\mathrm{ }}\;{\mathrm{M}}{\mathrm{H}}{\mathrm{z}} $
Fig. 4. Change of the infidelity with time for the three-level system: (a) The case without detuning ($ \varDelta =0) $; (b) the case with detuning ($ \varDelta =2{\mathrm{\pi }}{\mathrm{ }}\;{\mathrm{M}}{\mathrm{H}}{\mathrm{z}} $). The red solid line corresponds to the optimal STIRAP scheme and the blue dashed line corresponds to the standard STIRAP one. The pulse peak $ {\varOmega }_{0}=35\;{\mathrm{ }}{\mathrm{M}}{\mathrm{H}}{\mathrm{z}} $.
图 5 三能级系统失真度随脉冲峰值涨落的变化 (a)不存在失谐的情况($ \varDelta =0 $); (b)存在失谐的情况($ \varDelta =2{\mathrm{\pi }}\;{\mathrm{ }}{\mathrm{M}}{\mathrm{H}}{\mathrm{z}} $). 红色实线表示优化STIRAP, 蓝色虚线表示传统STIRAP; 脉冲峰值$ {\varOmega }_{0}=35{\mathrm{ }}\;{\mathrm{M}}{\mathrm{H}}{\mathrm{z}} $, 工作时间τ = 7.4 μs
Fig. 5. Change of the infidelity with the fluctuation of the pulse peak for the three-level system: (a) The case without detuning ($ \varDelta =0 $); (b) the case with detuning ($ \varDelta = $$ 2{\mathrm{\pi }}\;{\mathrm{ }}{\mathrm{M}}{\mathrm{H}}{\mathrm{z}} $). The red solid line denotes the optimal STIRAP scheme and the blue dashed line denotes the standard STIRAP one. The pulse peak $ {\varOmega }_{0}=35{\mathrm{ }}\;{\mathrm{M}}{\mathrm{H}}{\mathrm{z}} $ and the operating time τ = 7.4 μs.
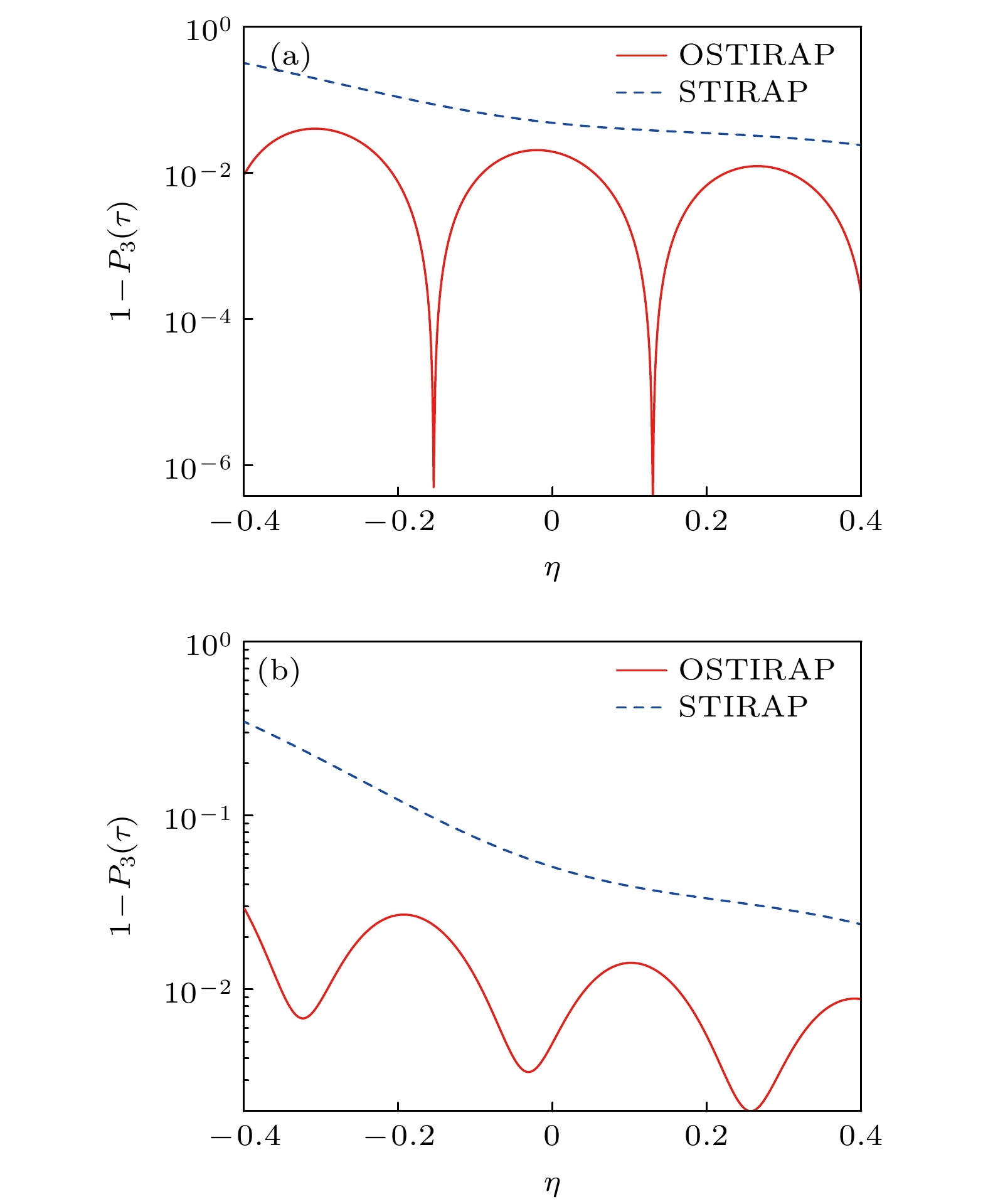
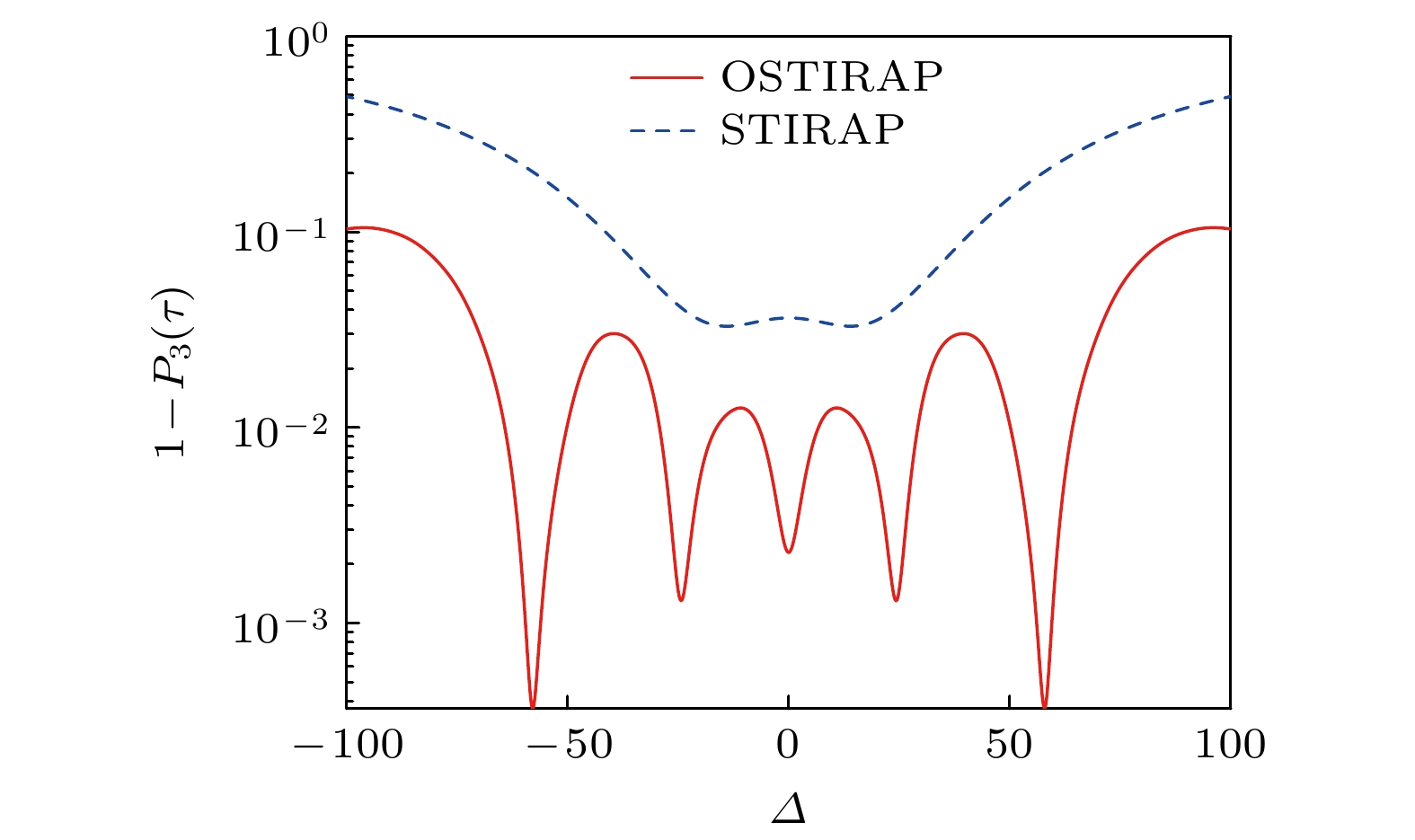
图 6 三能级系统失真度随单光子失谐量的变化. 红色实线表示优化STIRAP, 蓝色虚线表示传统STIRAP; 脉冲峰值$ {\varOmega }_{0}=35{\mathrm{ }}\;{\mathrm{M}}{\mathrm{H}}{\mathrm{z}} $, 脉冲工作时间τ = 7.4 μs
Fig. 6. Change of the infidelity with single-photon detuning for the three-level system. The red solid line denotes the optimal STIRAP scheme and the blue dashed line denotes the standard STIRAP one. The pulse peak $ {\varOmega }_{0}=35\;{\mathrm{ }}{\mathrm{M}}{\mathrm{H}}{\mathrm{z}} $ and the pulse operating time τ = 7.4 μs.
图 8 四能级系统拉比脉冲结构与粒子布居数的演化结果 (a)优化STIRAP脉冲结构; (b)优化STIRAP粒子布居数的演化结果; (c)传统STIRAP脉冲结构; (d)传统STIRAP粒子布居数的演化结果. 工作时间$ \tau =4{\mathrm{ }}\;{\text{μ}}{\mathrm{s}} $, 脉冲峰值$ {\varOmega }_{0}=35\;{\mathrm{ }}{\mathrm{M}}{\mathrm{H}}{\mathrm{z}} $, 失谐量$ \varDelta =2{\mathrm{\pi }}\;{\mathrm{M}}{\mathrm{H}}{\mathrm{z}} $
Fig. 8. Rabi pulse’s structures and the evolution results of the populations for the four-level system: (a) Pulse structures for the optimal STIRAP; (b) evolution of the populations for the optimal STIRAP; (c) pulse structures for the standard STIRAP; (d) evolution of the populations for the standard STIRAP. The pulse operating time $ \tau =4{\mathrm{ }}\;{\text{μ}}{\mathrm{s}} $, the pulse peak $ {\varOmega }_{0}=35\;{\mathrm{M}}{\mathrm{H}}{\mathrm{z}} $, and the detuning $ \varDelta =2{\mathrm{\pi }}\;{\mathrm{M}}{\mathrm{H}}{\mathrm{z}} $.
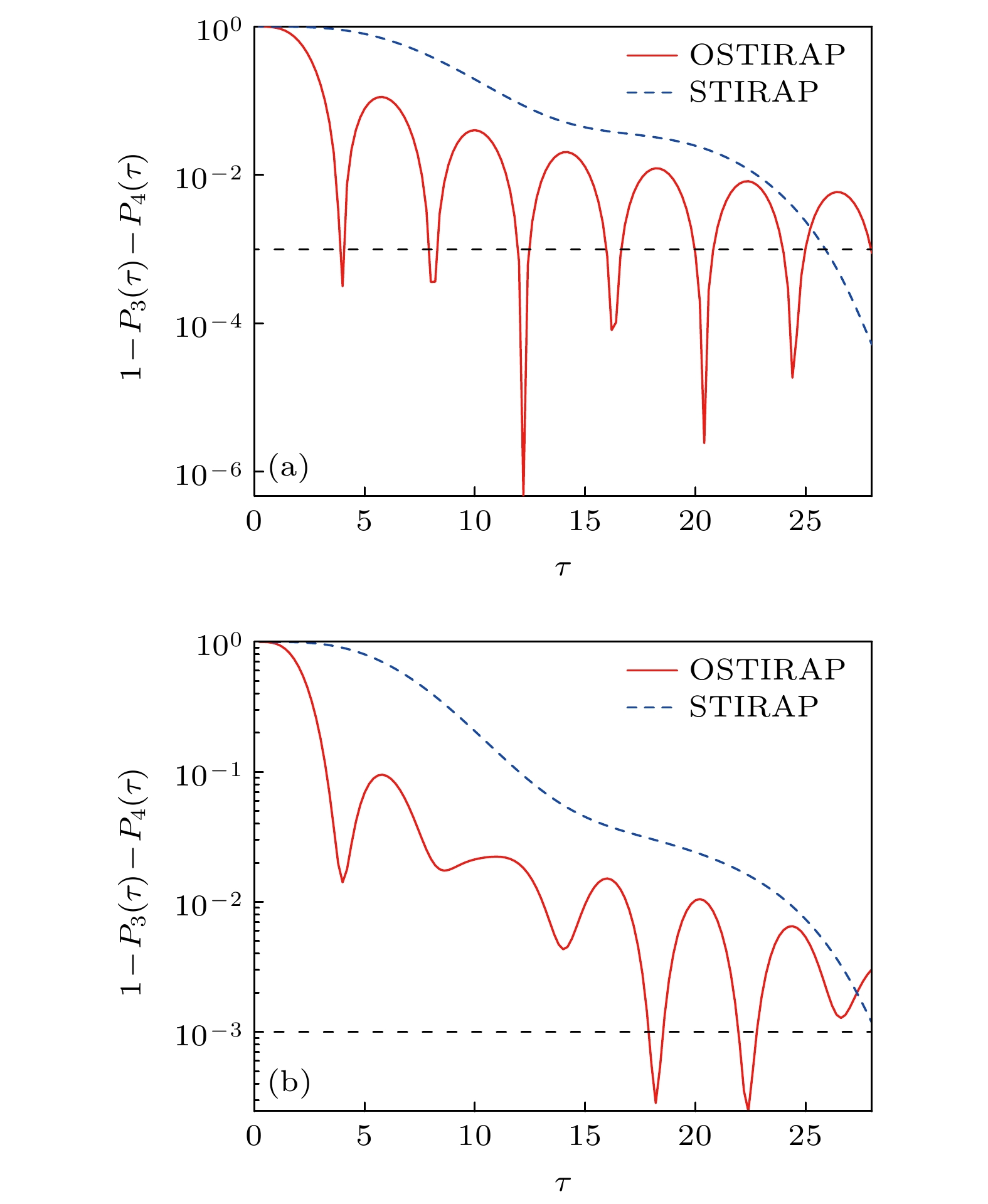
图 9 四能级系统失真度随工作时间的变化情况 (a)不存在失谐的情况($ \varDelta =0 $); (b)存在失谐的情况($ \varDelta =2{\mathrm{\pi }}\;{\mathrm{M}}{\mathrm{H}}{\mathrm{z}} $). 红色实线表示优化STIRAP, 蓝色虚线表示传统STIRAP; 脉冲峰值$ {\varOmega }_{0}=35\;{\mathrm{M}}{\mathrm{H}}{\mathrm{z}} $
Fig. 9. Change of the infidelity with time for the four-level system: (a) The case without detuning ($ \varDelta =0) $; (b) the case with detuning ($ \varDelta =2{\mathrm{\pi }}\;{\mathrm{M}}{\mathrm{H}}{\mathrm{z}} $). The red solid line corresponds to the optimal STIRAP scheme and the blue dashed line corresponds to the standard STIRAP one. The pulse peak $ {\varOmega }_{0}=35\;{\mathrm{ }}{\mathrm{M}}{\mathrm{H}}{\mathrm{z}} $.
图 10 四能级系统失真度随脉冲峰值涨落的变化 (a)不存在失谐的情况($ \varDelta =0 $); (b)存在失谐的情况($ \varDelta =2{\mathrm{\pi }}\;{\mathrm{ }}{\mathrm{M}}{\mathrm{H}}{\mathrm{z}} $). 红色实线表示优化STIRAP, 蓝色虚线表示传统STIRAP; 脉冲峰值$ {\varOmega }_{0}=35{\mathrm{ }}\;{\mathrm{M}}{\mathrm{H}}{\mathrm{z}} $, 脉冲工作时间τ = 7.4 μs
Fig. 10. Change of the infidelity with the fluctuation of the pulse peak for the four-level system: (a) The case without detuning ($ \varDelta =0 $); (b) the case with detuning ($ \varDelta = $$ 2{\mathrm{\pi }}\;{\mathrm{ }}{\mathrm{M}}{\mathrm{H}}{\mathrm{z}} $). The red solid line denotes the optimal STIRAP scheme and the blue dashed line denotes the standard STIRAP one. The pulse peak $ {\varOmega }_{0}=35{\mathrm{ }}\;{\mathrm{M}}{\mathrm{H}}{\mathrm{z}} $ and the operating time τ = 7.4 μs
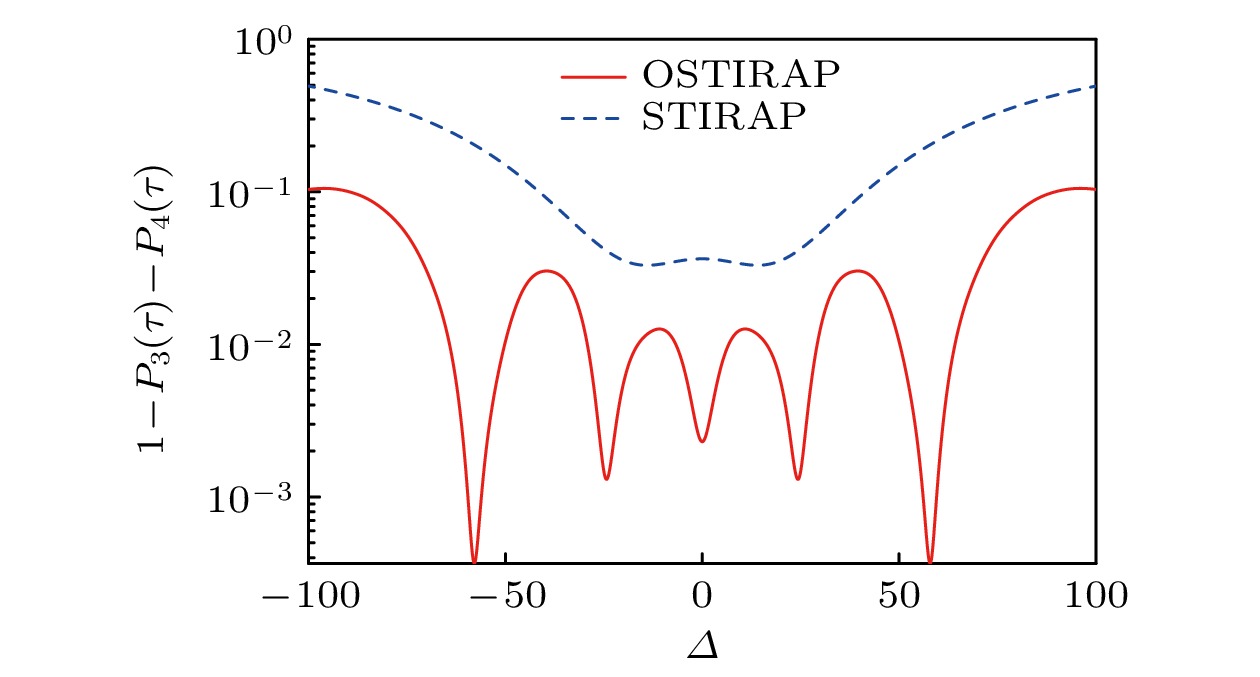
图 11 四能级系统失真度随失谐量$ {\mathrm{\varDelta }} $的变化. 红色实线为最优STIRAP, 蓝色虚线为传统STIRAP; 脉冲峰值$ {\varOmega }_{0}= $$ 35\;{\mathrm{M}}{\mathrm{H}}{\mathrm{z}} $, 脉冲工作时间τ = 7.4 μs
Fig. 11. Change of the infidelity with single-photon detuning for the four-level system. The red solid line denotes the optimal STIRAP scheme and the blue dashed line denotes the standard STIRAP one. The pulse peak $ {\varOmega }_{0}=35\;{\mathrm{M}}{\mathrm{H}}{\mathrm{z}} $ and the pulse operating time τ = 7.4 μs.
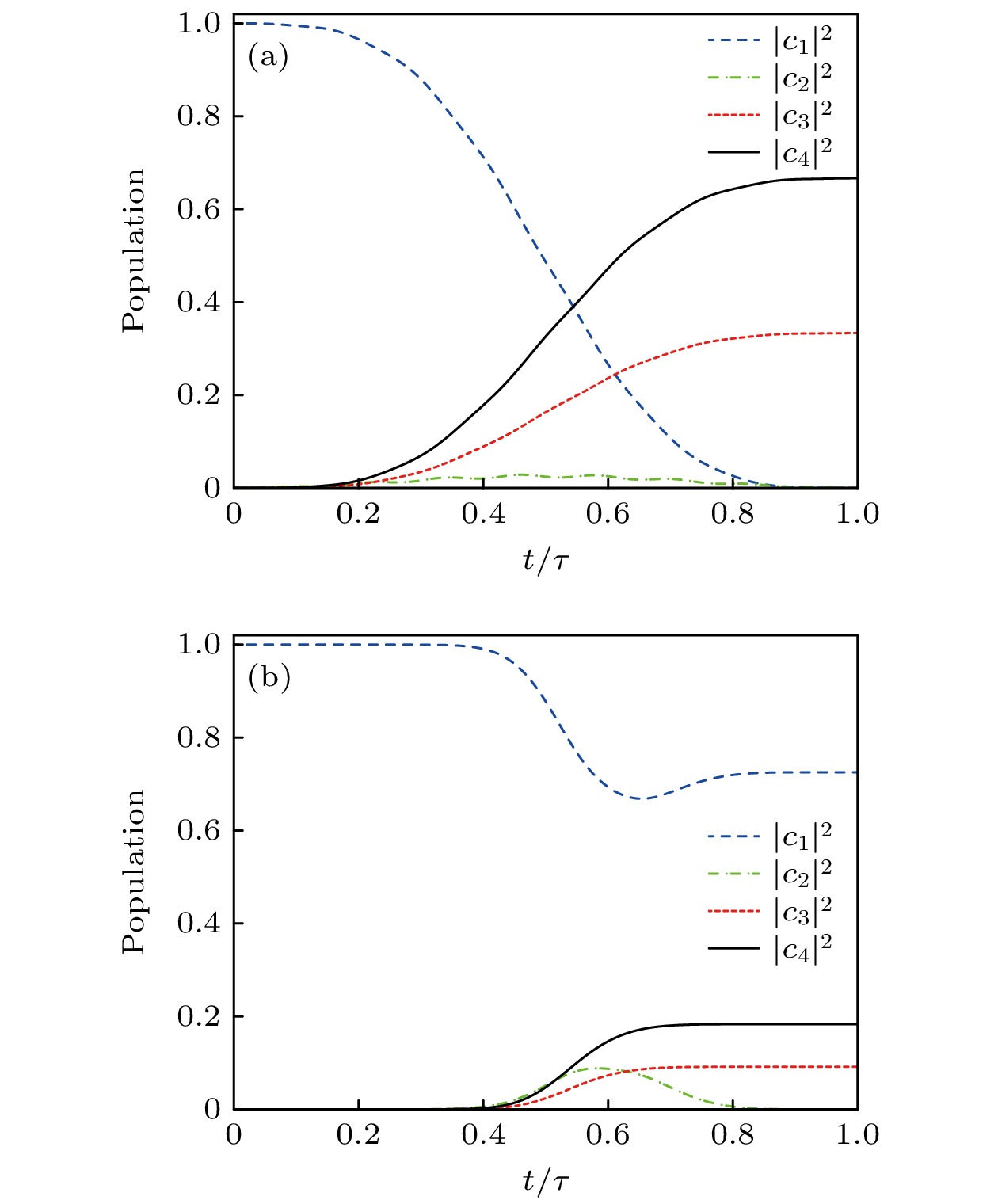
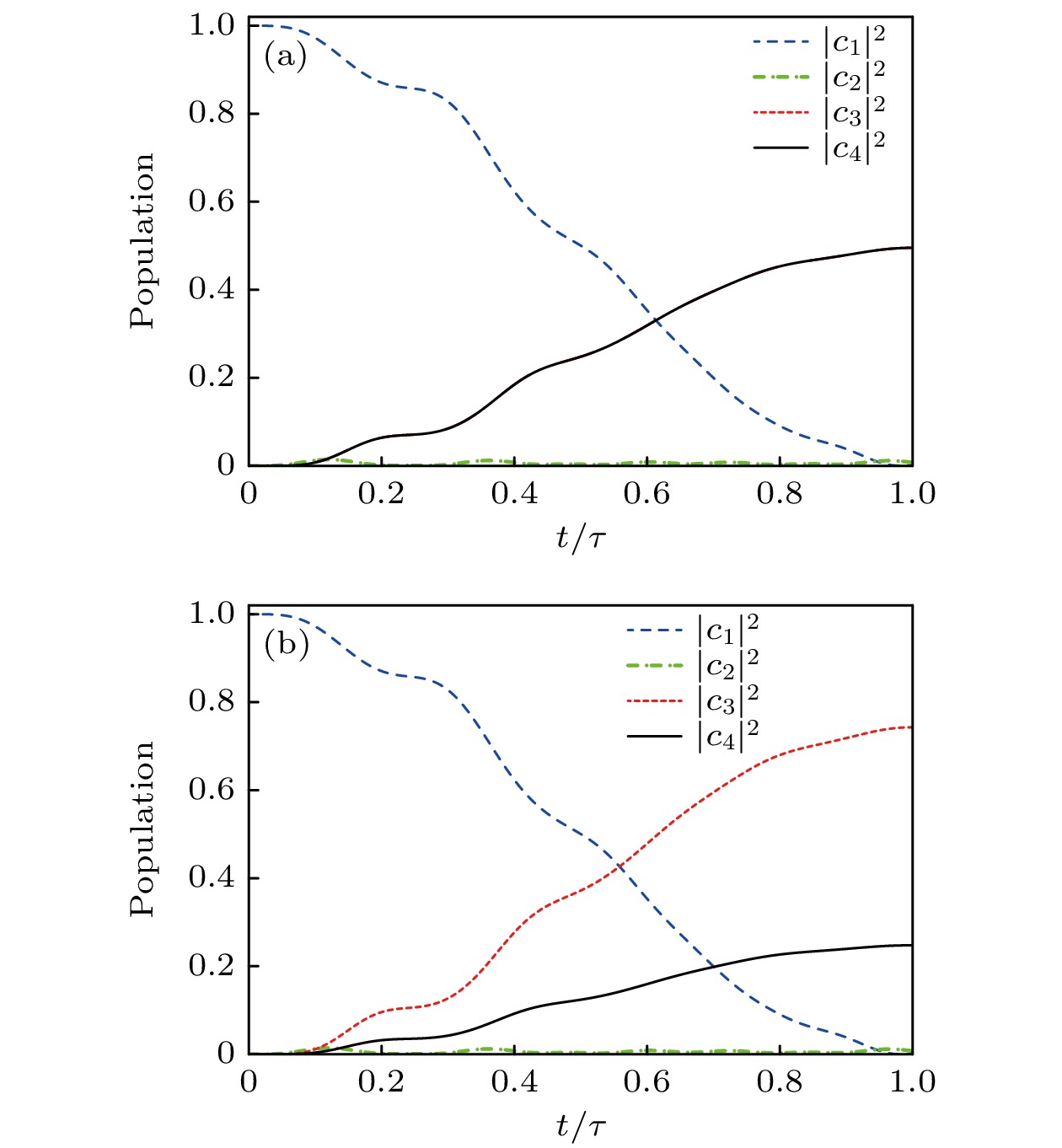
图 12 大失谐($ \varDelta > {\varOmega }_{0} $)情况下四能级系统布居数演化结果 (a)优化STIRAP演化结果; (b)传统STIRAP演化结果. 蓝色虚线为$ \left|1\right.\rangle $态量子数布居, 绿色点线为$ \left|2\right.\rangle $态量子数布居, 红色虚线为$ \left|3\right.\rangle $态量子数布居, 黑色实线为$ |4\rangle $态量子数布居; 工作时间τ = 7.4 μs, 脉冲峰值$ {\varOmega }_{0}= $$ 22.13\;{\mathrm{M}}{\mathrm{H}}{\mathrm{z}} $, 失谐量$ \varDelta =58.1\;{\mathrm{M}}{\mathrm{H}}{\mathrm{z}} $
Fig. 12. Population’s evolution of the four-level system for the large detuning ($ \varDelta > {\varOmega }_{0} $): (a) The result for the optimal STIRAP scheme; (b) the result for the standard STIRAP scheme. The blue dashed line, the green dotted line, the red dashed line and the black solid line correspond to the populations in the states $ \left|1\right.\rangle $, $ \left|2\right.\rangle $, $ \left|3\right.\rangle $ and $ \left|4\right.\rangle $, respectively. The pulse operating time $ \tau =7.4{\mathrm{ }}\;{\text{μ}}{\mathrm{s}} $, the pulse peak $ {\varOmega }_{0}=22.13\;{\mathrm{M}}{\mathrm{H}}{\mathrm{z}} $, and the detuning $ \varDelta = $$ 58.1\;{\mathrm{M}}{\mathrm{H}}{\mathrm{z}} $.
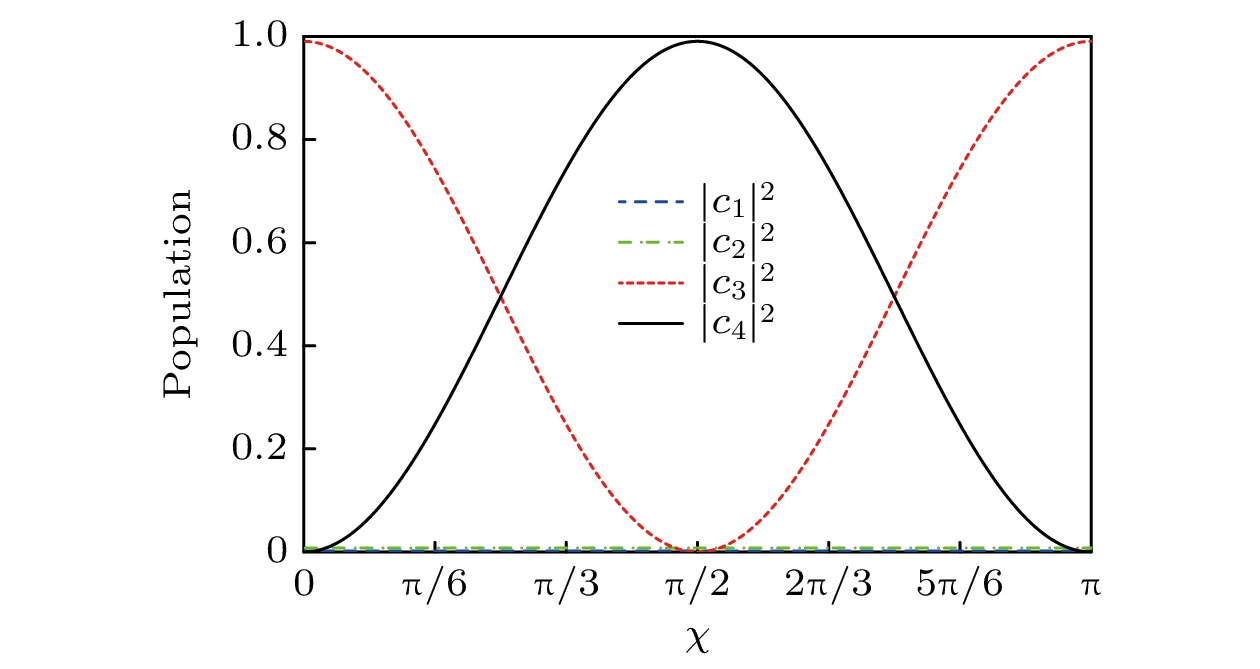
图 13 脉演化结果随脉冲参数$ \chi $变化情况. 蓝色虚线为$ \left|1\right.\rangle $态粒子数布居, 绿色点线为$ \left|2\right.\rangle $态粒子数布居, 红色虚线为$ \left|3\right.\rangle $态粒子数布居, 黑色实线为$ \left|4\right.\rangle $态粒子数布居; 工作时间τ = 7.4 μs, 脉冲峰值$ {\varOmega }_{0}=35\;{\mathrm{M}}{\mathrm{H}}{\mathrm{z}} $, 失谐量$ \varDelta =2 {\mathrm{\pi }}{\mathrm{ }}\;{\mathrm{M}}{\mathrm{H}}{\mathrm{z}} $
Fig. 13. Change of the populations for the four-level system with the parameter $ \chi $. The blue dashed line, the green dotted line, the red dashed line and the black solid line correspond to the populations in the states $ \left|1\right.\rangle $, $ \left|2\right.\rangle $, $ \left|3\right.\rangle $ and $ \left|4\right.\rangle $, respectively. The pulse operating time $ \tau =7.4\;{\mathrm{ }}{\text{μ}}{\mathrm{s}} $, the pulse peak $ {\varOmega }_{0}=35\;{\mathrm{M}}{\mathrm{H}}{\mathrm{z}} $, and the detuning $ \varDelta =2{\mathrm{\pi }}{\mathrm{ }}\;{\mathrm{M}}{\mathrm{H}}{\mathrm{z}} $.
图 14 布居数演化结果 (a)脉冲参数$ \eta $= $ {\mathrm{\pi }}/4 $; (b)脉冲参数$ \eta $= $ {\mathrm{\pi }}/6 $. 蓝色虚线为$ \left|1\right.\rangle $态粒子数布居, 绿色点线为$ \left|2\right.\rangle $态粒子数布居, 红色虚线为$ \left|3\right.\rangle $态粒子数布居, 黑色实线为$ \left|4\right.\rangle $态粒子数布居; 工作时间τ = 7.4 $ {\text{μ}}{\mathrm{s}} $, 脉冲峰值$ {\varOmega }_{0}= $$ 35{\mathrm{ }}\;{\mathrm{M}}{\mathrm{H}}{\mathrm{z}} $, 失谐量$ \varDelta =2{\mathrm{\pi }}\;{\mathrm{M}}{\mathrm{H}}{\mathrm{z}} $
Fig. 14. Evolution results of the populations: (a) Pulse parameter $ \eta $= $ {\mathrm{\pi }}/4 $; (b) pulse parameter $ \eta $= $ {\mathrm{\pi }}/6 $. The blue dashed line, the green dotted line, the red dashed line and the black solid line correspond to the populations in the states $ \left|1\right.\rangle $, $ \left|2\right.\rangle $, $ \left|3\right.\rangle $ and $ \left|4\right.\rangle $, respectively. The pulse operating time $ \tau =7.4\;{\mathrm{ }}{\text{μ}}{\mathrm{s}} $, the pulse peak $ {\varOmega }_{0}=35{\mathrm{ }}\;{\mathrm{M}}{\mathrm{H}}{\mathrm{z}} $, and the detuning $ \varDelta =2{\mathrm{\pi }}\;{\mathrm{M}}{\mathrm{H}}{\mathrm{z}} $.
-
[1] Born M, Fock V 1928 Zeitschrift für Physik 51 165
 Google Scholar
Google Scholar
[2] Wu Z, Yang H 2005 Phys. Rev. A 72 012114
 Google Scholar
Google Scholar
[3] Holonomy S B 1983 Phys. Rev. Lett. 51 2167
 Google Scholar
Google Scholar
[4] Xiao D, Chang M C, Niu Q 2010 Rev. Mod. Phys. 82 1959
 Google Scholar
Google Scholar
[5] Vitanov N V, Rangelov A A, Shore B W, Bergmann K 2017 Rev. Mod. Phys. 89 015006
 Google Scholar
Google Scholar
[6] Shore B W, Bergmann K, Oreg J, Rosenwaks S 1991 Phys. Rev. A 44 7442
 Google Scholar
Google Scholar
[7] Shore B W 2013 Acta Phys. Slovaca 63 361
 Google Scholar
Google Scholar
[8] 孙晓鹏, 冯志芳, 李卫东, 贾锁堂 2007 56 5727
 Google Scholar
Google Scholar
Sun X P, Feng Z F, Li W D, Jia S T 2007 Acta Phys. Sin. 56 5727
 Google Scholar
Google Scholar
[9] 孟少英, 吴炜, 刘彬 2009 58 6902
 Google Scholar
Google Scholar
Meng S Y, Wu W, Liu B 2009 Acta Phys. Sin. 58 6902
 Google Scholar
Google Scholar
[10] 李冠强, 彭娉 2011 60 110304
 Google Scholar
Google Scholar
Li G Q, Peng P 2011 Acta Phys. Sin. 60 110304
 Google Scholar
Google Scholar
[11] 李冠强, 彭娉, 曹振洲, 薛具奎 2012 61 090301
 Google Scholar
Google Scholar
Li G Q, Peng P, Cao Z Z, Xue J K 2012 Acta Phys. Sin. 61 090301
 Google Scholar
Google Scholar
[12] Bergmann K, Vitanov N V, Shore B W 2015 J. Chem. Phys. 142 170901
 Google Scholar
Google Scholar
[13] Fewell M P, Shore B W, Bergmann K 1997 Austra. J. Phys 50 281
 Google Scholar
Google Scholar
[14] Guéry-Odelin D, Ruschhaupt A, Kiely A, Torrontegui E, Martínez-Garaot S, Muga J G 2019 Rev. Mod. Phys. 91 045001
 Google Scholar
Google Scholar
[15] Hatomura T 2024 J. Phys. B: At. Mol. Opt. Phys. 57 102001
 Google Scholar
Google Scholar
[16] Chen X, Lizuain I, Ruschhaupt A, Guéry-Odelin D, Muga J G 2010 Phys. Rev. Letts. 105 123003
 Google Scholar
Google Scholar
[17] Minář Jí, Söyler Ş G, Rotondo P, Lesanovsky I 2017 New J. Phys. 19 063033
 Google Scholar
Google Scholar
[18] Ban Y, Chen X, Sherman E Y, Muga J G 2012 Phys. Rev. Letts. 109 206602
 Google Scholar
Google Scholar
[19] Opatrný T, Saberi H, Brion E, Mølmer K 2016 Phys. Rev. A 93 023815
 Google Scholar
Google Scholar
[20] Tian L 2012 Phys. Rev. Lett. 108 153604
 Google Scholar
Google Scholar
[21] Barrett S, Hammerer K, Harrison S, Northup T E, Osborne T J 2013 Phys. Rev. Lett. 110 090501
 Google Scholar
Google Scholar
[22] Yu X M, Zhou K, Zhang H Y, Li S X, Huang Z, Wen J, Zhang R, Yu Y 2025 Phys. Rev. A 111 012623
 Google Scholar
Google Scholar
[23] Masuda S 2012 Phys. Rev. A 86 063624
 Google Scholar
Google Scholar
[24] Masuda S, Güngördü U, Chen X, Ohmi T, Nakahara M 2016 Phys. Rev. A 93 013626
 Google Scholar
Google Scholar
[25] Demirplak M, Rice S A 2003 J. Phys. Chem. A 107 9937
 Google Scholar
Google Scholar
[26] Berry M V 2009 J. Phys. A: Math. Theor. 42 365303
 Google Scholar
Google Scholar
[27] Lewis H R, Riesenfeld W B 1969 J. Math. Phys. 10 1458
 Google Scholar
Google Scholar
[28] Chen X, Torrontegui E, Muga J G 2011 Phys. Rev. A 83 062116
 Google Scholar
Google Scholar
[29] Masuda S, Rice S A 2015 J. Phys. Chem. A 119 3479
 Google Scholar
Google Scholar
[30] Masuda S, Nakamura K 2008 Phys. Rev. A 78 062108
 Google Scholar
Google Scholar
[31] Torosov B T, Della Valle G, Longhi S 2014 Phys. Rev. A 89 063412
 Google Scholar
Google Scholar
[32] Torosov B T, Della Valle G, Longhi S 2013 Phys. Rev. A 87 052502
 Google Scholar
Google Scholar
[33] Li G Q, Chen G D, Peng P, Qi W 2017 Euro. Phys. J. D 71 14
 Google Scholar
Google Scholar
[34] Li K Z, Tian J Z, Xiao L T 2024 Phys. Rev. A 109 022443
 Google Scholar
Google Scholar
[35] Chen J F 2022 Phys. Rev. Res. 4 023252
 Google Scholar
Google Scholar
[36] Sun C P 1988 J. Phys. A: Math. Gen. 21 1595
 Google Scholar
Google Scholar
[37] Rigolin G, Ortiz G, Ponce V H 2008 Phys. Rev. A 78 052508
 Google Scholar
Google Scholar
[38] Chen J F, Sun C P, Dong H 2019 Phys. Rev. E 100 062140
 Google Scholar
Google Scholar
[39] Oh S, Shim Y P, Fei J, et al. 2013 Phys. Rev. A 87 022332
 Google Scholar
Google Scholar
[40] Gaubatz U, Rudecki P, Schiemann S, Bergmann K 1990 J. Chem. Phys. 92 5363
 Google Scholar
Google Scholar
[41] Unanyan R G, Shore B W, Bergmann K 2001 Phys. Rev. A 63 043401
 Google Scholar
Google Scholar
[42] Vitanov N V 1998 Phys. Rev. A 58 2295
 Google Scholar
Google Scholar
[43] Vitanov N V, Halfmann T, Shore B W, Bergmann K 2001 Ann. Rev. Phys. Chem. 52 763
 Google Scholar
Google Scholar
[44] Unanyan R, Fleischhauer M, Shore B W, Bergmann K 1998 Opt. Commun. 155 144
 Google Scholar
Google Scholar
[45] Madasu C S, Rathod K D, Kwong C C, Wilkowski D 2024 Phys. Rev. Appl. 21 L051001
 Google Scholar
Google Scholar
[46] Shi Z C, Wang J H, Zhang C, Song J, Xia Y 2024 Phys. Rev. A 109 022441
 Google Scholar
Google Scholar
[47] Jin Z Y, Jing J 2025 Phys. Rev. A 111 022628
 Google Scholar
Google Scholar
[48] Li G Q, Guo H, Zhang Y Q, Yang B, Peng P 2025 Commun. Theor. Phys. 77 015103
 Google Scholar
Google Scholar
计量
- 文章访问数: 1029
- PDF下载量: 37
- 被引次数: 0














 下载:
下载: