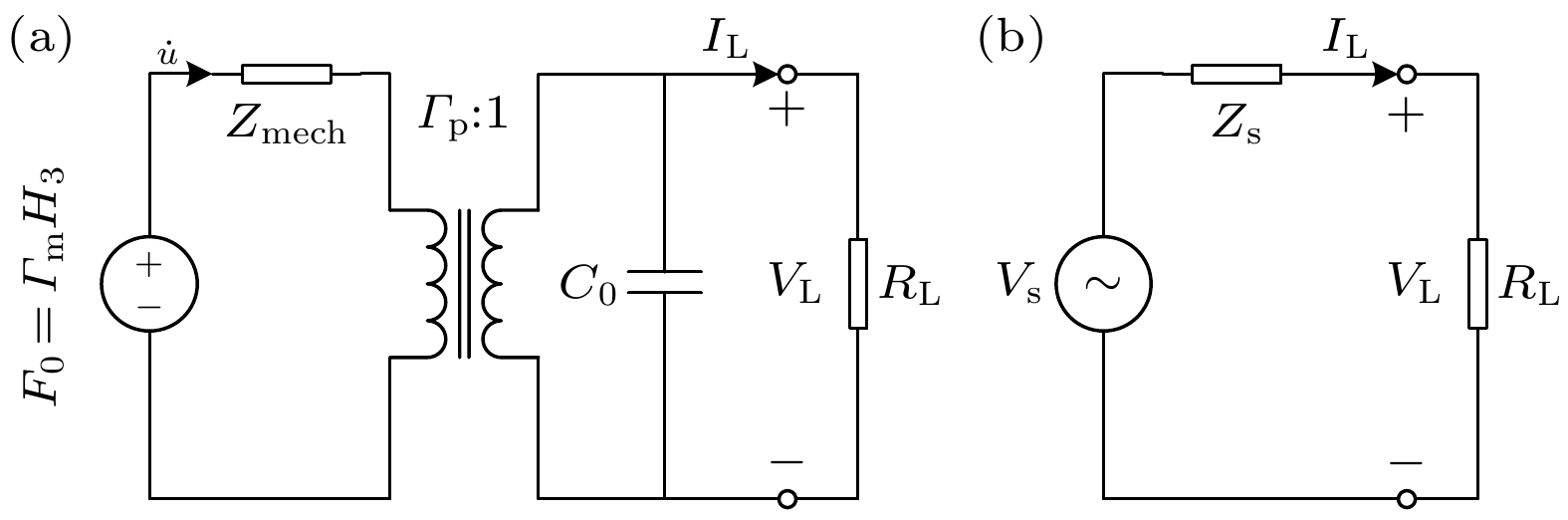
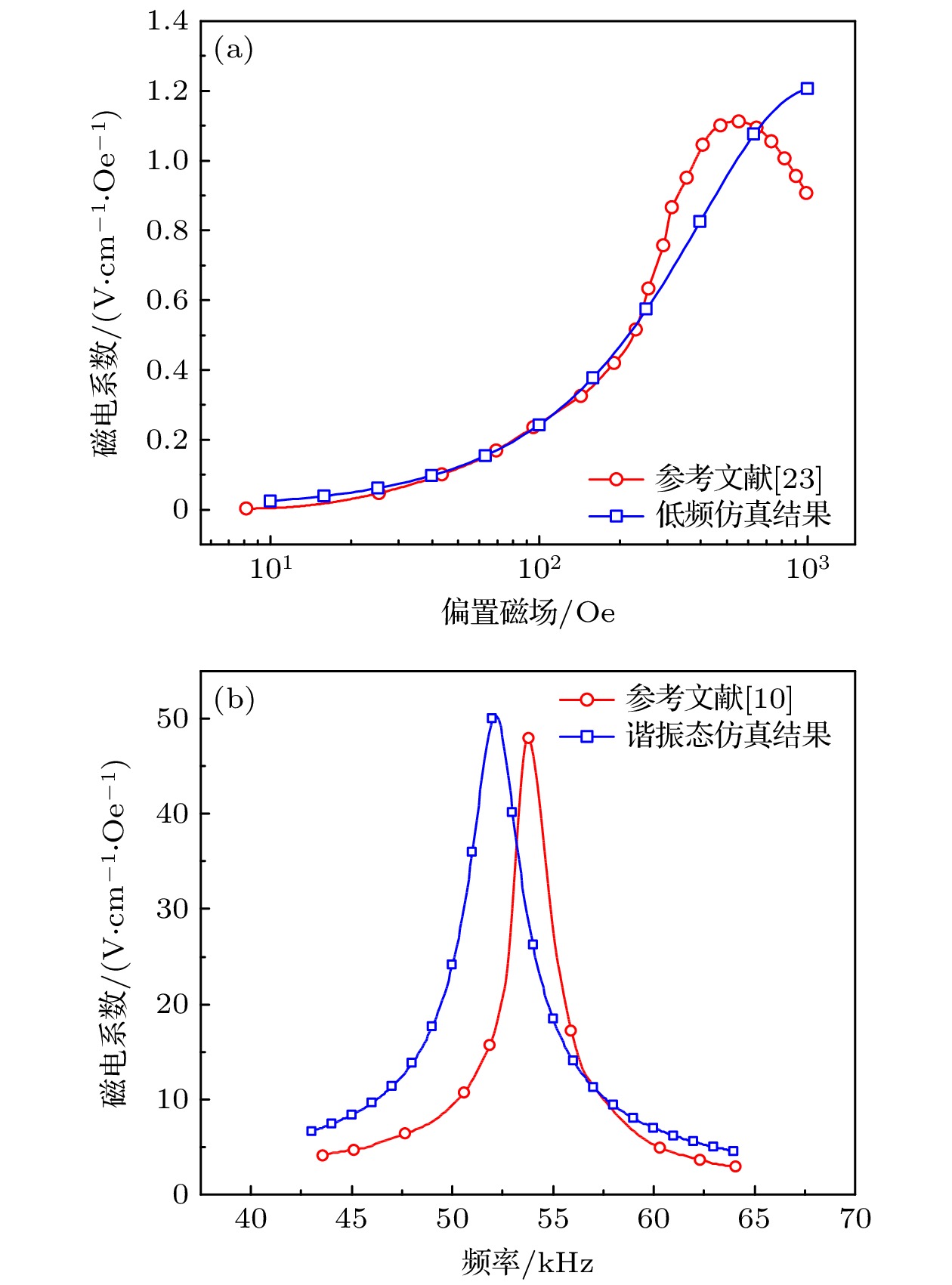
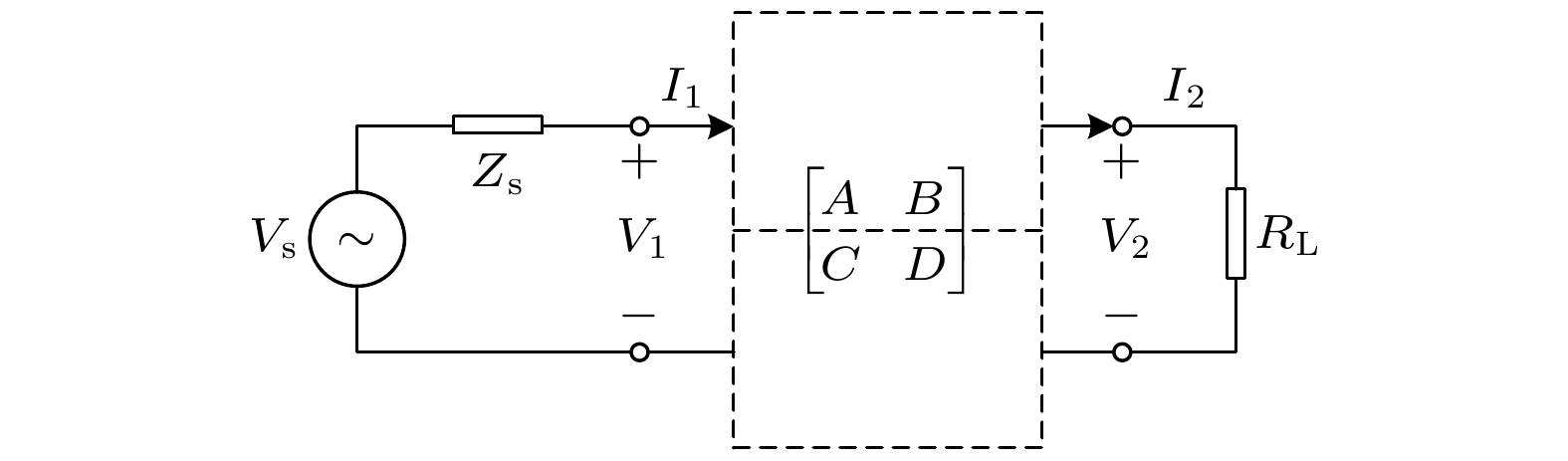
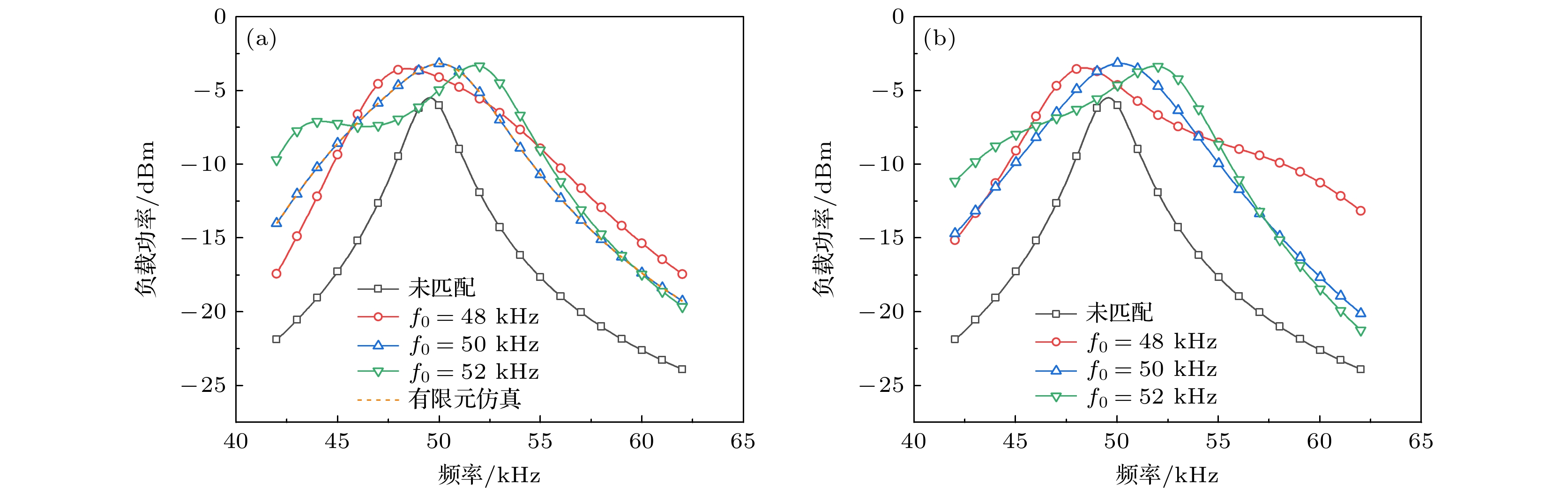
-
基于非线性磁致伸缩材料的本构方程, 建立了一种“磁-力-电”多场耦合的层合磁电换能器的有限元模型, 研究了不同偏置磁场下的谐振磁电效应. 基于等效电路模型和二端口网络理论, 实现了对谐振状态下磁电系数和等效源阻抗的完整求解. 在磁电换能器与负载电阻之间引入优化的L节匹配网络, 在提升负载功率的同时拓展了工作带宽. 仿真结果与相关文献数据吻合, 证实了该模型的准确性和有效性. 仿真结果表明, 所研究的层合磁电换能器, 其磁电系数在450 Oe的偏置磁场下达到51.79 V/(cm·Oe) @ 51.4 kHz, 在350 Oe的偏置磁场下达到极限输出功率–3.01 dBm@ 50.4 kHz. 以保证负载功率为前提, 通过优化匹配网络, 可实现2.30 dB的功率提升和2.27倍的带宽拓展. 本文所建立的非线性有限元模型充分考虑了偏置磁场对谐振磁电效应的影响, 该研究结果对小型化磁耦合无线功率传输系统的设计和性能提升具有重要的指导意义.Magnetoelectric composites comprised of piezoelectric and magnetostrictive materials, are widely used in magnetic field sensing, energy harvesting, and transducers. This work establishes a finite element model of a laminated magnetoelectric transducer coupled with magneto-elastic-electric fields based on the constitutive equation of the nonlinear magnetostrictive material. Then, the resonant magnetoelectric effect under different biased magnetic fields is studied. Based on the equivalent circuit model and the two-port network theory, the magnetoelectric coefficient and the equivalent source impedance under the resonant state are completely solved for the first time. Introducing optimized L-section matching networks between the magnetoelectric transducer and the load resistor can increase the load power and expand the operating bandwidth. The simulation results are consistent with the data in the literature, thus confirming the accuracy and effectiveness of the model. The simulation results demonstrate that the magnetoelectric coefficient reaches 51.79 V/(cm·Oe) at 51.4 kHz and 450 Oe bias magnetic field, and the ultimate output power of –3.01 dBm at 50.4 kHz and 350 Oe bias magnetic field. To ensure the load power, the power increase of 2.30 dB and the bandwidth expansion of 2.27 times are achieved by optimizing the matching network. The nonlinear finite element model in this work takes into account of the magnetoelectric effect under the acoustic resonance state and quantifies the ultimate output power. The magnetoelectric transducer model can obtain high magnetoelectric coefficient, load power, and power density in a small volume, providing a significant advantage in terms of equilibrium. The research results are of great importance in guiding the design and performance improvement of miniaturized magnetically coupled wireless power transfer systems.
-
Keywords:
- magnetoelectric effect /
- equivalent circuit /
- two-port network /
- impedance matching
[1] Wang Y, Gray D, Berry D, Gao J, Li M, Li J, Viehland D 2011 Adv. Mater. 23 4111
Google Scholar
[2] Nan C W, Bichurin M I, Dong S X, Viehland D, Srinivasan G 2008 J. Appl. Phys. 103 031101
Google Scholar
[3] Ju S, Chae S H, Choi Y, Lee S, Lee H W, Ji C H 2013 Smart Mater. Struct. 22 115037
Google Scholar
[4] Ryu J, Carazo A V, Uchino K, Kim H E 2001 Jpn. J. Appl. Phys. 40 4948
Google Scholar
[5] Dong S, Li J, Viehland D 2003 IEEE Trans. Ultrason. Ferroelectr. Freq. Control 50 1253
Google Scholar
[6] Dong S, Li J, Viehland D 2004 J. Appl. Phys. 95 2625
Google Scholar
[7] Zhang X, Zhou J, Yao X, Yang Z, Zhang G 2020 J. Magn. Magn. Mater. 501 166411
Google Scholar
[8] Nan T, Lin H, Gao Y, Matyushov A, Yu G, Chen H, Sun N, Wei S, Wang Z, Li M, Wang X, Belkessam A, Guo R, Chen B, Zhou J, Qian Z, Hui Y, Rinaldi M, McConney M E, Howe B M, Hu Z, Jones J G, Brown G J, Sun N X 2017 Nat. Commun. 8 296
Google Scholar
[9] Zaeimbashi M, Nasrollahpour M, Khalifa A, Romano A, Liang X, Chen H, Sun N, Matyushov A, Lin H, Dong C, Xu Z, Mittal A, Martos-Repath I, Jha G, Mirchandani N, Das D, Onabajo M, Shrivastava A, Cash S, Sun N X 2021 Nat. Commun. 12 3141
Google Scholar
[10] Truong B D 2020 IEEE Sens. J. 20 5322
Google Scholar
[11] Truong B D, Roundy S 2020 Smart Mater. Struct. 29 085053
Google Scholar
[12] Truong B D, Andersen E, Casados C, Roundy S 2020 Sens. Actuator A-Phys. 316 112269
Google Scholar
[13] Hosur S, Sriramdas R, Karan S K, Liu N, Priya S, Kiani M 2021 IEEE Trans. Biomed. Circuits Syst. 15 1079
Google Scholar
[14] Singer A, Robinson J T 2021 Adv. Healthc. Mater. 10 2100664
Google Scholar
[15] Saha O, Truong B D, Roundy S 2022 Smart Mater. Struct. 31 113001
Google Scholar
[16] 施展, 南策文 2004 53 2766
Google Scholar
Shi Z, Nan C W 2004 Acta Phys. Sin. 53 2766
Google Scholar
[17] 代显智 2014 63 207501
Google Scholar
Dai X Z 2014 Acta Phys. Sin. 63 207501
Google Scholar
[18] Li J, Wen Y, Li P, Yang J 2017 IEEE Trans. Magn. 53 2500406
Google Scholar
[19] Zhou J, Zhang Y, Zhang G, Liu P 2013 J. Appl. Phys. 113 043907
Google Scholar
[20] Zhou J, Ma Y, Zhang G, Chen X 2014 Appl. Phys. Lett. 104 202904
Google Scholar
[21] Zhang X, Yao X, Zhou J, Yang Z 2018 J. Mater. Sci. Mater. Electron. 29 17706
Google Scholar
[22] 周勇, 李纯健, 潘昱融 2018 67 077702
Google Scholar
Zhou Y, Li C J, Pan Y R 2018 Acta Phys. Sin. 67 077702
Google Scholar
[23] Han J, Zhang J, Gao Y 2018 J. Magn. Magn. Mater. 466 200
Google Scholar
[24] Stampfli R, Youssef G 2020 Int. J. Mech. Sci. 177 105573
Google Scholar
[25] Liu X E, Zheng X J 2005 Acta Mech. Sin. 21 278
Google Scholar
[26] Zhou H M, Cui X L 2014 Smart Mater. Struct. 23 105014
Google Scholar
[27] Yang F, Wen Y M, Li P, Zheng M, Bian L X 2008 Sens. Actuator A-Phys. 141 129
Google Scholar
[28] Zhou J, Yang Y, Zhang G, Peng J, Liu P 2016 Compos. Struct. 155 107
Google Scholar
[29] 波扎尔D M著 (张肇仪, 周乐柱, 吴德明译) 2015 微波工程(北京: 电子工业出版社)第190—192页
Pozar D M(translated by Zhang Z Y, Zhou L Z, Wu D M)2015 Microwave Engineering (Beijing: Publishing House of Electronics Industry) pp190–192 (in Chinese)
[30] Saha O, Andersen E, Roundy S 2021 IEEE 20th International Conference on Micro and Nanotechnology for Power Generation and Energy Conversion Applications (PowerMEMS) Exeter, United Kingdom, December 6–8, 2021 pp36–39
[31] Mukherjee D, Mallick D 2023 Appl. Phys. Lett. 122 014102
Google Scholar
-
图 1 (a) 层合磁电换能器结构示意图; (b) 压电层的局部坐标系, 与空间全局坐标系一致; (c) 磁致伸缩层的局部坐标系
Fig. 1. (a) Schematic a laminated magnetoelectric transducer; (b) local coordinate of the piezoelectric layer is consistent with the global coordinate; (c) local coordinate of the magnetostrictive layer.
图 2 等效电路模型 (a) 磁-力-电耦合电路; (b) 戴维南等效电路
Fig. 2. Equivalent circuit model: (a) Magneto-elastic-electric coupling; (b) Thevenin equivalent circuit.
图 3 有限元模型中各模块之间的耦合及仿真计算方案
Fig. 3. The coupling between each module in the finite element model and the simulation calculation scheme.
图 4 切向压磁应变常数和切向磁导率的随偏置磁场的变化
Fig. 4. Variation of tangent piezomagnetic coupling coefficient and tangent magnetic susceptibility with bias magnetic fields.
图 5 仿真结果与参考文献数据对比 (a) 低频磁电系数; (b) 谐振磁电系数
Fig. 5. Comparison of simulation results with reference data: (a) Low-frequency magnetoelectric coefficient; (b) resonance magnetoelectric coefficient.
图 6 磁电换能器在不同偏置磁场下的频域响应 (a) 等效源电阻; (b) 等效源电抗; (c) 磁电系数; (d) 负载极限功率
Fig. 6. Frequency domain response of magnetoelectric transducer under different biased magnetic fields: (a) Equivalent source resistance; (b) equivalent source reactance; (c) magnetoelectric coefficient; (d) ultimate load power.
图 7 引入匹配网络后的等效电路
Fig. 7. The equivalent circuit after the matching network is introduced.
图 8 (a) L节匹配网络,
${\text{j}}B$ 和${\text{j}}X$ 分别表示并联电纳和串联电抗; (b) 匹配网络Ⅰ; (c) 匹配网络ⅡFig. 8. (a) L-section matching network,
${\text{j}}B$ and${\text{j}}X$ represent parallel susceptance and series reactance, respectively; (b) matching network Ⅰ; (c) matching network Ⅱ.
图 9 引入匹配网络后的效果 (a) 匹配网络Ⅰ; (b) 匹配网络Ⅱ
Fig. 9. The effect after the introduction of the matching network: (a) Matching network Ⅰ; (b) matching network Ⅱ.
表 1 引入匹配网络前后的性能对比
Table 1. Performance comparison before and after the matching network is introduced.
匹配网络 匹配元件 匹配性能 ${L_{\text{m}}}$/mH ${C_{\text{m}}}$/nF $\max {P_L}$/dBm 带宽/kHz 未匹配 无 –5.49 2.58 ${f_0} = 48$kHz Ⅰ 0.38 20.60 –3.51 6.98 Ⅱ 0.53 8.36 –3.46 5.66 ${f_0} = 50$kHz Ⅰ 0.40 24.96 –3.19 5.86 Ⅱ 0.41 18.38 –3.15 5.70 ${f_0} = 52$kHz Ⅰ 0.63 13.84 –3.30 5.04 Ⅱ 0.69 11.05 –3.35 6.14 
下载: 导出CSV
表 2 不同磁电换能器的性能指标对比
Table 2. Performance comparison of different magnetoelectric transducers.
参考文献 材料类型 体积/mm3 磁电系数
/(${\text{V} } \cdot {\text{c} }{ {\text{m} }^{ { { - 1} } } } \cdot {\text{O} }{ {\text{e} }^{ {{ - 1} } } }$)负载功率*/dBm 功率密度
/(${\text{mW} } \cdot {\text{c} }{ {\text{m} }^{ {{ - 3} } } } \cdot {\text{O} }{ {\text{e} }^{ {{ - 2} } } }$)压电 磁致伸缩 [11,12] PZT-5A Galfenol 352 41.17 –0.16 2.74 [13] PZT Galfenol 152.61 N/A –2.11 4.03 [30] PZT-5A Ni和Metglas 50.73 52.00 –7.85 3.23 [31] PVDF Metglas 1.75 0.133 –18.54 8.00 本文 PZT-5H Terfenol-D 100 51.79 –3.15 4.84 注: * 为1 Oe交流磁场激励下的负载功率.
下载: 导出CSV
-
[1] Wang Y, Gray D, Berry D, Gao J, Li M, Li J, Viehland D 2011 Adv. Mater. 23 4111
Google Scholar
[2] Nan C W, Bichurin M I, Dong S X, Viehland D, Srinivasan G 2008 J. Appl. Phys. 103 031101
Google Scholar
[3] Ju S, Chae S H, Choi Y, Lee S, Lee H W, Ji C H 2013 Smart Mater. Struct. 22 115037
Google Scholar
[4] Ryu J, Carazo A V, Uchino K, Kim H E 2001 Jpn. J. Appl. Phys. 40 4948
Google Scholar
[5] Dong S, Li J, Viehland D 2003 IEEE Trans. Ultrason. Ferroelectr. Freq. Control 50 1253
Google Scholar
[6] Dong S, Li J, Viehland D 2004 J. Appl. Phys. 95 2625
Google Scholar
[7] Zhang X, Zhou J, Yao X, Yang Z, Zhang G 2020 J. Magn. Magn. Mater. 501 166411
Google Scholar
[8] Nan T, Lin H, Gao Y, Matyushov A, Yu G, Chen H, Sun N, Wei S, Wang Z, Li M, Wang X, Belkessam A, Guo R, Chen B, Zhou J, Qian Z, Hui Y, Rinaldi M, McConney M E, Howe B M, Hu Z, Jones J G, Brown G J, Sun N X 2017 Nat. Commun. 8 296
Google Scholar
[9] Zaeimbashi M, Nasrollahpour M, Khalifa A, Romano A, Liang X, Chen H, Sun N, Matyushov A, Lin H, Dong C, Xu Z, Mittal A, Martos-Repath I, Jha G, Mirchandani N, Das D, Onabajo M, Shrivastava A, Cash S, Sun N X 2021 Nat. Commun. 12 3141
Google Scholar
[10] Truong B D 2020 IEEE Sens. J. 20 5322
Google Scholar
[11] Truong B D, Roundy S 2020 Smart Mater. Struct. 29 085053
Google Scholar
[12] Truong B D, Andersen E, Casados C, Roundy S 2020 Sens. Actuator A-Phys. 316 112269
Google Scholar
[13] Hosur S, Sriramdas R, Karan S K, Liu N, Priya S, Kiani M 2021 IEEE Trans. Biomed. Circuits Syst. 15 1079
Google Scholar
[14] Singer A, Robinson J T 2021 Adv. Healthc. Mater. 10 2100664
Google Scholar
[15] Saha O, Truong B D, Roundy S 2022 Smart Mater. Struct. 31 113001
Google Scholar
[16] 施展, 南策文 2004 53 2766
Google Scholar
Shi Z, Nan C W 2004 Acta Phys. Sin. 53 2766
Google Scholar
[17] 代显智 2014 63 207501
Google Scholar
Dai X Z 2014 Acta Phys. Sin. 63 207501
Google Scholar
[18] Li J, Wen Y, Li P, Yang J 2017 IEEE Trans. Magn. 53 2500406
Google Scholar
[19] Zhou J, Zhang Y, Zhang G, Liu P 2013 J. Appl. Phys. 113 043907
Google Scholar
[20] Zhou J, Ma Y, Zhang G, Chen X 2014 Appl. Phys. Lett. 104 202904
Google Scholar
[21] Zhang X, Yao X, Zhou J, Yang Z 2018 J. Mater. Sci. Mater. Electron. 29 17706
Google Scholar
[22] 周勇, 李纯健, 潘昱融 2018 67 077702
Google Scholar
Zhou Y, Li C J, Pan Y R 2018 Acta Phys. Sin. 67 077702
Google Scholar
[23] Han J, Zhang J, Gao Y 2018 J. Magn. Magn. Mater. 466 200
Google Scholar
[24] Stampfli R, Youssef G 2020 Int. J. Mech. Sci. 177 105573
Google Scholar
[25] Liu X E, Zheng X J 2005 Acta Mech. Sin. 21 278
Google Scholar
[26] Zhou H M, Cui X L 2014 Smart Mater. Struct. 23 105014
Google Scholar
[27] Yang F, Wen Y M, Li P, Zheng M, Bian L X 2008 Sens. Actuator A-Phys. 141 129
Google Scholar
[28] Zhou J, Yang Y, Zhang G, Peng J, Liu P 2016 Compos. Struct. 155 107
Google Scholar
[29] 波扎尔D M著 (张肇仪, 周乐柱, 吴德明译) 2015 微波工程(北京: 电子工业出版社)第190—192页
Pozar D M(translated by Zhang Z Y, Zhou L Z, Wu D M)2015 Microwave Engineering (Beijing: Publishing House of Electronics Industry) pp190–192 (in Chinese)
[30] Saha O, Andersen E, Roundy S 2021 IEEE 20th International Conference on Micro and Nanotechnology for Power Generation and Energy Conversion Applications (PowerMEMS) Exeter, United Kingdom, December 6–8, 2021 pp36–39
[31] Mukherjee D, Mallick D 2023 Appl. Phys. Lett. 122 014102
Google Scholar
下载:
计量
- 文章访问数: 4275
- PDF下载量: 147
- 被引次数: 0
