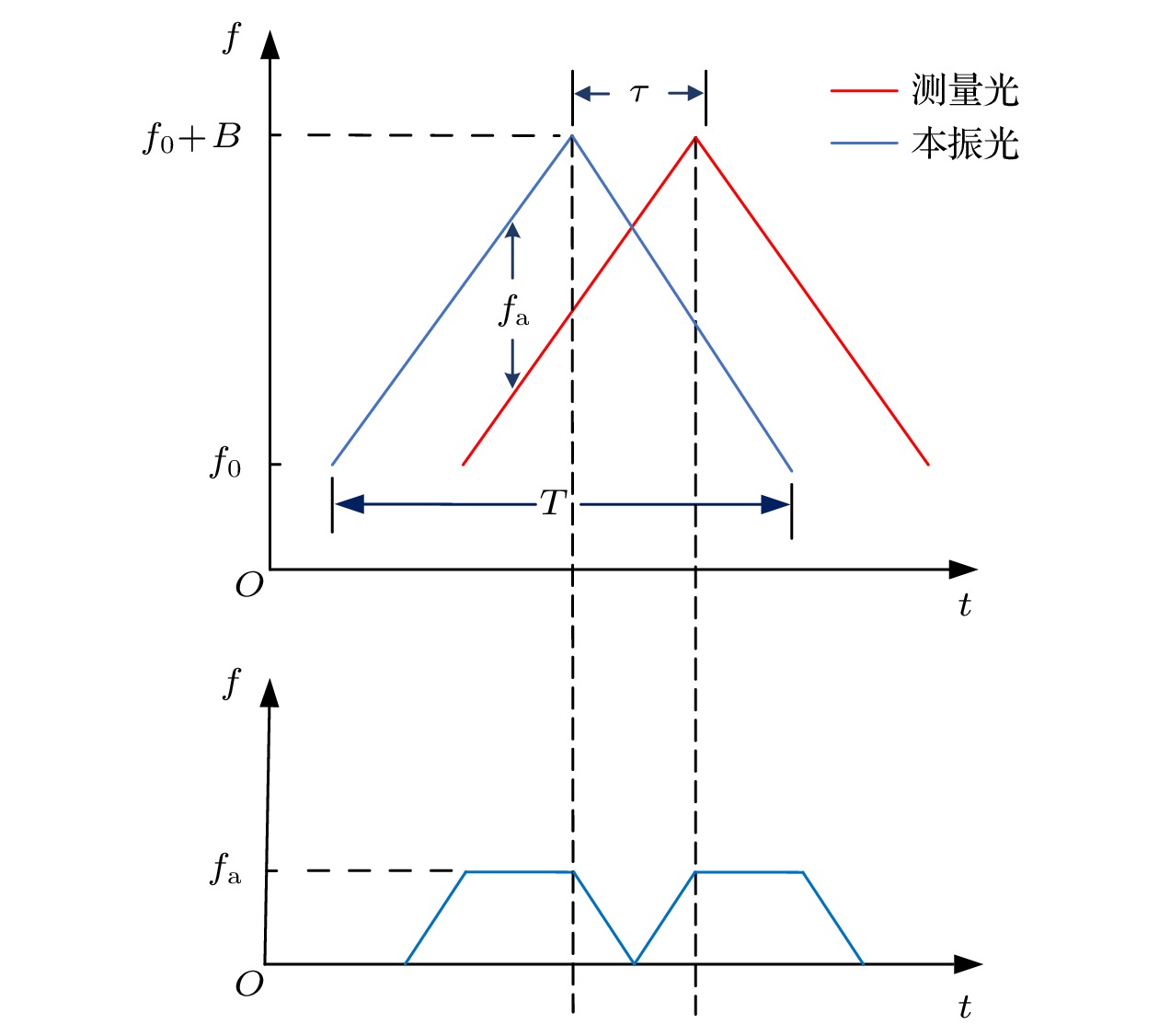
-
激光扫频干涉测量技术因其精度高、抗干扰能力强等优势成为研究热点. 而激光器调频的非线性问题一直是影响测量精度的关键因素, 非线性带来的直观结果就是拍信号的频谱严重展宽, 造成测距精度下降. 为解决该问题, 本文提出了一种基于Lomb-Scargle算法的非线性校正方法, 搭建了具有辅助干涉仪的激光扫频干涉测量系统, 通过对辅助路拍信号进行希尔伯特变换提取相位, 再基于提取到的相位信息生成一个新的时间序列, 结合Lomb-Scargle算法, 将非线性校正与拍信号频率计算同时进行. 作为验证, 对于0.5—1.3 m范围内的目标进行了测量, 最大误差为14 μm. 区别于传统频率采样法校正原理, 本文提出的校正方法并不是以辅助路的拍信号对测量路进行重采样, 所以无需满足辅助干涉仪光程差大于测量路光程差两倍的条件, 因而可为增大测距量程提供一种思路.
-
关键词:
- 激光扫频干涉 /
- 非线性校正 /
- Lomb-Scargle算法
Laser frequency scanning interference technology has become a research hotspot due to its high precision and strong anti-interference capability and other advantages. The nonlinear problem of laser frequency modulation has always been a key factor affecting the accuracy of the measurement system. The most direct result of the nonlinearity of frequency modulation is that the spectrum of the beat signal is severely broadened, resulting in a decrease in the ranging accuracy. In order to solve this problem, this paper proposes a nonlinear correction method based on the Lomb-Scargle algorithm, and builds a laser frequency sweep interferometry system with an auxiliary interferometer. The phase is extracted by performing Hilbert transform on the auxiliary path beat signal, thereby generating a new time series based on the extracted phase information. The generated time series carries the phase change information of the auxiliary path beat signal, and it is combined with the Lomb-Scargle algorithm to perform the nonlinear correction of the measurement system and the frequency calculation of the beat signal simultaneously. As a verification, the targets in the range of 0.5–1.3 m are measured with a maximum error of 14 μm. The traditional frequency sampling method is limited by the Nyquist sampling theorem, and the laser emission and reception need to travel a round-trip distance, which means that the frequency sampling method must meet the requirement that the distance of the measured target cannot exceed a quarter of the optical path difference of the auxiliary interferometer. Therefore, the range of distance measurement is limited when the optical path difference of the auxiliary interferometer is constant. Different from the correction principle of the traditional frequency sampling method, the correction method proposed in this paper does not use the beat signal of the auxiliary path to resample the measurement path, so there is no need to satisfy the condition that the optical path difference of the auxiliary interferometer is greater than four times the measuring distance. Therefore, in the case of a certain optical path difference of the auxiliary interferometer, it can provide a way to increase the ranging range of the system.[1] Okano M, Chong C 2020 Opt. Express 28 23898
Google Scholar
[2] Zhang X B Q, Kong M, Guo T T, Zhao J, Wang D D, Liu L, Liu W, Xu X K 2021 Appl. Opt. 60 3446
Google Scholar
[3] Pan H, Zhang F, Shi C, Qu X 2017 Appl. Opt. 56 6956
Google Scholar
[4] You C W, Chen S T, Wang T Y, Liu J S, Wang K J, Yang Z G, 2021 Opt. Express 29 34510
Google Scholar
[5] Tseng C H, Hung Y H, Hwang S K 2019 Opt. Lett. 44 3334
Google Scholar
[6] 许新科, 刘国栋, 刘炳国, 陈凤东, 庄志涛, 甘雨 2015 64 219501
Google Scholar
Xu X K, Liu G D, Liu B G, Chen F D, Zhuang Z T, Gan Y 2015 Acta Phys. Sin. 64 219501
Google Scholar
[7] Iiyama K, Wang L T, Hayashi K L 1996 J. Lightwave Technol. 14 173
Google Scholar
[8] Roos P A, Reibel R R, Berg T, Kaylor B, Barber Z W, Babbitt W R 2009 Opt. Lett. 34 3692
Google Scholar
[9] Behroozpour B, Sandborn P A, Quack N, Seok T J, Matsui Y, Wu M C, Boser B E 2017 IEEE J. Solid-State Circuits. 52 161
Google Scholar
[10] Cao X, Wu K, Li C, Zhang G, Chen J 2021 J. Opt. Soc. Am. B. 38 D8
Google Scholar
[11] Zhang X, Pouls J, Wu M C 2019 Opt. Express 27 9965
Google Scholar
[12] 徐靖翔, 孔明, 许新科 2021 70 034205
Google Scholar
Xu J X, Kong M, Xu X K 2021 Acta Phys. Sin. 70 034205
Google Scholar
[13] 孟祥松, 张福民, 曲兴华 2015 64 230601
Google Scholar
Meng X S, Zhang F M, Qu X H 2015 Acta Phys. Sin. 64 230601
Google Scholar
[14] 时光, 张福民, 曲兴华, 孟祥松 2014 63 184209
Google Scholar
Shi G, Zhang F M, Qu X H, Meng X S 2014 Acta Phys. Sin. 63 184209
Google Scholar
[15] Glombitza U, Brinkmeyer E 1993 J. Lightwave Technol. 11 1377
Google Scholar
[16] Ahn T J, Lee J Y, Kim D Y 2005 Appl. Opt. 44 7630
Google Scholar
[17] Shi G, Wang W, Zhang F 2018 Opt. Commun. 411 152
Google Scholar
[18] 李超林, 刘俊辰, 张福民, 曲兴华 2022 光电工程 49 210438
Google Scholar
Li C L, Liu J C, Zhang F M, Qu X H 2022 Opto-Electron Eng. 49 210438
Google Scholar
[19] 包为政, 张福民, 曲兴华 2020 激光技术 44 1
Google Scholar
Bao W Z, Zhang F M, Qu X H 2020 Laser Technol. 44 1
Google Scholar
[20] Jiang S, Liu B, Wang H 2021 Appl. Opt. 60 918
Google Scholar
[21] Zhao Q W 2014 M. S. Thesis (Xiangtan: Xiangtan University) (in Chinese) [赵秋雯 2014 硕士学位论文 (湘潭: 湘潭大学)]
[22] Horne J H, Baliunas S L 1986 ApJ. 302 757
Google Scholar
[23] Press W H, Rybicki G B 1989 ApJ. 338 277
Google Scholar
[24] Sandborn P A M 2017 Ph. D. Dissertation (Berkeley: University of California)
-
图 1 激光扫频干涉测距系统示意图
Fig. 1. Schematic diagram of the laser scanning interference ranging system.
图 2 理想情况下拍信号形成示意图
Fig. 2. Schematic diagram of beat signal formation under ideal conditions.
图 4 激光调频非线性对拍信号的影响 (a) FFT变化得到的频谱图; (b)相位偏差; (c)时频曲线局部图
Fig. 4. Effect of laser scanning nonlinearity on the beat signal: (a) Spectrogram obtained by FFT variation; (b) phase deviation; (c) partial diagram of time-frequency curve.
图 5 希尔伯特变换提取相位 (a)局部折叠相位; (b)解包裹后相位
Fig. 5. Hilbert transform to extract phase: (a) Locally folded phase; (b) phase after unwrapping.
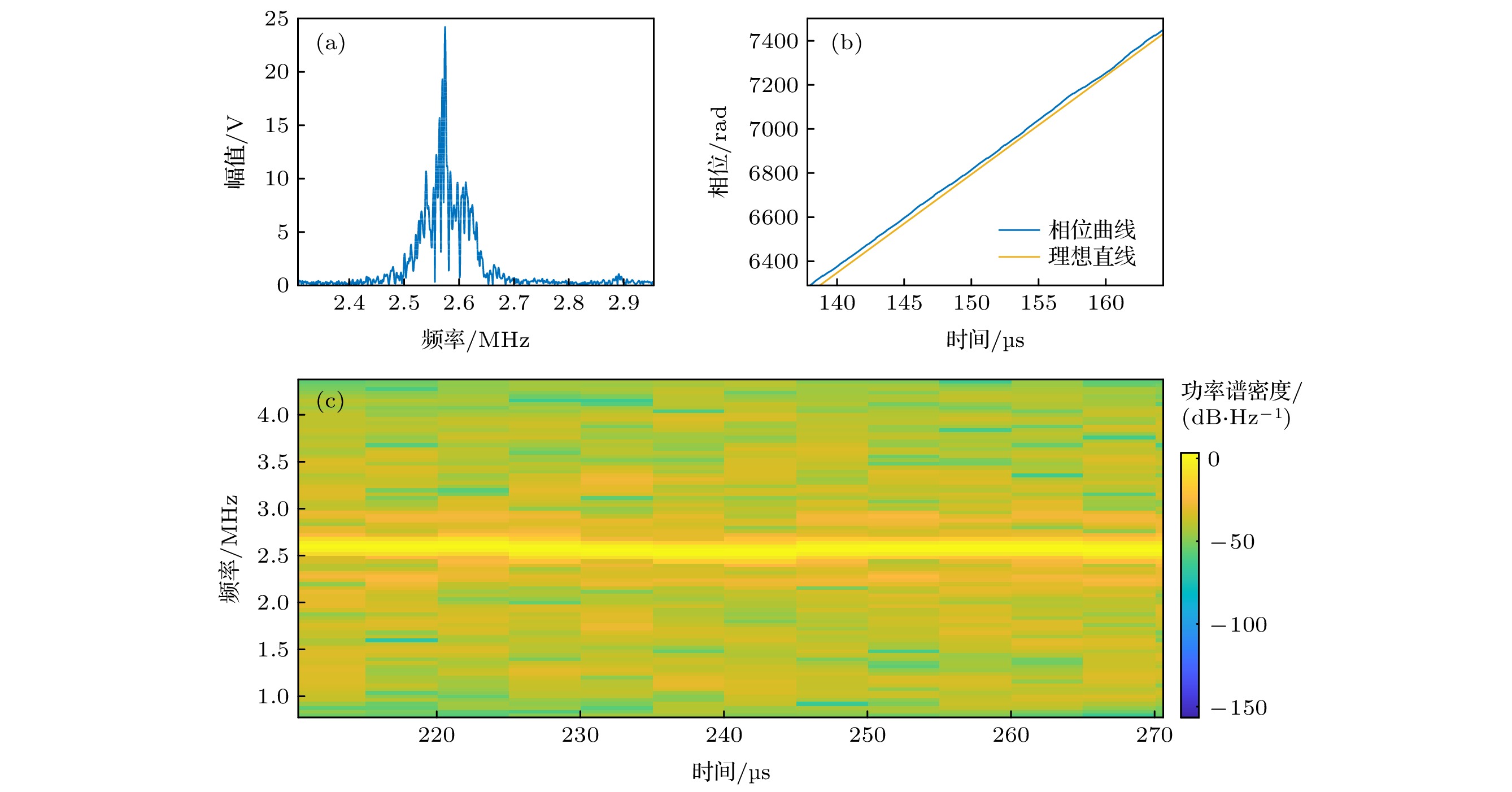
图 6 引入新的时间序列前后LS谱对比图 (a)辅助路引入时间序列前; (b)辅助路引入时间序列后; (c)测量路引入时间序列前; (d)测量路引入时间序列后
Fig. 6. Comparison of LS spectra before and after the introduction of the new time series: (a) Auxiliary path before introduction of time series; (b) auxiliary path after introduction of time series; (c) measurement path before introduction of time series; (d) measurement path after introduction of time series.
图 7 测距误差 (a)频率采样法; (b) LS法
Fig. 7. Distance measurement error: (a) Frequency sampling method; (b) LS method.
表 1 频率采样法不适用情况下LS法测距的绝对误差
Table 1. Ranging error of Lomb algorithm when frequency sampling method is not applicable.
距初始位置的位移量ΔR/mm LS法误差/mm 100.1480 0.012 350.1931 0.014 550.1871 0.007 699.7001 0.008 850.2181 –0.010 
下载: 导出CSV
-
[1] Okano M, Chong C 2020 Opt. Express 28 23898
Google Scholar
[2] Zhang X B Q, Kong M, Guo T T, Zhao J, Wang D D, Liu L, Liu W, Xu X K 2021 Appl. Opt. 60 3446
Google Scholar
[3] Pan H, Zhang F, Shi C, Qu X 2017 Appl. Opt. 56 6956
Google Scholar
[4] You C W, Chen S T, Wang T Y, Liu J S, Wang K J, Yang Z G, 2021 Opt. Express 29 34510
Google Scholar
[5] Tseng C H, Hung Y H, Hwang S K 2019 Opt. Lett. 44 3334
Google Scholar
[6] 许新科, 刘国栋, 刘炳国, 陈凤东, 庄志涛, 甘雨 2015 64 219501
Google Scholar
Xu X K, Liu G D, Liu B G, Chen F D, Zhuang Z T, Gan Y 2015 Acta Phys. Sin. 64 219501
Google Scholar
[7] Iiyama K, Wang L T, Hayashi K L 1996 J. Lightwave Technol. 14 173
Google Scholar
[8] Roos P A, Reibel R R, Berg T, Kaylor B, Barber Z W, Babbitt W R 2009 Opt. Lett. 34 3692
Google Scholar
[9] Behroozpour B, Sandborn P A, Quack N, Seok T J, Matsui Y, Wu M C, Boser B E 2017 IEEE J. Solid-State Circuits. 52 161
Google Scholar
[10] Cao X, Wu K, Li C, Zhang G, Chen J 2021 J. Opt. Soc. Am. B. 38 D8
Google Scholar
[11] Zhang X, Pouls J, Wu M C 2019 Opt. Express 27 9965
Google Scholar
[12] 徐靖翔, 孔明, 许新科 2021 70 034205
Google Scholar
Xu J X, Kong M, Xu X K 2021 Acta Phys. Sin. 70 034205
Google Scholar
[13] 孟祥松, 张福民, 曲兴华 2015 64 230601
Google Scholar
Meng X S, Zhang F M, Qu X H 2015 Acta Phys. Sin. 64 230601
Google Scholar
[14] 时光, 张福民, 曲兴华, 孟祥松 2014 63 184209
Google Scholar
Shi G, Zhang F M, Qu X H, Meng X S 2014 Acta Phys. Sin. 63 184209
Google Scholar
[15] Glombitza U, Brinkmeyer E 1993 J. Lightwave Technol. 11 1377
Google Scholar
[16] Ahn T J, Lee J Y, Kim D Y 2005 Appl. Opt. 44 7630
Google Scholar
[17] Shi G, Wang W, Zhang F 2018 Opt. Commun. 411 152
Google Scholar
[18] 李超林, 刘俊辰, 张福民, 曲兴华 2022 光电工程 49 210438
Google Scholar
Li C L, Liu J C, Zhang F M, Qu X H 2022 Opto-Electron Eng. 49 210438
Google Scholar
[19] 包为政, 张福民, 曲兴华 2020 激光技术 44 1
Google Scholar
Bao W Z, Zhang F M, Qu X H 2020 Laser Technol. 44 1
Google Scholar
[20] Jiang S, Liu B, Wang H 2021 Appl. Opt. 60 918
Google Scholar
[21] Zhao Q W 2014 M. S. Thesis (Xiangtan: Xiangtan University) (in Chinese) [赵秋雯 2014 硕士学位论文 (湘潭: 湘潭大学)]
[22] Horne J H, Baliunas S L 1986 ApJ. 302 757
Google Scholar
[23] Press W H, Rybicki G B 1989 ApJ. 338 277
Google Scholar
[24] Sandborn P A M 2017 Ph. D. Dissertation (Berkeley: University of California)
下载:
计量
- 文章访问数: 3894
- PDF下载量: 86
- 被引次数: 0
