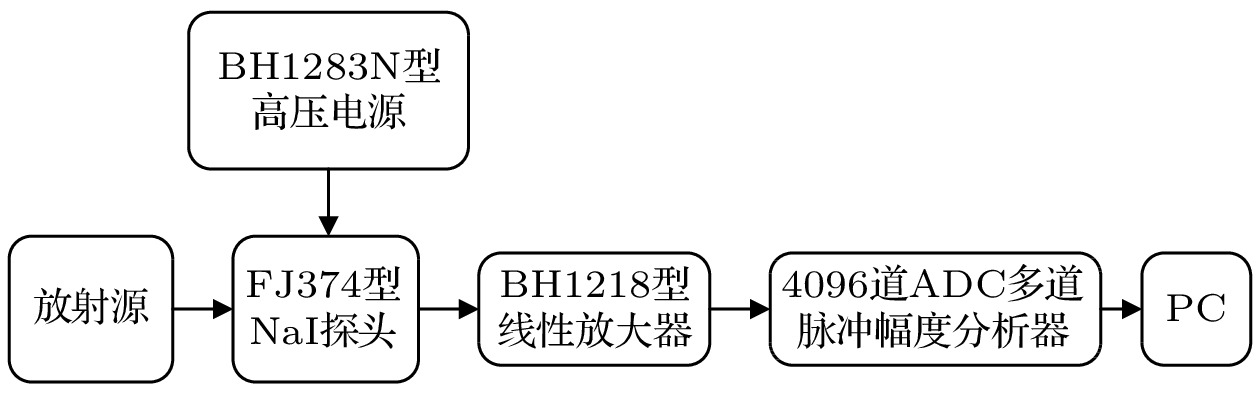
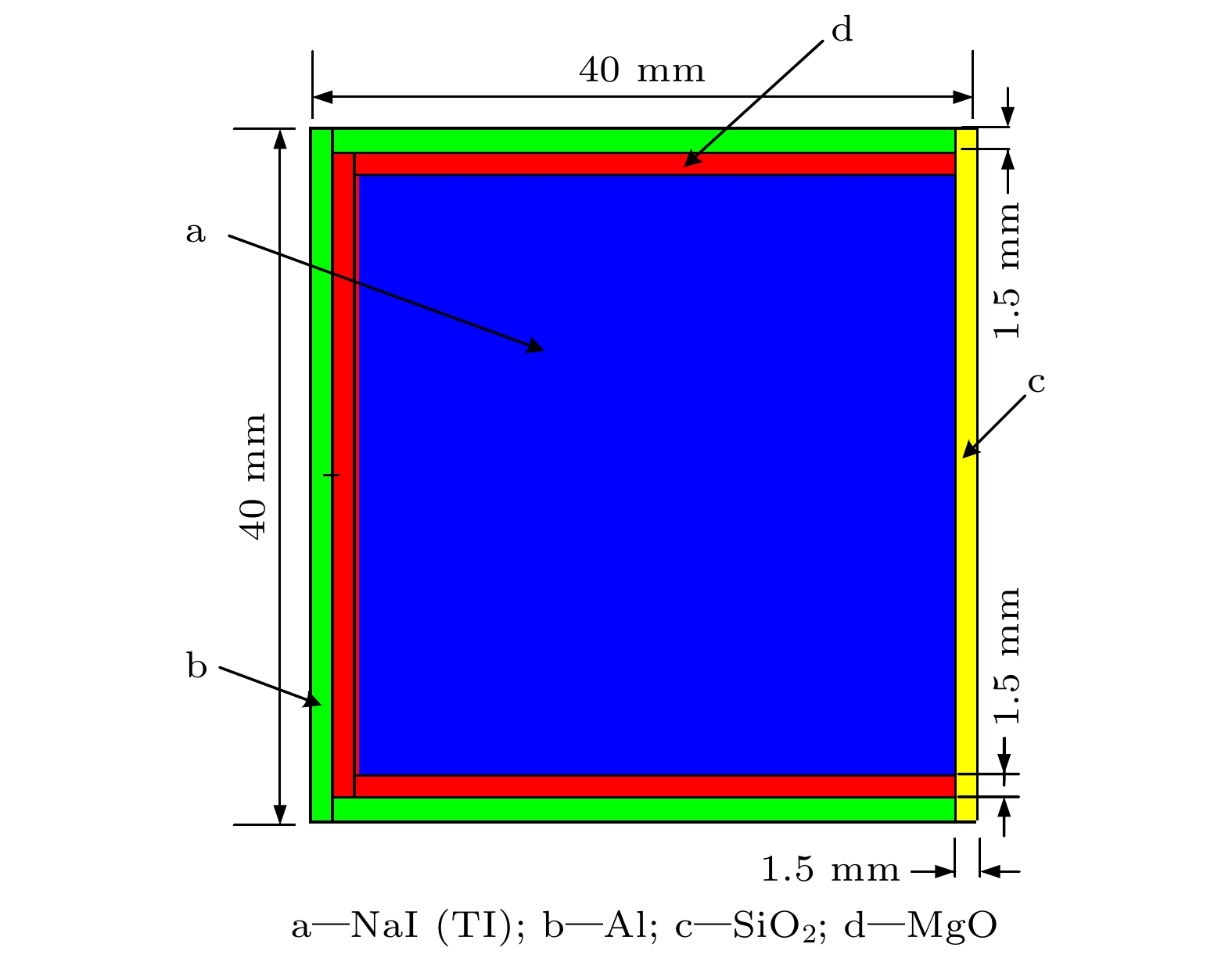
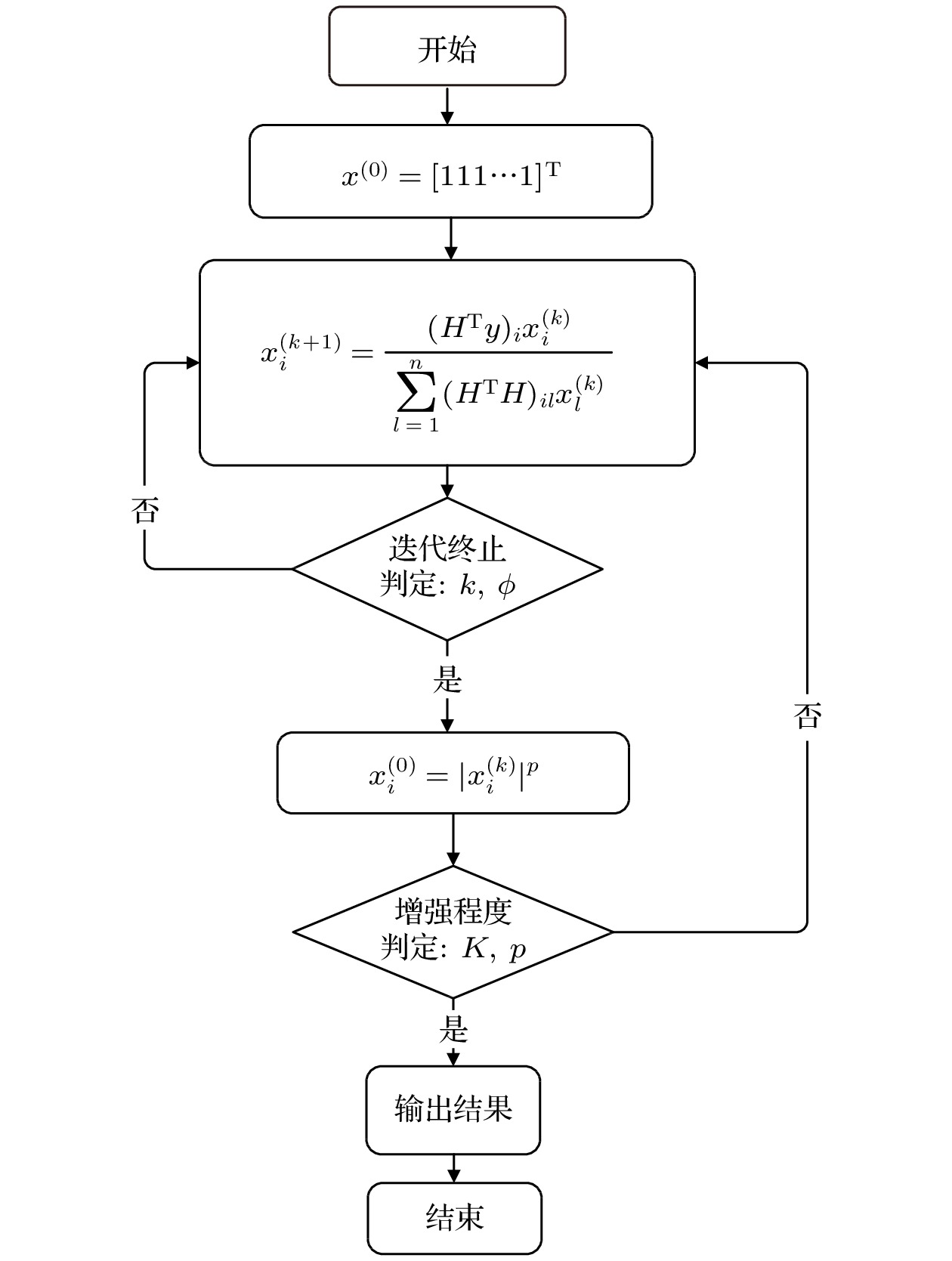
-
为了利用低能量分辨率探测器γ能谱分析获取未知放射性核素的特征信息, 提高γ能谱中重峰及弱峰分析的准确性和有效性, 本文开展了基于Boosted-Gold算法的NaI(Tl)探测器γ能谱分析研究. 采用MCNPX建立NaI(Tl)探测器模拟模型,获得了维度201×200的探测器响应矩阵. 基于Boosted-Gold算法开发了γ能谱反演程序. 实验测量了γ源22Na, 133Ba和152Eu的探测器响应能谱, 并以不同γ射线能量、不同能差
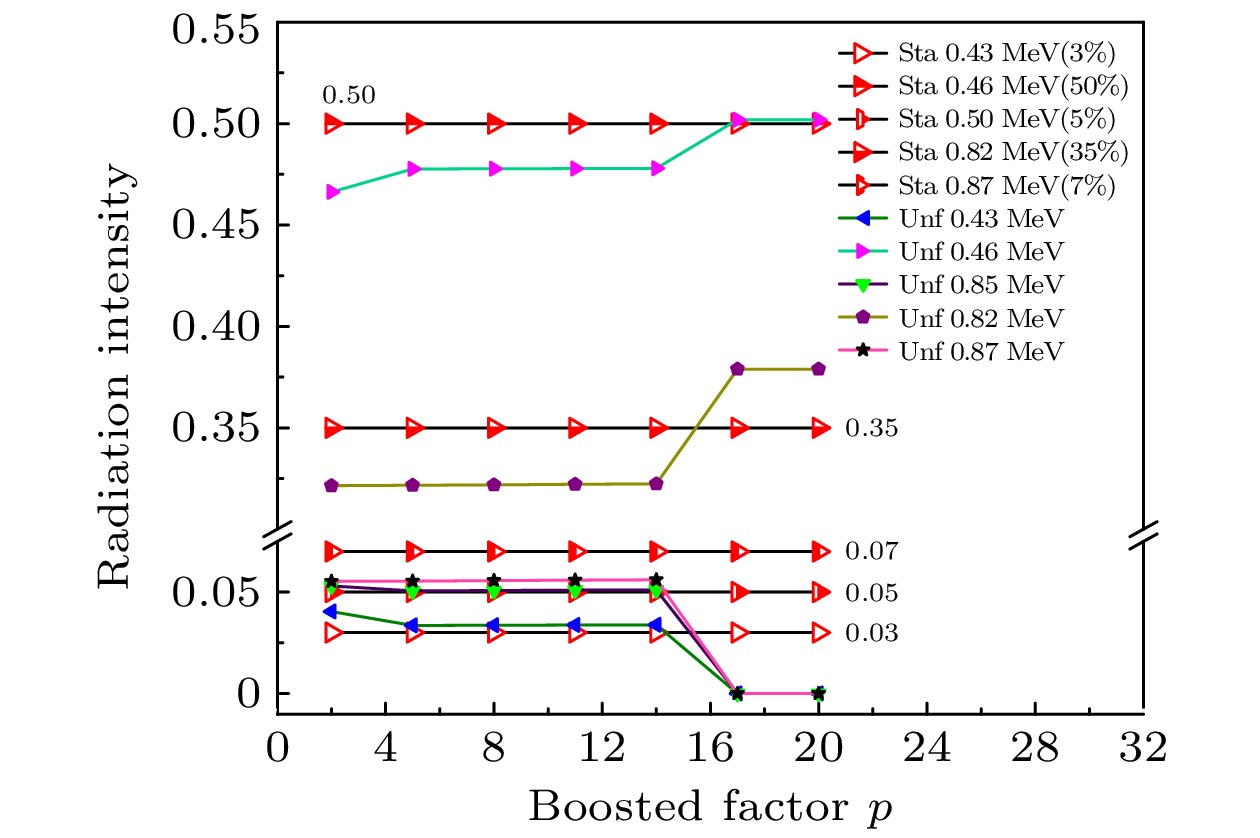
$(\Delta E)$ 、不同相对强度为条件构建了3组低分辨率模拟γ能谱, 结合响应矩阵及反演程序对实测γ能谱和模拟γ能谱进行反演. 以IAEA数据库核素标准特征信息对反演结果进行分析. 结果表明: Boosted-Gold算法对实测γ能谱特征能量反演误差最大为2.17% (133Ba源0.276MeV), 反演强度与标准强度最大差为0.197(152Eu源1.408 MeV). 对模拟γ能谱核素特征能量均可准确分析, 反演强度与标准强度差值保持在0.01以内; 当增强系数p≤14时, Boosted-Gold算法有利于γ放射性核素的定量分析, 对于相对强度大于10%的γ射线, 该算法具有更好的分析准确性.-
关键词:
- γ能谱分析 /
- 响应函数 /
- 能谱反演 /
- Boosted-Gold算法
To obtain the characteristic information of unknown radionuclides by analyzing the γ-energy spectrum of a low-resolution detector, and to improve the accuracy and validity of the analysis of overlapping and weak peaks in the γ-energy spectrum, in this paper we analyze the γ-energy spectrum of NaI(Tl) detectors based on the Boosted-Gold algorithm. A simulation model of NaI(TI) detector is established by using MCNPX, and a detector response matrix with dimension 201 × 200 is obtained. The γ-energy spectrum unfolding program is developed based on the Boosted-Gold algorithm. The detector response spectra of the γ radioactive sources 22Na, 133Ba, and 152Eu are measured. Three groups of low-resolution γ spectra are constructed with different γ-ray energy, different energy differences ($ \Delta E $ ) and different relative intensities by simulation. Combining the response matrix and the unfolding procedures, the measured and simulated γ energy spectra are unfolded. The unfolding results are analyzed with the nuclide standard characteristics information from the IAEA database. The results show that the maximum unfolding error of the characteristic energy of the measured γ-energy spectrum is 2.17% (0.276 MeV for 133Ba source) by the Boosted-Gold algorithm, and the maximum deviation between the unfolded intensity and the standard intensity is 0.197 (1.408 MeV for 152Eu source). For the simulated γ energy spectrum, the characteristic energy of nuclide can be accurately analyzed, and the deviation between unfolded intensity and standard intensity maintains 0.01. When the enhancement factor p ≤ 14, the Boosted-Gold algorithm is beneficial to the quantitative analysis of γ-radionuclides. For the relative intensity of γ-rays greater than 10%, this algorithm has better analysis accuracy.-
Keywords:
- γ energy spectrum analysis /
- response function /
- energy spectrum unfolding /
- Boosted-Gold algorithm
[1] Li F, Cheng Z Y, Tian C S, Xiao H F, Zhang M, Ge L Q 2020 Appl. Spectrosc. Rev. 56 255
[2] 陈晔 2021 博士学位论文 (北京: 军事科学院)
Chen Y 2021 Ph. D. Dissertation (Beijing: Academy of Military Sciences) (in Chinese)
[3] Rahman M S, Cho G, Kang B S 2009 Radiat. Prot. Dosim. 135 203
Google Scholar
[4] Alizadeh D, Ashrafi S 2019 Nucl. Instrum. Methods Phys. Res., Sect. A 915 1
Google Scholar
[5] Demir N, Kuluöztürk Z N 2021 Nucl. Eng. Technol. 53 3759
Google Scholar
[6] Milbrath B D, Choate B J, Fast J E, Hensley W K, Kouzes R T, Schweppe J E 2007 Nucl. Instrum. Methods Phys. Res., Sect. A 572 774
Google Scholar
[7] Baré J, Tondeur F 2011 Appl. Radiat. Isot. 69 1121
Google Scholar
[8] Morháč M, Matoušek V 2009 Digital Signal Proces. 19 372
Google Scholar
[9] Kwan E, Wu C Y, Haight R C, Lee H Y, Bredeweg T A, Chyzh A, Devlin M, Fotiades N, Gostic J M, Henderson R A, Jandel M, Laptev A, Nelson R O, O’Donnell J M, Perdue B A, Taddeucci T N, Ullmann J L, Wender S A 2014 Nucl. Data Sheets 119 221
Google Scholar
[10] Meng L J, Ramsden D 2000 IEEE Trans. Nucl. Sci. 47 1329
[11] Shi R, Tuo X G, Li H L, Xu Y Y, Shi F R, Yang J B, Luo Y 2018 Nucl. Sci. Tech. 29 10
Google Scholar
[12] Li L, Tuo X G, Liu M Z, Wang J 2014 Nucl. Sci. Tech. 25 050202
[13] Wachtmeister S, Csillag S 2011 Ultramicroscopy 111 79
Google Scholar
[14] Zhou R J, Zhong G Q, Hu L Q, Tardocchi M, Rigamonti D, Giacomelli L, Nocente M, Gorini G, Fan T S, Zhang Y M, Hu Z M, Xiao M, Li K, Zhang Y K, Hong B, Zhang Y, Lin S Y, Zhang J Z 2019 Rev. Sci. Instrum. 90 123510
Google Scholar
[15] Morháč M, Matoušek V 2011 J. Comput. Appl. Math. 235 1629
Google Scholar
[16] Jandel M, Morháč M, Kliman J, Krupa L, Matoušek V, Hamilton J H, Ramayya A V 2004 Nucl. Instrum. Methods Phys. Res., Sect. A 516 172
Google Scholar
[17] He J F, Yang Y Z, Qu J H, Wu Q F, Xiao H L, Yu C C 2016 Nucl. Sci. Tech. 27 111
Google Scholar
[18] 赵日, 刘立业, 曹勤剑 2019 原子能科学技术 53 1495
Google Scholar
Zhao R, Liu L Y, Cao Q J 2019 At. Energy Sci. Technol. 53 1495
Google Scholar
[19] Zhang S J, Liu C Q, Yang X, Huang C, Xie Q, Hu Z J, Hu Z M, Han C, Bai X H, Huo D Y, Wu K, Wang J R, Zhang Y, Wei Z, Yao Z E 2021 Nucl. Instrum. Methods Phys. Res., Sect. A 1006 165407
Google Scholar
[20] 艾宪芸, 魏义祥, 肖无云 2006 清华大学学报(自然科学版) 46 821
Google Scholar
Ai X Y, Wei Y X, Xiao W Y 2006 J. Tsinghua. Univ. (Sci. & Tech.) 46 821
Google Scholar
[21] Khilkevitch E M, Shevelev A E, Chugunov I N, Naidenov V O, Gin D B, Doinikov D N 2013 Tech. Phys. Lett. 39 63
Google Scholar
[22] Morháč M, Hlaváč S, Veselský M, Matoušek V 2010 Nucl. Instrum. Methods Phys. Res. , Sect. A 621 539
Google Scholar
[23] 吴和喜, 袁新宇, 刘庆成, 刘玉娟, 杨磊 2012 原子能科学技术 46 1142
Wu H X, Yuan X Y, Liu Q C, Liu Y J, Yang L 2012 At. Energy Sci. Technol. 46 1142
[24] Salgado C M, Brandão L E B, Schirru R, Pereira C M N A, Conti C C 2012 Prog. Nucl. Energy 59 19
Google Scholar
[25] 陈伟, 苏川英, 冯天成, 刘文彪, 田自宁 2018 核技术 41 70
Cheng W, Su C Y, Feng T C, Liu W B, Tian Z N 2018 Nucl. Tech. 41 70
-
图 4 能量(Eγ)与半高宽(FWHM)对应关系
Fig. 4. The correspondence between energy (Eγ) and half-maximum width (FWHM).
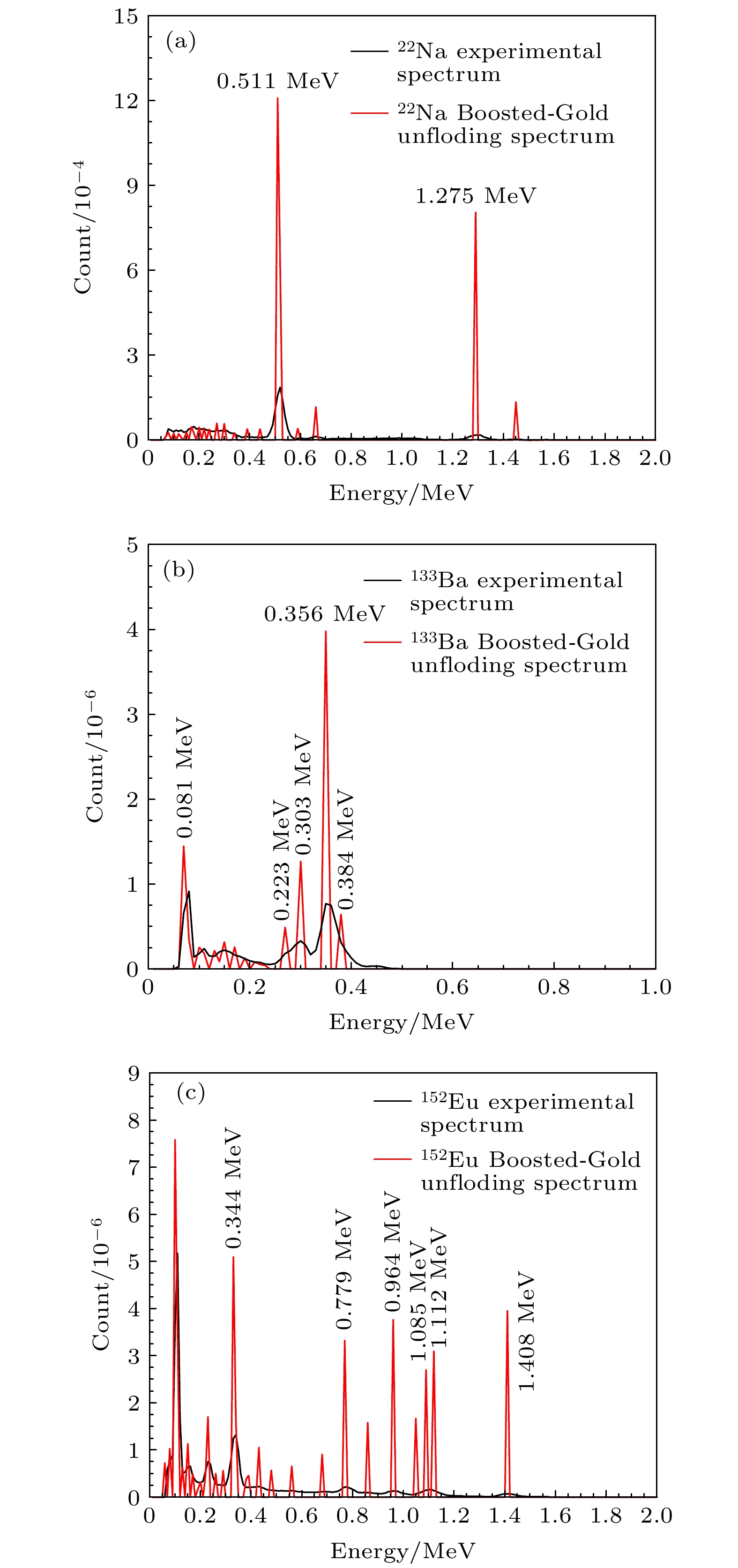
图 7 实测γ能谱与反演能谱的比较 (a) 22Naγ源能谱反演前后结果对比; (b)133Baγ源能谱反演前后结果对比; (c)152Eu γ源能谱反演前后结果对比
Fig. 7. The comparison between the measured γ energy spectra and the unfolded energy spectrum: (a) Comparison of the results before and after the unfolded of the energy spectrum of the 22Na γ source; (b) comparison of the results before and after the unfolded of the energy spectrum of the 133Baγ source; (c) comparison of the results before and after the algorithm unfolded of the energy spectrum of the 152Eu γ source.
图 8 模拟γ能谱反演前后结果对比 (a1) 4种能量γ射线模拟谱; (a2) 4种能量γ射线模拟谱反演前后结果对比; (b1)6种能量γ射线模拟谱; (b2) 6种能量γ射线模拟谱反演前后结果对比; (c1) 8种能量γ射线模拟谱; (c2) 8种能量γ射线模拟谱反演前后结果对比
Fig. 8. The comparison of between the results before and after the unfolding of the simulated γ energy spectrum: (a1) The simulation spectrum of gamma-rays of 4 energies; (a2) the comparison between the results before and after the unfolding of the simulated spectrum of energy γ-rays of 4 energies; (b1) the simulation spectrum of gamma-rays of 6 energies; (b2) the comparison between the results before and after the unfolding of the simulated spectrum of energy γ-rays of 6 energies; (c1) the simulation spectrum of gamma-rays of 8 energies; (c2) the comparison between the results before and after the unfolding of the simulated spectrum of energy γ-rays of 8 energies.
图 9 不同p值下标准相对强度数据与反演数据的对比
Fig. 9. The comparison of the standard relative intensity data and the unfolded data at different p-values.
表 1 实验谱与反演谱结果分析对比
Table 1. Analysis and comparison of the experimental spectrum and the unfolded spectrum results.
源 定性分析 定量分析 标准能量/MeV 反演能量/MeV 误差/% 标准谱
强度比反演谱
强度比偏差 22Na 0.511 0.51 0.2 1 1 0 1.275 1.29 1.18 0.556 0.438 0.118 133Ba 0.081 0.08 1.23 0.55 0.449 –0.101 0.276 0.27 2.17 0.115 0.123 0.008 0.303 0.30 0.99 0.295 0.319 0.024 0.356 0.35 1.69 1 1 0 0.384 0.38 1.04 0.144 0.162 0.018 152Eu 0.344 0.34 1.16 1 1 0 0.779 0.77 1.15 0.486 0.495 0.009 0.964 0.96 0.41 0.546 0.56 0.014 1.085 1.09 0.46 0.38 0.402 0.022 1.112 1.12 0.72 0.514 0.461 –0.053 1.408 1.4 0.57 0.785 0.588 –0.197 
下载: 导出CSV
表 2 模拟谱与反演谱结果对比
Table 2. Comparison of the simulated spectrum and the unfolded spectrum results.
谱 定性分析 定量分析 标准能量/MeV 反演能量/MeV 标准谱强度比 反演谱强度比 偏差 谱1 0.43 0.43 0.3 0.302 0.002 0.47 0.47 1 1 0 0.5 0.5 0.3 0.307 0.007 0.54 0.54 0.4 0.4 0 谱2 0.67 0.67 0.4 0.403 0.003 0.71 0.71 1 0.996 –0.004 0.76 0.76 0.4 0.397 –0.003 0.81 0.81 0.6 0.598 –0.002 1.03 1.03 1 1 0 1.08 1.08 0.6 0.602 0.002 谱3 0.63 0.63 0.5 0.49 –0.01 0.67 0.67 1 1 0 0.72 0.72 0.5 0.495 –0.005 0.77 0.77 0.5 0.495 –0.005 1.28 1.28 0.75 0.744 –0.006 1.34 1.34 0.5 0.494 –0.006 1.39 1.39 0.75 0.752 0.002 1.45 1.45 0.5 0.486 –0.014
下载: 导出CSV
-
[1] Li F, Cheng Z Y, Tian C S, Xiao H F, Zhang M, Ge L Q 2020 Appl. Spectrosc. Rev. 56 255
[2] 陈晔 2021 博士学位论文 (北京: 军事科学院)
Chen Y 2021 Ph. D. Dissertation (Beijing: Academy of Military Sciences) (in Chinese)
[3] Rahman M S, Cho G, Kang B S 2009 Radiat. Prot. Dosim. 135 203
Google Scholar
[4] Alizadeh D, Ashrafi S 2019 Nucl. Instrum. Methods Phys. Res., Sect. A 915 1
Google Scholar
[5] Demir N, Kuluöztürk Z N 2021 Nucl. Eng. Technol. 53 3759
Google Scholar
[6] Milbrath B D, Choate B J, Fast J E, Hensley W K, Kouzes R T, Schweppe J E 2007 Nucl. Instrum. Methods Phys. Res., Sect. A 572 774
Google Scholar
[7] Baré J, Tondeur F 2011 Appl. Radiat. Isot. 69 1121
Google Scholar
[8] Morháč M, Matoušek V 2009 Digital Signal Proces. 19 372
Google Scholar
[9] Kwan E, Wu C Y, Haight R C, Lee H Y, Bredeweg T A, Chyzh A, Devlin M, Fotiades N, Gostic J M, Henderson R A, Jandel M, Laptev A, Nelson R O, O’Donnell J M, Perdue B A, Taddeucci T N, Ullmann J L, Wender S A 2014 Nucl. Data Sheets 119 221
Google Scholar
[10] Meng L J, Ramsden D 2000 IEEE Trans. Nucl. Sci. 47 1329
[11] Shi R, Tuo X G, Li H L, Xu Y Y, Shi F R, Yang J B, Luo Y 2018 Nucl. Sci. Tech. 29 10
Google Scholar
[12] Li L, Tuo X G, Liu M Z, Wang J 2014 Nucl. Sci. Tech. 25 050202
[13] Wachtmeister S, Csillag S 2011 Ultramicroscopy 111 79
Google Scholar
[14] Zhou R J, Zhong G Q, Hu L Q, Tardocchi M, Rigamonti D, Giacomelli L, Nocente M, Gorini G, Fan T S, Zhang Y M, Hu Z M, Xiao M, Li K, Zhang Y K, Hong B, Zhang Y, Lin S Y, Zhang J Z 2019 Rev. Sci. Instrum. 90 123510
Google Scholar
[15] Morháč M, Matoušek V 2011 J. Comput. Appl. Math. 235 1629
Google Scholar
[16] Jandel M, Morháč M, Kliman J, Krupa L, Matoušek V, Hamilton J H, Ramayya A V 2004 Nucl. Instrum. Methods Phys. Res., Sect. A 516 172
Google Scholar
[17] He J F, Yang Y Z, Qu J H, Wu Q F, Xiao H L, Yu C C 2016 Nucl. Sci. Tech. 27 111
Google Scholar
[18] 赵日, 刘立业, 曹勤剑 2019 原子能科学技术 53 1495
Google Scholar
Zhao R, Liu L Y, Cao Q J 2019 At. Energy Sci. Technol. 53 1495
Google Scholar
[19] Zhang S J, Liu C Q, Yang X, Huang C, Xie Q, Hu Z J, Hu Z M, Han C, Bai X H, Huo D Y, Wu K, Wang J R, Zhang Y, Wei Z, Yao Z E 2021 Nucl. Instrum. Methods Phys. Res., Sect. A 1006 165407
Google Scholar
[20] 艾宪芸, 魏义祥, 肖无云 2006 清华大学学报(自然科学版) 46 821
Google Scholar
Ai X Y, Wei Y X, Xiao W Y 2006 J. Tsinghua. Univ. (Sci. & Tech.) 46 821
Google Scholar
[21] Khilkevitch E M, Shevelev A E, Chugunov I N, Naidenov V O, Gin D B, Doinikov D N 2013 Tech. Phys. Lett. 39 63
Google Scholar
[22] Morháč M, Hlaváč S, Veselský M, Matoušek V 2010 Nucl. Instrum. Methods Phys. Res. , Sect. A 621 539
Google Scholar
[23] 吴和喜, 袁新宇, 刘庆成, 刘玉娟, 杨磊 2012 原子能科学技术 46 1142
Wu H X, Yuan X Y, Liu Q C, Liu Y J, Yang L 2012 At. Energy Sci. Technol. 46 1142
[24] Salgado C M, Brandão L E B, Schirru R, Pereira C M N A, Conti C C 2012 Prog. Nucl. Energy 59 19
Google Scholar
[25] 陈伟, 苏川英, 冯天成, 刘文彪, 田自宁 2018 核技术 41 70
Cheng W, Su C Y, Feng T C, Liu W B, Tian Z N 2018 Nucl. Tech. 41 70
下载:
计量
- 文章访问数: 5063
- PDF下载量: 123
- 被引次数: 0
