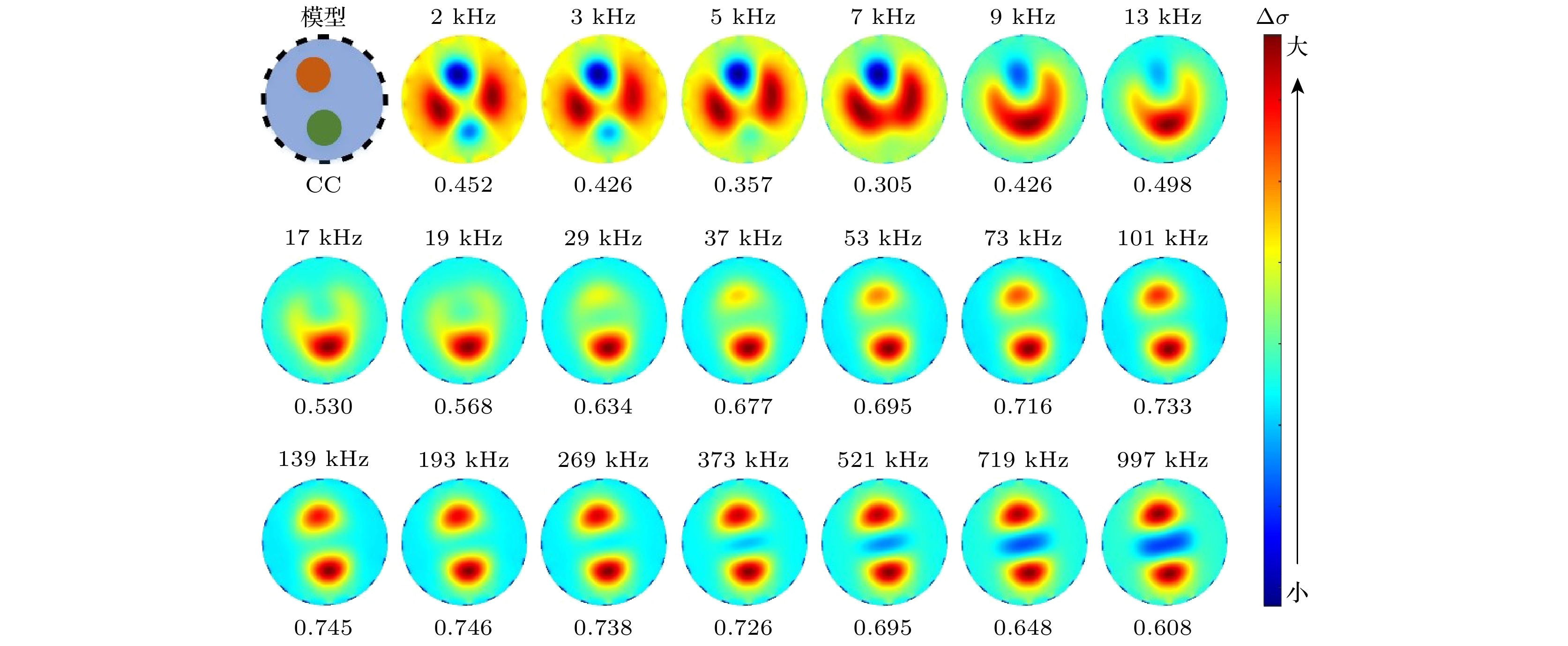
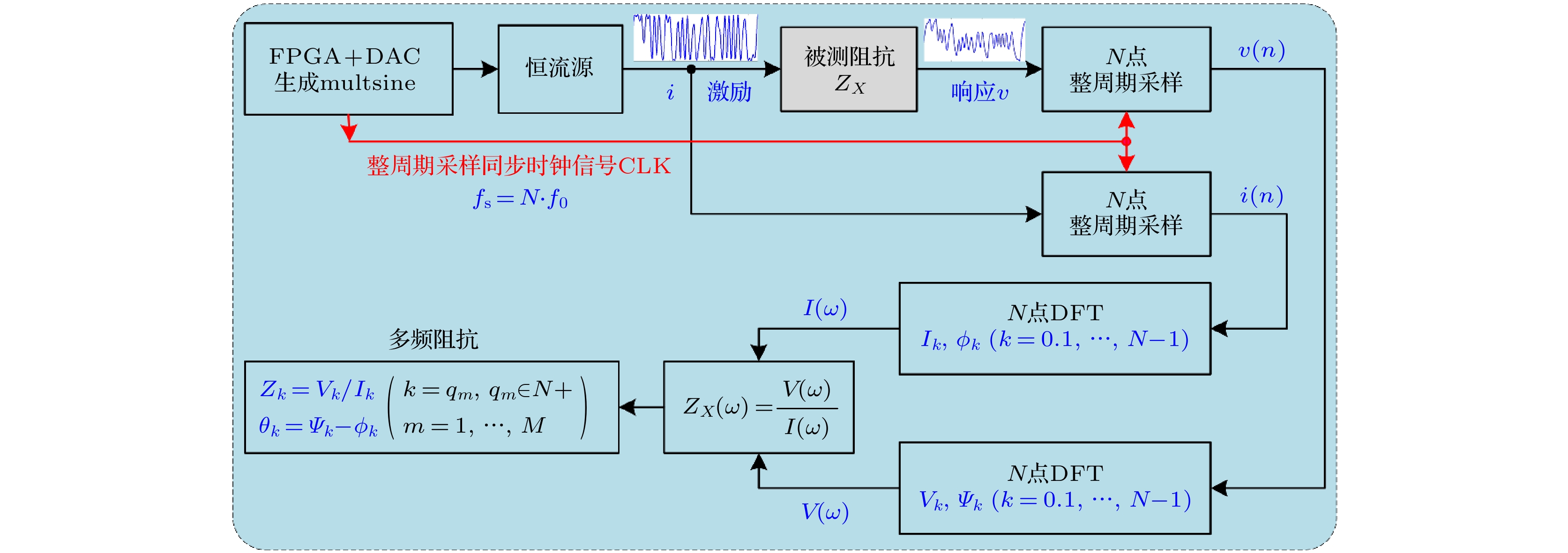
-
本文从周期信号的整周期采样无频谱泄露这一原理出发, 提出基于multisine信号的整周期采样理论, 从理论上推导出满足multisine整周期采样的采样率设置条件, 构建了基于FPGA+数模转换器+模数转换器的整周期采样实现方法, 研制了一种基于multisine激励和整周期采样的新型多频电阻抗成像(mfEIT)系统; 设计了胡萝卜棒+黄瓜棒的双目标成像模型, 并进行了多频时差成像和频差成像实验. 实验表明, 本mfEIT系统能够在一个基波周期(1 ms)内实现20个频率点(2—997 kHz)多目标组织边界的全频阻抗测量, 成像结果可区分具有不同电特性生物组织的结构与位置. 本文提出的基于multisine信号的整周期采样理论及其实现方法, 只需一个multisine基波周期即可完成一次全频阻抗测量, 为研制高速mfEIT系统奠定了理论和技术基础.Starting from the principle that the integer-period sampling (IPS) of periodic signals is free of spectrum leakage, in this paper we propose the multisine-IPS theory, deduce theoretically the sampling rate setting formula of multisine-IPS condition for the first time, and build its realization method based on field-programmable gate array (FPGA) plus digital-to-analog converter (DAC) plus analog-to-digital converter (ADC). A new multi-frequency electrical impedance tomography (mfEIT) system based on multisine excitation and its IPS theory is developed, and a dual-target imaging model including a carrot stick and a cucumber stick is designed. The experiments of multi-frequency time-difference imaging and frequency-difference imaging are carried out on the mfEIT system. The experimental results show that the newly-designed mfEIT system can achieve full-band impedance measurements on multiple objective tissue boundary at 20 frequency points (2–997 kHz) within one fundamental period (1 ms), and the structure and position of biological tissues with different electrical properties can also be distinguished from the resulting images. The proposed multisine-IPS theory and its implementation method can complete a full-band impedance measurement within one multisine fundamental period, which lays a theoretical and technical foundation for developing high-speed mfEIT system.
[1] Wu Y, Chen B, Liu K, Zhu C, Pan H, Jia J, Wu H, Yao J 2021 IEEE Sens. J. 21 9277
Google Scholar
[2] Coolen T, Lolli V, Sadeghi N, Rovai A, Trotta N, Taccone F S, Creteur J, Henrard S, Goffard J C, Dewitte O J N 2020 Neurology 95 e2016
Google Scholar
[3] Adhikari, Das N C 2020 Int. J. Comput. 38 73
[4] Cherepenin V, Karpov A, Korjenevsky A, Kornienko V, Mazaletskaya A, Mazourov D, Meister D 2001 Physiol. Meas. 22 9
Google Scholar
[5] Pulletz S, Adler A, Kott M, Elke G, Gawelczyk B, Schädler D, Zick G, Weiler N, Frerichs I 2012 J. Crit. Care 27 323
[6] Aristovich K Y, Packham B C, Koo H, Dos Santos G S, McEvoy A, Holder D S 2016 Neuro Image 124 204
Google Scholar
[7] Chen H, Yao J, Yang L, Liu K, Chen B, Li J, Takei M 2020 IEEE Sens. J. 21 3653
[8] Aguiar S S, Robens A, Boehm A, Leonhardt S, Teichmann D 2016 Sensors 16 1158
[9] Grimnes S, Martinsen O G 2015 Bioimpedance and Bioelectricity Basics (3rd Ed.) (London: Academic Press)
[10] Goren N, Avery J, Dowrick T, Mackle E, Witkowska-Wrobel A, Werring D, Holder D 2018 Sci. Data 5 180112
Google Scholar
[11] 姚佳烽, 万建芬, 杨璐, 刘凯, 陈柏, 吴洪涛 2020 69 163301
Google Scholar
Yao J F, Wan J F, Yang L, Liu K, Chen B, Wu H T 2020 Acta Phys. Sin. 69 163301
Google Scholar
[12] Padilha Leitzke J, Zangl H 2020 Sensors 20 5160
Google Scholar
[13] Yunjie Y, Jiabin J 2017 Rev. Sci. Instrum. 88 085110
Google Scholar
[14] Yang L, Xu C, Dai M, Fu F, Shi X, Dong X 2016 Physiol. Meas. 37 2317
Google Scholar
[15] Cao L, Li H, Xu C, Dai M, Ji Z, Shi X, Dong X, Fu F, Yang B 2019 Biomed. Eng. Online 18 84
Google Scholar
[16] Bai X, Liu D, Wei J, Bai X, Sun S, Tian W 2021 Biosensors 11 176
Google Scholar
[17] Avery J, Dowrick T, Faulkner M, Goren N, Holder D 2017 Sensors 17 280
Google Scholar
[18] Louarroudi E, Sanchez B 2017 Physiol. Meas. 38 N73
Google Scholar
[19] Kallel A Y, Bouchaala D, Kanoun O 2021 Meas. Sci. Technol. 32 084011
Google Scholar
[20] Oh T I, Koo H, Lee K H, Kim S M, Lee J, Kim S W, Seo J K, Woo E J 2008 Physiol. Meas. 29 295
Google Scholar
[21] Jaan O, Mart M 2018 J. Electrical Bioimpedance 9 133
Google Scholar
[22] Yuxiang Y, Lianhuan W, Peipei W, Xiufang Y, Fu Z, He W, Zhaosheng T 2015 Physiol. Meas. 36 1995
Google Scholar
[23] Kusche R, Malhotra A, Ryschka M, Ardelt G, Klimach P, Kaufmann S 2015 Electronics 4 507
Google Scholar
[24] Tan C, Liu S, Jia J, Dong F 2020 IEEE Trans. Instrum. Meas. 69 144
Google Scholar
[25] Min M, Land R, Paavle T, Parve T, Annus P, Trebbels D 2011 Physiol. Meas. 32 945
Google Scholar
[26] Sanchez B, Vandersteen G, Bragos R, Schoukens J 2012 Meas. Sci. Technol. 23 105501
Google Scholar
[27] Yan Z, Xu Y, Han B, Dong F 2020 IEEE Trans. Instrum. Meas. 70 1
[28] Yang Y, Zhang F, Tao K, Sanchez B, Wen H, Teng Z 2015 Physiol. Meas. 36 895
Google Scholar
[29] Schoukens J, Pintelon R, van der Ouderaa E, Renneboog J 1988 IEEE Trans. Instrum. Meas. 37 342
Google Scholar
[30] Schroeder M R 1970 IEEE Trans. Inform. Theory 16 85
Google Scholar
[31] Ojarand J, Min M, Annus P 2014 Physiol. Meas. 35 1019
Google Scholar
[32] 邓娟, 陈素华, 沙洪, 赵舒, 任超世 2012 中国生物医学工程学报 31 807
Google Scholar
Deng J, Chen S H, Sha H, Zhao S, Ren C S. 2012 Chinese J. Biomed. Eng. 31 807
Google Scholar
[33] Kao T J, Isaacson D, Newell J C, Saulnier G J 2006 Physiol. Meas. 27 S1
Google Scholar
[34] Yang Y, Jia J 2017 IEEE Trans. Instrum. Meas. 66 2295
Google Scholar
-
图 1 基于multisine整周期采样的多频阻抗测量原理图
Fig. 1. Schematic diagram of multi-frequency impedance measurement based on integer-period sampling.
图 2 基于FPGA的mfEIT系统结构原理图
Fig. 2. System structure diagram of the mfEIT system based on FPGA.
图 4 Multisine信号 (a)时域波形; (b)频谱分布
Fig. 4. Multisine signal: (a) Time domain waveform; (b) spectrum distribution.
表 1 合成的包含20个等幅值伪对数频谱分布的multisine信号频率、相位
Table 1. Frequencies and phases of the synthesized multisine signal with equivalent amplitude and pseudo-logarithmic spectral distribution.
谐波次数
qm频率fm/kHz
fm = qmf0相位φm
/rad谐波次数
qm频率fm/kHz
fm = qmf0相位φm
/radq1 2 1.3533 q11 53 –1.2911 q2 3 0.7238 q12 73 1.6648 q3 5 0.0514 q13 101 –1.0052 q4 7 –0.2595 q14 139 2.2122 q5 11 0.4941 q15 193 2.0298 q16 13 –0.4792 q16 269 –0.8110 q17 17 –0.0024 q17 373 1.9261 q18 19 –2.1192 q18 521 1.4672 q19 29 1.9941 q19 719 0.2117 q10 37 1.6186 q20 997 0.4343 
下载: 导出CSV
表 2 Multisine信号20个频率点的通道信噪比平均值及标准差
Table 2. Average and standard deviation of the channel SNR at 20 frequency points of the multisine signal.
频率fm
/kHz信噪比SNR
/dB标准差
/ ±频率fm
/kHz信噪比SNR
/dB标准差
/ ±2 46.7 4.8 53 55.7 6.0 3 51.3 5.4 73 55.8 5.7 5 53.4 6.2 101 54.7 6.0 7 55.0 5.8 139 51.6 5.0 11 55.4 6.3 193 54.6 6.1 13 55.4 6.4 269 56.1 6.7 17 54.7 6.3 373 57.0 7.3 19 54.6 5.9 521 58.1 7.9 29 53.6 5.5 719 56.0 7.6 37 55.1 5.8 997 56.6 8.0
下载: 导出CSV
-
[1] Wu Y, Chen B, Liu K, Zhu C, Pan H, Jia J, Wu H, Yao J 2021 IEEE Sens. J. 21 9277
Google Scholar
[2] Coolen T, Lolli V, Sadeghi N, Rovai A, Trotta N, Taccone F S, Creteur J, Henrard S, Goffard J C, Dewitte O J N 2020 Neurology 95 e2016
Google Scholar
[3] Adhikari, Das N C 2020 Int. J. Comput. 38 73
[4] Cherepenin V, Karpov A, Korjenevsky A, Kornienko V, Mazaletskaya A, Mazourov D, Meister D 2001 Physiol. Meas. 22 9
Google Scholar
[5] Pulletz S, Adler A, Kott M, Elke G, Gawelczyk B, Schädler D, Zick G, Weiler N, Frerichs I 2012 J. Crit. Care 27 323
[6] Aristovich K Y, Packham B C, Koo H, Dos Santos G S, McEvoy A, Holder D S 2016 Neuro Image 124 204
Google Scholar
[7] Chen H, Yao J, Yang L, Liu K, Chen B, Li J, Takei M 2020 IEEE Sens. J. 21 3653
[8] Aguiar S S, Robens A, Boehm A, Leonhardt S, Teichmann D 2016 Sensors 16 1158
[9] Grimnes S, Martinsen O G 2015 Bioimpedance and Bioelectricity Basics (3rd Ed.) (London: Academic Press)
[10] Goren N, Avery J, Dowrick T, Mackle E, Witkowska-Wrobel A, Werring D, Holder D 2018 Sci. Data 5 180112
Google Scholar
[11] 姚佳烽, 万建芬, 杨璐, 刘凯, 陈柏, 吴洪涛 2020 69 163301
Google Scholar
Yao J F, Wan J F, Yang L, Liu K, Chen B, Wu H T 2020 Acta Phys. Sin. 69 163301
Google Scholar
[12] Padilha Leitzke J, Zangl H 2020 Sensors 20 5160
Google Scholar
[13] Yunjie Y, Jiabin J 2017 Rev. Sci. Instrum. 88 085110
Google Scholar
[14] Yang L, Xu C, Dai M, Fu F, Shi X, Dong X 2016 Physiol. Meas. 37 2317
Google Scholar
[15] Cao L, Li H, Xu C, Dai M, Ji Z, Shi X, Dong X, Fu F, Yang B 2019 Biomed. Eng. Online 18 84
Google Scholar
[16] Bai X, Liu D, Wei J, Bai X, Sun S, Tian W 2021 Biosensors 11 176
Google Scholar
[17] Avery J, Dowrick T, Faulkner M, Goren N, Holder D 2017 Sensors 17 280
Google Scholar
[18] Louarroudi E, Sanchez B 2017 Physiol. Meas. 38 N73
Google Scholar
[19] Kallel A Y, Bouchaala D, Kanoun O 2021 Meas. Sci. Technol. 32 084011
Google Scholar
[20] Oh T I, Koo H, Lee K H, Kim S M, Lee J, Kim S W, Seo J K, Woo E J 2008 Physiol. Meas. 29 295
Google Scholar
[21] Jaan O, Mart M 2018 J. Electrical Bioimpedance 9 133
Google Scholar
[22] Yuxiang Y, Lianhuan W, Peipei W, Xiufang Y, Fu Z, He W, Zhaosheng T 2015 Physiol. Meas. 36 1995
Google Scholar
[23] Kusche R, Malhotra A, Ryschka M, Ardelt G, Klimach P, Kaufmann S 2015 Electronics 4 507
Google Scholar
[24] Tan C, Liu S, Jia J, Dong F 2020 IEEE Trans. Instrum. Meas. 69 144
Google Scholar
[25] Min M, Land R, Paavle T, Parve T, Annus P, Trebbels D 2011 Physiol. Meas. 32 945
Google Scholar
[26] Sanchez B, Vandersteen G, Bragos R, Schoukens J 2012 Meas. Sci. Technol. 23 105501
Google Scholar
[27] Yan Z, Xu Y, Han B, Dong F 2020 IEEE Trans. Instrum. Meas. 70 1
[28] Yang Y, Zhang F, Tao K, Sanchez B, Wen H, Teng Z 2015 Physiol. Meas. 36 895
Google Scholar
[29] Schoukens J, Pintelon R, van der Ouderaa E, Renneboog J 1988 IEEE Trans. Instrum. Meas. 37 342
Google Scholar
[30] Schroeder M R 1970 IEEE Trans. Inform. Theory 16 85
Google Scholar
[31] Ojarand J, Min M, Annus P 2014 Physiol. Meas. 35 1019
Google Scholar
[32] 邓娟, 陈素华, 沙洪, 赵舒, 任超世 2012 中国生物医学工程学报 31 807
Google Scholar
Deng J, Chen S H, Sha H, Zhao S, Ren C S. 2012 Chinese J. Biomed. Eng. 31 807
Google Scholar
[33] Kao T J, Isaacson D, Newell J C, Saulnier G J 2006 Physiol. Meas. 27 S1
Google Scholar
[34] Yang Y, Jia J 2017 IEEE Trans. Instrum. Meas. 66 2295
Google Scholar
下载:
计量
- 文章访问数: 4669
- PDF下载量: 127
- 被引次数: 0
