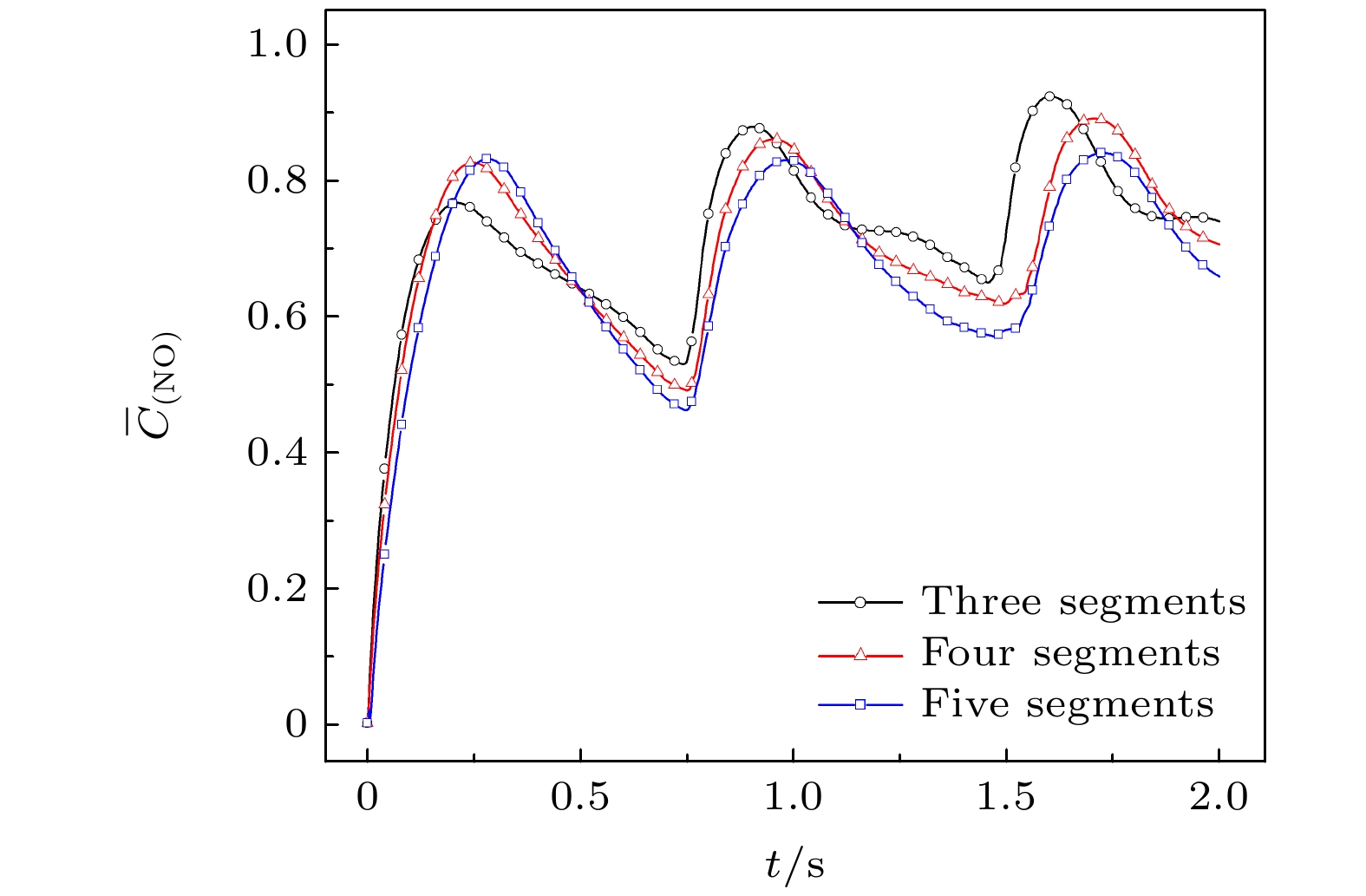
-
淋巴系统对人体的免疫及细胞的内环境稳态都有着重要的作用. 与血液循环系统相似, 淋巴系统也是遍布全身的管道系统, 主要由淋巴液、淋巴管和淋巴器官构成. 淋巴管的自发收缩驱动管内淋巴液的流动. 淋巴管的自发收缩-舒张机制由Ca2+和NO浓度的振荡反馈决定, NO在管内的分布对淋巴管的收缩循环起到重要作用. 因淋巴液流动而作用在淋巴瓣膜上的剪切力是瓣膜产生NO的主要原因. 在真实系统中, 某段淋巴管中的NO分布会受到与其连接的淋巴管的影响, 特别是上游的连接片段. 通过晶格玻尔兹曼方法, 建立了1个具有瓣膜结构的多段淋巴管模型, 再现了淋巴管内Ca2+和NO的反馈机制, 瓣膜变化和淋巴液流动情况. 该模型中存在3种淋巴管, 分别是初始淋巴管、中间淋巴管和出口淋巴管. 淋巴管的段数可以通过修改计算参数无限扩充. 本文计算的段数为3—5段, 每段淋巴管中有两对瓣膜. 通过模型研究了多段淋巴管中NO浓度分布、压力分布、NO平均浓度变化, 以及3段管模型中各管的流量随时间变化情况.The lymphatic system plays an important part in the body’s immunity and cell’s internal environment homeostasis. Like a blood circulatory system, the lymphatic system is a piping system throughout the body, which is composed mainly of lymphatic fluid and lymphatic vessels. The spontaneous contraction of the lymphatic vessels drives the flow of lymphatic fluid in the vessels. The spontaneous contraction-relaxation mechanism of lymphatic vessels is determined by the oscillating feedback of Ca2+ concentration and NO concentration. The distribution of NO in the vessels plays an important role in the contraction cycle of lymphatic vessels. The shear force acting on the lymphatic valves due to the flow of fluid is the main source of NO. In a real system, the distribution of NO in a certain section of lymphatic vessel will be affected by other lymphanion connected to it, especially the upstream connecting fragments. Through the lattice Boltzmann method, a multi-segment lymphatic vessel model with valve structure is established, which reproduces the feedback mechanism of Ca2+ and NO, valve change and fluid flow. There are three types of lymphatic vessels in the model, namely the initial lymphatic vessel, the collecting lymphatic vessel, and the outlet lymphatic vessel. The number of lymphatic vessels can be unlimited and inputted by the parameters. The number of lymphatic vessels is 3－5, and there are two pairs of valves in each lymphatic vessel. In this paper studied are the distribution of NO and pressure in multi-segment lymphatic vessel, and the change in the flow of each vessel in the three-segment vessel model over time.
-
Keywords:
- multi-segment lymphatic vessel /
- lattice Boltzmann method /
- lymphatic valve /
- NO /
- flux
[1] Matthew B, Wolf K T, Zhanna N 2018 Biomech. Model. Mechanobiol. 17 1343
Google Scholar
[2] Grimaldi A, Moriondo A, Sciacca L, Guidali M L, Tettamanti G, Negrini D 2006 Am. J. Physiol. Heart. Circ. Physiol. 291 876
Google Scholar
[3] Schmid-Schonbein G W 1990 Physiol. Rev. 70 987
[4] Gashev A A 2002 Ann. N. Y. Acad. Sci. 979 178
[5] Gasheva O Y, Zawieja D C, Gashev A A 2006 J. Physiol. 575 821
Google Scholar
[6] Zawieja, David C 2009 Lymphat. Res. Biol. 7 87
Google Scholar
[7] EisenhofferJ, Kagal A, Klein T, Johnston M 1995 Microvasc. Res. 49 97
Google Scholar
[8] 秦立鹏, 牛春雨, 赵自刚 2011 生理科学进展 42 237
Qin L P, Niu C Y, Zhao Z G 2011 Advances in Physiological Sciences. 42 237
[9] Margaris K N, Black R A 2012 J. R. Soc. Interface. 9 601
Google Scholar
[10] Bertram C D, Macaskill C, Moore J E 2011 J. Biomech. Eng. 133 11008
Google Scholar
[11] Bertram C D, Macaskill C, Jr J E M 2014 Comput. Methods. Biomech. Biomed. Eng. 17 1519
Google Scholar
[12] 朱炼华, 郭照立 2015 计算物理 32 20
Google Scholar
Zhu L H, Guo Z L 2015 Chin. J. Comput. Phys. 32 20
Google Scholar
[13] 李华兵 2004 博士学位论文 (上海: 复旦大学)
Li H B 2004 Ph. D. Dissertation (Shanghai: Fudan University) (in Chinese)
[14] Shan X, Chen H 1993 Phys. Rev. E 47 1815
[15] Mcnamara G R, Zanetti G 1988 Phys. Rev. Lett. 61 2332
Google Scholar
[16] Chen H, Chen S, Matthaeus W H 1992 Phys. Rev. A. 45 5339
Google Scholar
[17] Qian Y. H, D'Humières D, Lallemand P 1992 Euro. Phys. Lett. 17 479
Google Scholar
[18] Ladd A J C, Verberg R 2001 J. Stat. Phys. 104 1191
Google Scholar
[19] Baish J W, Kunert C, Padera T P, Munn L L 2016 PloS Comput. Biol. 12 e1005231
Google Scholar
[20] Bazigou E, Wilson J T 2014 Microvasc. Res. 96 38
Google Scholar
[21] Damarla M, Zaeh S, Niedermeyer S 2020 Am. J. Respir. Crit. Care. Med. 202 4
Google Scholar
[22] Li H B, Mei Y M, Maimon N 2019 Sci. Rep. 9 2014
Google Scholar
-
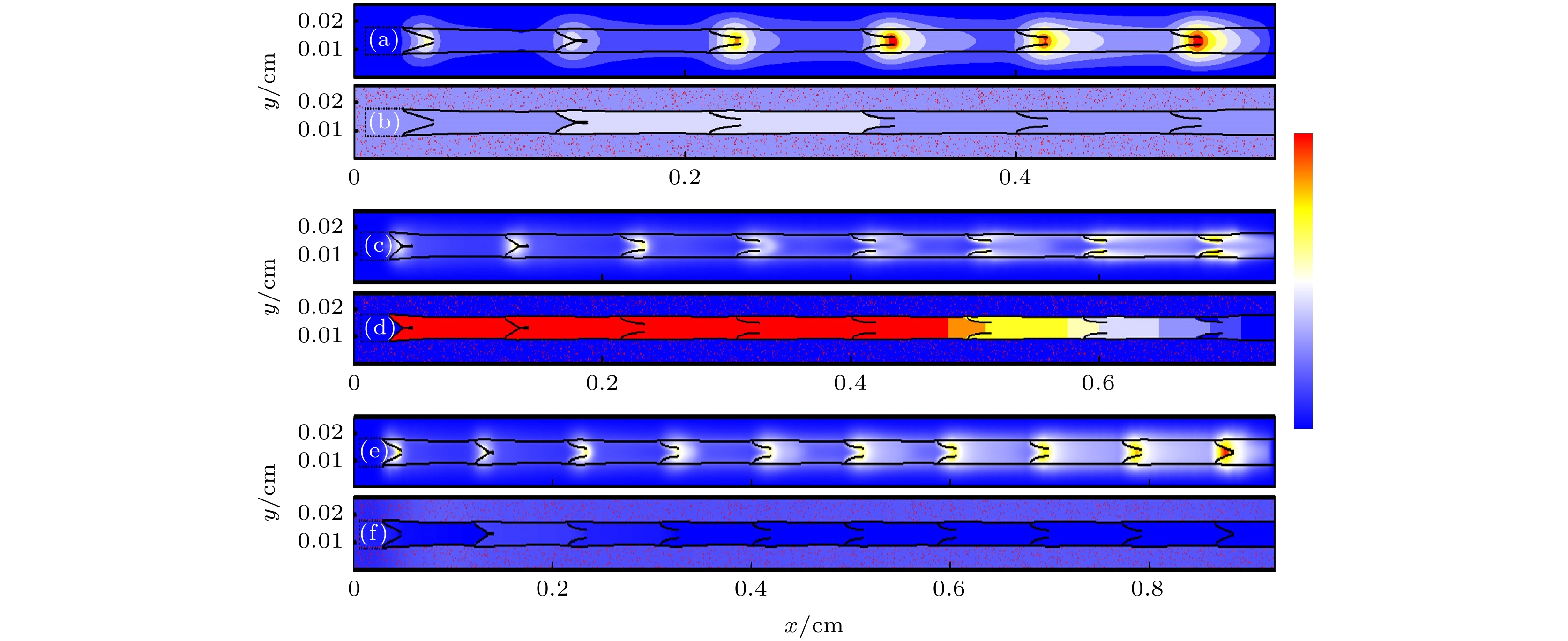
图 4 淋巴管的段数不同时, (a), (c), (e) NO浓度和(b), (d), (f)压力分布(颜色标尺顶部红色部分最大压强为3.045 × 103 Pa, 底部蓝色部分最小压强为3.010 × 103 Pa) (a), (b) 3段; (c), (d) 4段; (e), (f) 5段
Fig. 4. (a), (c), (e) NO concentration and (b), (d), (f) pressure distribution for different segment lymphatic vessel (The maximum pressure of the red part at the top of the color scale is 3.045 × 103 Pa, and the minimum pressure of the blue part at the bottom is 3.010 × 103 Pa): (a), (b) Three segments; (c), (d) four segments; (e), (f) five segments.
-
[1] Matthew B, Wolf K T, Zhanna N 2018 Biomech. Model. Mechanobiol. 17 1343
Google Scholar
[2] Grimaldi A, Moriondo A, Sciacca L, Guidali M L, Tettamanti G, Negrini D 2006 Am. J. Physiol. Heart. Circ. Physiol. 291 876
Google Scholar
[3] Schmid-Schonbein G W 1990 Physiol. Rev. 70 987
[4] Gashev A A 2002 Ann. N. Y. Acad. Sci. 979 178
[5] Gasheva O Y, Zawieja D C, Gashev A A 2006 J. Physiol. 575 821
Google Scholar
[6] Zawieja, David C 2009 Lymphat. Res. Biol. 7 87
Google Scholar
[7] EisenhofferJ, Kagal A, Klein T, Johnston M 1995 Microvasc. Res. 49 97
Google Scholar
[8] 秦立鹏, 牛春雨, 赵自刚 2011 生理科学进展 42 237
Qin L P, Niu C Y, Zhao Z G 2011 Advances in Physiological Sciences. 42 237
[9] Margaris K N, Black R A 2012 J. R. Soc. Interface. 9 601
Google Scholar
[10] Bertram C D, Macaskill C, Moore J E 2011 J. Biomech. Eng. 133 11008
Google Scholar
[11] Bertram C D, Macaskill C, Jr J E M 2014 Comput. Methods. Biomech. Biomed. Eng. 17 1519
Google Scholar
[12] 朱炼华, 郭照立 2015 计算物理 32 20
Google Scholar
Zhu L H, Guo Z L 2015 Chin. J. Comput. Phys. 32 20
Google Scholar
[13] 李华兵 2004 博士学位论文 (上海: 复旦大学)
Li H B 2004 Ph. D. Dissertation (Shanghai: Fudan University) (in Chinese)
[14] Shan X, Chen H 1993 Phys. Rev. E 47 1815
[15] Mcnamara G R, Zanetti G 1988 Phys. Rev. Lett. 61 2332
Google Scholar
[16] Chen H, Chen S, Matthaeus W H 1992 Phys. Rev. A. 45 5339
Google Scholar
[17] Qian Y. H, D'Humières D, Lallemand P 1992 Euro. Phys. Lett. 17 479
Google Scholar
[18] Ladd A J C, Verberg R 2001 J. Stat. Phys. 104 1191
Google Scholar
[19] Baish J W, Kunert C, Padera T P, Munn L L 2016 PloS Comput. Biol. 12 e1005231
Google Scholar
[20] Bazigou E, Wilson J T 2014 Microvasc. Res. 96 38
Google Scholar
[21] Damarla M, Zaeh S, Niedermeyer S 2020 Am. J. Respir. Crit. Care. Med. 202 4
Google Scholar
[22] Li H B, Mei Y M, Maimon N 2019 Sci. Rep. 9 2014
Google Scholar
下载:
计量
- 文章访问数: 4270
- PDF下载量: 44
- 被引次数: 0
