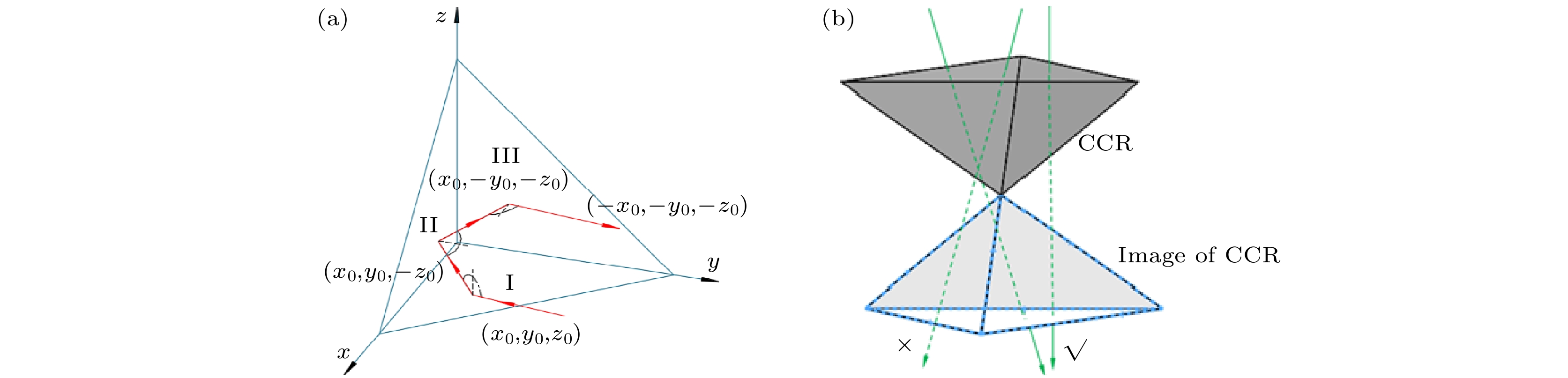
-
角锥棱镜是激光反射器的主要光学组件, 在合作目标卫星激光测距中发挥着关键作用. 精确计算角锥棱镜的远场衍射图样是估算激光测距回波能量的必要过程. 本文基于角锥棱镜的反射原理, 分析了不同激光入射方向对反射器有效反射面积的影响, 提出了一种适用范围更广的有效反射面积计算方法, 同时分析了激光入射方向对角锥棱镜光学反射率的影响. 在此基础上, 应用光学标量衍射理论建立了远场衍射图样算法, 分别对多种激光入射方向的镀金属(银)膜和无镀膜角锥棱镜进行了远场衍射图样仿真计算, 得到了两类角锥棱镜的远场衍射图样分布随入射方向变化的规律. 搭建了角锥棱镜远场衍射图样测试系统, 通过实测结果与仿真计算的对比分析, 验证了仿真计算的准确性.
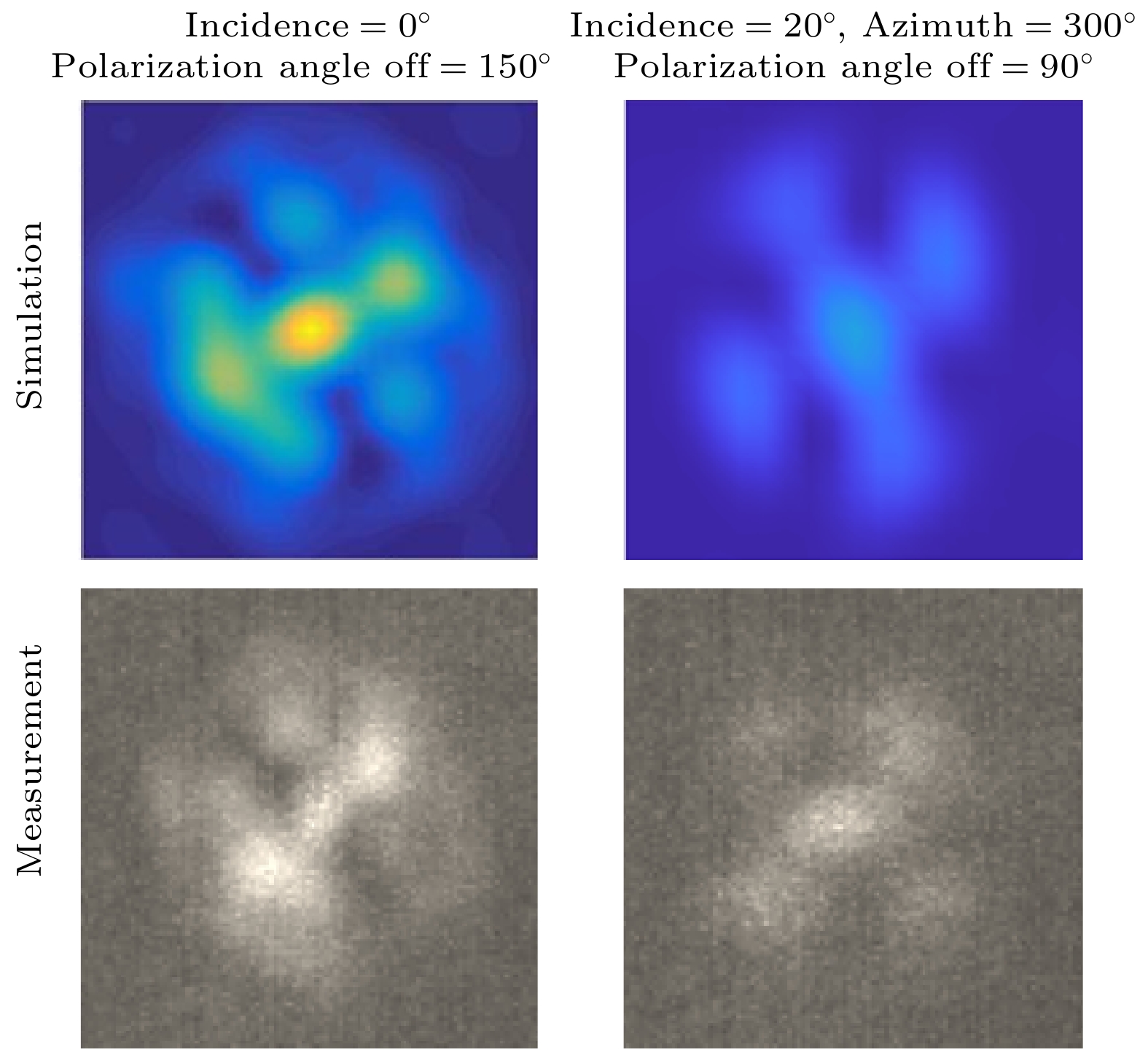
Corner cube retroreflector (CCR) as a main optical component of laser ranging retroreflector array plays a key role in satellite laser ranging (SLR) to cooperative targets. To accurately estimate the echo energy of SLR, it is necessary to precompute photons’ distribution in the distance of SLR station by calculating the Far field diffraction pattern (FFDP) of CCR under various conditions. In this paper, the analysis of the effective reflection area and optical reflectivity of CCR for arbitrary incidence angle are carried out, in which a method to calculate the CCR effective reflection area with a wider applicability is used, and the difference between optical reflectivity of metal-coated CCR and uncoated (total internal reflection) CCR is also discussed. On this basis, combined with optical diffraction theory, a simulation program for CCR FFDP calculation is established, thereby producing FFDPs of CCR for a variety of incidence angles under different coating conditions. The results show that the FFDP of metal-coated CCR is almost unrelated to the azimuth angle of incident light or polarization, but is determined only by elevation angle of incident light. The pattern is always like Airy spot or its tensile deformation. Relatively, uncoated CCR’s FFDP has a more complex figure, its reflected energy is divided into several lobes whose size, number and position are all influenced by elevation and azimuth angle of incidence, and also by the polarization. Generally, the incidence direction which has a large total intensity of far optical field is to an extent the same as that of large effective reflection area and optical reflectivity. Furthermore, simulation results with uncoated CCR presents a higher relevance of incidence direction and FFDP. To verify the reliability and correctness of these simulation results, a diffraction optical experimental system at a wavelength of 1550 nm is set up to conduct laboratory confirmation, including laser, camera, beam expander, rotating platform and other essential optics. A silver-coated CCR and an uncoated CCR (both made of fuse silica, each has different dihedral angle offset) are chosen to measure their FFDPs with random polarization directions at several random incidence angles. All the measurement results are in good agreement with the simulations of FFDPs. -
Keywords:
- corner cube retro-reflector /
- effective reflection area /
- far field diffraction pattern /
- simulation calculation
[1] 罗青山, 郭唐永, 邹彤, 朱威, 姚运生 2017 红外与激光工程 46 114
Google Scholar
Luo Q S, Guo T Y, Zou T, Zhu W, Yao Y S 2017 Infrared Laser Eng. 46 114
Google Scholar
[2] Method of Calculating Retroreflector-Array Transfer Functions, Arnold D A http://articles.adsabs.harvard.edu/pdf/1979 SAOSR.382.....A [2021-1-11]
[3] Sadovnikov M A, Sokolov A L 2009 Opt. Spectrosc. 107 201
Google Scholar
[4] 声远 1975 激光与红外 5 645
Google Scholar
Sheng Y 1975 Laser Infrared 5 645
Google Scholar
[5] 周辉, 李松, 石岩, 翁兴涛, 王嘉平 2004 红外与激光工程 33 418
Google Scholar
Zhou H, Li S, Shi Y, Weng X T, Wang J P 2004 Infrared Laser Eng. 33 418
Google Scholar
[6] 周辉, 李松, 郑国兴, 高俊玲 2009 光学学报 29 60
Google Scholar
Zhou H, Li S Zheng G X, Gao G L 2009 Acta Opt. Sin. 29 60
Google Scholar
[7] 万强, 郭延龙, 王小兵, 孙斌, 卢常勇, 韦尚方 2005 激光与光电子学进展 45 20
Google Scholar
Wan Q, Guo Y L, Wang X B, Sun B, Lu C Y, Wei S F 2005 Laser Optoelectron. Prog. 45 20
Google Scholar
[8] Wilkinson M, Appleby G 2011 Adv. Space Res. 48 578
Google Scholar
[9] Chang R F, Currie D G, Alley C O, Pittman, M E 1971 JOSA 61 431
Google Scholar
[10] Retroreflector Array Transfer Functions, Arnold D A https://ilrs.gsfc.nasa.gov/docs/retro_transfer_functions.pdf [2021-1-11]
[11] Otsubo T, Kunimori H, Noda H, Hanada, H 2010 Adv. Space Res. 45 733
Google Scholar
[12] 声远 1973 激光与红外 3 1
Google Scholar
Sheng Y 1973 Laser Infrared 3 1
Google Scholar
[13] 钟声远, 徐广平, 吴键 2009 激光与红外 39 128
Google Scholar
Zhong S Y, Xu G P, Wu J 2009 Laser Infrared 39 128
Google Scholar
[14] 聂辉, 翁兴涛, 李松, 刘基余 2003 光学学报 23 1470
Google Scholar
Nie H, Weng X T, Li S, Liu J Y 2003 Acta Opt. Sin. 23 1470
Google Scholar
[15] Eckhardt, H D 1971 Appl. opt. 10 1559
Google Scholar
[16] 焦仲科, 岳永坚 2014 半导体光电 35 811
Google Scholar
Jiao Z K, Yue Y J 2014 Semicond. Optoelectron. 35 811
Google Scholar
[17] 李松, 刘博 2002 测绘信息与工程 27 25
Google Scholar
Li S, Liu B 2002 J. Geomatics 27 25
Google Scholar
[18] Born M, Wolf E 1970 Principles of Optics (4th Ed.) (Oxford: Pergamon Press) pp38−41
[19] 汤凯, 程志恩, 张海峰, 李朴, 张忠萍 2016 光学精密工程 24 353
Google Scholar
Tang K, Cheng Z E, Li P, Zhang Z P 2016 Opt. Precis. Eng. 24 353
Google Scholar
[20] Turyshev S G, Williams J G, Folkner W M, Gu tt, G M. Baran, R T, He in, R C, Somawardhana R P, Lipa J A, Wang S W 2013 Exp. Astron. 36 105
Google Scholar
[21] Preston A, Merkowitz S 2013 Appl. Opt. 52 8676
Google Scholar
[22] He Y, Liu Q, Duan H Z, He J J, Jiang, Y Z, Y eh, H C 2018 Res. Astron. Astrophys. 18 136
Google Scholar
[23] 徐怀方 1985 中国激光 13 233
Google Scholar
Xu H F 1985 Chin. J. Las. 13 233
Google Scholar
-
图 1 (a)光线在经过CCR后向反射后的方向矢量变化; (b)光线在CCR中完成后向反射的条件
Fig. 1. (a) Change of ray’s direction vector retro-reflected by CCR; (b) condition of rays reflected backwards by CCR.
图 3 角锥棱镜的镜像和投影孔径的重叠决定了有效反射面积
Fig. 3. Active reflecting area determined by the overlap of image and projection of CCR front face.
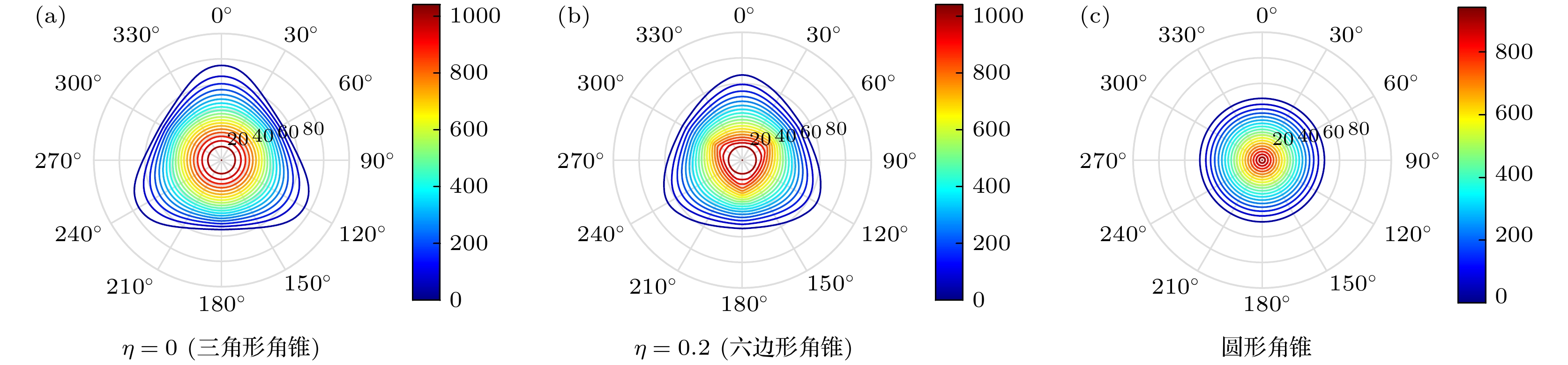
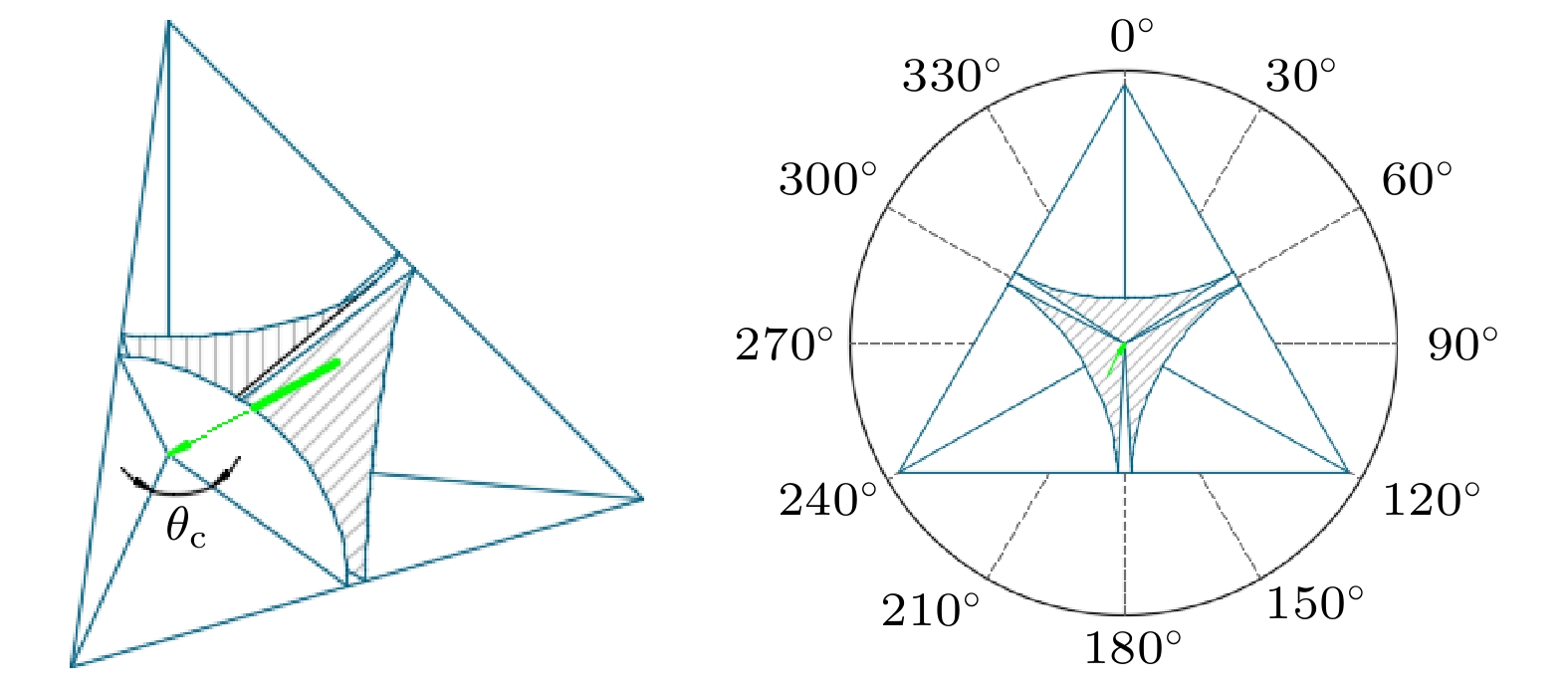
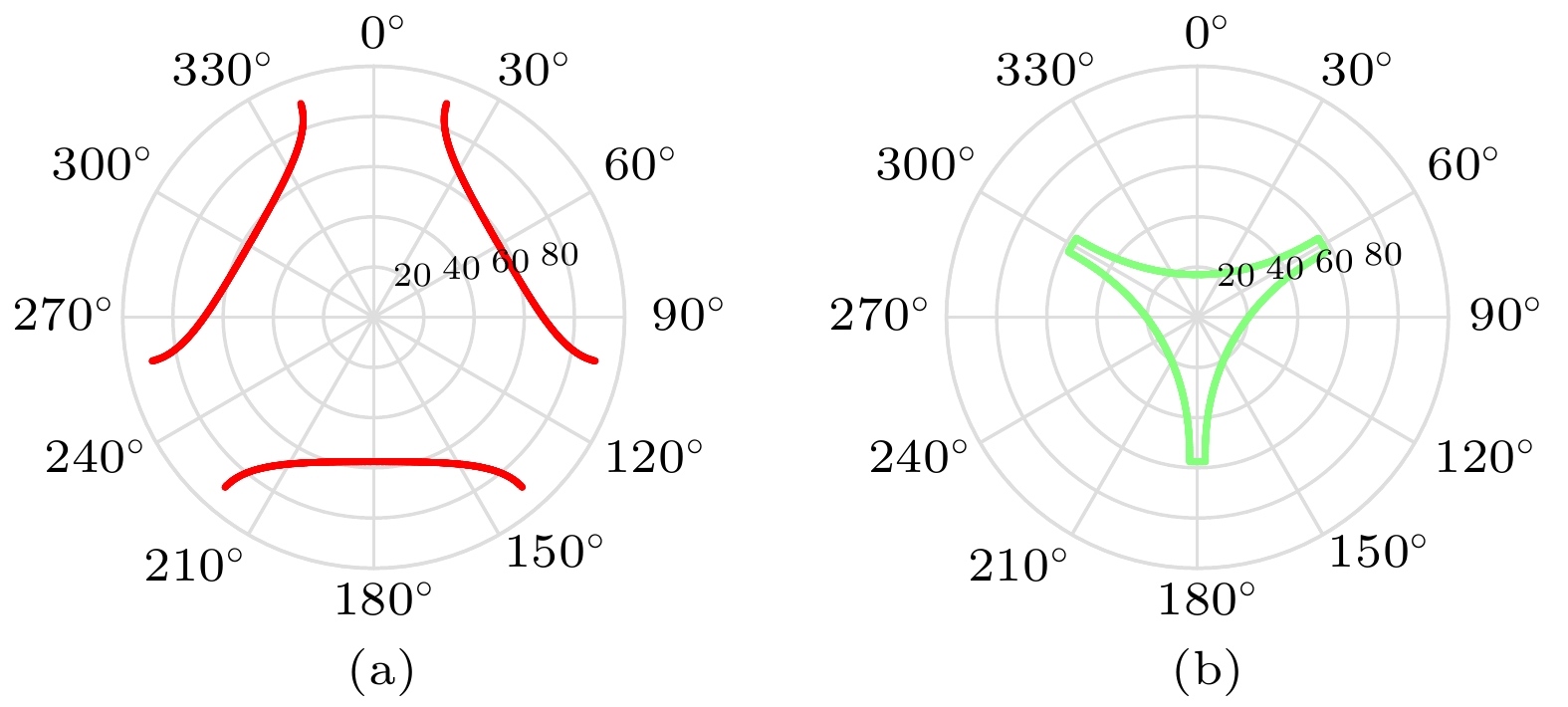
图 5 不同形状角锥棱镜有效反射面积(mm2)随入射角(θ, 0º−90º)和方位角(φ, 0º−360º)的分布
Fig. 5. CCR active reflecting area in dependence of incidence and azimuth angle for different cutting mode. Polar axis refers to incident angle (θ, 0º−90º) in degree, the other axis refers to azimuth angle (φ, 0º−360º) in degree, the color bar refers to the active reflecting area in square millimeter
图 6 金属膜角锥棱镜满足高效率后向反射的折射光线矢量
Fig. 6. High efficiency backward reflection ray vector in metal-coated CCR.
图 7 无镀膜角锥棱镜满足高效率后向反射的折射光线矢量
Fig. 7. High efficiency backward reflection ray vector in uncoated CCR.
图 8 (a)镀金属膜与(b)无镀膜角锥棱镜(n ≈ 1.44@1550 nm)的高反射率入射方向范围
Fig. 8. High optical efficiency incidence direction range of (a) metal-coated and (b) uncoated CCR (n ≈ 1.44@1550 nm)
图 9 镀银膜角锥棱镜不同入射角的FFDP (图样范围约
$ 16'' \times 16'' $ )Fig. 9. FFDP of Ag-coated CCR in various incidence direction.
图 12 不同入射角镀银膜角锥的FFDP仿真与实测结果对比(图样范围约60′′ × 60′′)
Fig. 12. Comparison of FFDP simulation and experimental results of metal coated CCR with different incident angles (The drawing range is about 60′′ × 60′′).
图 13 不同入射角无镀膜角锥的FFDP仿真与实测结果对比(图样范围约60′′ × 60′′)
Fig. 13. Comparison of FFDP simulation and experimental results of uncoated CCR with different incident angles (the drawing range is about 60′′ × 60′′).
-
[1] 罗青山, 郭唐永, 邹彤, 朱威, 姚运生 2017 红外与激光工程 46 114
Google Scholar
Luo Q S, Guo T Y, Zou T, Zhu W, Yao Y S 2017 Infrared Laser Eng. 46 114
Google Scholar
[2] Method of Calculating Retroreflector-Array Transfer Functions, Arnold D A http://articles.adsabs.harvard.edu/pdf/1979 SAOSR.382.....A [2021-1-11]
[3] Sadovnikov M A, Sokolov A L 2009 Opt. Spectrosc. 107 201
Google Scholar
[4] 声远 1975 激光与红外 5 645
Google Scholar
Sheng Y 1975 Laser Infrared 5 645
Google Scholar
[5] 周辉, 李松, 石岩, 翁兴涛, 王嘉平 2004 红外与激光工程 33 418
Google Scholar
Zhou H, Li S, Shi Y, Weng X T, Wang J P 2004 Infrared Laser Eng. 33 418
Google Scholar
[6] 周辉, 李松, 郑国兴, 高俊玲 2009 光学学报 29 60
Google Scholar
Zhou H, Li S Zheng G X, Gao G L 2009 Acta Opt. Sin. 29 60
Google Scholar
[7] 万强, 郭延龙, 王小兵, 孙斌, 卢常勇, 韦尚方 2005 激光与光电子学进展 45 20
Google Scholar
Wan Q, Guo Y L, Wang X B, Sun B, Lu C Y, Wei S F 2005 Laser Optoelectron. Prog. 45 20
Google Scholar
[8] Wilkinson M, Appleby G 2011 Adv. Space Res. 48 578
Google Scholar
[9] Chang R F, Currie D G, Alley C O, Pittman, M E 1971 JOSA 61 431
Google Scholar
[10] Retroreflector Array Transfer Functions, Arnold D A https://ilrs.gsfc.nasa.gov/docs/retro_transfer_functions.pdf [2021-1-11]
[11] Otsubo T, Kunimori H, Noda H, Hanada, H 2010 Adv. Space Res. 45 733
Google Scholar
[12] 声远 1973 激光与红外 3 1
Google Scholar
Sheng Y 1973 Laser Infrared 3 1
Google Scholar
[13] 钟声远, 徐广平, 吴键 2009 激光与红外 39 128
Google Scholar
Zhong S Y, Xu G P, Wu J 2009 Laser Infrared 39 128
Google Scholar
[14] 聂辉, 翁兴涛, 李松, 刘基余 2003 光学学报 23 1470
Google Scholar
Nie H, Weng X T, Li S, Liu J Y 2003 Acta Opt. Sin. 23 1470
Google Scholar
[15] Eckhardt, H D 1971 Appl. opt. 10 1559
Google Scholar
[16] 焦仲科, 岳永坚 2014 半导体光电 35 811
Google Scholar
Jiao Z K, Yue Y J 2014 Semicond. Optoelectron. 35 811
Google Scholar
[17] 李松, 刘博 2002 测绘信息与工程 27 25
Google Scholar
Li S, Liu B 2002 J. Geomatics 27 25
Google Scholar
[18] Born M, Wolf E 1970 Principles of Optics (4th Ed.) (Oxford: Pergamon Press) pp38−41
[19] 汤凯, 程志恩, 张海峰, 李朴, 张忠萍 2016 光学精密工程 24 353
Google Scholar
Tang K, Cheng Z E, Li P, Zhang Z P 2016 Opt. Precis. Eng. 24 353
Google Scholar
[20] Turyshev S G, Williams J G, Folkner W M, Gu tt, G M. Baran, R T, He in, R C, Somawardhana R P, Lipa J A, Wang S W 2013 Exp. Astron. 36 105
Google Scholar
[21] Preston A, Merkowitz S 2013 Appl. Opt. 52 8676
Google Scholar
[22] He Y, Liu Q, Duan H Z, He J J, Jiang, Y Z, Y eh, H C 2018 Res. Astron. Astrophys. 18 136
Google Scholar
[23] 徐怀方 1985 中国激光 13 233
Google Scholar
Xu H F 1985 Chin. J. Las. 13 233
Google Scholar
下载:
计量
- 文章访问数: 6285
- PDF下载量: 140
- 被引次数: 0
