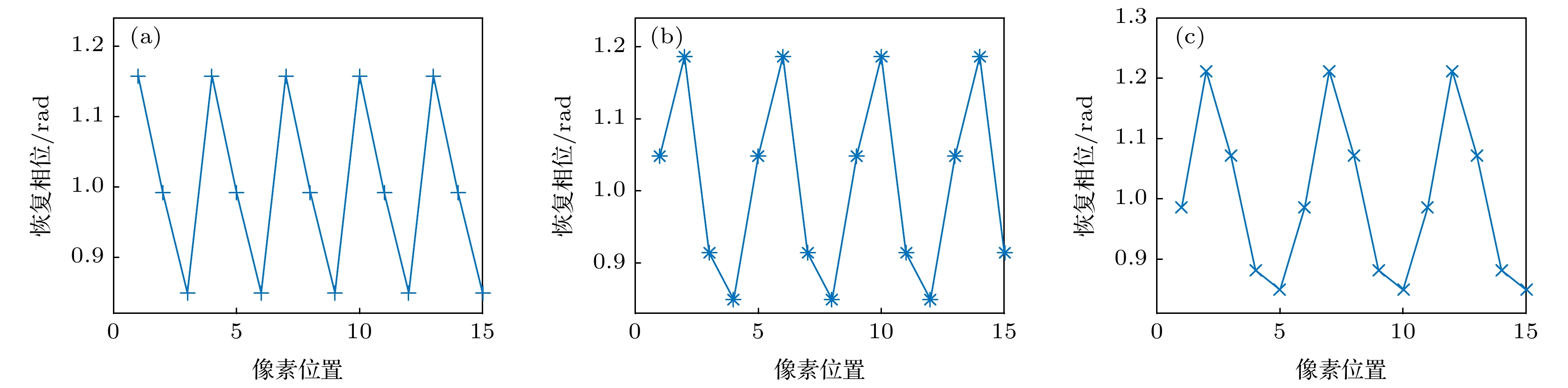
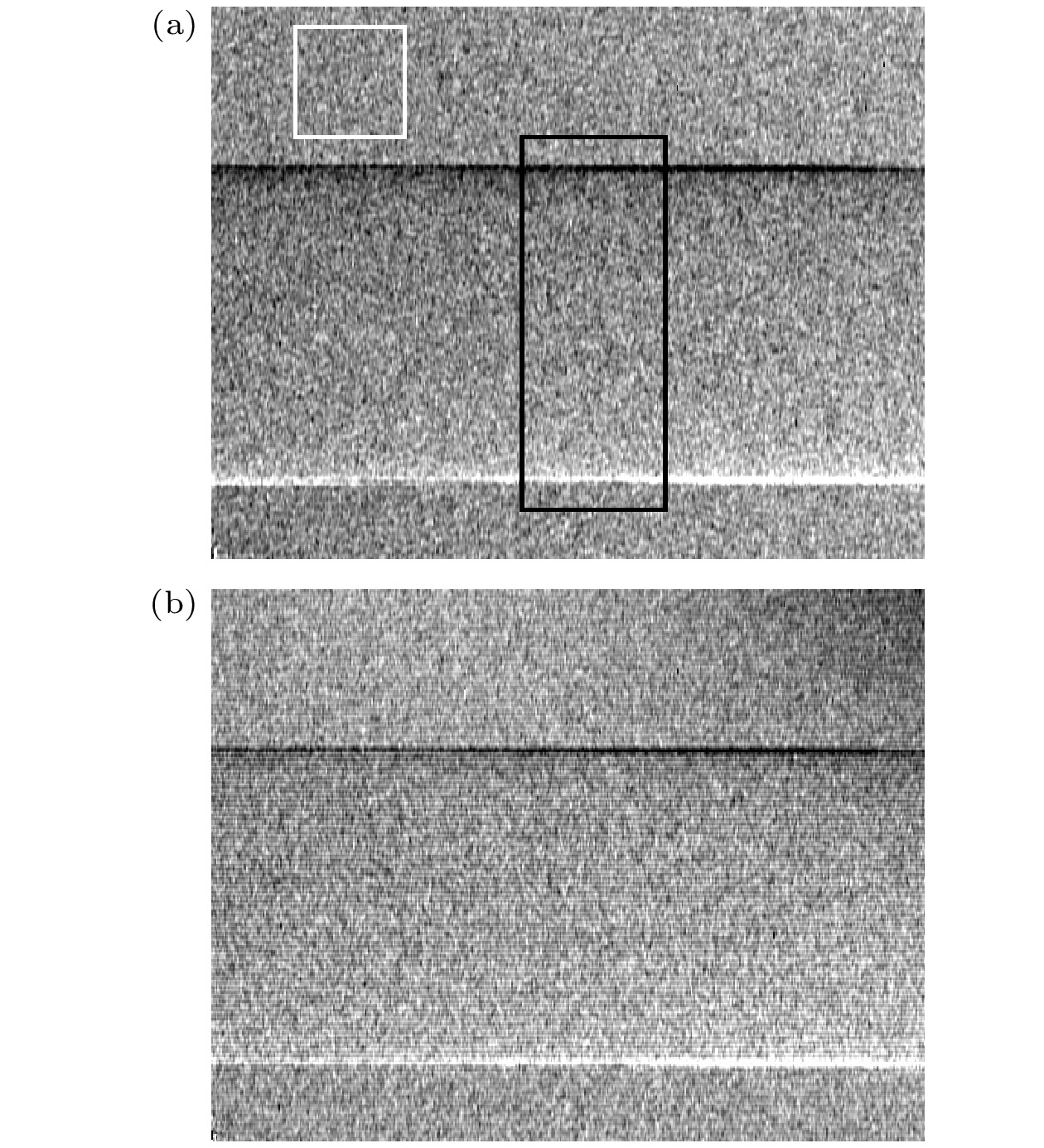
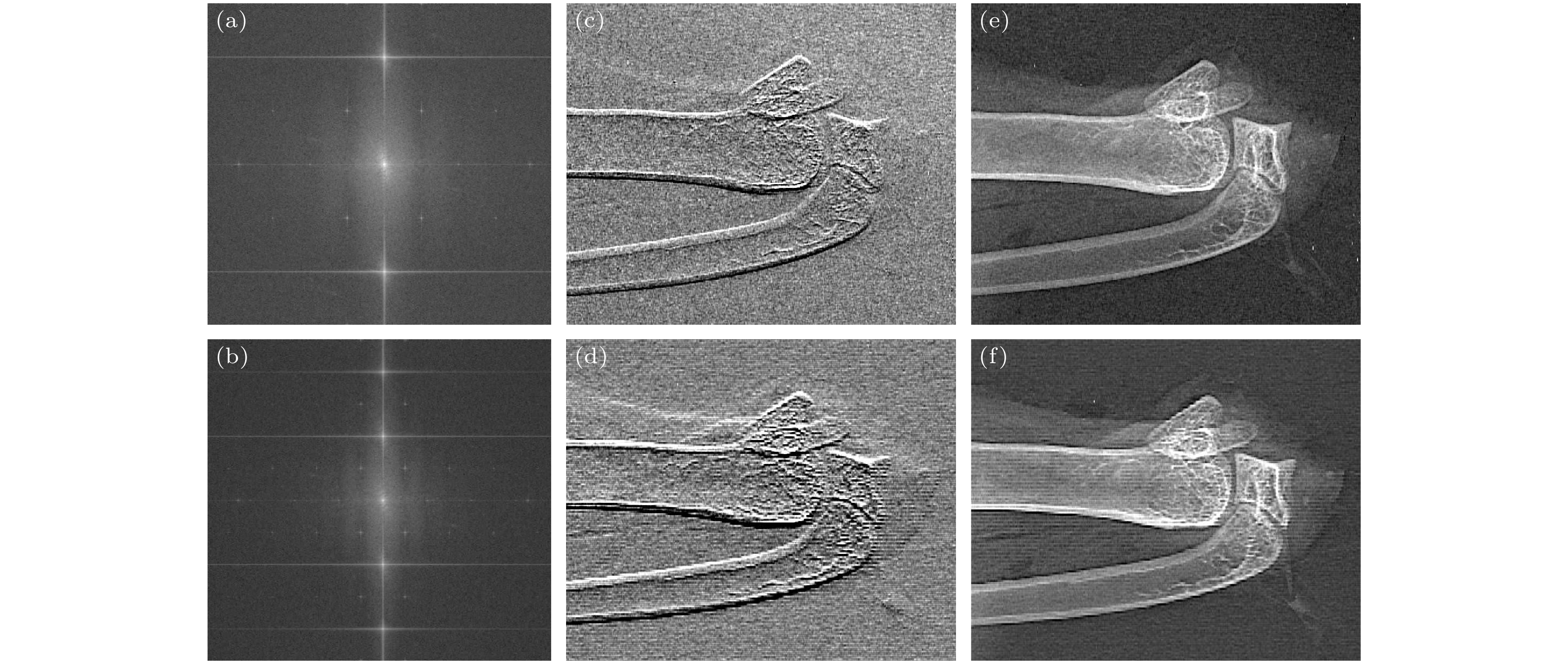
Over the last two decades, the grating-based phase-contrast imaging has aroused the interest of a number of researchers. It could provide an access to three complementary signals simultaneously: the conventional absorption contrast, the differential phase contrast related to refraction of incident wave, and the dark-field contrast that relates to ultra small angle scattering in a sample. The grating-based phase-contrast signals have higher contrast sensitivity for some types of soft samples than the absorption signals. Dark-field signals have better diagnostic effects in the detection of lung tumors, pneumothorax and the identification of microcalcifications in breast. There are two main phase retrieval methods in grating-based X-ray phase-contrast imaging, i.e. phase stepping method and Fourier transform method. The phase signals retrieved by phase stepping is high precise and has low noise. But the sample suffers high dose due to at least three exposures. The phase signals retrieved by Fourier transform is low-dose due to the fact that only one image with sample is needed, but it is easily affected by artifacts when the size of the filtering window is too large. However, when the size of the filtering window is too small, the high-frequency information of the phase-contrast image will be lost, and the image will become blurred. A trade-off between definitions of the image and artifacts should be made. Since the phase-contrast signal and the dark-field signal of the sample are modulated by carrier fringes, the frequency spectrum of the detected image consists of many different harmonics. The artifacts in the retrieved signals originate from the spectrum aliasing between primary peak around zero spatial frequency and first-order harmonic peaks. Therefore, the subtraction between two images with phase difference can remove the primary peak, and the artifacts in the phase-contrast signals and dark-field signals will be suppressed. In order to further suppress the artifacts, we increase the frequency of carrier fringes, which results in a larger distance between first-order harmonic peaks in frequency domain. We finally attain artifact-free phase-contrast images and dark-field images while maintaining high definition of the images. The method proposed here is not only applicable to incoherent imaging system, but also to Talbot-Lau interferometer, and it would be useful in fast and low-dose X-ray phase-contrast and dark-field imaging.