-
心血管疾病尤其是高血压已成为人类生命健康最大杀手之一. 本文探究主动脉瓣心音信号与血压之间的关系, 提出一种基于心音信号的无创血压估计方法. 首先, 根据血压与心音信号的关系, 提取第一心音和第二心音峰值点的时间间隔以及第二心音的峭度作为特征; 接着将第一心音和第二心音峰值点的时间间隔、第二心音的峭度与所测收缩压和舒张压进行线性拟合; 最后根据第一心音和第二心音峰值点的时间间隔、第二心音的峭度与血压的线性关系, 提出基于心音的血压评估公式. 实验结果表明, 第一心音和第二心音峰值点的时间间隔、第二心音的峭度能够作为血压评估的特征参数, 与血压具有良好的线性关系, 其拟合优度分别为0.801和0.765, 通过本文推导出的血压计算公式所得血压与商用电子血压计测量值的平均误差小于5 mmHg, 标准偏差小于8 mmHg. 本文提出基于心音对血压进行评估的一种新方法, 可用于血压的连续测量, 针对某些特殊条件下, 具有显著的应用前景.Cardiovascular disease, especially hypertension, has become one of the biggest killers of human life and health. Therefore, early detection and prevention of cardiovascular diseases are of great significance for people’s health. In this paper, we explore the relationship between aortic valve heart sound signal and blood pressure, and propose a method of non-invasively estimating blood pressure based on heart sound signals. First, according to the relationship between blood pressure and the heart sound signal, both the time interval between the peak point of the first heart sound and that of the second heart sound and the kurtosis of the second heart sound are extracted as features. Then the time interval between the first heart sound and the second heart sound, and the kurtosis of the second heart sound are linearly fitted to the measured blood pressure. Finally, according to the linear relationship between heart sound and blood pressure, a blood pressure evaluation formula based on the heart sound is established. The experimental results show that the time interval between the peak point of the first heart sound and that of the second heart sound, and the kurtosis of the second heart sound can be used as the characteristic parameters of blood pressure evaluation, which have a good linear relationship with blood pressure. The goodness of fit is 0.801 and 0.765, separately. The average error between the blood pressure calculated from the blood pressure calculation formula and the blood pressure measured by a commercial electronic sphygmomanometer is less than 5 mmHg, and the standard deviation is less than 8 mmHg. For hypertensive patients, the time interval between the peak point of the first heart sound and the second heart sound is shortened, and the kurtosis of the second heart sound is increased, which is a typical feature of heart sounds in patients with hypertension. Compared with the traditional blood pressure calculation method, the blood pressure assessment method proposed in this paper only needs to collect heart sound signals to effectively assess the blood pressure. The method is convenient to operate and can be used for continuously monitoring the blood pressure, and is especially suitable for monitoring the blood pressures of infants, disabled patients with limbs, and disabilities in certain medical environments.
-
Keywords:
- blood pressure /
- aortic valve heart sound /
- peak detection /
- kurtosis
[1] WHO NCD Mortality and Morbidity http://www.who.int/gho/ncd/mortality_morbidity/en/ [2015−09−07]
[2] Durand L G, Pibarot P 1995 Crit. Rev. Biomed. Eng. 23 163
Google Scholar
[3] Liu C C, Springer D B, Clifford G D 2017 Physiol. Meas. 38 1730
Google Scholar
[4] Bartels A, Harder D 1992 Clin. Phys. Physiol. Meas. 13 249
Google Scholar
[5] Tanigawa N, Smith D, Craige E 1991 Jpn. Circ. J. 55 737
Google Scholar
[6] Zhang X Y, Zhang Y T 2006 Proceedings of the IEEE Engineering in Medicine and Biology Society New York, USA, August 30−September 3, 2006 p2888
[7] Peng R C, Yan W R, Zhang N L, Lin W H, Zhou X L, Zhang Y T 2015 Sensors-basel 15 23653
Google Scholar
[8] Bombardini T, Gemignani V, Bianchini E, Venneri L, Petersen C 2008 Cardiovasc. Ultrasoun. 6 41
Google Scholar
[9] Tao Y W CN104510491A [2015−04−15]
[10] 黄正钦 2014 硕士学位论文 (昆明: 云南大学)
Huang Z Q 2014 M. S. Thesis (Kunming: Yunnan University) (in Chinese)
[11] Shriram R, Wakankar A, Daimiwal N 2010 Proceedings of International Conference on Bioinformatics and Biomedical Technology Chengdu, China, April 16−18, 2010 p100971242
[12] Landowne M 1957 Circ. Res. 5 594
Google Scholar
[13] Payne R A, Symeonides C N, Webb D J, Maxwell S R J 2006 J. Appl. Physiol. 100 136
Google Scholar
[14] 蔡任圃 2012 科学月刊 507 236
Cai R P 2012 Science Monthly 507 236
[15] 周静, 杨永明, 何为 2005 中国生物医学工程学报 24 685
Google Scholar
Zhou J, Yang Y M, He W 2005 Chin. J. Biomed. Eng. 24 685
Google Scholar
[16] Ding X R, Zhao R, Yang G Z, Pettigrew R 2016 IEEE J. Biomed. Health 20 1
Google Scholar
[17] Lim K H, Shin Y D, Park S H, Bae J H, Lee H J, Kim S J 2013 Pak. J. Med. Sci. 29 1023
-
图 1 心音信号采集位置和采集装置示意图 (a) 心脏听诊区位置; (b) Ω型肩戴式无线心音采集装置
Fig. 1. Heart sound signal acquisition position and acquisition device: (a) Heart auscultation area location; (b) shoulder-mounted wireless heart sound acquisition device of Ω shape.
图 2 第一心音和第二心音标定效果示意图 (a) 包络图; (b) 峰值定位图
Fig. 2. Calibration effect diagram of the first heart sound and the second heart sound: (a) Envelope; (b) peak location.
图 3 心音信号、脉搏波信号和心电信号时程关系图 (a)心音信号; (b) 脉搏波信号; (c) 心电信号
Fig. 3. Heart sound signal, pulse wave signal, and ECG signal time diagram: (a) Heart sound signal; (b) pulse wave signal; (c) ECG signal.
图 4 测试者HSTT(均值)与血压(均值)拟合结果 (a) 静止状态; (b) 运动状态; (c) 运动后恢复状态
Fig. 4. Fitting results of HSTT (mean) and blood pressure (mean): (a) Stationary state; (b) exercise state; (c) recovery state after exercise.
图 5 测试者K (均值)与血压(均值)拟合结果 (a) 静止状态; (b) 运动状态; (c) 运动后恢复状态
Fig. 5. Fitting results of K (mean) and blood pressure (mean): (a) Stationary state; (b) exercise state; (c) recovery state after exercise
图 6 第5组测试者血压实测值(均值)和评估值(均值)对比图 (a) 静止状态; (b) 运动状态; (c) 运动后恢复状态
Fig. 6. Comparison of measured blood pressure (mean) and evaluation (mean) of blood pressure in the test subjects of the 5th group: (a) Stationary state; (b) exercise state; (c) recovery state after exercise
图 7 35名测试者血压实测值(均值)和评估值(均值)对比 (a) CC; (b) MAE; (c) ME; (d) SD
Fig. 7. Comparison of measured blood pressure (mean) and evaluation values (mean) of 35 subjects: (a) Pearson correlation coefficient CC; (b) mean absolute error MAE; (c) mean error ME; (d) standard deviation SD.
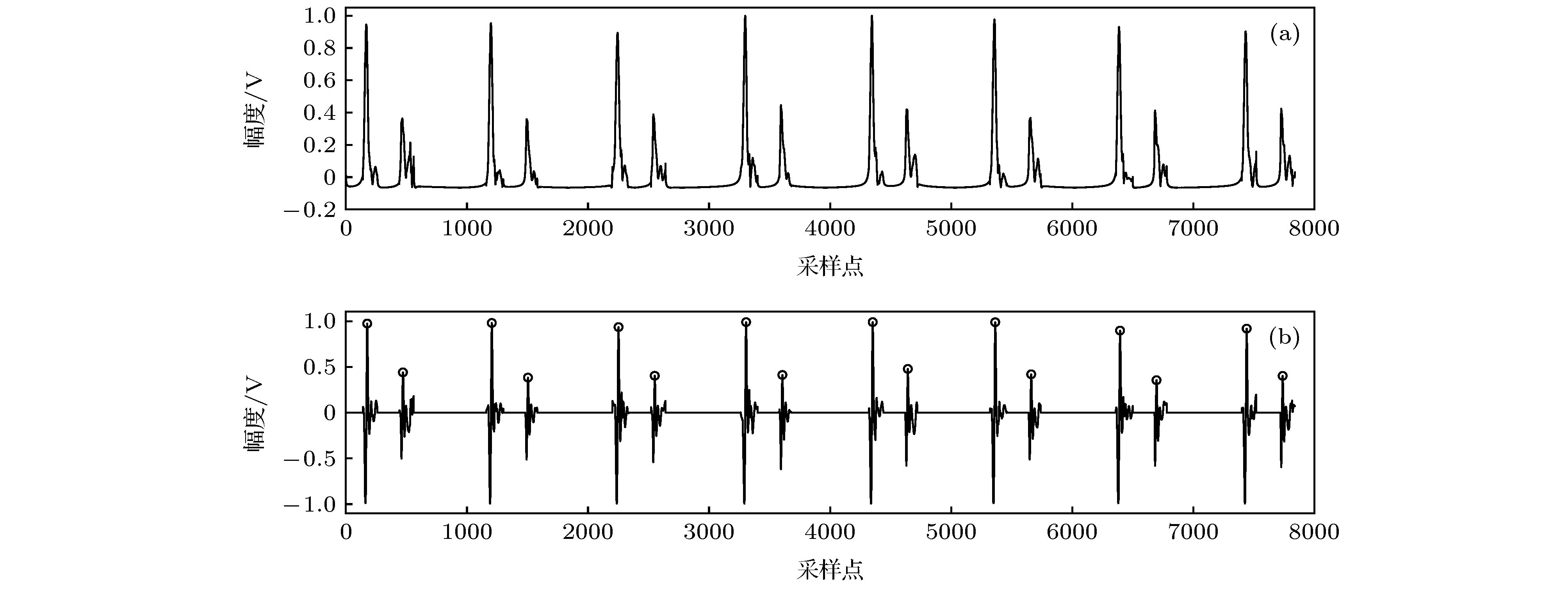
表 1 编号5-2测试者第二心音峰值点与脉搏波峰值点在时间上的对应关系
Table 1. Correspondence between the peak point of the second heart sound and pulse wave of No. 5-2 subject.
信号类型 时间/ms 第二心音
峰值点205 976 1722 2481 3233 3956 4697 5446 6184 脉搏波
峰值点209 982 1727 2486 3238 3960 4704 5454 6189 
下载: 导出CSV
表 2 HSTT与血压拟合优度
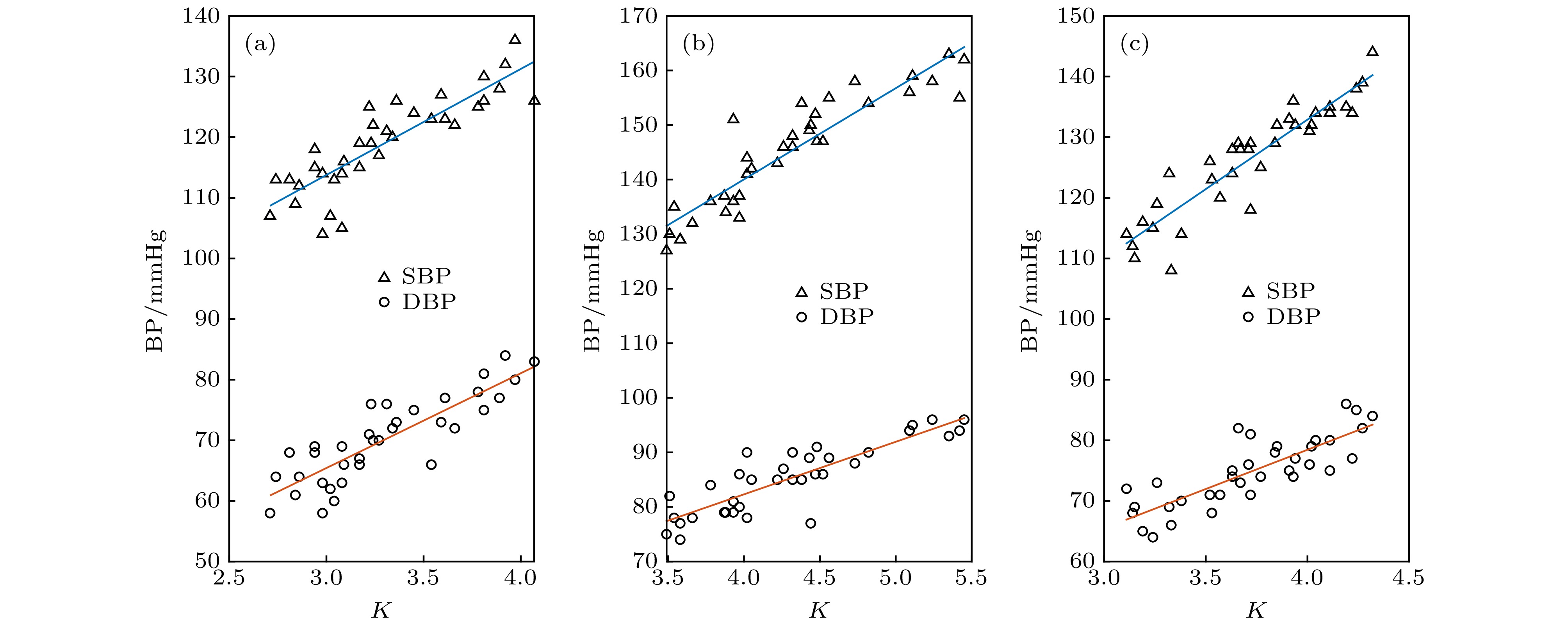
Table 2. Goodness of fit between HSTT and BP.
状态 SBP DBP 均值 静止 0.847 0.774 0.801 运动 0.826 0.753 运动后恢复 0.839 0.768
下载: 导出CSV
表 3 第二心音峭度(K )与血压拟合优度
Table 3. The second heart sound kurtosis (K ) and goodness of fit of blood pressure.
状态 SBP DBP 均值 静止 0.824 0.703 0.765 运动 0.818 0.724 运动后恢复 0.806 0.718
下载: 导出CSV
表 4 第5组测试者3种状态下心音与血压相关数据统计结果(均值)
Table 4. Data related to heart sounds and blood pressure in the 5th group.
测试者状态 HSTT/ms K 计算${\rm{SBP}}$/mmHg 实测${\rm{SBP}}$/mmHg 计算${\rm{DBP}}$/mmHg 实测${\rm{DBP}}$/mmHg 静止 277.9 3.31 120.28 122.6 71.86 71.2 运动 246.7 4.19 139.00 140.2 84.34 83.0 运动后恢复 264.3 3.72 128.43 128.8 77.29 75.8
下载: 导出CSV
表 5 所有测试者数据参数统计结果
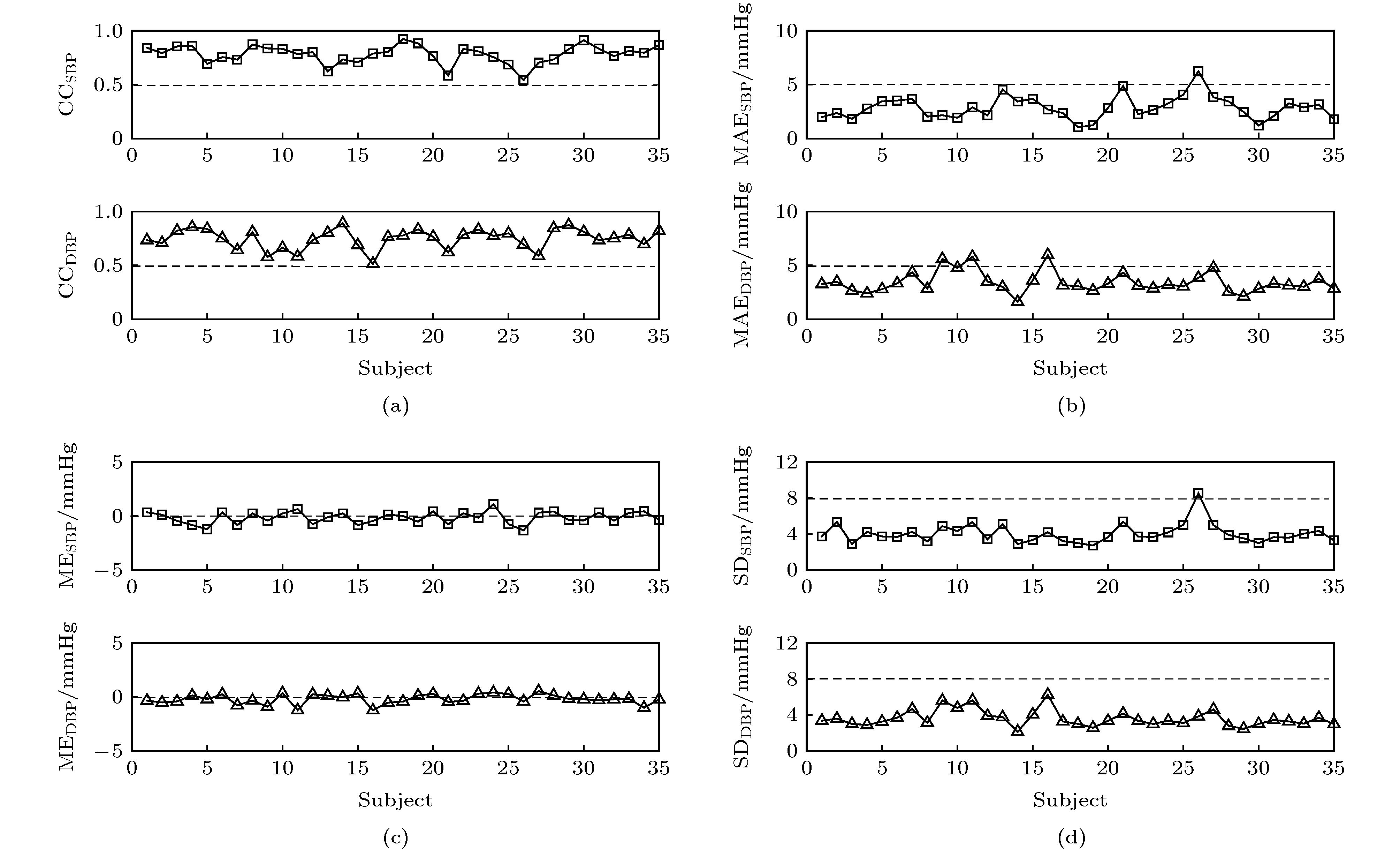
Table 5. Data parameter statistics of all sujects.
参数 最大值 最小值 平均值 ${{\rm{CC}}_{\rm{SBP}}}$ 0.923 0.542 0.781 ${{\rm{CC}}_{\rm{DBP}}}$ 0.891 0.515 0.748 ${{\rm{MAE}}_{\rm{SBP}}}$/mmHg 6.245 1.054 2.712 ${{\rm{MAE}}_{\rm{DBP}}}$/mmHg 5.968 1.628 3.264 ${{\rm{ME}}_{\rm{SBP}}}$/mmHg 1.091 –1.331 –0.401 ${{\rm{ME}}_{\rm{DBP}}}$/mmHg 0.526 –1.254 –0.812 ${{\rm{SD}}_{\rm{SBP}}}$/mmHg 8.534 2.723 3.584 ${{\rm{SD}}_{\rm{DBP}}}$/mmHg 6.234 2.132 4.053
下载: 导出CSV
表 6 15名测试者血压和心音相关数据统计结果
Table 6. Datas related to heart sounds and blood pressure of 15 testers.
测试者状态 ${\rm{HSTT}}$/ms K 计算SBP/mmHg 实测SBP/mmHg 计算DBP/mmHg 实测DBP/mmHg 健康 279.4 3.38 122.46 121.63 73.16 72.02 高血压患者 267.8 4.52 151.94 148.58 84.34 81.17
下载: 导出CSV
-
[1] WHO NCD Mortality and Morbidity http://www.who.int/gho/ncd/mortality_morbidity/en/ [2015−09−07]
[2] Durand L G, Pibarot P 1995 Crit. Rev. Biomed. Eng. 23 163
Google Scholar
[3] Liu C C, Springer D B, Clifford G D 2017 Physiol. Meas. 38 1730
Google Scholar
[4] Bartels A, Harder D 1992 Clin. Phys. Physiol. Meas. 13 249
Google Scholar
[5] Tanigawa N, Smith D, Craige E 1991 Jpn. Circ. J. 55 737
Google Scholar
[6] Zhang X Y, Zhang Y T 2006 Proceedings of the IEEE Engineering in Medicine and Biology Society New York, USA, August 30−September 3, 2006 p2888
[7] Peng R C, Yan W R, Zhang N L, Lin W H, Zhou X L, Zhang Y T 2015 Sensors-basel 15 23653
Google Scholar
[8] Bombardini T, Gemignani V, Bianchini E, Venneri L, Petersen C 2008 Cardiovasc. Ultrasoun. 6 41
Google Scholar
[9] Tao Y W CN104510491A [2015−04−15]
[10] 黄正钦 2014 硕士学位论文 (昆明: 云南大学)
Huang Z Q 2014 M. S. Thesis (Kunming: Yunnan University) (in Chinese)
[11] Shriram R, Wakankar A, Daimiwal N 2010 Proceedings of International Conference on Bioinformatics and Biomedical Technology Chengdu, China, April 16−18, 2010 p100971242
[12] Landowne M 1957 Circ. Res. 5 594
Google Scholar
[13] Payne R A, Symeonides C N, Webb D J, Maxwell S R J 2006 J. Appl. Physiol. 100 136
Google Scholar
[14] 蔡任圃 2012 科学月刊 507 236
Cai R P 2012 Science Monthly 507 236
[15] 周静, 杨永明, 何为 2005 中国生物医学工程学报 24 685
Google Scholar
Zhou J, Yang Y M, He W 2005 Chin. J. Biomed. Eng. 24 685
Google Scholar
[16] Ding X R, Zhao R, Yang G Z, Pettigrew R 2016 IEEE J. Biomed. Health 20 1
Google Scholar
[17] Lim K H, Shin Y D, Park S H, Bae J H, Lee H J, Kim S J 2013 Pak. J. Med. Sci. 29 1023
下载:
计量
- 文章访问数: 11372
- PDF下载量: 137
- 被引次数: 0
