-
In recent years, the study of partial synchronization of coupled oscillators in complex networks has attracted great attention. The underlying reason is both the extensive existence of the patterns of partial synchronization in brain network and their close relationship to brain functions of cognition and memory. In this paper, we briefly review the research progress in this field. According to the researches by different groups, we classify them as three types, i.e. chimera state, remote synchronization, and clustering synchronization. We mainly discuss the conditions of these three states, as well as their models, detections, and their applications in biology. We discuss the relationship among the three types of states and give some outlooks for future studies.
-
Keywords:
- complex network /
- chimera state /
- remote synchronization /
- cluster synchronization
[1] Pecora L M, Carroll T L 1990 Phys. Rev. Lett. 64 821
 Google Scholar
Google Scholar
[2] 刘宗华 2018 混沌动力学基础及其在大脑功能方面的应用 (北京: 科学出版社)
Liu Z H 2018 Chaotic Dynamics Foundation and Its Applications in Brain Functions (Beijing: Science Press) (in Chinese)
[3] Gray C M, Konig P, Engel A K, Singer W 1989 Nature 338 334
 Google Scholar
Google Scholar
[4] Hasler M, Maistrenko Y, Popovych O 1998 Phys. Rev. E 58 6843
 Google Scholar
Google Scholar
[5] Hu G, Zhang Y, Cerdeira H A, Chen S 2000 Phys. Rev. Lett. 85 3377
 Google Scholar
Google Scholar
[6] Zhang Y, Hu G, Cerdeira H A, Chen S, Braun T, Yao Y 2001 Phys. Rev. E 63 026211
 Google Scholar
Google Scholar
[7] Fu C, Lin W, Huang L, Wang X 2014 Phys. Rev. E 89 052908
 Google Scholar
Google Scholar
[8] Heisler I A, Braun T, Zhang Y, Hu G, Cerdeira H A 2003 Chaos 13 185
 Google Scholar
Google Scholar
[9] Kumar P, Verma D K, Parmananda P 2017 Phys. Lett. A 381 2337
 Google Scholar
Google Scholar
[10] Panaggio M J, Abrams D M 2015 Nonlinearity 28 R67
 Google Scholar
Google Scholar
[11] Majhi., Bera B K, Ghosh D, Perc M 2019 Phys. Life Rev. 28 100
 Google Scholar
Google Scholar
[12] Majhi S, Perc M, Ghosh D 2016 Sci. Rep. 6 39033
 Google Scholar
Google Scholar
[13] Kang L, Tian C, Huo S, Liu Z 2019 Sci. Rep. 9 14389
 Google Scholar
Google Scholar
[14] Roelfsema P R, Engel A K 1997 Nature 385 157
 Google Scholar
Google Scholar
[15] Vogels T P, Abbott L F 2005 J. Neurosci. 25 10786
 Google Scholar
Google Scholar
[16] Diesmann M, Gewaltig M, Aertsen A 1999 Nature 402 529
 Google Scholar
Google Scholar
[17] Benedek M, Bergner S, Konen T, Fink A, Neubauer A C 2011 Neuropsychologia 49 3505
 Google Scholar
Google Scholar
[18] Ayala G F, Dichter M, Gumnit R J, Matsumoto H, Spencer W A 1973 Brain Res. 52 1
 Google Scholar
Google Scholar
[19] Levy R, Hutchison W D, Lozano A M, Dostrovsky J O 2000 J. Neurosci. 20 7766
 Google Scholar
Google Scholar
[20] Abrams D M, Strogatz S H 2004 Phys. Rev. Lett. 93 174102
 Google Scholar
Google Scholar
[21] Rattenborg N C, Amlaner C J, Lima S L 2000 Neurosci. Biobehav. Rev. 24 817
 Google Scholar
Google Scholar
[22] Mathews C G, Lesku J A, Lima S L, Amlaner C J 2006 Ethology 112 286
 Google Scholar
Google Scholar
[23] Tamaki M, Bang J W, Watanabe T, Sasaki Y 2016 Curr. Biol. 26 1190
 Google Scholar
Google Scholar
[24] Kuramoto Y, Battogtokh D 2002 Nonlinear Phenom. Complex Syst. 5 380
[25] Abrams D M, Mirollo R, Strogatz S H, Wiley D A 2008 Phys. Rev. Lett. 101 084103
 Google Scholar
Google Scholar
[26] Ott E, Antonsen T M 2008 Chaos 18 037113
 Google Scholar
Google Scholar
[27] Ma R, Wang J, Liu Z 2010 Europhys. Lett. 91 40006
 Google Scholar
Google Scholar
[28] Indic P, Schwartz W J, Paydarfar D 2008 J. R. Soc. Interface 5 873
 Google Scholar
Google Scholar
[29] Omelchenko O E, Wolfrum M, Yanchuk S, Maistrenko Y L, Sudakov O 2012 Phys. Rev. E 85 036210
 Google Scholar
Google Scholar
[30] Maistrenko Y, Sudakov O, Osiv O, Maistrenko V 2015 New J. Phys. 17 073037
 Google Scholar
Google Scholar
[31] Yeldesbay A, Pikovsky A, Rosenblum M 2014 Phys. Rev. Lett. 112 144103
 Google Scholar
Google Scholar
[32] Sethia G C, Sen A, Atay F M 2008 Phys. Rev. Lett. 100 144102
 Google Scholar
Google Scholar
[33] Tian C, Cao L, Bi H, Xu K, Liu Z 2018 Nonlinear Dyn. 93 1695
 Google Scholar
Google Scholar
[34] Zhu Y, Zheng Z, Yang J 2014 Phys. Rev. E 89 022914
 Google Scholar
Google Scholar
[35] 郑志刚, 翟云 2020 中国科学: 物理学 力学 天文学 50 010505
Zheng Z G, Zhai Y 2020 SCIENTIA SINICA Physica, Mechanica & Astronomica 50 010505
[36] Kemeth F P, Haugland S W, Schmidt L, Kevrekidis I G, Krischer K 2016 Chaos 26 094815
 Google Scholar
Google Scholar
[37] Omelchenko I, Omelchenko E, Hovel P, Scholl E 2013 Phys. Rev. Lett. 110 224101
 Google Scholar
Google Scholar
[38] Hizanidis J, Kanas V G, Bezerianos A, Bountis T 2014 Int. J. Bifurcation Chaos 24 1450030
 Google Scholar
Google Scholar
[39] Sakaguchi H 2006 Phys. Rev. E 73 031907
 Google Scholar
Google Scholar
[40] Olmi S, Politi A, Torcini A 2010 Europhys. Lett. 92 60007
 Google Scholar
Google Scholar
[41] Santos M S, et al. 2017 Chaos, Solitons Fractals 101 86
 Google Scholar
Google Scholar
[42] Hizanidis J, Kouvaris N E, Zamora-Lopez G, Diaz-Guilera A, Antonopoulos C G 2016 Sci. Rep. 6 19845
 Google Scholar
Google Scholar
[43] Tian C, Bi H, Zhang X, Guan S, Liu Z 2017 Phys. Rev. E 96 052209
 Google Scholar
Google Scholar
[44] Tian C, Zhang X, Wang Z, Liu Z 2017 Front. Phys. 12 128904
 Google Scholar
Google Scholar
[45] Chouzouris T, Omelchenko I, Zakharova A, et al. 2018 Chaos 28 045112
 Google Scholar
Google Scholar
[46] Bansal K, Garcia J O, Tompson S H, Verstynen T, Vettel J M, Muldoon S F 2019 Sci. Adv. 5 eaau8535
 Google Scholar
Google Scholar
[47] Huo S, Tian C, Kang L, Liu Z 2019 Nonlinear Dyn. 96 75
 Google Scholar
Google Scholar
[48] Hagmann P, et al. 2008 PLoS Biol. 6 1479
[49] Honey C J, et al. 2009 Proc. Natl. Acad. Sci. U.S.A. 106 2035
 Google Scholar
Google Scholar
[50] Tinsley M R, Nkomo S, Showalter K 2012 Nat. Phys. 8 662
 Google Scholar
Google Scholar
[51] Nkomo S, Tinsley M R, Showalter K 2013 Phys. Rev. Lett. 110 244102
 Google Scholar
Google Scholar
[52] Martens E A, Thutupalli S, Fourriere A, et al. 2013 Proc. Natl. Acad. Sci. U.S.A. 110 10563
 Google Scholar
Google Scholar
[53] Gambuzza L V, Buscarino A, Chessari S, et al. 2014 Phys. Rev. E 90 032905
 Google Scholar
Google Scholar
[54] Totz J F, Rode J, Tinsley M R, Showalter K, Engel H 2018 Nat. Phys. 14 282
 Google Scholar
Google Scholar
[55] Ito T, Kulkarni K R, Schultz D H, Mill R D, Chen R H, Solomyak L I, Cole M W 2017 Nat. Commun. 8 1027
 Google Scholar
Google Scholar
[56] Sporns O 2013 Curr. Opin. Neurobiol. 23 162
 Google Scholar
Google Scholar
[57] Bergner A, Frasca M, Sciuto G, Buscarino A, Ngamga E J, Fortuna L, Kurths J 2012 Phys. Rev. E 85 026208
 Google Scholar
Google Scholar
[58] Vicente R, Gollo L L, Mirasso C R, Fischer I, Pipa G 2008 Proc. Natl. Acad. Sci. U.S.A. 105 17157
 Google Scholar
Google Scholar
[59] Minati L 2015 Chaos 25 123107
 Google Scholar
Google Scholar
[60] Nicosia V, Valencia M, Chavez M, Diaz-Guilera A, Latora V 2013 Phys. Rev. Lett. 110 174102
 Google Scholar
Google Scholar
[61] Zhang L, Motter A E, Nishikawa T 2017 Phys. Rev. Lett. 118 174102
 Google Scholar
Google Scholar
[62] Kang L, Wang Z, Huo S, Tian C, Liu Z 2020 Nonlinear Dyn. 99 1577
 Google Scholar
Google Scholar
[63] Gambuzza L V, Frasca M, Gomez-Gardenes J 2015 Europhys. Lett. 110 20010
 Google Scholar
Google Scholar
[64] Punetha N, Ujjwal S R, Atay F M, Ramaswamy R 2015 Phys. Rev. E 91 022922
 Google Scholar
Google Scholar
[65] Leyva I, Sendina-Nadal I, Sevilla-Escoboza R, Vera-Avila V P, Chholak P, Boccaletti S 2018 Sci. Rep. 8 8629
 Google Scholar
Google Scholar
[66] Dahms T, Lehnert J, Scholl E 2012 Phys. Rev. E 86 016202
 Google Scholar
Google Scholar
[67] Jalan S, Singh A, Acharyya S, Kurths J 2015 Phys. Rev. E 91 022901
 Google Scholar
Google Scholar
[68] Cho Y S, Nishikawa T, Motter A E 2017 Phys. Rev. Lett. 119 084101
 Google Scholar
Google Scholar
[69] Siddique A B, Pecora L, Hart J D, Sorrentino F 2018 Phys. Rev. E 97 042217
 Google Scholar
Google Scholar
[70] 王新刚 2020 中国科学: 物理学 力学 天文学 50 010503
Wang X G 2020 SCIENTIA SINICA Physica, Mechanica & Astronomica 50 010503
[71] Williams C R S, Murphy T E, Roy R, Sorrentino F, Dahms T, Scholl E 2013 Phys. Rev. Lett. 110 064104
 Google Scholar
Google Scholar
[72] Pecora L M, Sorrentino F, Hagerstrom A M, Murphy T E, Roy R 2014 Nat. Commun. 5 4079
 Google Scholar
Google Scholar
[73] Sorrentino F, Pecora L M, Hagerstrom M, Murphy T E, Roy R 2016 Sci. Adv. 2 e1501737
 Google Scholar
Google Scholar
[74] Cao B, Wang Y F, Wang L, Yu Y Z, Wang X G 2018 Front. Phys. 13 130505
 Google Scholar
Google Scholar
[75] Wang R, Lin P, Liu M, Wu Y, Zhou T, Zhou C 2019 Phys. Rev. Lett. 123 038301
 Google Scholar
Google Scholar
-
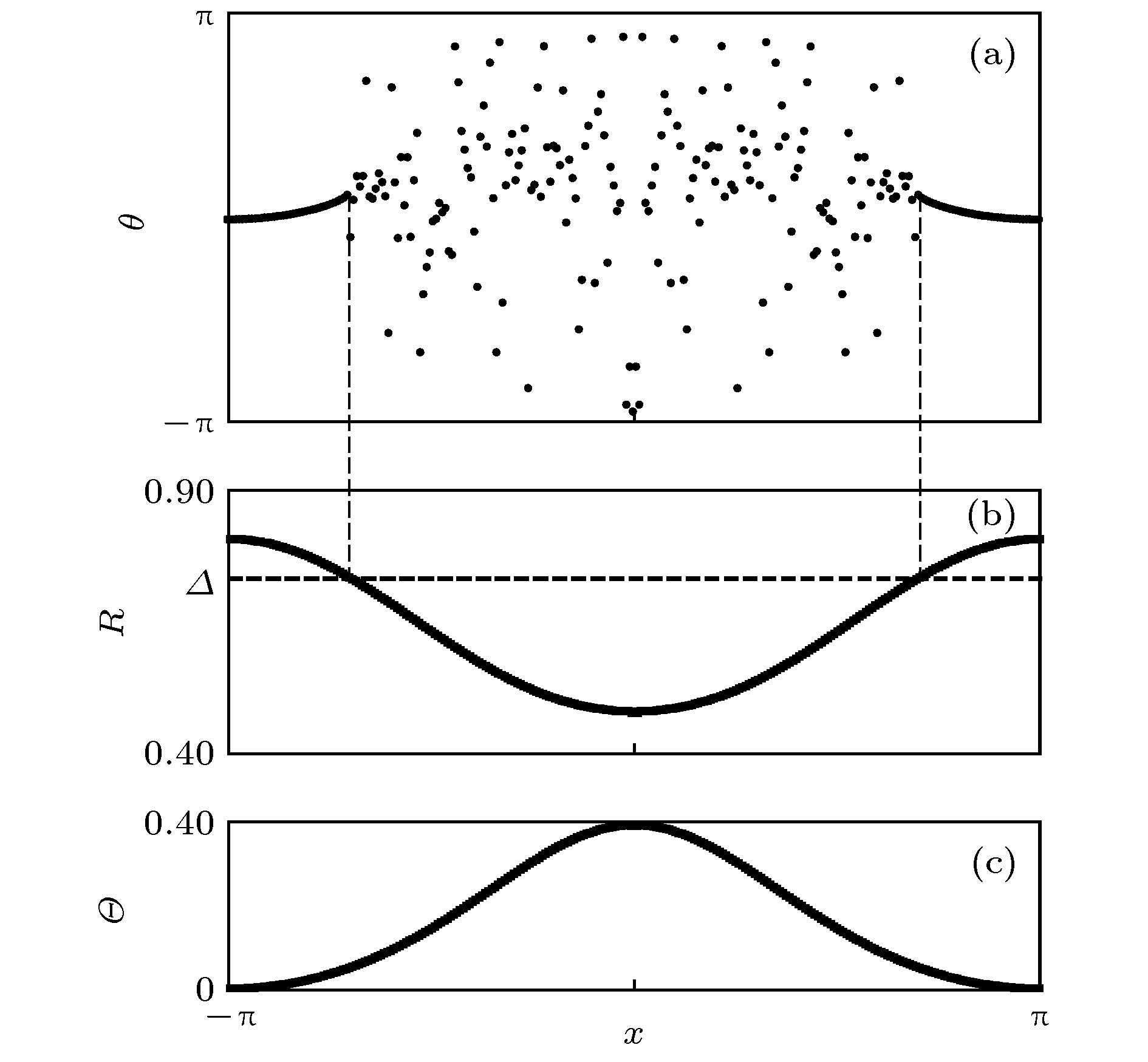
图 1 (a)奇异态的斑图, 参数:
$A=0.995,\; \beta=0.18, $ N = 256个振子; (b)来自于方程(3)的局部相关联$R(x)$ , 锁频的振子满足$R(x)\geqslant \varDelta$ ; (c) 局部平均相$\varTheta(x)$ . 改编自文献[20]Fig. 1. (a) Phase pattern for a chimera state, parameters:
$A=0.995,\; \beta=0.18, N=256$ oscillators; (b) local phase coherence$R(x)$ , computed from Eq. (3), locked oscillators satisfy$R(x)\geqslant \varDelta$ ; (c) local average phase$\varTheta(x)$ . Figure adapted from Ref. [20].图 2 序参量r随时间的变化, 其中参数
$N_1=N_2=128$ ,$\beta=0.1 $ (a)$ A=0.20 $ , 稳定奇异态; (b)$ A=0.28 $ , 呼吸奇异态; (c)$ A=0.35 $ , 长周期呼吸子. 初值条件取为靠近奇异态, 结果为丢掉了2000个暂态时间单位后的情形. 改编自文献[25]Fig. 2. Order parameter r versus time. In all three panels,
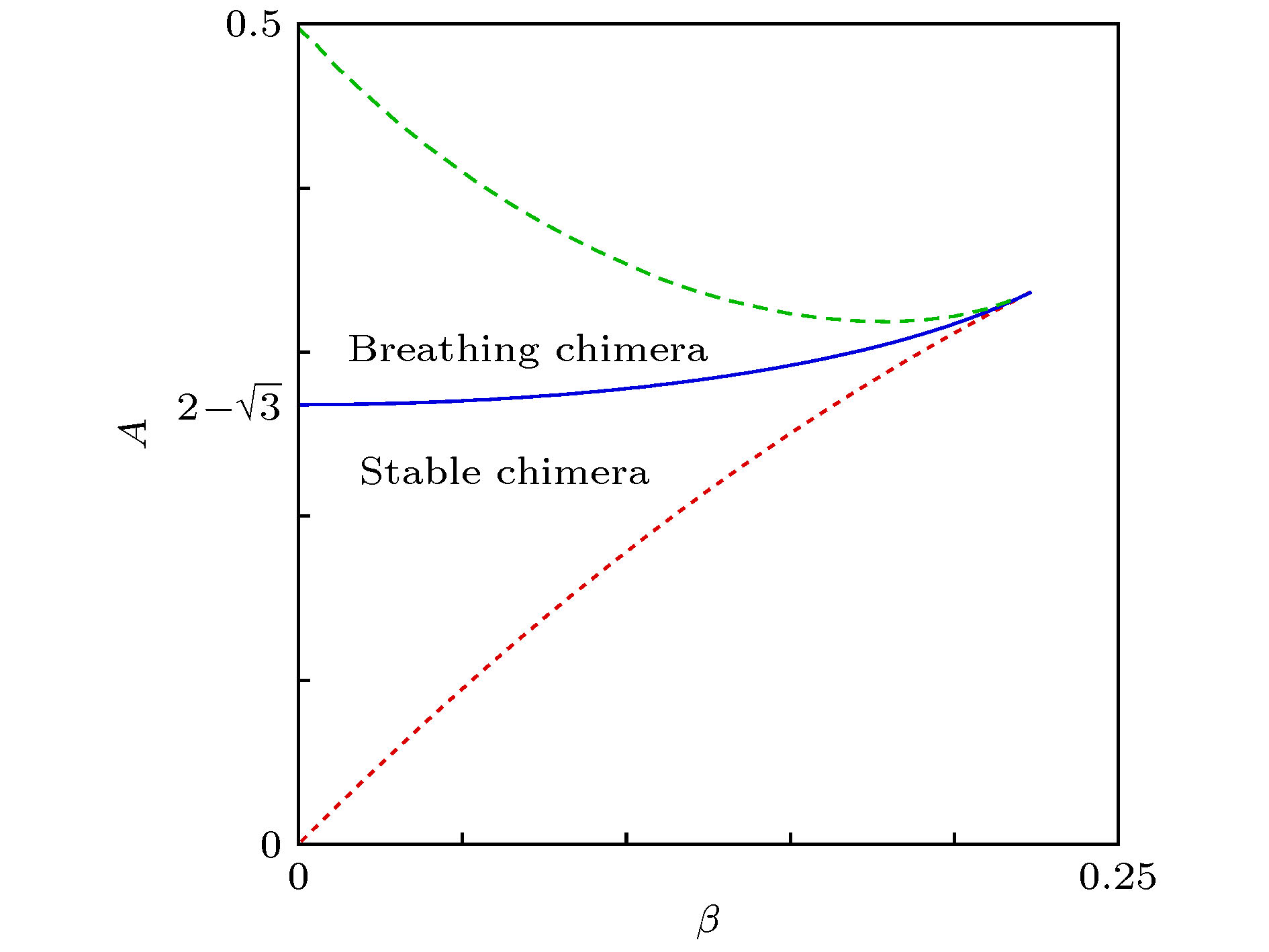
$N_1=N_2=128$ and$\beta=0.1$ : (a)$ A=0.20 $ , stable chimera; (b)$ A=0.28 $ , breathing chimera; (c)$ A=0.35 $ , long period breather. Numerical integration began from an initial condition close to the chimera state, and plots shown begin after allowing a transient time of 2000 units. Figure adapted from Ref. [25].图 3 奇异态的稳定性相图 分岔曲线: 鞍-节分岔(点划线)、超临界Hopf分岔(实线), 两者都是解析发现的; 同宿分岔(虚线), 数值发现的. 改编自文献[25]
Fig. 3. Stability diagram for chimera states. Bifurcation curves: saddle-node (dotted line) and supercritical Hopf (solid line), both found analytically; homoclinic (dashed line), found numerically. Figure adapted from Ref. [25].
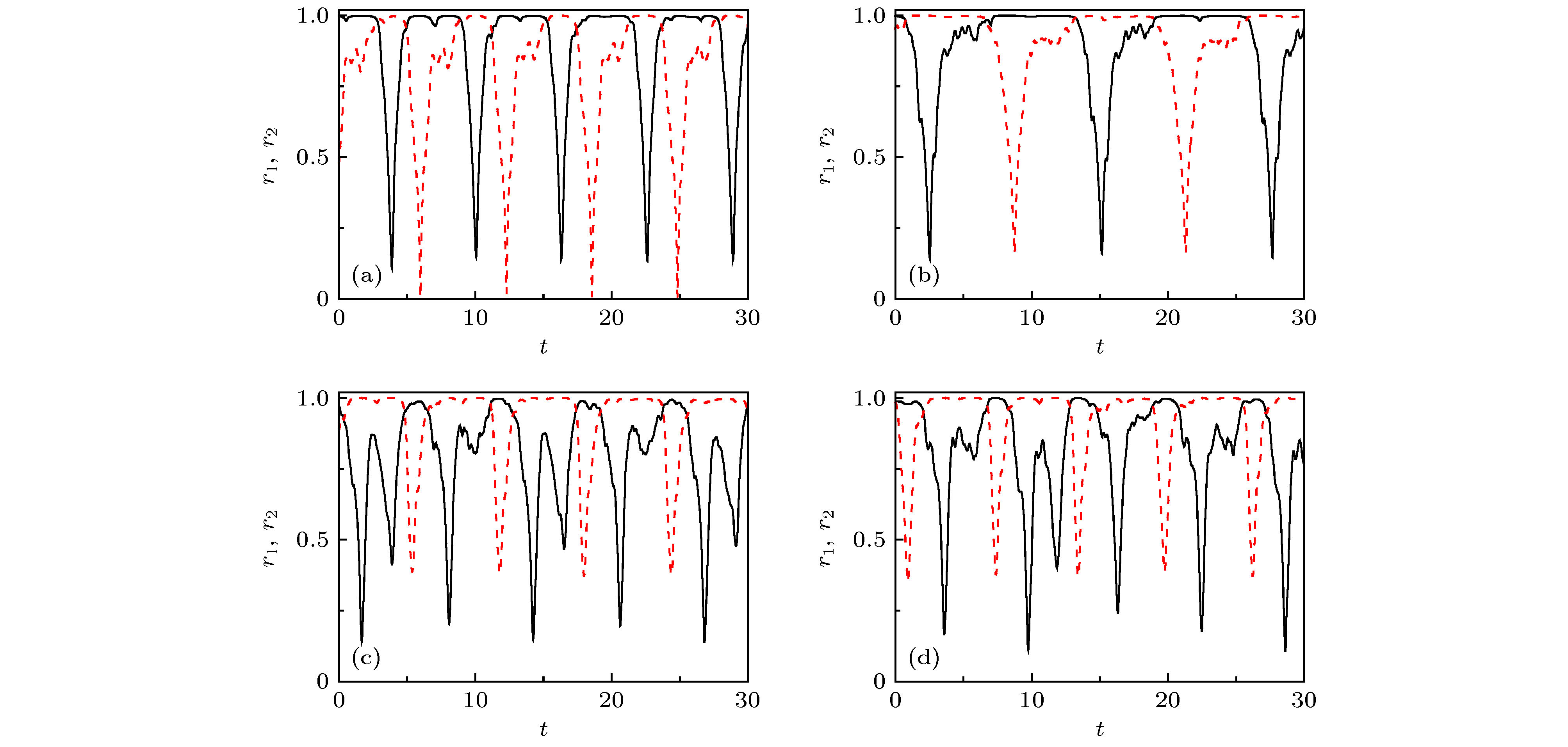
图 4 外部信号诱导的交替奇异态 (a)
$A=1, \varOmega=1$ 与$\tau_2 =1.5$ ; (b)$A=1, \varOmega=0.5$ 与$\tau_2 =1.5$ ; (c)$A=1.5, \varOmega=1$ 与$\tau_2 =1.5$ ; (d)$A=1, \varOmega=1$ 与$\tau_2 =3.0$ . 改编自文献[27]Fig. 4. Alternating chimera states induced by external signals: (a)
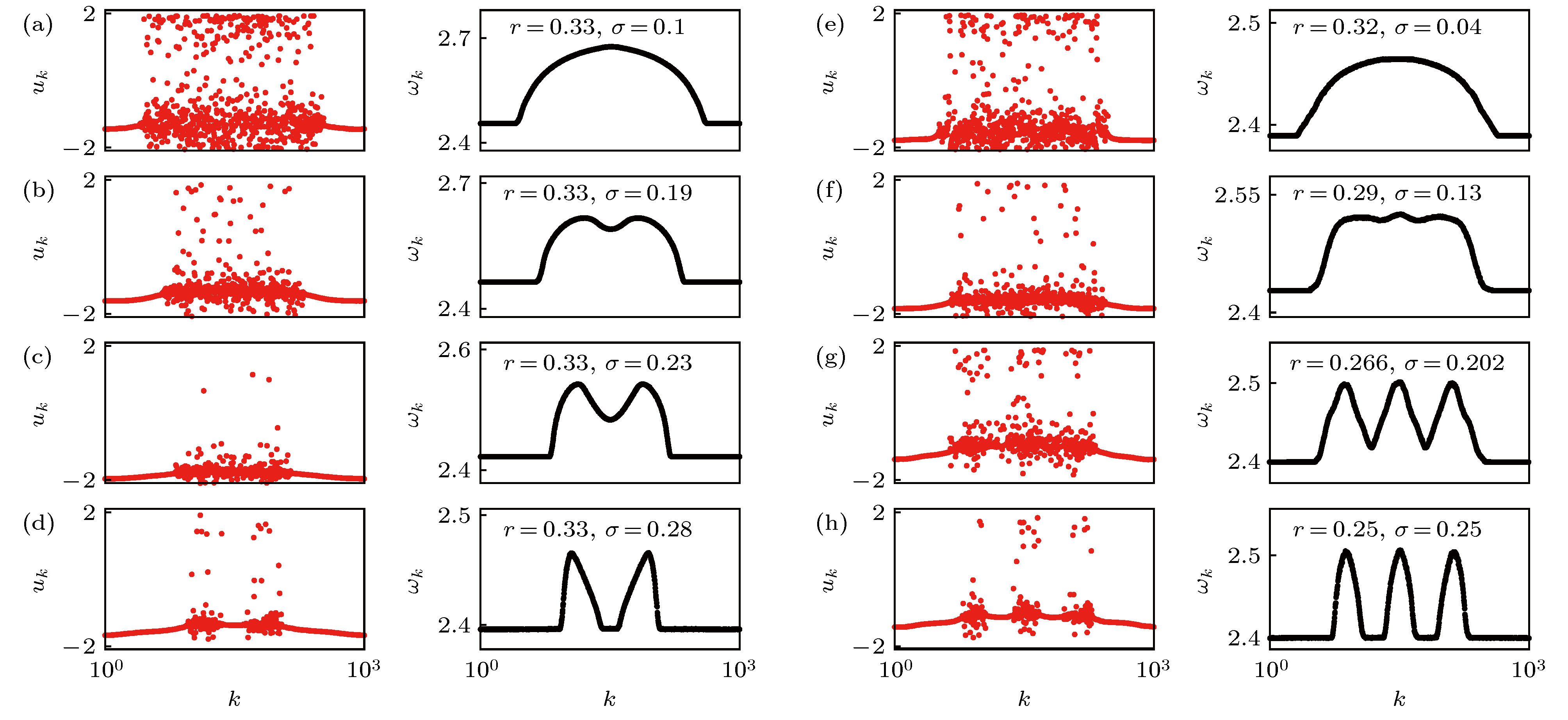
$A=1, \varOmega=1$ and$\tau_2 =1.5$ ; (b)$A=1, \varOmega=0.5$ and$\tau_2 =1.5$ ; (c)$A=1.5, \varOmega=1$ and$\tau_2 =1.5$ ; (d)$A=1, \varOmega=1$ and$\tau_2 =3.0$ . Figure adapted from Ref. [27].图 6 单一的非相关区域变成多个非相关区域的情形 (a)—(d)变成两个非相关区域: (e)—(h)变成三个非相关区域. 在每个子图中, 左列代表变量
$u_k$ 的快照, 右列为对应的平均相速度. 改编自文献[37]Fig. 6. Transition from a classical chimera state with one incoherent domain to multichimera states with two (a)–(d), and three (e)–(h) incoherent domains. In each panel the left column shows snapshot of variables uk, and the right column shows the corresponding mean phase velocities. Figure adapted from Ref. [37].
图 7 大脑皮层网中的四个典型的行为. 参数为
$\alpha={\text{π}}/2-0.1$ , 上层为网络A、下层为网络B、子图为它们对应的动力学$u_{i}$ (a)与(e)的耦合参数为($\lambda_{\rm {in}}=0.1$ ,$\lambda_{\rm {out}}=0.3$ ); (b)与(f)的耦合参数为($\lambda_{\rm {in}}=0.1$ ,$\lambda_{\rm out}=1.8$ )、(c)与(g)的耦合参数为($\lambda_{in}=0.4$ ,$\lambda_{\rm {out}}=3.5$ ); (d)与(h)的耦合参数为($\lambda_{\rm {in}}=4.0$ ,$\lambda_{\rm {out}}=3.5$ ). 改编自文献[13]Fig. 7. Four typical behaviors in the cerebral cortex with
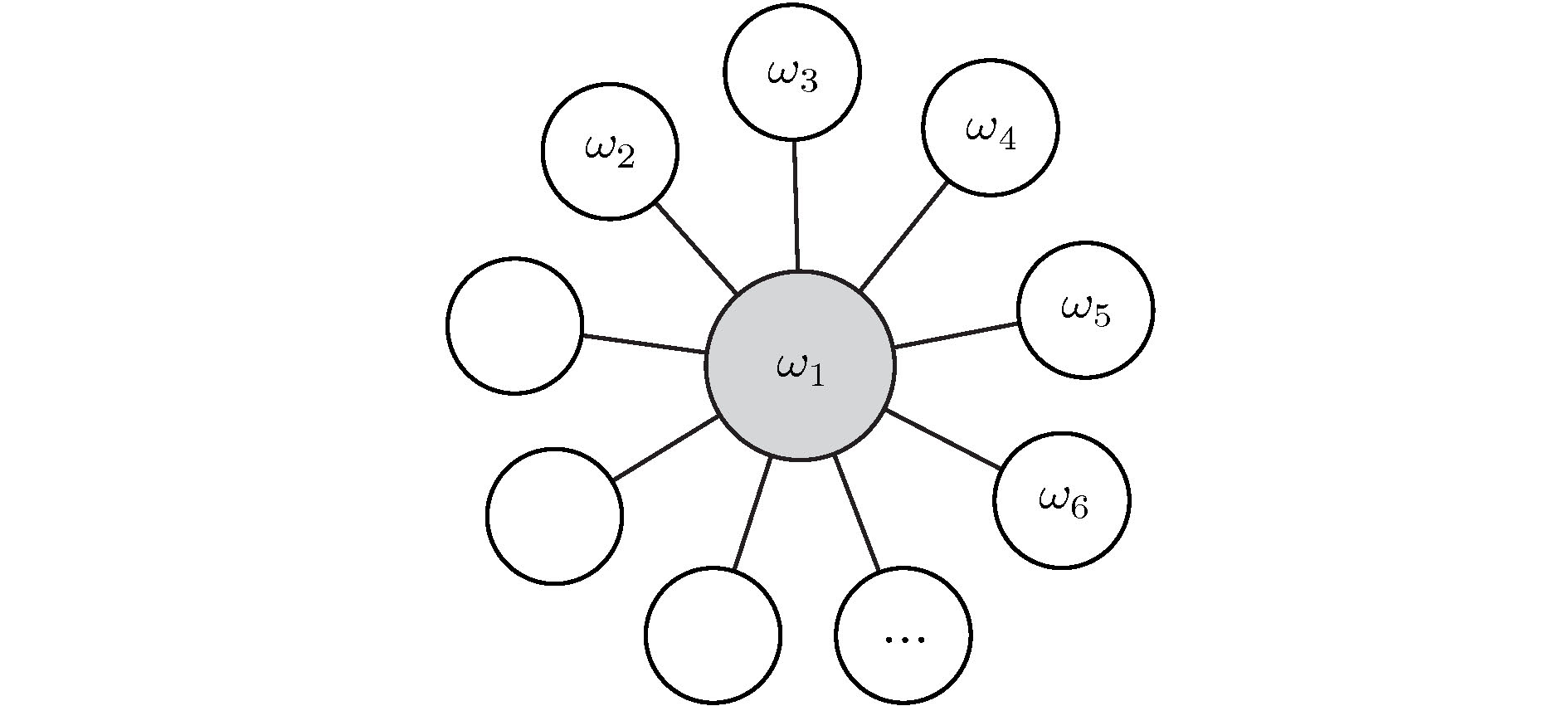
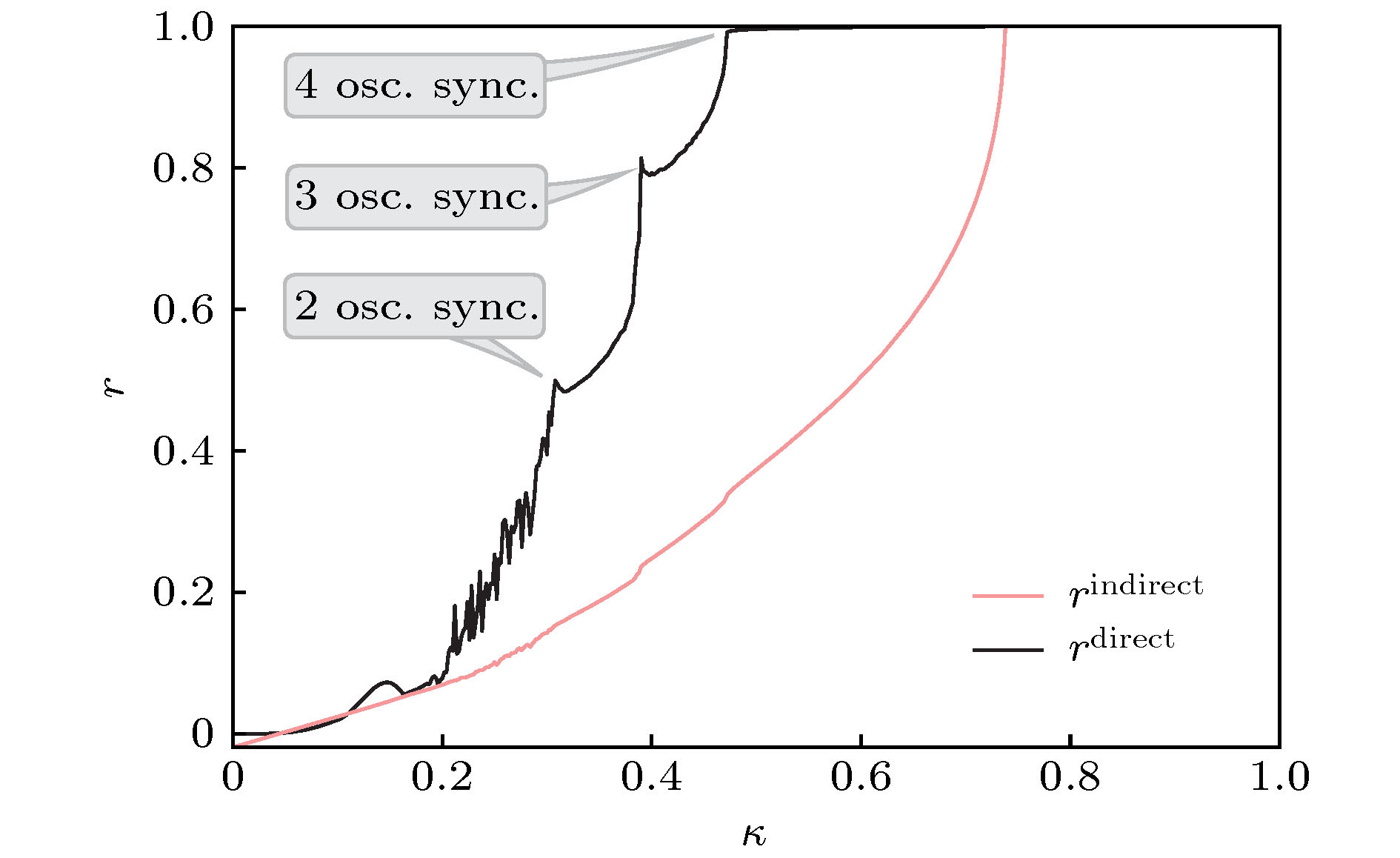
$\alpha={\text{π}}/2-0.1$ where the up and down panels represent the two hemispheres, respectively, and the insets are their corresponding dynamics of$u_{i}$ at a moment t. The parameters are$\lambda_{\rm {in}}=0.1$ and$\lambda_{\rm {out}}=0.3$ in panels (a) and (e) of disorder;$\lambda_{\rm {in}}=0.1$ and$\lambda_{\rm {out}}=1.8$ in panels (b) and (f) of chimera state;$\lambda_{\rm {in}}=0.4$ and$\lambda_{\rm {out}}=3.5$ in panels (c) and (g) of an emergent state conceptually similar to the state of unihemispheric sleep; and$\lambda_{\rm {in}}=4.0$ and$\lambda_{\rm {out}}=3.5$ in panels (d) and (h) of synchronization. Figure adapted from Ref. [13].图 9 相同步的过程. 相同步的建立清晰可见, 其中的三个标注分别表明两个、三个与四个叶子节点间的同步化. 改编自文献[57]
Fig. 9. Transition to PS for the hub motif. From the plot the onset of RS is clearly visible. The three annotations indicate synchronization between two, three, and four peripheral oscillators, respectively. Figure adapted from Ref. [57]
图 10 A与C组之间的遥同步同步由B组的介导来完成. 节点的颜色代表了它们的状态, 可见节点1与N是完全同步的, 而B组中节点的动力学是无关的. 改编自文献[61]
Fig. 10. Remote synchronization between node groups A and C mediated by incoherence in group B. The colors of the nodes schematically represent their states, indicating that nodes 1 and N are identically synchronized, while the dynamics of the nodes in B are incoherent. Figure adapted from Ref. [61].
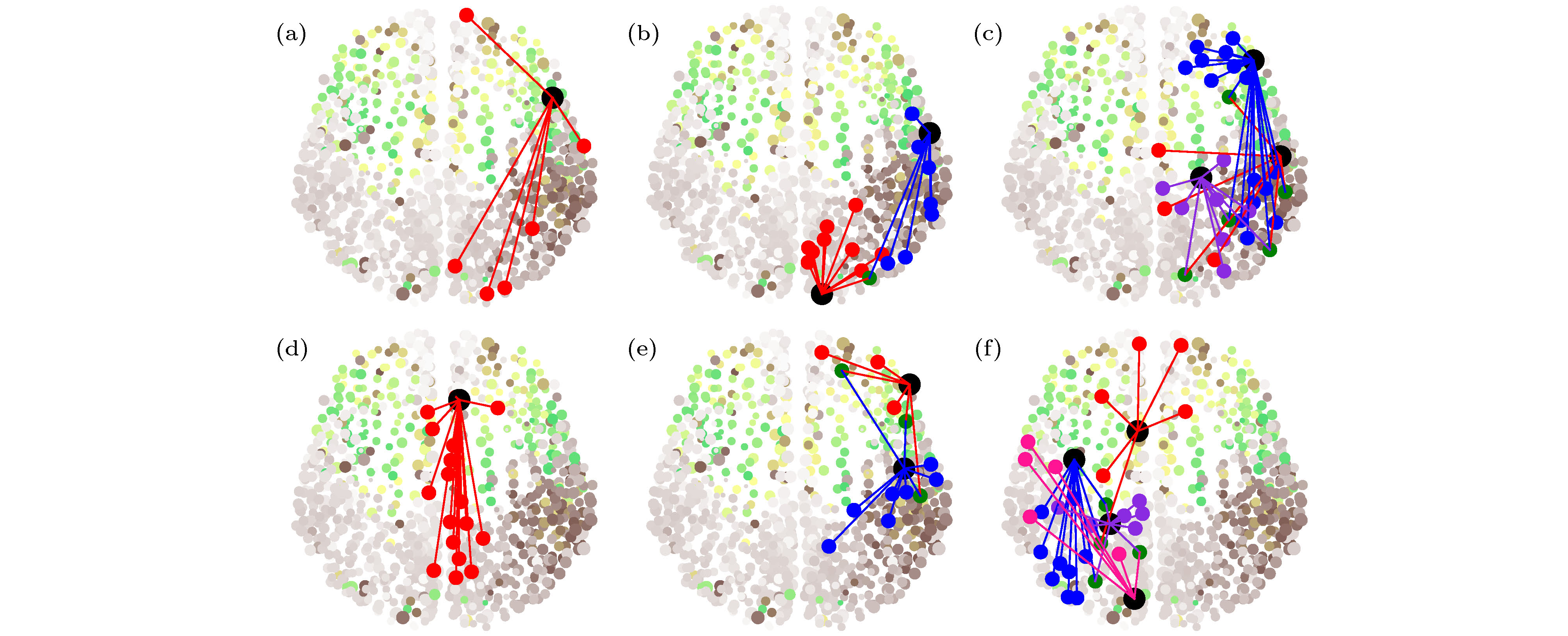
图 11 参数取
$\tau=0.5$ 与$\varepsilon=0.1$ 时的六个典型的遥同步斑图. 每个斑图都是按如下条件挑选的: (i) 在中心节点与叶子节点间没有同步化; (ii)所有的叶子节点均相互同步化. 改编自文献[62]Fig. 11. Six typical patterns of RS for
$\tau=0.5$ and$\varepsilon=0.1$ . Each pattern is chosen by the conditions: (i) There is no synchronization between the hub and its peripheral nodes; (ii) all the peripheral nodes are synchronized each other. Figure adapted from Ref. [62]图 13 网络中对称性的例子 (a) 四个全同振子通过三根连线耦合的网络; (b) 一个反射操作后的同一网络; (c) 一个旋转操作后的同一网络; (d) 一个
$11$ 个节点的网络有三个集团(蓝、绿、白). 改编自文献[73]Fig. 13. Examples of symmetries in networks: (a) A network of four identical oscillators coupled through three identical links; (b) the same network after a reflection opera-tion; (c) the same network after a rotation operation; (d) an 11-node network showing three clusters (blue, green, and white). Figure adapted from Ref. [73].
图 14 五个节点的网络中的集团斑图 左边: 当网络连接为邻接矩阵(方程(20))时所有可能的斑图; 右边: 当网络连接为拉普拉斯矩阵(方程(21))时额外的斑图. 改编自文献[73]
Fig. 14. Patterns of clusters in a five-node network. Left: All possible patterns displayed when the network connectivity is given by the adjacency matrix (Eq. (20)); right: Additional patterns displayed when the network connectivity is given by the Laplacian ma-trix (Eq. (21)). Figure adapted from Ref. [73].
-
[1] Pecora L M, Carroll T L 1990 Phys. Rev. Lett. 64 821
 Google Scholar
Google Scholar
[2] 刘宗华 2018 混沌动力学基础及其在大脑功能方面的应用 (北京: 科学出版社)
Liu Z H 2018 Chaotic Dynamics Foundation and Its Applications in Brain Functions (Beijing: Science Press) (in Chinese)
[3] Gray C M, Konig P, Engel A K, Singer W 1989 Nature 338 334
 Google Scholar
Google Scholar
[4] Hasler M, Maistrenko Y, Popovych O 1998 Phys. Rev. E 58 6843
 Google Scholar
Google Scholar
[5] Hu G, Zhang Y, Cerdeira H A, Chen S 2000 Phys. Rev. Lett. 85 3377
 Google Scholar
Google Scholar
[6] Zhang Y, Hu G, Cerdeira H A, Chen S, Braun T, Yao Y 2001 Phys. Rev. E 63 026211
 Google Scholar
Google Scholar
[7] Fu C, Lin W, Huang L, Wang X 2014 Phys. Rev. E 89 052908
 Google Scholar
Google Scholar
[8] Heisler I A, Braun T, Zhang Y, Hu G, Cerdeira H A 2003 Chaos 13 185
 Google Scholar
Google Scholar
[9] Kumar P, Verma D K, Parmananda P 2017 Phys. Lett. A 381 2337
 Google Scholar
Google Scholar
[10] Panaggio M J, Abrams D M 2015 Nonlinearity 28 R67
 Google Scholar
Google Scholar
[11] Majhi., Bera B K, Ghosh D, Perc M 2019 Phys. Life Rev. 28 100
 Google Scholar
Google Scholar
[12] Majhi S, Perc M, Ghosh D 2016 Sci. Rep. 6 39033
 Google Scholar
Google Scholar
[13] Kang L, Tian C, Huo S, Liu Z 2019 Sci. Rep. 9 14389
 Google Scholar
Google Scholar
[14] Roelfsema P R, Engel A K 1997 Nature 385 157
 Google Scholar
Google Scholar
[15] Vogels T P, Abbott L F 2005 J. Neurosci. 25 10786
 Google Scholar
Google Scholar
[16] Diesmann M, Gewaltig M, Aertsen A 1999 Nature 402 529
 Google Scholar
Google Scholar
[17] Benedek M, Bergner S, Konen T, Fink A, Neubauer A C 2011 Neuropsychologia 49 3505
 Google Scholar
Google Scholar
[18] Ayala G F, Dichter M, Gumnit R J, Matsumoto H, Spencer W A 1973 Brain Res. 52 1
 Google Scholar
Google Scholar
[19] Levy R, Hutchison W D, Lozano A M, Dostrovsky J O 2000 J. Neurosci. 20 7766
 Google Scholar
Google Scholar
[20] Abrams D M, Strogatz S H 2004 Phys. Rev. Lett. 93 174102
 Google Scholar
Google Scholar
[21] Rattenborg N C, Amlaner C J, Lima S L 2000 Neurosci. Biobehav. Rev. 24 817
 Google Scholar
Google Scholar
[22] Mathews C G, Lesku J A, Lima S L, Amlaner C J 2006 Ethology 112 286
 Google Scholar
Google Scholar
[23] Tamaki M, Bang J W, Watanabe T, Sasaki Y 2016 Curr. Biol. 26 1190
 Google Scholar
Google Scholar
[24] Kuramoto Y, Battogtokh D 2002 Nonlinear Phenom. Complex Syst. 5 380
[25] Abrams D M, Mirollo R, Strogatz S H, Wiley D A 2008 Phys. Rev. Lett. 101 084103
 Google Scholar
Google Scholar
[26] Ott E, Antonsen T M 2008 Chaos 18 037113
 Google Scholar
Google Scholar
[27] Ma R, Wang J, Liu Z 2010 Europhys. Lett. 91 40006
 Google Scholar
Google Scholar
[28] Indic P, Schwartz W J, Paydarfar D 2008 J. R. Soc. Interface 5 873
 Google Scholar
Google Scholar
[29] Omelchenko O E, Wolfrum M, Yanchuk S, Maistrenko Y L, Sudakov O 2012 Phys. Rev. E 85 036210
 Google Scholar
Google Scholar
[30] Maistrenko Y, Sudakov O, Osiv O, Maistrenko V 2015 New J. Phys. 17 073037
 Google Scholar
Google Scholar
[31] Yeldesbay A, Pikovsky A, Rosenblum M 2014 Phys. Rev. Lett. 112 144103
 Google Scholar
Google Scholar
[32] Sethia G C, Sen A, Atay F M 2008 Phys. Rev. Lett. 100 144102
 Google Scholar
Google Scholar
[33] Tian C, Cao L, Bi H, Xu K, Liu Z 2018 Nonlinear Dyn. 93 1695
 Google Scholar
Google Scholar
[34] Zhu Y, Zheng Z, Yang J 2014 Phys. Rev. E 89 022914
 Google Scholar
Google Scholar
[35] 郑志刚, 翟云 2020 中国科学: 物理学 力学 天文学 50 010505
Zheng Z G, Zhai Y 2020 SCIENTIA SINICA Physica, Mechanica & Astronomica 50 010505
[36] Kemeth F P, Haugland S W, Schmidt L, Kevrekidis I G, Krischer K 2016 Chaos 26 094815
 Google Scholar
Google Scholar
[37] Omelchenko I, Omelchenko E, Hovel P, Scholl E 2013 Phys. Rev. Lett. 110 224101
 Google Scholar
Google Scholar
[38] Hizanidis J, Kanas V G, Bezerianos A, Bountis T 2014 Int. J. Bifurcation Chaos 24 1450030
 Google Scholar
Google Scholar
[39] Sakaguchi H 2006 Phys. Rev. E 73 031907
 Google Scholar
Google Scholar
[40] Olmi S, Politi A, Torcini A 2010 Europhys. Lett. 92 60007
 Google Scholar
Google Scholar
[41] Santos M S, et al. 2017 Chaos, Solitons Fractals 101 86
 Google Scholar
Google Scholar
[42] Hizanidis J, Kouvaris N E, Zamora-Lopez G, Diaz-Guilera A, Antonopoulos C G 2016 Sci. Rep. 6 19845
 Google Scholar
Google Scholar
[43] Tian C, Bi H, Zhang X, Guan S, Liu Z 2017 Phys. Rev. E 96 052209
 Google Scholar
Google Scholar
[44] Tian C, Zhang X, Wang Z, Liu Z 2017 Front. Phys. 12 128904
 Google Scholar
Google Scholar
[45] Chouzouris T, Omelchenko I, Zakharova A, et al. 2018 Chaos 28 045112
 Google Scholar
Google Scholar
[46] Bansal K, Garcia J O, Tompson S H, Verstynen T, Vettel J M, Muldoon S F 2019 Sci. Adv. 5 eaau8535
 Google Scholar
Google Scholar
[47] Huo S, Tian C, Kang L, Liu Z 2019 Nonlinear Dyn. 96 75
 Google Scholar
Google Scholar
[48] Hagmann P, et al. 2008 PLoS Biol. 6 1479
[49] Honey C J, et al. 2009 Proc. Natl. Acad. Sci. U.S.A. 106 2035
 Google Scholar
Google Scholar
[50] Tinsley M R, Nkomo S, Showalter K 2012 Nat. Phys. 8 662
 Google Scholar
Google Scholar
[51] Nkomo S, Tinsley M R, Showalter K 2013 Phys. Rev. Lett. 110 244102
 Google Scholar
Google Scholar
[52] Martens E A, Thutupalli S, Fourriere A, et al. 2013 Proc. Natl. Acad. Sci. U.S.A. 110 10563
 Google Scholar
Google Scholar
[53] Gambuzza L V, Buscarino A, Chessari S, et al. 2014 Phys. Rev. E 90 032905
 Google Scholar
Google Scholar
[54] Totz J F, Rode J, Tinsley M R, Showalter K, Engel H 2018 Nat. Phys. 14 282
 Google Scholar
Google Scholar
[55] Ito T, Kulkarni K R, Schultz D H, Mill R D, Chen R H, Solomyak L I, Cole M W 2017 Nat. Commun. 8 1027
 Google Scholar
Google Scholar
[56] Sporns O 2013 Curr. Opin. Neurobiol. 23 162
 Google Scholar
Google Scholar
[57] Bergner A, Frasca M, Sciuto G, Buscarino A, Ngamga E J, Fortuna L, Kurths J 2012 Phys. Rev. E 85 026208
 Google Scholar
Google Scholar
[58] Vicente R, Gollo L L, Mirasso C R, Fischer I, Pipa G 2008 Proc. Natl. Acad. Sci. U.S.A. 105 17157
 Google Scholar
Google Scholar
[59] Minati L 2015 Chaos 25 123107
 Google Scholar
Google Scholar
[60] Nicosia V, Valencia M, Chavez M, Diaz-Guilera A, Latora V 2013 Phys. Rev. Lett. 110 174102
 Google Scholar
Google Scholar
[61] Zhang L, Motter A E, Nishikawa T 2017 Phys. Rev. Lett. 118 174102
 Google Scholar
Google Scholar
[62] Kang L, Wang Z, Huo S, Tian C, Liu Z 2020 Nonlinear Dyn. 99 1577
 Google Scholar
Google Scholar
[63] Gambuzza L V, Frasca M, Gomez-Gardenes J 2015 Europhys. Lett. 110 20010
 Google Scholar
Google Scholar
[64] Punetha N, Ujjwal S R, Atay F M, Ramaswamy R 2015 Phys. Rev. E 91 022922
 Google Scholar
Google Scholar
[65] Leyva I, Sendina-Nadal I, Sevilla-Escoboza R, Vera-Avila V P, Chholak P, Boccaletti S 2018 Sci. Rep. 8 8629
 Google Scholar
Google Scholar
[66] Dahms T, Lehnert J, Scholl E 2012 Phys. Rev. E 86 016202
 Google Scholar
Google Scholar
[67] Jalan S, Singh A, Acharyya S, Kurths J 2015 Phys. Rev. E 91 022901
 Google Scholar
Google Scholar
[68] Cho Y S, Nishikawa T, Motter A E 2017 Phys. Rev. Lett. 119 084101
 Google Scholar
Google Scholar
[69] Siddique A B, Pecora L, Hart J D, Sorrentino F 2018 Phys. Rev. E 97 042217
 Google Scholar
Google Scholar
[70] 王新刚 2020 中国科学: 物理学 力学 天文学 50 010503
Wang X G 2020 SCIENTIA SINICA Physica, Mechanica & Astronomica 50 010503
[71] Williams C R S, Murphy T E, Roy R, Sorrentino F, Dahms T, Scholl E 2013 Phys. Rev. Lett. 110 064104
 Google Scholar
Google Scholar
[72] Pecora L M, Sorrentino F, Hagerstrom A M, Murphy T E, Roy R 2014 Nat. Commun. 5 4079
 Google Scholar
Google Scholar
[73] Sorrentino F, Pecora L M, Hagerstrom M, Murphy T E, Roy R 2016 Sci. Adv. 2 e1501737
 Google Scholar
Google Scholar
[74] Cao B, Wang Y F, Wang L, Yu Y Z, Wang X G 2018 Front. Phys. 13 130505
 Google Scholar
Google Scholar
[75] Wang R, Lin P, Liu M, Wu Y, Zhou T, Zhou C 2019 Phys. Rev. Lett. 123 038301
 Google Scholar
Google Scholar
计量
- 文章访问数: 16081
- PDF下载量: 542
- 被引次数: 0





















 下载:
下载: