典型磁性材料价电子结构研究面临的机遇与挑战

唐贵德; 李壮志; 马丽; 吴光恒; 胡凤霞

doi:10.7498/aps.69.20191655

摘要

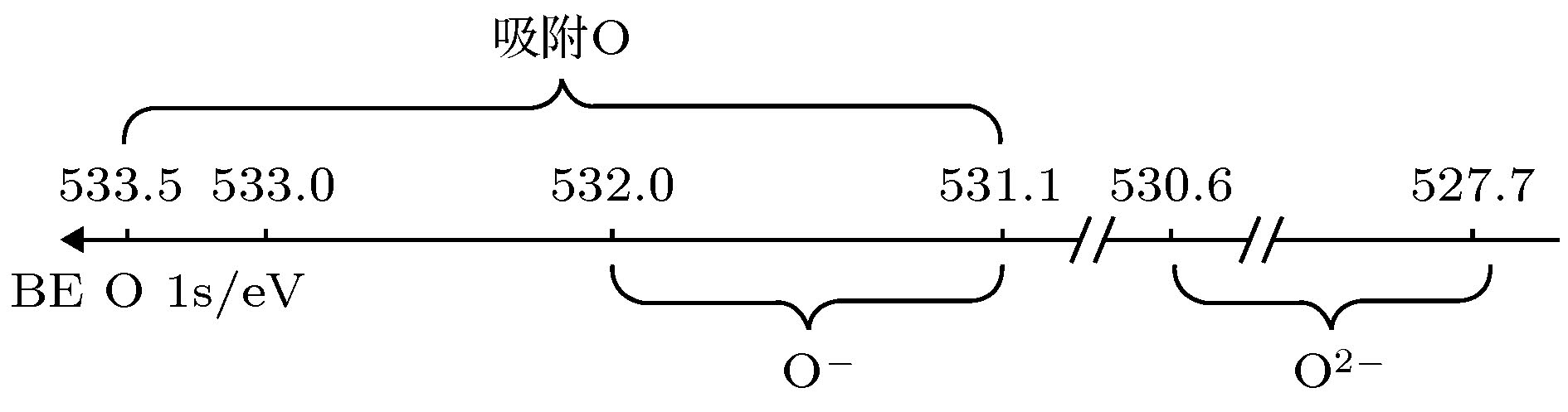
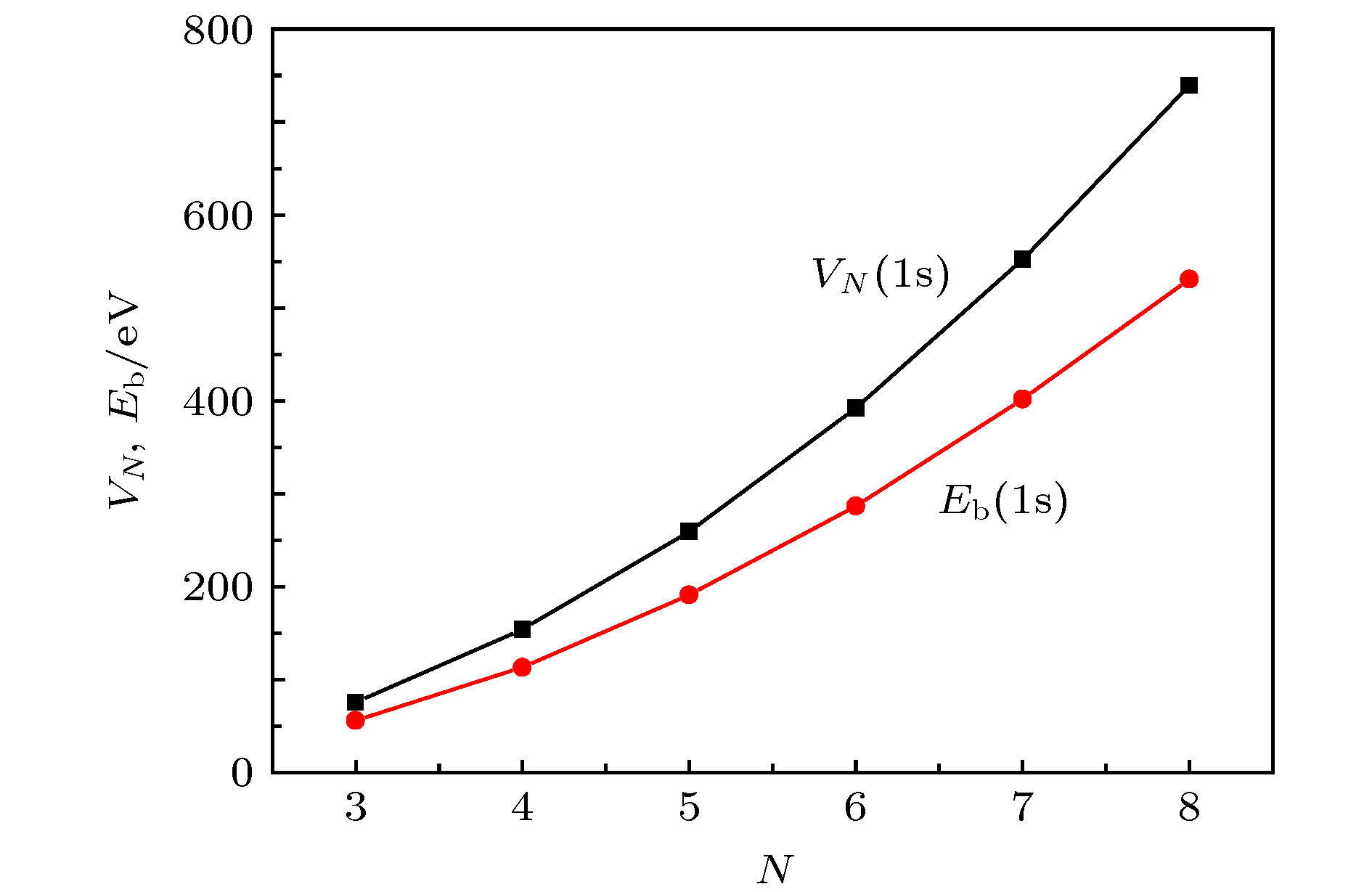
目前在磁性材料磁有序现象研究中广泛使用的交换作用、超交换作用和双交换作用模型形成于1950年代及其以前, 这些模型都涉及材料中的价电子状态, 但那时还没有充分的价电子状态实验依据. 1970年代以来, 有关价电子结构实验结果的报道越来越多, 这些实验结果表明传统的磁有序模型需要改进. 首先, 大量电子谱实验表明, 在氧化物中除存在负二价氧离子之外, 还存在负一价氧离子, 并且负一价氧离子的含量可达30%或更多. 这说明以所有氧离子都是负二价离子为基本假设的超交换和双交换作用模型需要改进. 其次, 一些实验证明, 铁、钴、镍自由原子的一部分4s电子在形成铁磁性金属的过程中变成了3d电子, 这为探讨金属磁性与电输运性质的关系提供了依据. 此外, 即使在现代的密度泛函计算中, 仍不能给出磁性交换作用能的函数表达式, 只能采取各种不同模型进行模拟计算, 从而使磁性材料的模拟计算遇到严重困难. 寻求一个磁有序能的函数表达式可能是解决这个困难的途径. 这些研究表明磁性材料价电子结构研究面临着重大的机遇与挑战. 本文首先介绍一些典型的实验例证, 然后介绍了基于这些实验结果的一套典型磁性材料的磁有序新模型, 随后介绍了基于新模型的磁性材料价电子结构与旧模型的主要区别, 最后指出了未来研究工作面临的挑战.

关键词:

Abstract

The conventional magnetic ordering models, exchange interaction, super-exchange (SE) interaction and double exchange (DE) interaction models relating to the valence electron structure in the materials, were proposed about in or before the 1950's, the time when there was little experimental evidence. Since the 1970's, more and more experimental results for the valence electron states have been reported. These experimental results suggested that the conventional magnetic ordering models need improving. i) Many experimental results, including the electron energy-loss spectra (EELS), X-ray absorption spectra (XAS), and X-ray photoelectron spectra (XPS), indicate that there are O^– anions in addition to O^2– anions in oxides, and that the percentage of O^– anions may reach 30% or more. This suggests that the SE model and DE model both need to improving, in which all oxygen anions are assumed to be O^2– anions. ii) Several experimental results, including gamma radiation diffraction, XAS and magnetic circular dichroism spectra (XMCD), suggest that part of 4s electrons enter into 3d orbits and transit into the 3d electrons in the process of forming metals from free atoms. The effect of the orbital magnetic moment on the magnetic moment of a bulk metal is far smaller than the spin magnetic moments. These provide the evidence of exploring the relation between magnetic moment and electrical resistivity of the magnetic metal. iii) Using density function theory (DFT) to fit physical properties yields plenty of results for many materials, but there exist serious difficulties for magnetic materials. This is due to magnetic ordering energy is included in the exchange correlation energy, which has been find no phenomenological expression so far, and has to be fitted using various models in DFT calculation. These investigations provide an opportunity to improve magnetic ordering models. Therefore, our group proposed three models of magnetic ordering in typical magnetic materials, they including an O 2p itinerant electron model for magnetic oxides (IEO model), a new itinerant electron model for magnetic metal (IEM model), and a Weiss electron pair (WEP) model for the origin of magnetic ordering energy. Replacing the SE model and DE model with the IEO model, the magnetic structures of Co, Ni, Cu doped spinel ferrites as well as Cr and Ti doped spinel ferrites can be explained. The dependence of the magnetic moment on the Sr content in perovskite manganites La_1–xSr_xMnO₃ can also be explained, for which there have been many ongoing disputes about the cation distributions. With the IEM model, we can explain qualitatively the relation of the magnetic moment with the resitivity for each of Fe, Co, Ni, Cu metals, and fit the curves of the resistivity of NiCu alloy versus test temperature and the Cu doped level. With the WEP model, we can explain why Fe, Co, Ni metal, NiCu alloys, Fe₃O₄ and La_0.7Sr_0.3MnO₃ oxides have different Curie temperature values. The new itinerant electron model is different from the classical model in the following three elementary characteristics. First, the s electrons in free 3d transition metal atoms are divided into two parts when they form a metal or alloy. One part of these s electrons enter into the d orbits and change into the d electrons. and the other part of those electrons are the free electrons which are no longer called the s electrons. Second, only the d electrons occupying the outer orbit of an ion core in a metal or alloy may form itinerant electrons with a certain probability, while the remaining d electrons are local electrons. Third, whether in a magnetic metal or in a magnetic oxide, the transition of the itinerant electrons is the spin-dependent transition below the Curie temperature, and the transition probability decreases with test temperature increasing. The transition of the itinerant electrons turns into the spin-independent transition when the temperature is above the Curie temperature. In this paper, first, we introduce several typical experimental results of the valence electron states. Then, we present the new magnetic ordering models proposed by our group and analyze the elementary differences between the new models and the conventional models. Finally, we point out the challenge to the future work.

Keywords:

作者及机构信息

1.
中国科学院物理研究所, 磁学国家重点实验室, 北京　100190

2.
河北师范大学物理学院, 河北省新型薄膜材料实验室, 石家庄　050024

通信作者: 唐贵德, tanggd@hebtu.edu.cn

基金项目: 国家自然科学基金(批准号: 11174069)和河北省自然科学基金(批准号: E2015205111)资助的课题

Authors and contacts

1.
State Key Laboratory of Magnetism, Institute of Physics, Chinese Academy of Sciences, Beijing 100190, China

2.
Hebei Advanced Thin Film Laboratory, College of Physics and Information Engineering, Hebei Normal University, Shijiazhuang 050024, China

Corresponding author: Tang Gui-De, tanggd@hebtu.edu.cn

Funds: Project supported by the National Natural Science Foundation of China (Grant No. 11174069) and the Natural Science Foundation of Hebei Province, China (Grant No. E2015205111)

文章全文 : translate this paragraph

参考文献

[1]	戴道生, 钱昆明 1987 铁磁学 (上册) (北京: 科学出版社) 第103−122, 198, 323页 Dai D S, Qian K M 1987 Ferromagnetism (Vol. 1) (Beijing: Science Press) pp103−122, 198, 323 (in Chinese)
[2]	van Vleck J H 1937 Phys. Rev. 52 1178 Google Scholar
[3]	Sato H, Arrott A 1959 Phys. Rev. 114 1427 Google Scholar
[4]	Anderson P W 1950 Phys. Rev. 79 350 Google Scholar
[5]	Zener C 1951 Phys. Rev. 82 403 Google Scholar
[6]	Tang G D, Li Z Z, Ma L, Qi W H, Wu L Q, Ge X S, Wu G H, Hu F X 2018 Phys. Rep. 758 1 Google Scholar
[7]	Li Z Z, Qi W H, Ma L, Tang G D, Wu G H, Hu F X 2019 J. Magn. Magn. Mater. 482 173 Google Scholar
[8]	Qian J J, Qi W H, Li Z Z, Ma L, Tang G D, Du Y N, Chen M Y, Wu G H, Hu F X 2018 RSC Adv. 8 4417 Google Scholar
[9]	Qian J J, Li Z Z, Qi W H, Ma L, Tang G D, Du Y N, Chen M Y 2018 J. Alloys Compd. 764 239 Google Scholar
[10]	Nücker N, Fink J, Fuggle J C, Durham P J 1988 Phys. Rev. B 37 5158 Google Scholar
[11]	Ju H L, Sohn H C, Krishnan K M 1997 Phys. Rev. Lett. 79 3230 Google Scholar
[12]	Urushibara A, Moritomo Y, Arima T, Asamitsu A, Kido G, Tokura Y 1995 Phys. Rev. B 51 14103 Google Scholar
[13]	Mizoroki T, Itou M, Taguchi Y, Iwazumi T, Sakurai Y 2011 Appl. Phys. Lett. 98 052107 Google Scholar
[14]	Grenier S, Thomas K J, Hill J P, Staub U, Bodenthin Y, García-Fernández M, Scagnoli V, Kiryukhin V, Cheong S W, Kim B G, Tonnerre J M 2007 Phys. Rev. Lett. 99 206403 Google Scholar
[15]	Ibrahim K, Qian H J, Wu X, Abbas M I, Wang J O, Hong C H, Su R, Zhong J, Dong Y H, Wu Z Y, Wei L, Xian D C, Li Y X, Lapeyre G J, Mannella N, Fadley C S, Baba Y 2004 Phys. Rev. B 70 224433 Google Scholar
[16]	Papavassiliou G, Pissas M, Belesi M, Fardis M, Karayanni M, Ansermet J P, Carlier D, Dimitropoulos C, Dolinsek J 2004 Europhys. Lett. 68 453 Google Scholar
[17]	Dupin J C, Gonbeau D, Vinatier P, Levasseur A 2000 Phys. Chem. Chem. Phys. 2 1319 Google Scholar
[18]	Cohen R E 1992 Nature 358 136 Google Scholar
[19]	Cohen R E, Krakauer H 1990 Phys. Rev. B 42 6416 Google Scholar
[20]	Wu L Q, Li Y C, Li S Q, Li Z Z, Tang G D, Qi W H, Xue L C, Ge X S, Ding L L 2015 AIP Adv. 5 097210 Google Scholar
[21]	Wu L Q, Li S Q, Li Y C, Li Z Z, Tang G D, Qi W H, Xue L C, Ding L L, Ge X S 2016 Appl. Phys. Lett. 108 021905 Google Scholar
[22]	Chen C T, Idzerda Y U, Lin H J, Smith N V, Meigs G, Chaban E, Ho G H, Pellegrin E, Sette F 1995 Phys. Rev. Lett. 75 152 Google Scholar
[23]	Wu R, Wang D, Freeman A J 1993 Phys. Rev. Lett. 71 3581 Google Scholar
[24]	Wu R, Freeman A J 1994 Phys. Rev. Lett. 73 1994 Google Scholar
[25]	Jauch W, Reehuis M 2007 Phys. Rev. B 76 235121 Google Scholar
[26]	Pacchioni G E, Gragnaniello L, Donati F, Pivetta M, Autès G, Yazyev O V, Rusponi S, Brune H 2015 Phys. Rev. B 91 235426 Google Scholar
[27]	韩汝珊 1998 高温超导物理 (北京: 北京大学出版社) 第19−20 页 Han R S 1998 Physics of High Temperature Super-conductor (Beijing: Peking University Press) pp19−20 (in Chinese)
[28]	Alexandrov A S, Bratkovsky A M, Kabanov V V 2006 Phys. Rev. Lett. 96 117003 Google Scholar
[29]	Alexandrov A S, Bratkovsky A M 1999 Phys. Rev. Lett. 82 141 Google Scholar
[30]	Xu J, Ma L, Li Z Z, Lang L L, Qi W H, Tang G D, Wu L Q, Xue L C, Wu G H 2015 Phys. Status Solidi B 252 2820 Google Scholar
[31]	武力乾, 齐伟华, 李雨辰, 李世强, 李壮志, 唐贵德, 薛立超, 葛兴烁, 丁丽莉 2016 65 027501 Google Scholar Wu L Q, Qi W H, Li Y C, Li S Q, Li Z Z, Tang G D, Xue L C, Ge X S, Ding L L 2016 Acta Phys. Sin. 65 027501 Google Scholar
[32]	齐伟华, 马丽, 李壮志, 唐贵德, 吴光恒 2017 66 027101 Google Scholar Qi W H, Ma L, Li Z Z, Tang G D, Wu G H 2017 Acta Phys. Sin. 66 027101 Google Scholar
[33]	齐伟华, 李壮志, 马丽, 唐贵德, 吴光恒, 胡凤霞 2017 66 067501 Google Scholar Qi W H, Li Z Z, Ma L, Tang G D, Wu G H, Hu F X 2017 Acta Phys. Sin. 66 067501 Google Scholar
[34]	Tang G D, Han Q J, Xu J, Ji D H, Qi W H, Li Z Z, Shang Z F, Zhang X Y 2014 Physica B 438 91 Google Scholar
[35]	Shang Z F, Qi W H, Ji D H, Xu J, Tang G D, Zhang X Y, Li Z Z, Lang L L 2014 Chin. Phys. B 23 107503 Google Scholar
[36]	Lang L L, Xu J, Qi W H, Li Z Z, Tang G D, Shang Z F, Zhang X Y, Wu L Q, Xue L C 2014 J. Appl. Phys. 116 123901 Google Scholar
[37]	Lang L L, Xu J, Li Z Z, Qi W H, Tang G D, Shang Z F, Zhang X Y, Wu L Q, Xue L C 2015 Physica B 462 47 Google Scholar
[38]	Xue L C, Lang L L, Xu J, Li Z Z, Qi W H, Tang G D, Wu L Q 2015 AIP Adv. 5 097167 Google Scholar
[39]	Zhang X Y, Xu J, Li Z Z, Qi W H, Tang G D, Shang Z F, Ji D H, Lang L L 2014 Physica B 446 92 Google Scholar
[40]	Tang G D, Shang Z F, Zhang X Y, Xu J, Li Z Z, Zhen C M, Qi W H, Lang L L 2015 Physica B 463 26 Google Scholar
[41]	Xu J, Ji D H, Li Z Z, Qi W H, Tang G D, Zhang X Y, Shang Z F, Lang L L 2015 Phys. Status Solidi B 252 411 Google Scholar
[42]	徐静, 齐伟华, 纪登辉, 李壮志, 唐贵德, 张晓云, 尚志丰, 郎莉莉 2015 64 017501 Google Scholar Xu J, Qi W H, Ji D H, Li Z Z, Tang G D, Zhang X Y, Shang Z F, Lang L L 2015 Acta Phys. Sin. 64 017501 Google Scholar
[43]	Ding L L, Xue L C, Li Z Z, Li S Q, Tang G D, Qi W H, Wu L Q, Ge X S 2016 AIP Adv. 6 105012 Google Scholar
[44]	Du Y N, Xu J, Li Z.Z, Tang G D, Qian J J, Chen M Y, Qi W H 2018 RSC Adv. 8 302 Google Scholar
[45]	Chen M Y, Xu J, Li Z Z, Zhang Y, Qi W H, Tang G D 2019 Results Phys. 14 102389 Google Scholar
[46]	Wu L Q, Qi W H, Ge X S, Ji D H, Li Z Z, Tang G D, Zhong W 2017 Europhys. Lett. 120 27001 Google Scholar
[47]	Ge X S, Wu L Q, Li S Q, Li Z Z, Tang G D, Qi W H, Zhou H J, Xue L C, Ding L L 2017 AIP Adv. 7 045302 Google Scholar
[48]	Ge X S, Li Z Z, Qi W H, Ji D H, Tang G D, Ding L L, Qian J J, Du Y N 2017 AIP Adv. 7 125002 Google Scholar
[49]	Li S Q, Wu L Q, Qi W H, Ge X S, Li Z Z, Tang G D, Zhong W 2018 J. Magn. Magn. Mater. 460 501 Google Scholar
[50]	Qi W H, Li Z Z, Ma L, Tang G D, Wu G H 2018 AIP Adv. 8 065105 Google Scholar
[51]	谢希德, 陆栋 1998 固体能带理论(上海: 复旦大学出版社) 第11页 Xie X D, Lu D 1998 Energy Band Theory of Solids (Shanghai: Fudan University Press) p11 (in Chinese)
[52]	廖立兵 2000 晶体化学及晶体物理学 (北京: 地质出版社) 第161页 Liao L B 2000 Crystal Chemistry and Crystal Physics (Beijing: Geological Publishing House) p161 (in Chinese)
[53]	Wagner C D, Davis W M, Moulder J F, Muilenberg G E 1979 Handbook of X-ray Photoelectron Spectroscopy (Eden Prairie, Minnesota, USA: Perkin-Elmer coporation) pp182–182
[54]	Ley L, Dabbousi O B, Kowalczyk S P, McFeely F R, Shiley D A 1977 Phys. Rew. B 16 5372 Google Scholar
[55]	Ding L L, Wu L Q, Ge X S, Du Y N, Qian J J, Tang G D, Zhong W 2018 Results Phys. 9 866 Google Scholar
[56]	Salamon M B, Jaime M 2001 Rev. Moder. Phys. 73 583 Google Scholar
[57]	Dagotto E, Hotta T, Moreo A 2001 Phys. Rep. 344 1 Google Scholar
[58]	Tokura Y, Tomioka Y 1999 J. Magn. Magn. Mater. 200 1 Google Scholar
[59]	方俊鑫, 陆栋 1980 固体物理学(上册) (上海: 上海科学技术出版社) 第314页 Fang J X, Lu D 1980 Solid State Physics (Shanghai: Shanghai Scientific & Technical Publishers) p314 (in Chinese)

施引文献

图 1 由Ju等^[11]报道的La_1–xSr_xMnO₃系列样品　(a)电阻率ρ随测试温度T的变化关系; (b)电子能量损失谱

Fig. 1. La_1–xSr_xMnO₃ reported by Ju et al.^[11]: (a) Curves of the resistivity ρ versus the test temperature T; (b) electron energy loss spectra.

下载: 全尺寸图片幻灯片

图 2 Dupin等^[17]提出的O 1s谱峰所对应的氧离子价态示意图

Fig. 2. A binding energy scale for valence state of oxygen at the O 1s peaks, proposed by Dupin et al. ^[17]

下载: 全尺寸图片幻灯片

图 3 近邻离子外层电子轨道的(a)外斯电子对和(b), (c)巡游电子示意图^[33]

Fig. 3. Illustrations of (a) a Weiss electron pair and (b) and (c) itinerant electrons in the outer orbits of adjacent ions^[33].

下载: 全尺寸图片幻灯片

图 4 晶体中离子对1s电子的束缚能E_b和自由原子中1s电子的电离能V_N随原子序数N的变化^[52,53]

Fig. 4. Dependences on the atom number (N) of the binding energy (E_b) of 1 s electron in a crystal and the ionization energy (V_N) of 1 s electron in an free atom^[52,53]

下载: 全尺寸图片幻灯片

图 5 CaO, ZnO, MnFe₂O₄, ZnFe₂O₄的价带光电子谱^[55]

Fig. 5. Valence band photoelectron spectra of samples CaO, ZnO, MnFe₂O₄ and ZnFe₂O₄^[55]

下载: 全尺寸图片幻灯片

map

[1]	戴道生, 钱昆明 1987 铁磁学 (上册) (北京: 科学出版社) 第103−122, 198, 323页 Dai D S, Qian K M 1987 Ferromagnetism (Vol. 1) (Beijing: Science Press) pp103−122, 198, 323 (in Chinese)
[2]	van Vleck J H 1937 Phys. Rev. 52 1178 Google Scholar
[3]	Sato H, Arrott A 1959 Phys. Rev. 114 1427 Google Scholar
[4]	Anderson P W 1950 Phys. Rev. 79 350 Google Scholar
[5]	Zener C 1951 Phys. Rev. 82 403 Google Scholar
[6]	Tang G D, Li Z Z, Ma L, Qi W H, Wu L Q, Ge X S, Wu G H, Hu F X 2018 Phys. Rep. 758 1 Google Scholar
[7]	Li Z Z, Qi W H, Ma L, Tang G D, Wu G H, Hu F X 2019 J. Magn. Magn. Mater. 482 173 Google Scholar
[8]	Qian J J, Qi W H, Li Z Z, Ma L, Tang G D, Du Y N, Chen M Y, Wu G H, Hu F X 2018 RSC Adv. 8 4417 Google Scholar
[9]	Qian J J, Li Z Z, Qi W H, Ma L, Tang G D, Du Y N, Chen M Y 2018 J. Alloys Compd. 764 239 Google Scholar
[10]	Nücker N, Fink J, Fuggle J C, Durham P J 1988 Phys. Rev. B 37 5158 Google Scholar
[11]	Ju H L, Sohn H C, Krishnan K M 1997 Phys. Rev. Lett. 79 3230 Google Scholar
[12]	Urushibara A, Moritomo Y, Arima T, Asamitsu A, Kido G, Tokura Y 1995 Phys. Rev. B 51 14103 Google Scholar
[13]	Mizoroki T, Itou M, Taguchi Y, Iwazumi T, Sakurai Y 2011 Appl. Phys. Lett. 98 052107 Google Scholar
[14]	Grenier S, Thomas K J, Hill J P, Staub U, Bodenthin Y, García-Fernández M, Scagnoli V, Kiryukhin V, Cheong S W, Kim B G, Tonnerre J M 2007 Phys. Rev. Lett. 99 206403 Google Scholar
[15]	Ibrahim K, Qian H J, Wu X, Abbas M I, Wang J O, Hong C H, Su R, Zhong J, Dong Y H, Wu Z Y, Wei L, Xian D C, Li Y X, Lapeyre G J, Mannella N, Fadley C S, Baba Y 2004 Phys. Rev. B 70 224433 Google Scholar
[16]	Papavassiliou G, Pissas M, Belesi M, Fardis M, Karayanni M, Ansermet J P, Carlier D, Dimitropoulos C, Dolinsek J 2004 Europhys. Lett. 68 453 Google Scholar
[17]	Dupin J C, Gonbeau D, Vinatier P, Levasseur A 2000 Phys. Chem. Chem. Phys. 2 1319 Google Scholar
[18]	Cohen R E 1992 Nature 358 136 Google Scholar
[19]	Cohen R E, Krakauer H 1990 Phys. Rev. B 42 6416 Google Scholar
[20]	Wu L Q, Li Y C, Li S Q, Li Z Z, Tang G D, Qi W H, Xue L C, Ge X S, Ding L L 2015 AIP Adv. 5 097210 Google Scholar
[21]	Wu L Q, Li S Q, Li Y C, Li Z Z, Tang G D, Qi W H, Xue L C, Ding L L, Ge X S 2016 Appl. Phys. Lett. 108 021905 Google Scholar
[22]	Chen C T, Idzerda Y U, Lin H J, Smith N V, Meigs G, Chaban E, Ho G H, Pellegrin E, Sette F 1995 Phys. Rev. Lett. 75 152 Google Scholar
[23]	Wu R, Wang D, Freeman A J 1993 Phys. Rev. Lett. 71 3581 Google Scholar
[24]	Wu R, Freeman A J 1994 Phys. Rev. Lett. 73 1994 Google Scholar
[25]	Jauch W, Reehuis M 2007 Phys. Rev. B 76 235121 Google Scholar
[26]	Pacchioni G E, Gragnaniello L, Donati F, Pivetta M, Autès G, Yazyev O V, Rusponi S, Brune H 2015 Phys. Rev. B 91 235426 Google Scholar
[27]	韩汝珊 1998 高温超导物理 (北京: 北京大学出版社) 第19−20 页 Han R S 1998 Physics of High Temperature Super-conductor (Beijing: Peking University Press) pp19−20 (in Chinese)
[28]	Alexandrov A S, Bratkovsky A M, Kabanov V V 2006 Phys. Rev. Lett. 96 117003 Google Scholar
[29]	Alexandrov A S, Bratkovsky A M 1999 Phys. Rev. Lett. 82 141 Google Scholar
[30]	Xu J, Ma L, Li Z Z, Lang L L, Qi W H, Tang G D, Wu L Q, Xue L C, Wu G H 2015 Phys. Status Solidi B 252 2820 Google Scholar
[31]	武力乾, 齐伟华, 李雨辰, 李世强, 李壮志, 唐贵德, 薛立超, 葛兴烁, 丁丽莉 2016 65 027501 Google Scholar Wu L Q, Qi W H, Li Y C, Li S Q, Li Z Z, Tang G D, Xue L C, Ge X S, Ding L L 2016 Acta Phys. Sin. 65 027501 Google Scholar
[32]	齐伟华, 马丽, 李壮志, 唐贵德, 吴光恒 2017 66 027101 Google Scholar Qi W H, Ma L, Li Z Z, Tang G D, Wu G H 2017 Acta Phys. Sin. 66 027101 Google Scholar
[33]	齐伟华, 李壮志, 马丽, 唐贵德, 吴光恒, 胡凤霞 2017 66 067501 Google Scholar Qi W H, Li Z Z, Ma L, Tang G D, Wu G H, Hu F X 2017 Acta Phys. Sin. 66 067501 Google Scholar
[34]	Tang G D, Han Q J, Xu J, Ji D H, Qi W H, Li Z Z, Shang Z F, Zhang X Y 2014 Physica B 438 91 Google Scholar
[35]	Shang Z F, Qi W H, Ji D H, Xu J, Tang G D, Zhang X Y, Li Z Z, Lang L L 2014 Chin. Phys. B 23 107503 Google Scholar
[36]	Lang L L, Xu J, Qi W H, Li Z Z, Tang G D, Shang Z F, Zhang X Y, Wu L Q, Xue L C 2014 J. Appl. Phys. 116 123901 Google Scholar
[37]	Lang L L, Xu J, Li Z Z, Qi W H, Tang G D, Shang Z F, Zhang X Y, Wu L Q, Xue L C 2015 Physica B 462 47 Google Scholar
[38]	Xue L C, Lang L L, Xu J, Li Z Z, Qi W H, Tang G D, Wu L Q 2015 AIP Adv. 5 097167 Google Scholar
[39]	Zhang X Y, Xu J, Li Z Z, Qi W H, Tang G D, Shang Z F, Ji D H, Lang L L 2014 Physica B 446 92 Google Scholar
[40]	Tang G D, Shang Z F, Zhang X Y, Xu J, Li Z Z, Zhen C M, Qi W H, Lang L L 2015 Physica B 463 26 Google Scholar
[41]	Xu J, Ji D H, Li Z Z, Qi W H, Tang G D, Zhang X Y, Shang Z F, Lang L L 2015 Phys. Status Solidi B 252 411 Google Scholar
[42]	徐静, 齐伟华, 纪登辉, 李壮志, 唐贵德, 张晓云, 尚志丰, 郎莉莉 2015 64 017501 Google Scholar Xu J, Qi W H, Ji D H, Li Z Z, Tang G D, Zhang X Y, Shang Z F, Lang L L 2015 Acta Phys. Sin. 64 017501 Google Scholar
[43]	Ding L L, Xue L C, Li Z Z, Li S Q, Tang G D, Qi W H, Wu L Q, Ge X S 2016 AIP Adv. 6 105012 Google Scholar
[44]	Du Y N, Xu J, Li Z.Z, Tang G D, Qian J J, Chen M Y, Qi W H 2018 RSC Adv. 8 302 Google Scholar
[45]	Chen M Y, Xu J, Li Z Z, Zhang Y, Qi W H, Tang G D 2019 Results Phys. 14 102389 Google Scholar
[46]	Wu L Q, Qi W H, Ge X S, Ji D H, Li Z Z, Tang G D, Zhong W 2017 Europhys. Lett. 120 27001 Google Scholar
[47]	Ge X S, Wu L Q, Li S Q, Li Z Z, Tang G D, Qi W H, Zhou H J, Xue L C, Ding L L 2017 AIP Adv. 7 045302 Google Scholar
[48]	Ge X S, Li Z Z, Qi W H, Ji D H, Tang G D, Ding L L, Qian J J, Du Y N 2017 AIP Adv. 7 125002 Google Scholar
[49]	Li S Q, Wu L Q, Qi W H, Ge X S, Li Z Z, Tang G D, Zhong W 2018 J. Magn. Magn. Mater. 460 501 Google Scholar
[50]	Qi W H, Li Z Z, Ma L, Tang G D, Wu G H 2018 AIP Adv. 8 065105 Google Scholar
[51]	谢希德, 陆栋 1998 固体能带理论(上海: 复旦大学出版社) 第11页 Xie X D, Lu D 1998 Energy Band Theory of Solids (Shanghai: Fudan University Press) p11 (in Chinese)
[52]	廖立兵 2000 晶体化学及晶体物理学 (北京: 地质出版社) 第161页 Liao L B 2000 Crystal Chemistry and Crystal Physics (Beijing: Geological Publishing House) p161 (in Chinese)
[53]	Wagner C D, Davis W M, Moulder J F, Muilenberg G E 1979 Handbook of X-ray Photoelectron Spectroscopy (Eden Prairie, Minnesota, USA: Perkin-Elmer coporation) pp182–182
[54]	Ley L, Dabbousi O B, Kowalczyk S P, McFeely F R, Shiley D A 1977 Phys. Rew. B 16 5372 Google Scholar
[55]	Ding L L, Wu L Q, Ge X S, Du Y N, Qian J J, Tang G D, Zhong W 2018 Results Phys. 9 866 Google Scholar
[56]	Salamon M B, Jaime M 2001 Rev. Moder. Phys. 73 583 Google Scholar
[57]	Dagotto E, Hotta T, Moreo A 2001 Phys. Rep. 344 1 Google Scholar
[58]	Tokura Y, Tomioka Y 1999 J. Magn. Magn. Mater. 200 1 Google Scholar
[59]	方俊鑫, 陆栋 1980 固体物理学(上册) (上海: 上海科学技术出版社) 第314页 Fang J X, Lu D 1980 Solid State Physics (Shanghai: Shanghai Scientific & Technical Publishers) p314 (in Chinese)

[1]	王艺琳, 兰自轩, 杜汇伟, 赵磊, 马忠权. 重掺杂多晶硅薄膜中磷氧化物的探究. , 2022, 71(18): 188201. doi: 10.7498/aps.71.20220706
[2]	任县利, 张伟伟, 伍晓勇, 吴璐, 王月霞. 高熵合金短程有序现象的预测及其对结构的电子、磁性、力学性质的影响. , 2020, 69(4): 046102. doi: 10.7498/aps.69.20191671
[3]	邓韬, 杨海峰, 张敬, 李一苇, 杨乐仙, 柳仲楷, 陈宇林. 拓扑半金属材料角分辨光电子能谱研究进展. , 2019, 68(22): 227102. doi: 10.7498/aps.68.20191544
[4]	刘畅, 刘祥瑞. 强三维拓扑绝缘体与磁性拓扑绝缘体的角分辨光电子能谱学研究进展. , 2019, 68(22): 227901. doi: 10.7498/aps.68.20191450
[5]	杨蒙生, 易泰民, 郑凤成, 唐永建, 张林, 杜凯, 李宁, 赵利平, 柯博, 邢丕峰. 沉积态铀薄膜表面氧化的X射线光电子能谱. , 2018, 67(2): 027301. doi: 10.7498/aps.67.20172055
[6]	郭晶, 郭福明, 陈基根, 杨玉军. 高频激光脉宽对原子光电子发射谱的影响. , 2018, 67(7): 073202. doi: 10.7498/aps.67.20172440
[7]	杨明宇, 杨倩, 张勃, 张旭, 蔡颂, 薛玉龙, 周铁戈. 5d过渡金属原子掺杂六方氮化铝单层的磁性及自旋轨道耦合效应:可能存在的二维长程磁有序. , 2017, 66(6): 063102. doi: 10.7498/aps.66.063102
[8]	齐伟华, 李壮志, 马丽, 唐贵德, 吴光恒, 胡凤霞. 磁性材料磁有序的分子场来源. , 2017, 66(6): 067501. doi: 10.7498/aps.66.067501
[9]	崔鑫, 李苏宇, 郭福明, 田原野, 陈基根, 曾思良, 杨玉军. 高频激光脉冲作用下原子的光子和光电子发射. , 2015, 64(4): 043201. doi: 10.7498/aps.64.043201
[10]	胡妮, 刘雍, 汤五丰, 裴玲, 方鹏飞, 熊锐, 石兢. La0.4Ca0.6MnO3中Mn-位Fe和Cr掺杂对磁性质的影响. , 2014, 63(23): 237502. doi: 10.7498/aps.63.237502
[11]	羊新胜, 赵勇. 铁磁性锰氧化物掺杂的ZnO压敏电阻性能研究. , 2008, 57(5): 3188-3192. doi: 10.7498/aps.57.3188
[12]	冯玉清, 赵昆, 朱涛, 詹文山. 磁性隧道结热稳定性的x射线光电子能谱研究. , 2005, 54(11): 5372-5376. doi: 10.7498/aps.54.5372
[13]	杨海涛, 申承民, 杜世萱, 苏轶坤, 王岩国, 汪裕萍, 高鸿钧. 钴纳米粒子自组装有序阵列与磁性. , 2003, 52(12): 3114-3119. doi: 10.7498/aps.52.3114
[14]	覃东欢, 彭勇, 王成伟, 力虎林. Co-Ni合金纳米线有序阵列的制备与磁性研究. , 2001, 50(1): 144-148. doi: 10.7498/aps.50.144
[15]	肖定全, 韦力凡, 李子森, 朱建国, 钱正洪, 彭文斌. 金属氧化物薄膜的多离子束反应共溅射模型(Ⅰ)——模型建立. , 1996, 45(2): 330-338. doi: 10.7498/aps.45.330
[16]	张训生, 范朝阳, 隋华, 鲍世宁, 徐亚伯, 徐世红, 潘海斌, 徐彭寿. Na在Si(111)表面(3×1)有序吸附结构的光电子能谱研究. , 1996, 45(7): 1244-1248. doi: 10.7498/aps.45.1244
[17]	虞心南. Cu-Zr合金在氢气氛中退火后表面Cu沉积物的光电子能谱研究. , 1991, 40(9): 1501-1504. doi: 10.7498/aps.40.1501
[18]	谢侃, 齐上雪, 伍乃娟, 林彰达. 蓝色氧化钨的光电子能谱研究. , 1990, 39(5): 809-813. doi: 10.7498/aps.39.809
[19]	赵良仲. Ce(Ⅳ)和Ce(Ⅲ)化合物系列的X射线光电子能谱研究. , 1989, 38(6): 987-990. doi: 10.7498/aps.38.987
[20]	潘士宏, 莫党, 秦关根, W. E. SPICER. 贵金属—GaAs(110)界面价带紫外光电子谱. , 1987, 36(10): 1255-1263. doi: 10.7498/aps.36.1255

计量

文章访问数: 9101
PDF下载量: 241
被引次数: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

搜索

留言板

典型磁性材料价电子结构研究面临的机遇与挑战