-
实际安全性是目前量子密钥分发系统中最大的挑战. 在实际实现中, 接收单元的单光子探测器在雪崩过程的二次光子发射(反向荧光)会导致信息泄露. 目前, 已有研究表明该反向荧光会泄露时间和偏振信息并且窃听行为不会在通信过程中产生额外误码率, 在自由空间量子密钥分发系统中提出了利用反向荧光获取偏振信息的攻击方案, 但是在光纤量子密钥分发系统中暂未见报道. 本文提出了在光纤偏振编码量子密钥分发系统中利用反向荧光获取信息的窃听方案与减少信息泄露的解决方法, 在时分复用偏振补偿的光纤偏振编码量子密钥分发系统的基础上对该方案中窃听者如何获取密钥信息进行了理论分析. 实验上测量了光纤偏振编码量子密钥分发系统中反向荧光的概率为0.05, 并对本文提出的窃听方案中的信息泄露进行量化, 得出窃听者获取密钥信息的下限为2.5 × 10–4.Nowadays, the practical security of quantum key distribution (QKD) is the biggest challenge. In practical implementation, the security of a practical system strongly depends on its device implementation, and device defects will create security holes. The information leakage from a receiving unit due to secondary photon emission (backflash) is caused by a single-photon detector in the avalanche process. Now studies have shown that the backflash will leak the information about time and polarization and the eavesdropping behavior will not generate additional error rate in the communication process. An eavesdropping scheme obtaining time information by using backflash is proposed. Targeting this security hole for backflash leaking polarization information, an eavesdropping scheme for obtaining polarization information by using backflash is proposed in free-space QKD; however, it has not been reported in fiber QKD. In this study, the eavesdropping scheme and countermeasures for obtaining information by using backflash in fiber polarization-coded QKD is proposed. Since the polarization state of the fiber polarization-coded QKD system is easy to change, the scheme is proposed based on the time-division multiplexing polarization compensation fiber polarization-coded QKD system. In theory, the eavesdropper in this scheme obtaining the key information by using the backflash is theoretically deduced, and corrects the polarization change of the backflash by time-division multiplexing polarization compensation method, thus obtaining the accurate polarization information. The probability of backflash in the fiber polarization-coded QKD is measured to be 0.05, and the information leakage in the proposed eavesdropping scheme is quantified. The lower limit of the information obtained by the eavesdropper is 2.5 × 10–4. Due to the fact that the polarization compensation process increases invalid information in actual operation, the information obtained by the eavesdropper will be further reduced, thus obtaining the lower limit of information leakage. The results show that the backflash leaks a small amount of key information in a time-multiplexed polarization-compensated fiber polarization-coded QKD system. The wavelength characteristics of the backflash can be utilized to take corresponding defense methods. Backflash has a wide spectral range, and the count of backflash has a peak wavelength. So, tunable filters and isolators can be used to reduce backflash leakage, thereby reducing the information leakage.
-
Keywords:
- quantum key distribution /
- polarization-coded /
- time division multiplexing polarization compensation /
- backflash
[1] Gisin N, Ribordy G, Tittel W, Zbinden H 2002 Rev. Mod. Phys. 74 145
Google Scholar
[2] Xu G, Chen X B, Dou Z, Yang Y X, Li Z 2015 Quantum Inf. Process 14 2959
Google Scholar
[3] 岳孝林, 王金东, 魏正军, 郭邦红, 刘颂豪 2012 61 184215
Yue X L, WangJ D, Wei Z J, Guo B H, Liu S H 2012 Acta Phys. Sin. 61 184215
[4] 杨璐, 马鸿洋, 郑超, 丁晓兰, 高健存, 龙桂鲁 2017 66 230303
Google Scholar
Yang L, Ma H X, Zhen C, Ding X L, Gao J C, Long G L 2017 Acta Phys. Sin. 66 230303
Google Scholar
[5] 邓富国, 李熙涵, 李涛 2018 67 130301
Google Scholar
Deng G F, Li X H, Li T 2018 Acta Phys. Sin. 67 130301
Google Scholar
[6] Bennett C H, Brassard G 1984 IEEE International Conference on Computers New York 198 4
[7] Chen X B, Tang X, Xu G, Dou Z, Chen Y L, Yang Y X 2018 Quantum Inf. Process 17 225
Google Scholar
[8] Chen X B, SunY R, Xu G, Jia H Y, Qu Z, Yang Y X 2017 Quantum Inf. Process 16 244
Google Scholar
[9] Xu G, Xiao K, Li Z, Niu X X, Ryan M 2019 Comput. Mater. Con. 58 809
[10] Xu G, Chen X B, Li J, Wang C, Yang Y X, Li Z 2015 Quantum Inf. Process 14 4297
Google Scholar
[11] Chen X B, Wang Y L, Xu G, Yang Y X 2019 IEEE Access 7 13634
Google Scholar
[12] Liu H W, Qu W X, Dou T Q, Wang J P, Zhang Y, Ma H Q 2018 Chin. Phys. B 27 212
[13] Zhang H, Mao Y, Hang D, Guo Y, Wu X D, Zhang L 2018 Chin. Phys. B 27 90307
Google Scholar
[14] Lo H 1999 Science 283 2050
Google Scholar
[15] Norbert L 2000 Phys. Rev. A 61 052304
Google Scholar
[16] Shor P W, Preskill J 2000 Appl. Phys. Lett. 85 441
Google Scholar
[17] Renner R 2005 Phys. Rev. A 72 012332
Google Scholar
[18] 吴承峰, 杜亚男, 王金东, 魏正军, 秦晓娟, 赵峰, 张智明 2016 65 100302
Google Scholar
Wu C F, Du Y N, Wang J D, Wei Z J, Qin X J, Zhao F, Zhang Z M 2016 Acta Phys. Sin. 65 100302
Google Scholar
[19] Wang J D, Qin X J, Jiang Y Z, Wang X J, Chen L W, Zhao F, Wei Z J, Zhang Z M 2016 Opt. Express 24 8302
Google Scholar
[20] Brassard G, Lütkenhaus N, Mor T, Sanders B C 2000 Appl. Phys. Lett. 85 1330
Google Scholar
[21] Lydersen L, Wiechers C, Wittmann C, Elser D, Skaar J, Makarov V 2010 Nat. Photon. 4 686
Google Scholar
[22] Wang J D, Wang H, Qin X J, Wei Z J, Zhang Z M 2016 Eur. Phys. J. D 70 1
Google Scholar
[23] Vadim M, Hjelme D R 2005 J. Mod. Opt. 52 691
Google Scholar
[24] Qi B, Fung C H F, Lo H K, Ma X 2007 Quantum Inf. Comput. 7 73
[25] Hadfield R H 2009 Nat. Photon. 3 696
Google Scholar
[26] Newman R 1955 Phys. Rev. 100 700
Google Scholar
[27] Chynoweth A G, Mckay K G 1956 Phys. Rev. 102 369
Google Scholar
[28] Childs P A, Eccleston W 1984 J. Appl. Phys. 55 4304
Google Scholar
[29] Waldschmidt M, Wittig S 1968 Nucl. Instrum. Meth. 64 189
Google Scholar
[30] Gautam D K, Khokle W S, Garg K B 1988 Solid State Electron 31 219
Google Scholar
[31] Lacaita A L, Zappa F, Bigliardi S, Manfredi M 1993 IEEE Trans. Electron Dev. 40 577
[32] Lacaita A, Cova S, Spinelli A, Zappa F 1993 Appl. Phys. Lett. 62 606
Google Scholar
[33] Villa S, Lacaita A L, Pacelli A 1995 Phys. Rev. B 52 10993
Google Scholar
[34] Akil N, Kerns S E, Kerns D V, Charles J P 1998 Appl. Phys. Lett. 73 871
Google Scholar
[35] Kurtsiefer C, Zarda P, Mayer S, Weinfurter H 2001 J. Mod. Opt. 48 2039
[36] Acerbi F, Tosi A, Zappa F 2013 IEEE Photon. Tech. L. 25 1778
Google Scholar
[37] Meda A, Degiovanni I P, Tosi A, Yuan Z, Brida G, Genovese M 2017 Light-Sci. Appl. 6 e16261
Google Scholar
[38] Marini L, Camphausen R, Xiong C, Eggleton B J, Palomba S 2016 Conference on Optical Fibre Technology Australian OSA, September, 2016pAW5C-4
[39] Shi Y, Lim J Z J, Poh H S, Tan P K, Tan P A, Ling A, Kurtsiefer C 2017 Opt. Express 25 30388
Google Scholar
[40] Pinheiro P V P, Chaiwongkhot P, Sajeed S, Horn R T, Bourgoin J P, Jennewein T, Makarov V 2018 Opt. Express 26 21020
Google Scholar
[41] Chen J, Wu G, Li Y, Wu E, Zeng H 2007 Opt. Express 15 17928
Google Scholar
[42] Temporao G P 2009 New J. Phys. 11 045015
Google Scholar
[43] Chen J, Wu G, Xu L, Gu X, Wu E, Zeng H 2009 New J. Phys. 11 065004
Google Scholar
-
图 1 在时分复用的光纤偏振编码QKD中利用反向荧光窃取偏振信息, 其中LD1–5为激光器; ATT1–7为可调谐光衰减器; PBS1–6为偏振分束器; PC1–8为手动偏振控制器; BS1–9为分束器; EPC1–4为电动偏振控制器; AMP为电压放大器; APD1–14为雪崩光电探测器; CIR为环形器
Fig. 1. Polarization information is obtained by backflash in a TDM fiber polarization coded QKD. LD1–5, laser; ATT1–7, variable optical attenuators; PBS1–6, polarization beam splitters; PC1–8, manual polarization-controllers; BS1–9, beam splitters; EPC1–4, electronic polarization-controllers; AMP, voltage amplifier; APD1–14, avalanche photodetector; CIR, circulator.
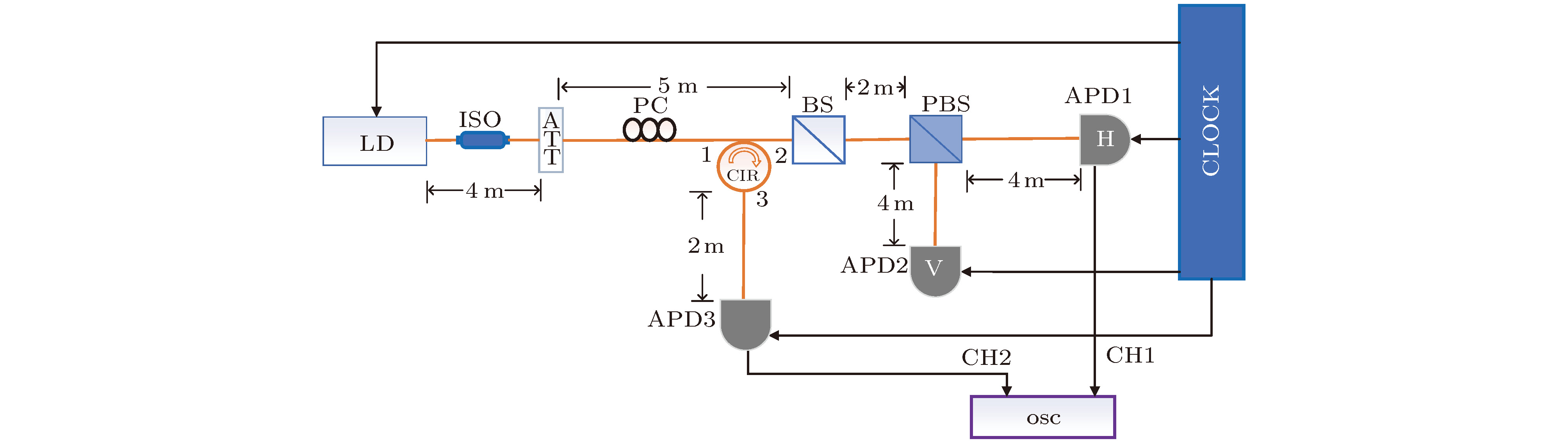
图 2 探测光纤偏振编码QKD中携带有偏振信息的反向荧光概率 LD为激光器(QCL-102); ATT为衰减器(SM3301); APD1–3为单光子探测器(ID200, ID200, ID201); CLOCK为时钟信号源(DG645); OSC为示波器(WAVERUNNER 8404 M); 电线长度相同
Fig. 2. Probability detection of the backflash of the polarization-encoded QKD carrying polarization information. LD, laser (qcl-102); ATT, attenuator (SM3301); APD1–3, avalanche photodetector (ID200, ID200, ID201); CLOCK, clock (DG645); OSC, oscilloscope (WAVERUNNER 8404 M); the cables are the same length.
图 3 Eve和Bob之间的符合计数直方图, 第三个峰为探测到的反向荧光光子数分布, 其他峰值为光学仪器的端面反射光
Fig. 3. Coincidence count histogram between Bob and Eve. The third peak is the detected backflash photon number distribution, and the other peaks denote the reflected light of the optical instrument.
-
[1] Gisin N, Ribordy G, Tittel W, Zbinden H 2002 Rev. Mod. Phys. 74 145
Google Scholar
[2] Xu G, Chen X B, Dou Z, Yang Y X, Li Z 2015 Quantum Inf. Process 14 2959
Google Scholar
[3] 岳孝林, 王金东, 魏正军, 郭邦红, 刘颂豪 2012 61 184215
Yue X L, WangJ D, Wei Z J, Guo B H, Liu S H 2012 Acta Phys. Sin. 61 184215
[4] 杨璐, 马鸿洋, 郑超, 丁晓兰, 高健存, 龙桂鲁 2017 66 230303
Google Scholar
Yang L, Ma H X, Zhen C, Ding X L, Gao J C, Long G L 2017 Acta Phys. Sin. 66 230303
Google Scholar
[5] 邓富国, 李熙涵, 李涛 2018 67 130301
Google Scholar
Deng G F, Li X H, Li T 2018 Acta Phys. Sin. 67 130301
Google Scholar
[6] Bennett C H, Brassard G 1984 IEEE International Conference on Computers New York 198 4
[7] Chen X B, Tang X, Xu G, Dou Z, Chen Y L, Yang Y X 2018 Quantum Inf. Process 17 225
Google Scholar
[8] Chen X B, SunY R, Xu G, Jia H Y, Qu Z, Yang Y X 2017 Quantum Inf. Process 16 244
Google Scholar
[9] Xu G, Xiao K, Li Z, Niu X X, Ryan M 2019 Comput. Mater. Con. 58 809
[10] Xu G, Chen X B, Li J, Wang C, Yang Y X, Li Z 2015 Quantum Inf. Process 14 4297
Google Scholar
[11] Chen X B, Wang Y L, Xu G, Yang Y X 2019 IEEE Access 7 13634
Google Scholar
[12] Liu H W, Qu W X, Dou T Q, Wang J P, Zhang Y, Ma H Q 2018 Chin. Phys. B 27 212
[13] Zhang H, Mao Y, Hang D, Guo Y, Wu X D, Zhang L 2018 Chin. Phys. B 27 90307
Google Scholar
[14] Lo H 1999 Science 283 2050
Google Scholar
[15] Norbert L 2000 Phys. Rev. A 61 052304
Google Scholar
[16] Shor P W, Preskill J 2000 Appl. Phys. Lett. 85 441
Google Scholar
[17] Renner R 2005 Phys. Rev. A 72 012332
Google Scholar
[18] 吴承峰, 杜亚男, 王金东, 魏正军, 秦晓娟, 赵峰, 张智明 2016 65 100302
Google Scholar
Wu C F, Du Y N, Wang J D, Wei Z J, Qin X J, Zhao F, Zhang Z M 2016 Acta Phys. Sin. 65 100302
Google Scholar
[19] Wang J D, Qin X J, Jiang Y Z, Wang X J, Chen L W, Zhao F, Wei Z J, Zhang Z M 2016 Opt. Express 24 8302
Google Scholar
[20] Brassard G, Lütkenhaus N, Mor T, Sanders B C 2000 Appl. Phys. Lett. 85 1330
Google Scholar
[21] Lydersen L, Wiechers C, Wittmann C, Elser D, Skaar J, Makarov V 2010 Nat. Photon. 4 686
Google Scholar
[22] Wang J D, Wang H, Qin X J, Wei Z J, Zhang Z M 2016 Eur. Phys. J. D 70 1
Google Scholar
[23] Vadim M, Hjelme D R 2005 J. Mod. Opt. 52 691
Google Scholar
[24] Qi B, Fung C H F, Lo H K, Ma X 2007 Quantum Inf. Comput. 7 73
[25] Hadfield R H 2009 Nat. Photon. 3 696
Google Scholar
[26] Newman R 1955 Phys. Rev. 100 700
Google Scholar
[27] Chynoweth A G, Mckay K G 1956 Phys. Rev. 102 369
Google Scholar
[28] Childs P A, Eccleston W 1984 J. Appl. Phys. 55 4304
Google Scholar
[29] Waldschmidt M, Wittig S 1968 Nucl. Instrum. Meth. 64 189
Google Scholar
[30] Gautam D K, Khokle W S, Garg K B 1988 Solid State Electron 31 219
Google Scholar
[31] Lacaita A L, Zappa F, Bigliardi S, Manfredi M 1993 IEEE Trans. Electron Dev. 40 577
[32] Lacaita A, Cova S, Spinelli A, Zappa F 1993 Appl. Phys. Lett. 62 606
Google Scholar
[33] Villa S, Lacaita A L, Pacelli A 1995 Phys. Rev. B 52 10993
Google Scholar
[34] Akil N, Kerns S E, Kerns D V, Charles J P 1998 Appl. Phys. Lett. 73 871
Google Scholar
[35] Kurtsiefer C, Zarda P, Mayer S, Weinfurter H 2001 J. Mod. Opt. 48 2039
[36] Acerbi F, Tosi A, Zappa F 2013 IEEE Photon. Tech. L. 25 1778
Google Scholar
[37] Meda A, Degiovanni I P, Tosi A, Yuan Z, Brida G, Genovese M 2017 Light-Sci. Appl. 6 e16261
Google Scholar
[38] Marini L, Camphausen R, Xiong C, Eggleton B J, Palomba S 2016 Conference on Optical Fibre Technology Australian OSA, September, 2016pAW5C-4
[39] Shi Y, Lim J Z J, Poh H S, Tan P K, Tan P A, Ling A, Kurtsiefer C 2017 Opt. Express 25 30388
Google Scholar
[40] Pinheiro P V P, Chaiwongkhot P, Sajeed S, Horn R T, Bourgoin J P, Jennewein T, Makarov V 2018 Opt. Express 26 21020
Google Scholar
[41] Chen J, Wu G, Li Y, Wu E, Zeng H 2007 Opt. Express 15 17928
Google Scholar
[42] Temporao G P 2009 New J. Phys. 11 045015
Google Scholar
[43] Chen J, Wu G, Xu L, Gu X, Wu E, Zeng H 2009 New J. Phys. 11 065004
Google Scholar
下载:
计量
- 文章访问数: 7760
- PDF下载量: 70
- 被引次数: 0
