-
Modern systems are always coupled. Previous studies indicate that coupled systems are more fragile than single systems. In a single system, when a fraction of 1-p nodes are removed, the percolation process is often of the second order. In a coupled system, due to the lack of resilience, the phase transition is always of the first order when removing a fraction of nodes. Most of previous studies on coupled systems focus on one-to-one dependency relation. This kind of relationship is called a no-feedback condition. Existing studies suppose that coupled systems are much more fragile without a no-feedback condition. That is to say, if a node depends on more than one node, the coupled system will breakdown even when a small fraction of nodes are removed from the coupled system. By observing the real world system, real nodes are often dependent on a dependency cluster, which consists of more than one other node. For example, in an industry chain, an electronic equipment factory may need several raw material factories to supply production components. Despite part of the raw material factories being bankrupt, the electronic equipment factory can carry out productionnormally because the remaining raw material factories still supply the necessary production components. But theoretical analysis shows that the robustness of such a coupled system is worse than that of one-to-one dependency system. Actually, the coupled system in real world does not usually disintegrate into pieces after some nodes have become invalid. To explain this phenomenon, we model a coupled system as interdependent networks and study, both analytically and numerically, the percolation in interdependent networks with conditional dependency clusters. A node in our model survives until the number of failed nodes in its dependency cluster is greater than a threshold. Our exact solutions of giant component size are in good agreement with the simulation results. Though our model does not have second order phase transition, we still find ways to improve the robustness of interdependent networks. One way is to increase the dependency cluster failure threshold. A higher threshold means that more nodes in the dependency cluster can be removed without breaking down the node depending on the cluster. Other way is to increase the size of dependency clusters, the more the nodes in the dependency cluster, the more the failure combinations are, which increases the survival probability of the node depending on cluster. Our model offers a useful strategy to enhance the robustness of coupled system and makes a good contribution to the study of interdependent networks with dependency clusters.
-
Keywords:
- interdependent networks /
- cascading failures /
- percolation /
- phase transition
[1] Albert R, Barabási A 2002 Rev. Mod. Phys. 74 47
Google Scholar
[2] Dorogovtsev S N, Goltsev A V, Mendes J F 2008 Rev. Mod. Phys. 80 1275
Google Scholar
[3] Buldyrev S V, Parshani R, Paul G, Stanley H E, Havlin S 2010 Nature 464 1025
Google Scholar
[4] Rosato V, Issacharoff L, Tiriticco F, Meloni S, Porcellinis S, Setola R 2008 Int. J. Crit. Infr. 4 63
Google Scholar
[5] Parshani R, Buldyrev S V, Havlin S 2010 Phys. Rev. Lett. 105 48701
Google Scholar
[6] Shao J, Buldyrev S V, Havlin S, Stanley H E 2011 Phys. Rev. E 83 36116
Google Scholar
[7] Parshani R, Buldyrev S V, Havlin S 2011 Proc. Natl. Acad. Sci. USA 108 1007
Google Scholar
[8] Li M, Wang B H 2014 Chin. Phys. B 23 79
[9] Wang H, Li M, Deng L, Wang B H 2015 Plos One 10 e126674
[10] Liu R R, Li M, Jia C X, Wang B H 2016 Sci. Rep. 6 25294
Google Scholar
[11] Di Muro M A, La Rocca C E, Stanley H E, Havlin S, Braunstein L A 2016 Sci. Rep. 6 22834
Google Scholar
[12] Yuan X, Hu Y Q, Stanley H E, Havlin S 2017 Proc. Natl. Acad. Sci. USA 114 3311
Google Scholar
[13] 吴佳键, 龚凯, 王聪, 王磊 2018 67 088901
Google Scholar
Wu J J, Gong K, Wang C, Wang L 2018 Acta Phys. Sin. 67 088901
Google Scholar
[14] Newman M 2010 Networks. An introduction(1st Ed.) (Oxford: Oxford University Press) pp 414 − 423
[15] La Rocca C E, Stanley H E, Braunstein L A 2018 Physica A 508 577
Google Scholar
[16] Kong L W, Li M, Liu R R, Wang B H 2017 Phys. Rev. E 95 32301
[17] Li W, Bashan A, Buldyrev S V, Stanley H E, Havlin S 2012 Phys. Rev. Lett. 108 228702
Google Scholar
[18] Gao J X, Buldyrev S V, Stanley H E, Havlin S 2012 Nat. Phys. 8 40
Google Scholar
[19] Di Muro M A, Buldyrev S V, Stanley H E, Braunstein L A 2016 Phys. Rev. E 94 42304
[20] Wang Z X, Zhou D, Hu Y Q 2018 Phys. Rev. E 97 32306
[21] Wang H, Li M, Deng L, Wang B H 2018 Physica A 502 195
Google Scholar
[22] Newman M E, Strogatz S H, Watts D J 2001 Phys. Rev. E 64 26118
Google Scholar
[23] Feng L, Monterola C P, Hu Y Q 2015 New J. Phys. 17 63025
Google Scholar
[24] Erdős P, Rényi A 1959 Publ. Math. 62 90
[25] Wilf H S 1994 Generating Functionology (2nd Ed.)(London: Academic Press) pp 1 − 16
-
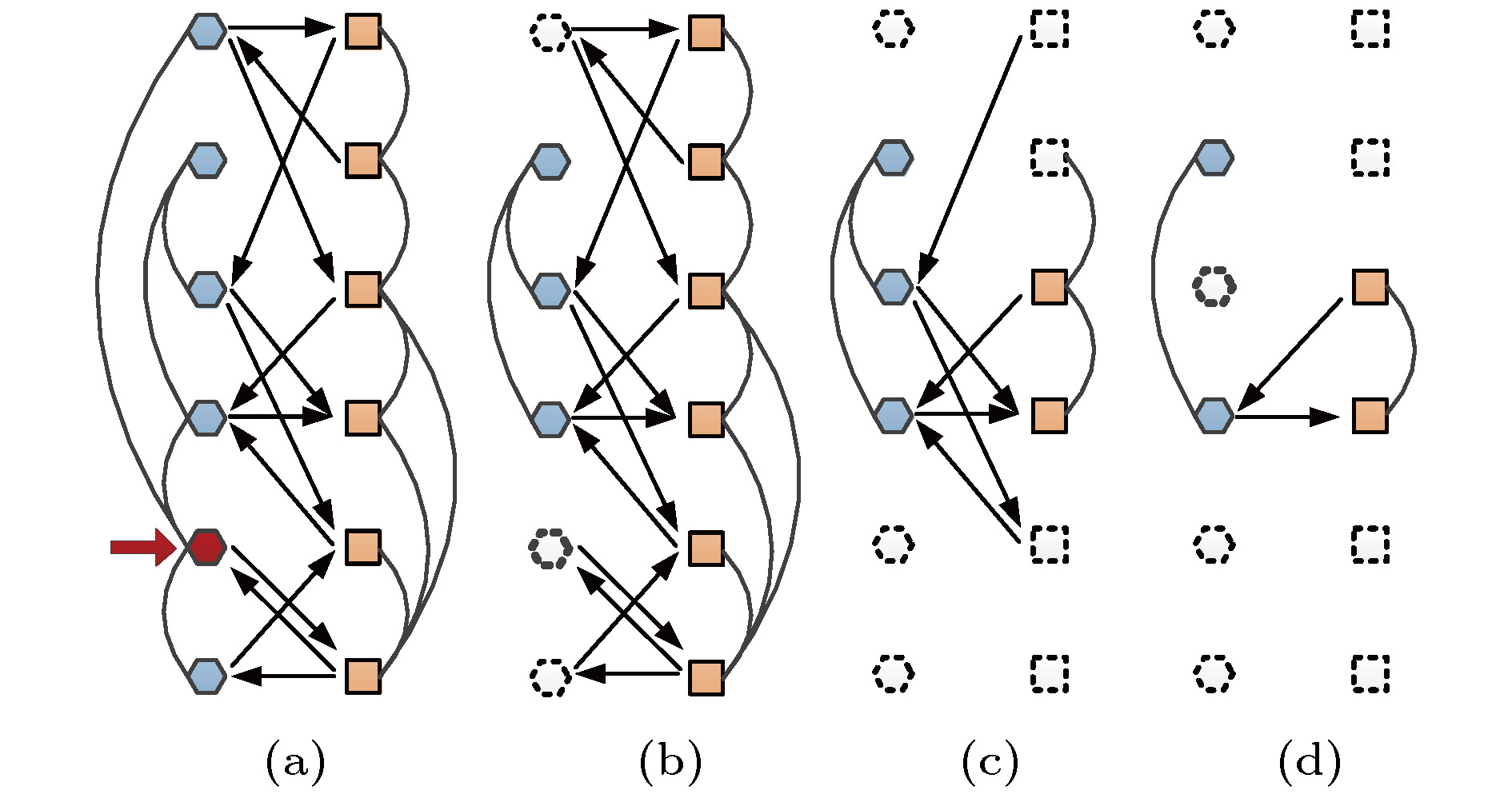
图 1 具有多依赖关系的相依网络逾渗示意图, 初始攻击导致红色节点失效, 随后发生级联失效过程, 最终相依网络仅有极少数节点存活
Fig. 1. The model of percolation of interdependent networks with multiple support-dependence relations. The red node fails after the initial attack. Then the cascading failure process leads to a catastrophiccollapse of the interdependent networks. Finally, only a small fraction of nodes survive.
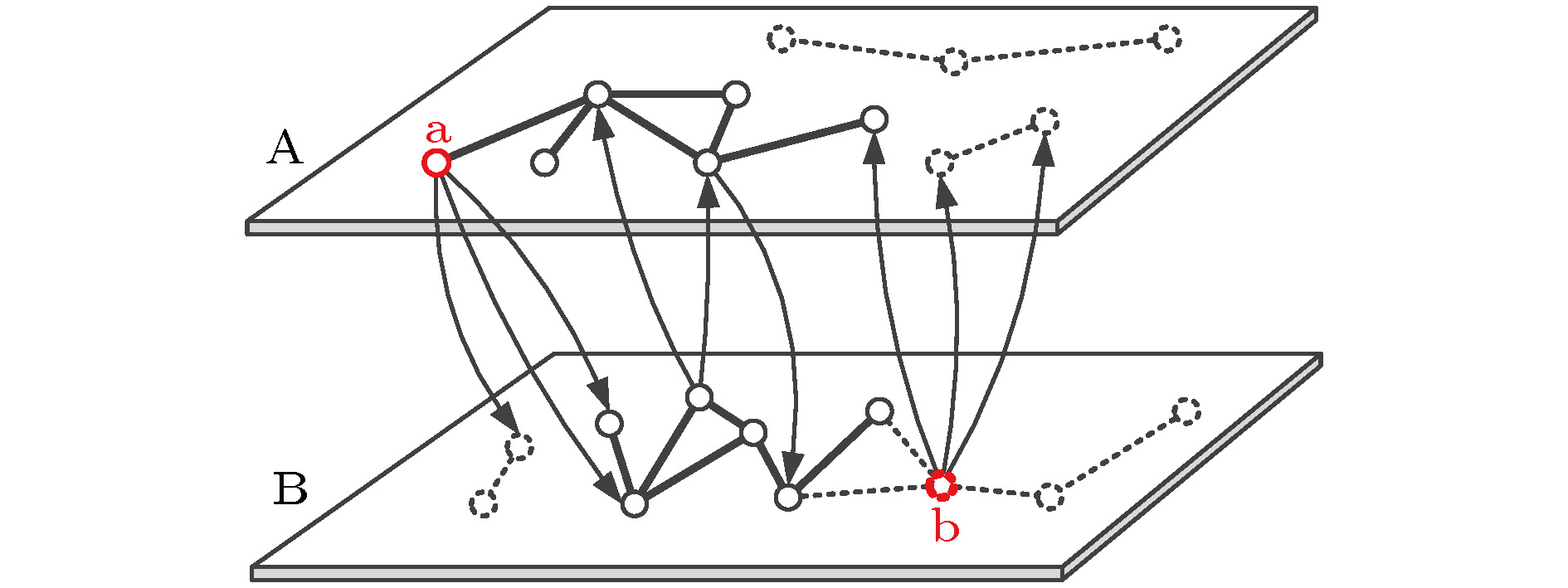
图 2 相依网络的条件依赖群逾渗模型 A网络节点随机依赖于B网络的
$\tilde k$ 个节点($\tilde k \geqslant 0$ ), 反之亦然; A网络的a节点依赖的3个节点有一个失效, 但失效比例未超过容忍度$\gamma = 0.5$ , a节点继续工作; B网络的b节点依赖的节点失效比例超过$\gamma = 0.5$ , 所以b节点失效Fig. 2. The model of percolation in interdependent networks with conditional dependency clusters. Nodes in network A randomly depends on
$\tilde k$ ($\tilde k \geqslant 0$ ) nodes in network B and vice versa. One of the three nodes which node a in network A depends on fails, but the failure proportion does not exceed$\gamma = 0.5$ , node a still works. Two of the three nodes which node b in network B depends on fail, the failure proportion exceeds$\gamma = 0.5$ , node bfails.
图 3 不同
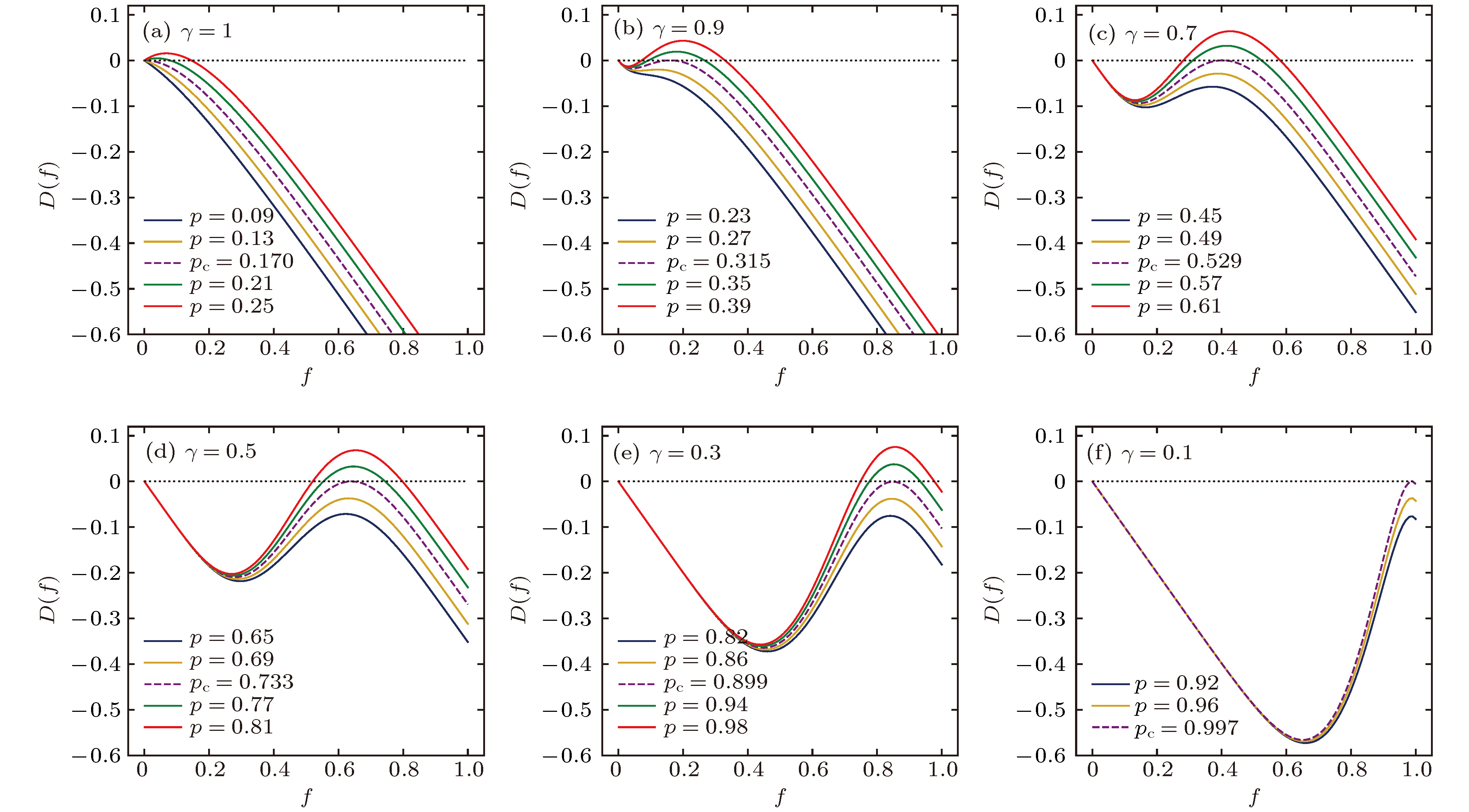
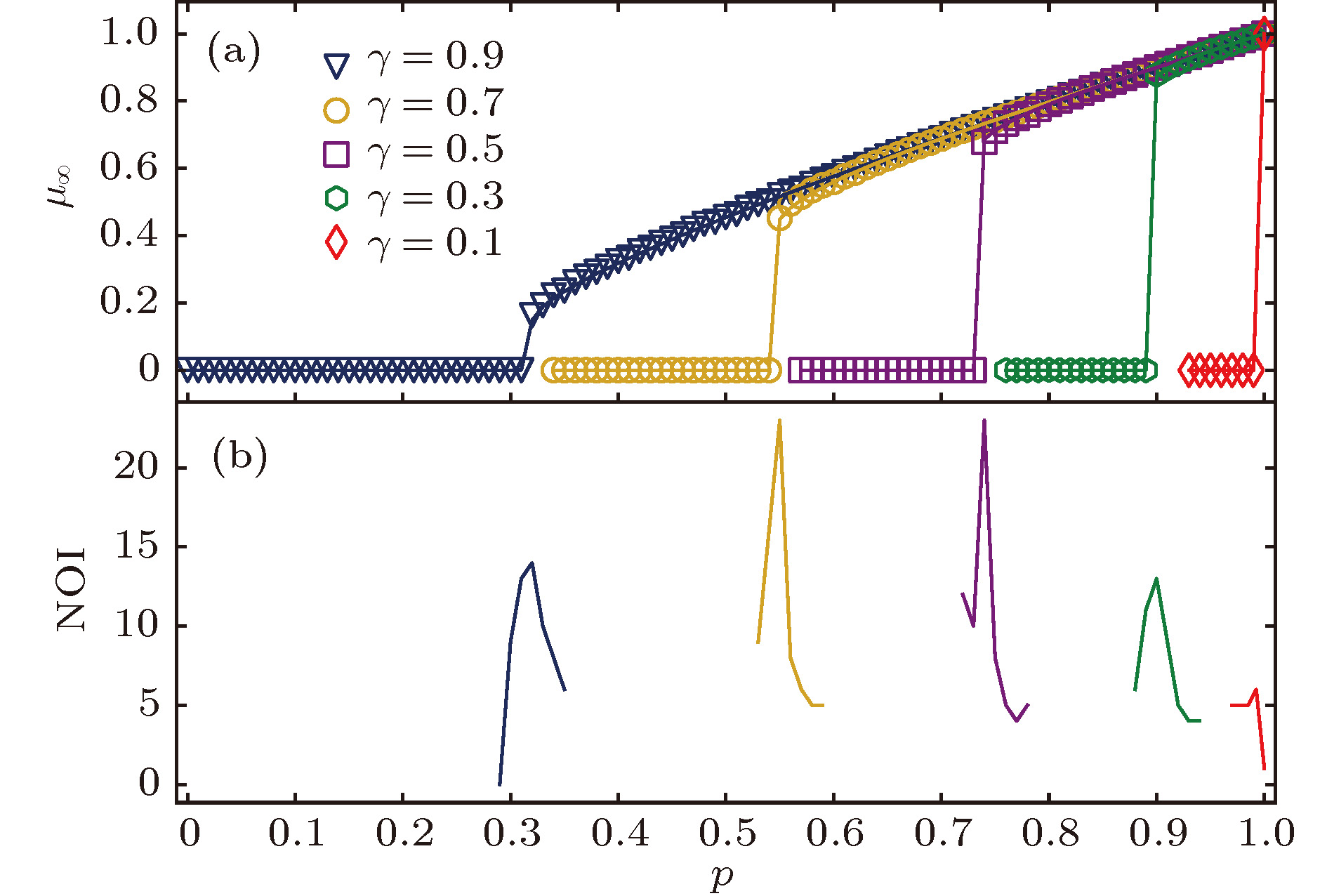
$\gamma $ 取值的RR-RR相依网络逾渗, 每个网络节点数$N = 200000$ , 平均度$\left\langle k \right\rangle = 6$ ,$\tilde P(10) = 1$ , 空心标记为仿真值, 实线为逾渗方程数值解 (a) 巨分量${\mu _\infty }$ 与$p$ 对应关系; (b) 级联失效迭代次数(number of iterations, NOI)Fig. 3. The results of the percolation of RR-RR interdependent networks for different
$\gamma $ , each network has 200000 nodes, average degree is 6,$\tilde P(10) = 1$ . The symbols represent the simulation results, and the solid linesshow the corresponding analytical predictions. (a) The size ofthe giant component${\mu _\infty }$ versus$p$ ; (b) number of iterations.
图 4 不同
$\gamma $ 值对应的ER-ER相依网络相变点, 网络平均度$\left\langle k \right\rangle = 6$ ,$\tilde P(10) = 1$ Fig. 4. Graphical solutions of ER-ER interdependent networks transition for different
$\gamma $ . The average degree is 6,$\tilde P(10) = 1$ .
图 5 不同
$\gamma $ 取值的ER-ER相依网络逾渗, 每个网络节点数$N = 200000$ , 平均度$\left\langle k \right\rangle = 6$ ,$\tilde P(10) = 1$ , 空心标记为仿真值, 实线为逾渗方程数值解 (a) 巨分量${\mu _\infty }$ 与$p$ 对应关系; (b) 级联失效迭代次数Fig. 5. The results of the percolation of ER-ER interdependent networks for different
$\gamma $ , each network has 200000 nodes, average degree is 6,$\tilde P(10) = 1$ . The symbols represent the simulation results, and the solid linesshow the corresponding analytical predictions. (a) The size ofthe giant component${\mu _\infty }$ versus$p$ ; (b) number of iterations.
图 6 不同依赖度分布的ER-ER相依网络逾渗,
$\tilde P(10) = 1$ ,$\tilde P(5) = 1$ 分别用实心和空心标记表示, 每个网络节点数$N = 200000$ , 平均度$\left\langle k \right\rangle = 6$ (a) 巨分量${\mu _\infty }$ 与$p$ 对应关系; (b) 级联失效迭代次数Fig. 6. The results of the percolation of ER-ER interdependent networks for different dependency degrees, each network has 200000 nodes, average degree is 6. The dependency degrees are
$\tilde P(10) = 1$ (solid symbols) and$\tilde P(5) = 1$ (empty symbols). (a) The size ofthe giant component${\mu _\infty }$ versus$p$ ; (b) number of iterations.
图 7 不同
$\gamma $ 取值的ER-ER相依网络相变点, 空心标记为仿真结果, 实线是理论值Fig. 7. The critical point
${p_{\rm{c}}}$ versus$\gamma $ of ER-ER interdependent networks, The symbols represent the simulation results, and the solid linesshow the corresponding analytical predictions.
图 8 不同
$\gamma $ 取值的SF-SF相依网络逾渗, 每个网络节点数$N = 200000$ , 平均度$\left\langle k \right\rangle = 6$ ,$\lambda = 2.7$ ,$\tilde P(10) = 1$ , 空心标记为仿真值, 实线为逾渗方程数值解 (a) 巨分量${\mu _\infty }$ 与$p$ 对应关系; (b) 级联失效迭代次数Fig. 8. The results of the percolation of SF-SF interdependent networks for different
$\gamma $ , each network has 200000 nodes, average degree is 6,$\lambda = 2.7$ ,$\tilde P(10) = 1$ . The symbols represent the simulation results, and the solid linesshow the corresponding analytical predictions. (a) The size ofthe giant component${\mu _\infty }$ versus$p$ ; (b) number of iterations. -
[1] Albert R, Barabási A 2002 Rev. Mod. Phys. 74 47
Google Scholar
[2] Dorogovtsev S N, Goltsev A V, Mendes J F 2008 Rev. Mod. Phys. 80 1275
Google Scholar
[3] Buldyrev S V, Parshani R, Paul G, Stanley H E, Havlin S 2010 Nature 464 1025
Google Scholar
[4] Rosato V, Issacharoff L, Tiriticco F, Meloni S, Porcellinis S, Setola R 2008 Int. J. Crit. Infr. 4 63
Google Scholar
[5] Parshani R, Buldyrev S V, Havlin S 2010 Phys. Rev. Lett. 105 48701
Google Scholar
[6] Shao J, Buldyrev S V, Havlin S, Stanley H E 2011 Phys. Rev. E 83 36116
Google Scholar
[7] Parshani R, Buldyrev S V, Havlin S 2011 Proc. Natl. Acad. Sci. USA 108 1007
Google Scholar
[8] Li M, Wang B H 2014 Chin. Phys. B 23 79
[9] Wang H, Li M, Deng L, Wang B H 2015 Plos One 10 e126674
[10] Liu R R, Li M, Jia C X, Wang B H 2016 Sci. Rep. 6 25294
Google Scholar
[11] Di Muro M A, La Rocca C E, Stanley H E, Havlin S, Braunstein L A 2016 Sci. Rep. 6 22834
Google Scholar
[12] Yuan X, Hu Y Q, Stanley H E, Havlin S 2017 Proc. Natl. Acad. Sci. USA 114 3311
Google Scholar
[13] 吴佳键, 龚凯, 王聪, 王磊 2018 67 088901
Google Scholar
Wu J J, Gong K, Wang C, Wang L 2018 Acta Phys. Sin. 67 088901
Google Scholar
[14] Newman M 2010 Networks. An introduction(1st Ed.) (Oxford: Oxford University Press) pp 414 − 423
[15] La Rocca C E, Stanley H E, Braunstein L A 2018 Physica A 508 577
Google Scholar
[16] Kong L W, Li M, Liu R R, Wang B H 2017 Phys. Rev. E 95 32301
[17] Li W, Bashan A, Buldyrev S V, Stanley H E, Havlin S 2012 Phys. Rev. Lett. 108 228702
Google Scholar
[18] Gao J X, Buldyrev S V, Stanley H E, Havlin S 2012 Nat. Phys. 8 40
Google Scholar
[19] Di Muro M A, Buldyrev S V, Stanley H E, Braunstein L A 2016 Phys. Rev. E 94 42304
[20] Wang Z X, Zhou D, Hu Y Q 2018 Phys. Rev. E 97 32306
[21] Wang H, Li M, Deng L, Wang B H 2018 Physica A 502 195
Google Scholar
[22] Newman M E, Strogatz S H, Watts D J 2001 Phys. Rev. E 64 26118
Google Scholar
[23] Feng L, Monterola C P, Hu Y Q 2015 New J. Phys. 17 63025
Google Scholar
[24] Erdős P, Rényi A 1959 Publ. Math. 62 90
[25] Wilf H S 1994 Generating Functionology (2nd Ed.)(London: Academic Press) pp 1 − 16
下载:
计量
- 文章访问数: 7104
- PDF下载量: 69
- 被引次数: 0
