-
本文采用耦合凝固和熔化效应的二维元胞自动机(cellular automaton, CA)模型, 对温度梯度区域熔化(temperature gradient zone melting, TGZM)效应引起的熔池在固液两相区中的迁移现象进行模拟研究. 模拟分析了抽拉速度、熔池初始位置、温度梯度和合金成分等因素对TGZM动力学的影响, 并将模拟结果与解析模型的预测结果进行比较验证. 通过模拟发现, 在温度梯度作用下, 熔池总是向着高温方向迁移; 当抽拉速度低于或高于临界抽拉速度时, 熔池朝向移动的液相线或固相线迁移; 对于给定的抽拉速度, 位于糊状区内临界位置以上的熔池会迁移进入液相, 而位于临界位置以下的熔池会逐步靠近固相线. 此外, 温度梯度越高, 合金成分越低, 熔池的迁移速度越快.Directional solidification is a common and important process in both scientific research and industrial practice. Owing to the presence of temperature gradients during directional solidification, local remelting and solidification in the mushy zone occurs, resulting in some typical phenomena such as temperature gradient zone melting (TGZM). The TGZM influences the solidifying microstructure and microsegregation significantly. In the present work, a two-dimensional (2D) cellular automaton (CA) model involving the mechanism of both solidification and melting is adopted to investigate the migration phenomena of molten liquid pools in the mushy zone due to the TGZM. The effect of pulling velocity, initial liquid pool position, temperature gradient, and alloy composition on the TGZM kinetics are studied. The simulation results are compared with the analytical predictions, and good agreement between two models is obtained. It is found that under a temperature gradient, the liquid pool always migrates towards the high temperature direction. When the pulling velocity is lower than the critical velocity, the liquid pool migrates through the liquidus into the bulk liquid and the time required for a liquid pool to reach the liquidus increases with pulling velocity increasing. On the other hand, when a pulling velocity higher than the critical value is adopted, the liquid pool moves towards the solidus and the time required for migrating liquid pool to reach the solidus decreases with pulling velocity increasing. For a given pulling velocity, the liquid pools located above the critical position move towards the liquidus, while the others gradually approach to the solidus. When a molten liquid pool migrates towards the liquidus, the migration velocity and liquid pool thickness are found to gradually increase, while the liquid pool composition decreases with time. Inversely, for the molten liquid pool that moves towards the solidus, the migration velocity and liquid pool thickness gradually decrease, while the liquid pool composition increases with time going by. The average migration velocity of liquid pool caused by the TGZM effect increases with temperature gradient increasing and alloy composition decreasing. The CA simulations provide an insight into the complicated interactions among the local temperature, solute distribution and diffusion, and the kinetics of local remelting and solidification in the TGZM process.
-
Keywords:
- directional solidification /
- temperature gradient zone melting /
- liquid pool migration /
- cellular automaton
[1] Rettenmayr M 2009 Int. Mater. Rev. 54 1
Google Scholar
[2] Pfann W G 1955 Trans. AIME 203 961
[3] Tiller W A 1963 J. Appl. Phys. 34 2757
Google Scholar
[4] Allen D J, Hunt J D 1976 Metall. Mater. Trans. A 7 767
Google Scholar
[5] Kinoshita K, Arai Y, Inatomi Y, Tsukada T, Miyata H, Tanaka R 2016 J. Cryst. Growth 455 49
Google Scholar
[6] Wang H, Li S, Li X, Zhong H 2017 J. Cryst. Growth 466 56
Google Scholar
[7] Ma X, Yoshikawa T, Morita K 2014 Sci. Adv. Mater. 6 1697
Google Scholar
[8] Shirzadi A A, Wallach E R 1999 Acta Mater. 47 3551
Google Scholar
[9] Nguyen-Thi H, Reinhart G, Buffet A, Schenk T, Mangelinck-Noel N, Jung H, Bergeon N, Billia B, Hartwig J, Baruchel J 2008 J. Cryst. Growth 310 2906
Google Scholar
[10] Buchmann M, Rettenmayr M 2005 J. Cryst. Growth 284 544
Google Scholar
[11] Bösenberg U, Buchmann M, Rettenmayr M 2007 J. Cryst. Growth 304 281
Google Scholar
[12] Löffler A, Reuther K, Engelhardt H, Liu D, Rettenmayr M 2015 Acta Mater. 91 34
Google Scholar
[13] Liu D M, Li X Z, Su Y Q, Peng P, Luo L S, Guo J J, Fu H Z 2012 Acta Mater. 60 2679
Google Scholar
[14] Liu D M, Li X Z, Su Y Q, Rettenmayr M, Guo J J, Fu H Z 2014 Appl. Phys. A 116 1821
Google Scholar
[15] Pan S Y, Zhang Q Y, Zhu M F, Rettenmayr M 2015 Acta Mater. 86 229
Google Scholar
[16] 朱鸣芳, 汤倩玉, 张庆宇, 潘诗琰, 孙东科 2016 金属学报 52 1297
Google Scholar
Zhu M F, Tang Q Y, Zhang Q Y, Pan S Y, Sun D K 2016 Acta Metall. Sin. 52 1297
Google Scholar
[17] 陈瑞, 许庆彦, 柳百成 2014 63 188102
Google Scholar
Chen R, Xu Q Y, Liu B C 2014 Acta Phys. Sin . 63 188102
Google Scholar
[18] 张云鹏, 林鑫, 魏雷, 王猛, 彭东剑, 黄卫东 2012 61 228106
Google Scholar
Zhang Y P, Lin X, Wei L, Wang M, Peng D J, Huang W D 2012 Acta Phys. Sin. 61 228106
Google Scholar
[19] 江鸿翔, 赵九洲 2011 金属学报 47 1099
Jiang H X, Zhao J Z 2011 Acta Metall. Sin. 47 1099
[20] Zhu M F, Stefanescu D M 2007 Acta Mater. 55 1741
Google Scholar
[21] Pan S, Zhu M 2010 Acta Mater. 58 340
Google Scholar
[22] 李强, 李殿中, 钱百年 2004 53 3477
Google Scholar
Li Q, Li D Z, Qian B N 2004 Acta Phys. Sin. 53 3477
Google Scholar
[23] Wei L, Lin X, Wang M, Huang W D 2015 Chin. Phys. B 24 078108
Google Scholar
[24] Zhang Q Y, Fang H, Xue H, Pan S Y, Rettenmayr M, Zhu M F 2017 Sci. Rep. 7 17809
Google Scholar
[25] 朱鸣芳, 邢丽科, 方辉, 张庆宇, 汤倩玉, 潘诗琰 2018 金属学报 54 789
Google Scholar
Zhu M F, Xing L K, Fang H, Zhang Q Y, Tang Q Y, Pan S Y 2018 Acta Metall. Sin. 54 789
Google Scholar
[26] Zhang Q Y, Xue H, Tang Q Y, Pan S Y, Rettenmayr M, Zhu M F 2018 Comput. Mater. Sci. 146 20
[27] Zhang Q Y, Fang H, Xue H, Tang Q Y, Pan S Y, Rettenmayr M, Zhu M F 2018 Scr. Mater. 151 28
Google Scholar
[28] Farup I, Drezet J M, Rappaz M 2001 Acta Mater. 49 1261
Google Scholar
-
图 1 SCN–0.3 wt.% ACE合金在
$\scriptstyle V_{\rm p}=0.6\;{\text{μ}}{\rm m/s}$ ($\scriptstyle {\tilde y_0} = 0.05$ )和G = 12°C/mm条件下, 迁移熔池位置随时间变化的CA模拟结果与解析模型[15]预测结果的比较Fig. 1. Comparison of the CA simulation with the analytical prediction[15]regarding the time evolution of the location of a migrating liquid pool for a SCN–0.3 wt.% ACE alloy at
$\scriptstyle V_{\rm p}=0.6\;{\text{μ}}{\rm m/s}$ ,$\scriptstyle{\tilde y_0} = 0.05$ and G = 12°C/mm.
图 2 SCN–0.3 wt.%ACE合金在
$\scriptstyle V_{\rm p}=0.6\;{\text{μ}}{\rm m/s}$ ($\scriptstyle{\tilde y_0} = 0.05$ )和G = 12 °C/mm条件下, (a)熔池迁移速度和(b)熔池成分随时间变化的CA模拟和解析模型[15]预测结果的比较Fig. 2. Comparison of the CA simulation with the analytical prediction[15] regarding the time evolution of (a) liquid pool velocity and (b) liquid pool composition for a SCN–0.3 wt.% ACE alloy at
$\scriptstyle V_{\rm p}=0.6\;{\text{μ}}{\rm m/s}$ ,$\scriptstyle{\tilde y_0} = 0.05$ and G = 12°C/mm.
图 3 SCN–0.3 wt.% ACE合金在
$\scriptstyle V_{\rm p}=5\;{\text{μ}}{\rm m/s}$ ($\scriptstyle{\tilde y_0} = 0.65$ )和G = 12 °C/mm条件下, 迁移熔池位置随时间变化的CA模拟结果与解析模型[15]预测结果的比较Fig. 3. Comparison of the CA simulation with the analytical prediction[15] regarding the time evolution of the location of a migrating liquid pool for a SCN–0.3 wt.% ACE alloy at
$\scriptstyle V_{\rm p}=5\;{\text{μ}}{\rm m/s}$ ,$\scriptstyle {\tilde y_0} = 0.65$ and G = 12 °C/mm.
图 4 SCN–0.3 wt.%ACE合金在
$\scriptstyle V_{\rm p}=5\;{\text{μ}}{\rm m/s}$ ($\scriptstyle {\tilde y_0} = 0.65$ )和G = 12°C/mm条件下, (a)熔池迁移速度和(b)熔池成分随时间变化的CA模拟和解析模型[15]预测结果的比较Fig. 4. Comparison of the CA simulation with the analytical prediction[15] regarding the time evolution of (a) liquid pool velocity and (b) liquid pool composition for a SCN–0.3 wt.% ACE alloy at
$\scriptstyle V_{\rm p}=5\;{\text{μ}}{\rm m/s}$ ,$\scriptstyle {\tilde y_0} = 0.65$ and G = 12°C/mm.
图 5 SCN–0.3 wt.% ACE合金在G = 12 °C/mm条件下, (a) Vp < Vp, cr时熔池从初始位置(
$\scriptstyle {\tilde y_0} = 0.05$ )迁移到移动的液相线所需时间tl和(b) Vp > Vp, cr情况下熔池从初始位置($\scriptstyle {\tilde y_0} = 0.65$ )迁移到移动的固相线所需时间ts随抽拉速度变化的CA模拟与解析模型[15]预测结果的比较Fig. 5. Comparison of the CA simulations with the analytical predictions [15] regarding the times required for a liquid pool to reach (a) the moving liquidus from
$\scriptstyle{\tilde y_0} = 0.05$ at Vp < Vp, cr and (b) the moving solidus from$\scriptstyle{\tilde y_0} = 0.65$ at Vp > Vp, cr as a function of the pulling velocity for a SCN–0.3 wt.% ACE alloy at G = 12 °C/mm.
图 6 CA模拟的SCN–0.3 wt.% ACE合金在Vp =
$ \scriptstyle 2.89\;{\text{μ}}{\rm m/s} $ 和G = 12°C/mm的条件下 (相应的临界位置$\scriptstyle{\tilde y_{0, {\rm{cr}}}}$ = 0.5), 5个不同初始位置的熔池的迁移演化过程 (a) 2 s; (b) 20 s; (c) 37 s. 5个熔池的无量纲初始位置分别为${\tilde y_0}$ = 0.08, 0.3, 0.5, 0.6和0.75.$\scriptstyle\widetilde y$ 为无量纲液滴位置, y为液滴位置Fig. 6. Simulated sequence of liquid pool migration for a SCN–0.3 wt.% ACE alloy at G = 12 °C/mm,
$ \scriptstyle V_{\rm p}=2.89\;{\text{μ}}{\rm m/s} $ corresponding to$\scriptstyle {\tilde y_{0, {\rm{cr}}}}$ = 0.5: (a) 2 s; (b) 20 s; (c) 37 s. The dimensionless initial positions of the five liquid pools are$\scriptstyle {\tilde y_0}$ = 0.08, 0.3, 0.5, 0.6 and 0.75, respectively.$\scriptstyle \widetilde y$ is the dimensionless droplet position, y is the droplet position.
图 7 CA模拟的SCN–0.3 wt.% ACE合金在Vp = 2.89
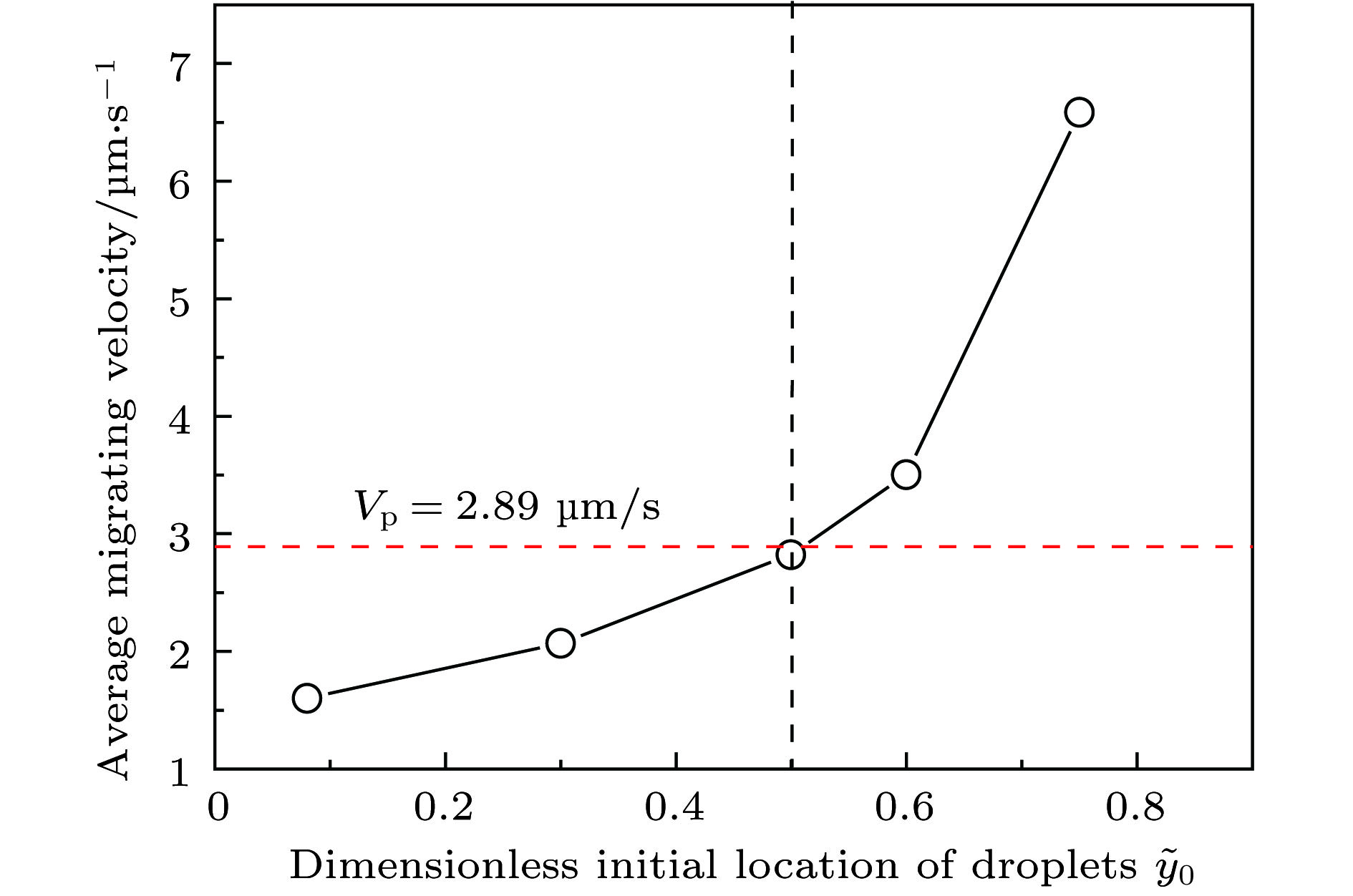
${\text{μ}}$ m/s和G = 12°C/mm条件下(相应的临界位置${\tilde y_{0, {\rm{cr}}}}$ = 0.5), 平均迁移速度随无量纲初始熔池位置的变化Fig. 7. CA simulated average migrating velocity as a function of the dimensionless initial liquid pool position for a SCN–0.3 wt.% ACE alloy at G = 12°C/mm and Vp = 2.89
${\text{μ}}$ m/s, corresponding to${\tilde y_{0, {\rm{cr}}}}$ = 0.5.
图 8 CA模拟的SCN–0.3 wt.% ACE合金在不同温度梯度条件下, 熔池迁移速度随时间的变化 (a)
$ V_{\rm p} = 0.6 \;{\text{μ}}{\rm m/s}$ ,$ {\tilde y_0}$ = 0.3, Vp < Vp, cr; (b)$ V_{\rm p} = 5{\text{μ}}{\rm m/s}$ ,$ {\tilde y_0} $ = 0.7, Vp > Vp, crFig. 8. CA simulated time evolution of liquid pool velocity for a SCN–0.3 wt.% ACE alloy at different temperature gradients and (a)
$ V_{\rm p} = 0.6{\text{μ}}{\rm m/s}$ ,$ {\tilde y_0}$ = 0.3, Vp < Vp, cr; (b)$ V_{\rm p} = 5{\text{μ}}{\rm m/s}$ ,${\tilde y_0}$ = 0.7, Vp > Vp, cr.
图 9 CA模拟的不同成分SCN–ACE合金熔池在G = 14°C/mm时迁移速度随时间的变化 (a)
${V_{\rm p} = 0.2 \;{\text{μ}}{\rm m/s}}$ ,${\tilde y_0}$ = 0.4, Vp < Vp, cr; (b)$ V_{\rm p} = 6\;{\text{μ}}{\rm m/s}$ ,${\tilde y_0}$ = 0.7, Vp > Vp, crFig. 9. CA simulated time evolution of liquid pool velocity for SCN-ACE alloys at G = 14°C/mm, different compositions and (a)
$ V_{\rm p} = 0.2 \;{\text{μ}}{\rm m/s}$ ,${\tilde y_0}$ = 0.4, Vp < Vp, cr; (b)$ V_{\rm p} = 6\;{\text{μ}}{\rm m/s}$ ,${\tilde y_0}$ = 0.7, Vp > Vp, cr.符号 物理意义 数值 Dl/m2·s–1 溶质在液相中的扩散系数 1 × 10–9 k 平衡分配系数 0.1 ml/K·(wt.%)–1 液相线斜率 –2.8 Tm/°C 纯SCN的熔点 58.081 
下载: 导出CSV
-
[1] Rettenmayr M 2009 Int. Mater. Rev. 54 1
Google Scholar
[2] Pfann W G 1955 Trans. AIME 203 961
[3] Tiller W A 1963 J. Appl. Phys. 34 2757
Google Scholar
[4] Allen D J, Hunt J D 1976 Metall. Mater. Trans. A 7 767
Google Scholar
[5] Kinoshita K, Arai Y, Inatomi Y, Tsukada T, Miyata H, Tanaka R 2016 J. Cryst. Growth 455 49
Google Scholar
[6] Wang H, Li S, Li X, Zhong H 2017 J. Cryst. Growth 466 56
Google Scholar
[7] Ma X, Yoshikawa T, Morita K 2014 Sci. Adv. Mater. 6 1697
Google Scholar
[8] Shirzadi A A, Wallach E R 1999 Acta Mater. 47 3551
Google Scholar
[9] Nguyen-Thi H, Reinhart G, Buffet A, Schenk T, Mangelinck-Noel N, Jung H, Bergeon N, Billia B, Hartwig J, Baruchel J 2008 J. Cryst. Growth 310 2906
Google Scholar
[10] Buchmann M, Rettenmayr M 2005 J. Cryst. Growth 284 544
Google Scholar
[11] Bösenberg U, Buchmann M, Rettenmayr M 2007 J. Cryst. Growth 304 281
Google Scholar
[12] Löffler A, Reuther K, Engelhardt H, Liu D, Rettenmayr M 2015 Acta Mater. 91 34
Google Scholar
[13] Liu D M, Li X Z, Su Y Q, Peng P, Luo L S, Guo J J, Fu H Z 2012 Acta Mater. 60 2679
Google Scholar
[14] Liu D M, Li X Z, Su Y Q, Rettenmayr M, Guo J J, Fu H Z 2014 Appl. Phys. A 116 1821
Google Scholar
[15] Pan S Y, Zhang Q Y, Zhu M F, Rettenmayr M 2015 Acta Mater. 86 229
Google Scholar
[16] 朱鸣芳, 汤倩玉, 张庆宇, 潘诗琰, 孙东科 2016 金属学报 52 1297
Google Scholar
Zhu M F, Tang Q Y, Zhang Q Y, Pan S Y, Sun D K 2016 Acta Metall. Sin. 52 1297
Google Scholar
[17] 陈瑞, 许庆彦, 柳百成 2014 63 188102
Google Scholar
Chen R, Xu Q Y, Liu B C 2014 Acta Phys. Sin . 63 188102
Google Scholar
[18] 张云鹏, 林鑫, 魏雷, 王猛, 彭东剑, 黄卫东 2012 61 228106
Google Scholar
Zhang Y P, Lin X, Wei L, Wang M, Peng D J, Huang W D 2012 Acta Phys. Sin. 61 228106
Google Scholar
[19] 江鸿翔, 赵九洲 2011 金属学报 47 1099
Jiang H X, Zhao J Z 2011 Acta Metall. Sin. 47 1099
[20] Zhu M F, Stefanescu D M 2007 Acta Mater. 55 1741
Google Scholar
[21] Pan S, Zhu M 2010 Acta Mater. 58 340
Google Scholar
[22] 李强, 李殿中, 钱百年 2004 53 3477
Google Scholar
Li Q, Li D Z, Qian B N 2004 Acta Phys. Sin. 53 3477
Google Scholar
[23] Wei L, Lin X, Wang M, Huang W D 2015 Chin. Phys. B 24 078108
Google Scholar
[24] Zhang Q Y, Fang H, Xue H, Pan S Y, Rettenmayr M, Zhu M F 2017 Sci. Rep. 7 17809
Google Scholar
[25] 朱鸣芳, 邢丽科, 方辉, 张庆宇, 汤倩玉, 潘诗琰 2018 金属学报 54 789
Google Scholar
Zhu M F, Xing L K, Fang H, Zhang Q Y, Tang Q Y, Pan S Y 2018 Acta Metall. Sin. 54 789
Google Scholar
[26] Zhang Q Y, Xue H, Tang Q Y, Pan S Y, Rettenmayr M, Zhu M F 2018 Comput. Mater. Sci. 146 20
[27] Zhang Q Y, Fang H, Xue H, Tang Q Y, Pan S Y, Rettenmayr M, Zhu M F 2018 Scr. Mater. 151 28
Google Scholar
[28] Farup I, Drezet J M, Rappaz M 2001 Acta Mater. 49 1261
Google Scholar
下载:
计量
- 文章访问数: 7738
- PDF下载量: 74
- 被引次数: 0
