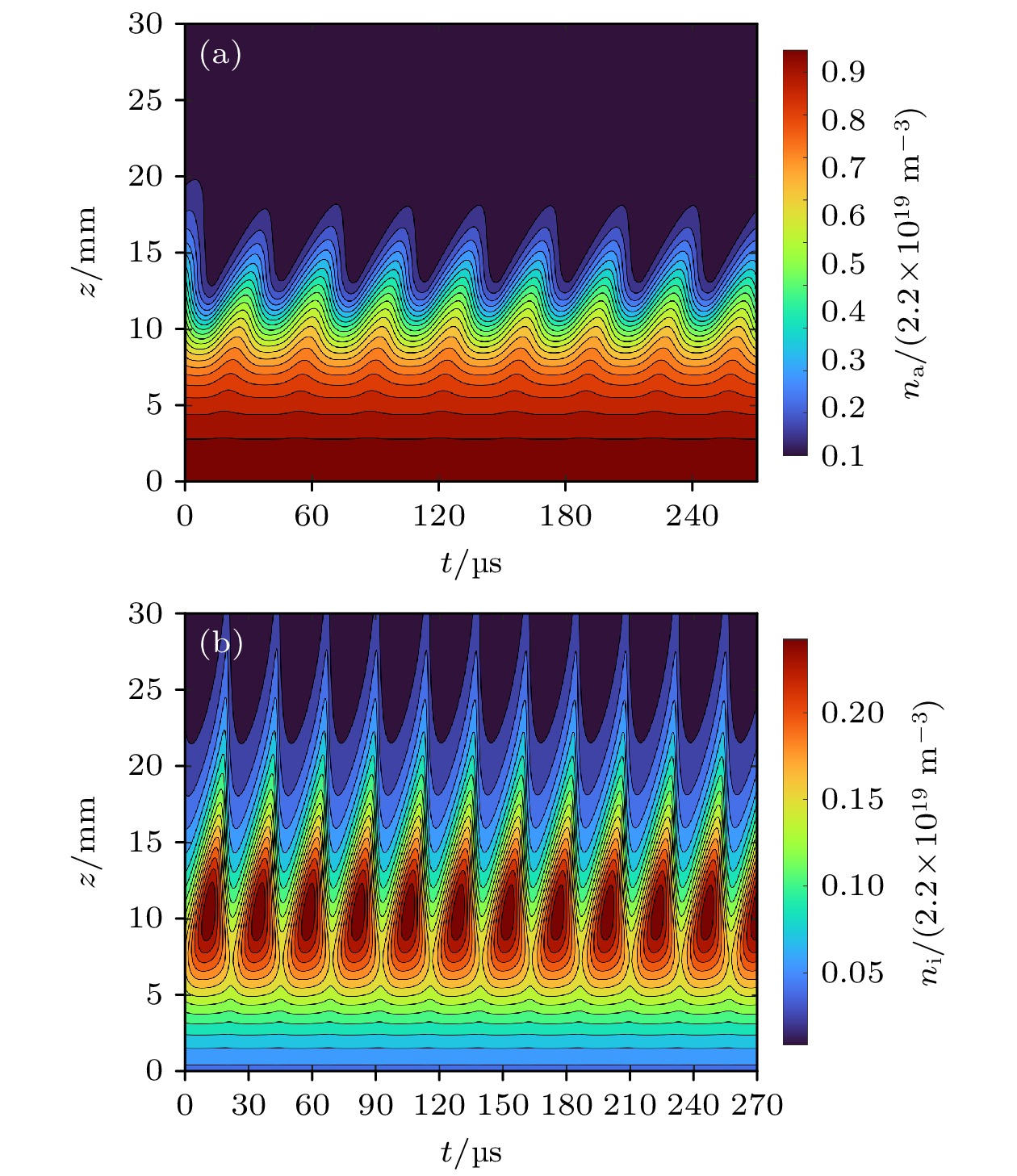
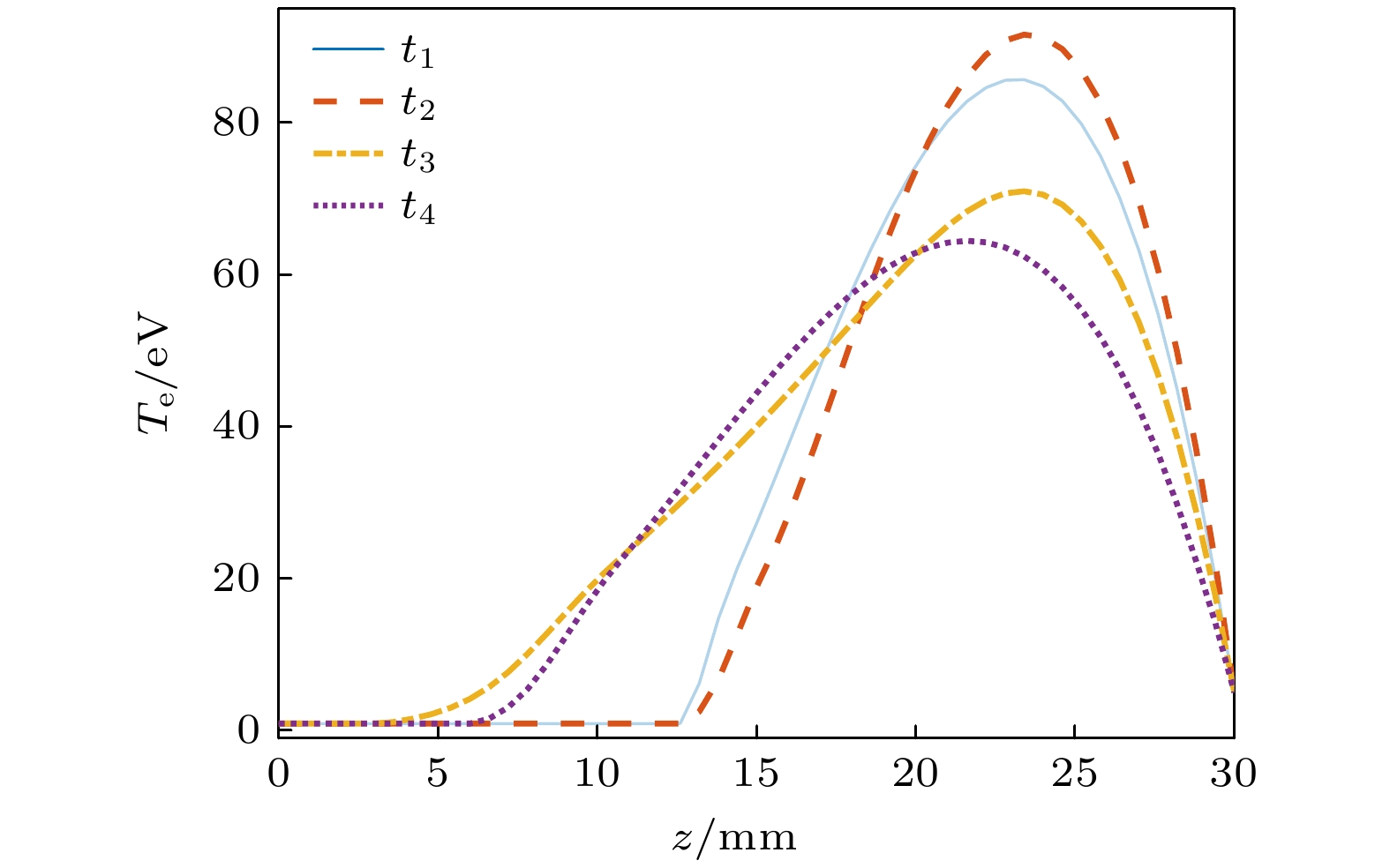
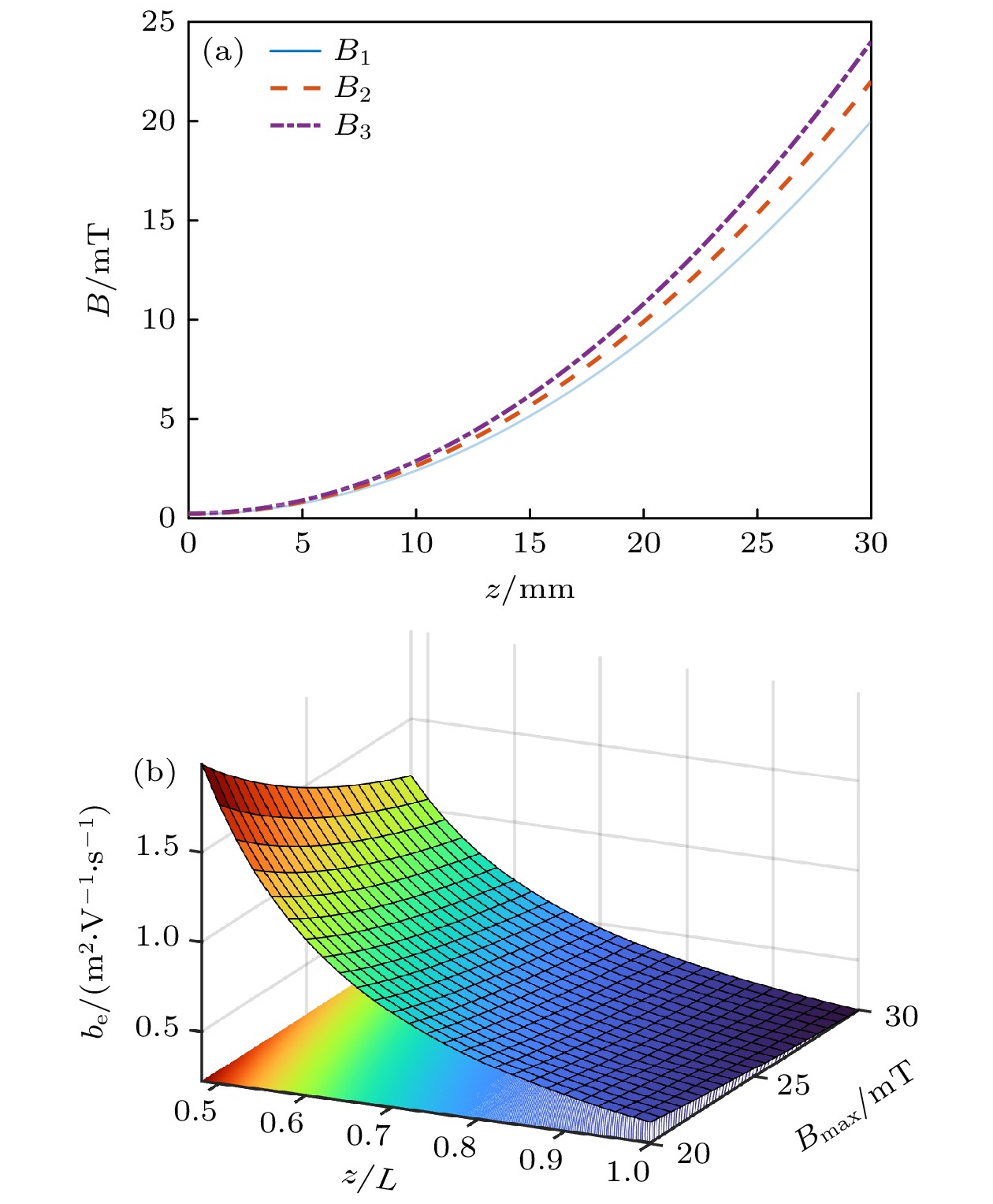
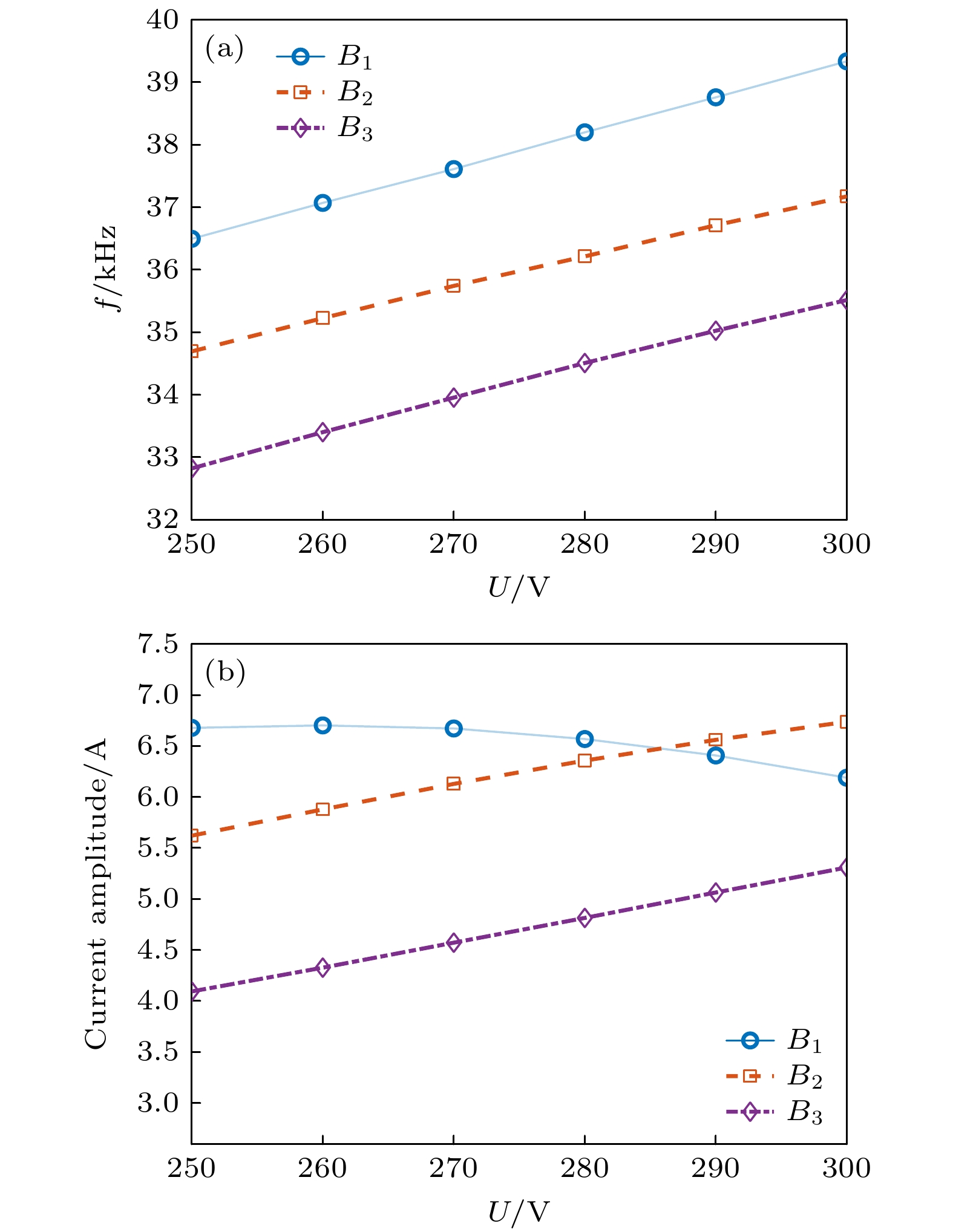
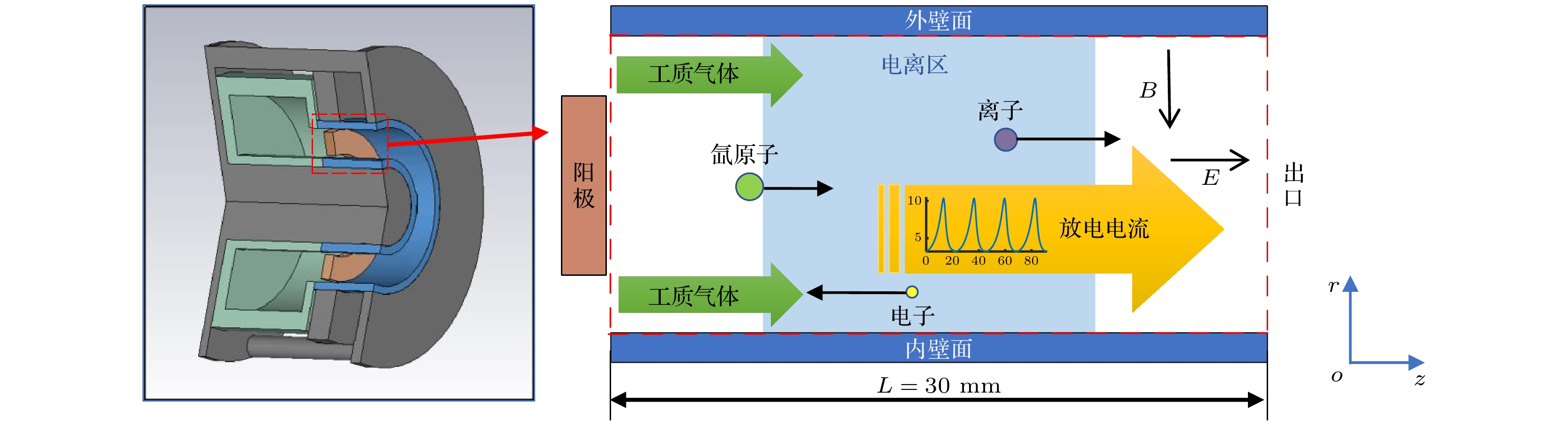
-
霍尔推力器放电通道低频振荡是影响其性能和稳定性的重要物理现象. 本文通过一维流体模型数值研究了霍尔推力器放电电流低频振荡特性及其抑制方法. 假设放电通道内满足准中性条件, 考虑电子与中性气体碰撞、电子反常传导及电子与壁面碰撞频率对电导率的影响, 研究了等离子体参数的变化以及磁场、放电电压、原子速度和预电离率对放电电流振荡的幅值及频率的影响. 结果表明, 在典型工况下, 放电通道内电流出现频率约为40 kHz的稳定周期性振荡; 随着通道内部磁场强度的增大, 电流振荡频率减小, 放电电流振幅在较高的磁场强度下随着电压的升高逐渐降低; 当预电离率升至4%以上时, 电流振幅随电压的升高逐渐降低; 通过降低原子速度, 升高预电离率, 可达到部分或完全抑制电流振荡的效果. 模拟结果表明, 通过提高通道磁场强度、降低放电电压、提高工质预电离率和调整进气方式以降低原子轴向速度等方式, 可有效减弱或完全抑制放电电流的低频振荡.Low frequency oscillation in the discharge channel of Hall thruster is an important physical phenomenon that affects its performance and stability. In this paper, the characteristics of low-frequency oscillation of the discharge current of Hall thruster and its suppression method are numerically studied by using a one-dimensional fluid model. Assuming that the discharge channel satisfies the quasi-neutral condition, the effects of electron-neutral collision, electron anomalous transport and electron-wall collision on conductivity are considered. The changes of plasma parameters and the effects of magnetic field, preionization rate, and atomic velocity on the amplitude and frequency of discharge current oscillation are also studied. Research results show that the variation of electron temperature in the discharge channel is closely related to the ionization process, and the electron temperature increases as the ionization intensity increases. The fluctuations in neutral gas flow rate and atomic density in the discharge process cause the ionization region to move forward and backward and the ionization intensity to change, which are the main driving forces for the low-frequency oscillation of discharge current in the channel. The magnetic field intensity in the discharge channel affects the axial current by influencing the electron mobility. With the increase of field strength, the oscillation frequency of current decreases, and under different magnetic field strengths, the current amplitude drops as the discharge voltage decreases. When the preionization rate of the working gas increases to above 4%, the amplitude of the discharge current oscillation gradually decreases. When the preionization rate is greater than 3% and the atomic velocity is less than 160 m/s, the discharge current oscillation in the channel exhibits damping attenuation, achieving a stabilizing effect which conduces to stabilizing the discharge of the Hall thruster.
-
Keywords:
- low-frequency oscillation /
- fluid simulation /
- discharge characteristics /
- preionization rate
[1] Morozov A I 2003 Plasma Phys. Rep. 29 235
Google Scholar
[2] 杨三祥, 王倩楠, 高俊, 贾艳辉, 耿海, 郭宁, 陈新伟, 袁兴龙, 张鹏 2022 71 105201
Google Scholar
Yang S X, Wang Q N, Gao J, Jia Y H, Geng H, Guo N, Chen X W, Yuan X L, Zhang P 2022 Acta Phys. Sin. 71 105201
Google Scholar
[3] 魏立秋, 李文博, 蔡海阔, 孙建宁, 杨鑫勇, 于达仁 2020 宇航学报 41 666
Google Scholar
Wei L Q, Li W B, Cai H K, Sun J N Yang X Y, Yu D R 2020 J. Astronaut. 41 666
Google Scholar
[4] Duan P, Bian X Y, Cao A N, Liu G R, Chen L, Yin Y 2016 Plasma Sci. Technol. 18 525
Google Scholar
[5] Duan P, Liu G R, Bian X Y, Chen L, Yin Y, Cao A N 2016 Plasma Sci. Technol. 18 382
Google Scholar
[6] Wei L Q, Li W B, Ding Y J, Yu D R 2018 Plasma Sci. Technol. 20 075502
Google Scholar
[7] 韩轲, 汪颖, 鲁海峰 2020 推进技术 41 1434
Google Scholar
Han K, Wang Y, Lu H F 2020 Journal of Propulsion Technology 41 1434
Google Scholar
[8] Wei L Q, Han L, Ding Y J, Yu D R, Zhang C H 2017 Rev. Sci. Instrum. 88 073502
Google Scholar
[9] Wang C, Wei L, Yu D 2011 Contrib. Plasma Phys. 51 981
Google Scholar
[10] Han L, Ding Y J, Wei L Q, Yu D R 2014 Rev. Sci. Instrum. 85 066113
Google Scholar
[11] Chauhan S, Ranjan M, Bandyopadhyay M, Mukherjee S 2016 Phys. Plasmas 23 123524
Google Scholar
[12] Yu D R, Wei L Q, Zhao Z Y, Han K, Yan G J 2008 Phys. Plasmas 15 043502
Google Scholar
[13] Wang C S, Wei L Q, Ning Z X, Yu D R 2011 Phys. Plasmas 18 013507
Google Scholar
[14] Fife J, Martinez-Sanchez M, Szabo J 1997 33rd Joint Propulsion Conference and Exhibit (Seattle: AIAA) p3052
[15] Morozov A I, Savel’ev V 2000 Plasma Phys. Rep. 26 219
Google Scholar
[16] Morozov A I, Savel’ev V 2000 Plasma Phys. Rep. 26 875
Google Scholar
[17] Boeuf J P, Garrigues L 1998 J. Appl. Phys. 84 3541
Google Scholar
[18] 张雯, 史锐, 费王华 2015 导弹与航天运载技术 4 99
Zhang W, Shi R, Fei W H 2015 Missiles Space Veh. 4 99
[19] Hara K, Mikellides I G 2018 Joint Propulsion Conference (Cincinnati: AIAA) p4904
[20] Wei L Q, Li W B, Ding Y J, Han L, Yu D R, Cao Y 2017 Eur. Phys. J. Plus 132 452
Google Scholar
[21] Lafleur T, Chabert P, Bourdon A 2021 J. Appl. Phys. 130 053305
Google Scholar
[22] Dorf L, Raitses Y, Fisch N J 2006 Phys. Plasmas 13 057104
Google Scholar
[23] Bareilles J, Hagelaar G J M, Garrigues L, Boniface C, Boeuf J P, Gascon N 2004 Phys. Plasmas 11 3035
Google Scholar
[24] Hara K 2015 Ph. D. Dissertation (Ann Arbor: University of Michigan)
[25] Yu D R, Wang C S, Wei L Q, Gao C, Yu G 2008 Phys. Plasmas 15 113503
Google Scholar
[26] Barral S, Ahedo E 2009 Phys. Rev. E 79 046401
Google Scholar
[27] Garrigues L, Heron A, Adam J C 2000 Plasma Sources Sci. Technol. 9 219
Google Scholar
[28] Chapurin O, Smolyakov A I, Hagelaar G, Raitses Y 2021 J. Appl. Phys. 129 233307
Google Scholar
[29] 王雨龙 2013 硕士学位论文 (哈尔滨: 哈尔滨工业大学)
Wang Y L 2013 M. S. Dissertation (Harbin: Harbin Institute of Technology) (in Chinese)
[30] Hara K, Sekerak M J, Boyd I D, Gallimore A D 2014 Phys. Plasmas 21 122103
Google Scholar
[31] Dale E T, Jorns B A 2019 Phys. Plasmas 26 013516
Google Scholar
[32] Hara K 2019 Plasma Sources Sci. Technol. 28 044001
Google Scholar
[33] Andreussi T, Giannetti V, Leporini A, Saravia M M, Andrenucci M 2017 Phys. Plasmas 60 014015
Google Scholar
[34] Lafleur T, Baalrud S D, Chabert P 2016 Phys. Plasmas 23 053503
Google Scholar
[35] 权路路, 邢伟, 鹿畅, 龚科瑜, 曹勇 2016 中国空间科学技术 36 51
Quan L L, Xing W, Lu C, Gong K Y, Cao Y 2016 Chin. Space Sci. Technol. 36 51
-
图 1 霍尔推力器典型立体结构及放电通道模拟区域示意图
Fig. 1. Typical 3D structure of a Hall thruster and schematic of the simulation region for discharge channels.
图 2 放电通道原子数密度和离子数密度分布 (a) 原子数密度; (b) 离子数密度
Fig. 2. Atomic number density and ion number density distribution in the discharge channel: (a) Atomic number density; (b) ion number density.
图 3 通道放电电流低频振荡变化规律
Fig. 3. Low frequency oscillation of the channel discharge current.
图 4 放电通道电子温度分布特性
Fig. 4. Electron temperature distribution through the discharge channel.
图 6 电子与壁面碰撞频率对电场分布的影响
Fig. 6. The influence of electron-wall collision frequency on the distribution of electric field.
图 7 通道轴向磁场强度分布及对电子迁移率的影响 (a)磁场强度在轴向上的分布; (b)磁场分布对轴向电子迁移率的影响
Fig. 7. The distributions of axial magnetic field intensity and their effects on electron mobility: (a) Distributions of axial magnetic field intensity; (b) effects of magnetic field on electron mobility.
图 8 不同磁场强度分布下放电电流频率和振幅随放电电压变化规律 (a)振荡频率变化规律; (b)振荡幅值变化规律
Fig. 8. The variation of discharge current frequency and amplitude with discharge voltage under different magnetic field intensity distribution: (a) Oscillation frequency variation; (b) oscillation amplitude variation.
图 9 不同预电离率下电流振荡振幅随通道电压变化规律
Fig. 9. The variation of current oscillation amplitude with channel voltage under different preionization rates.
图 10 通道原子速度对电流振荡和电离区位置的影响 (a)
${V_{\text{a}}} = 100 {\text{m}}/{\text{s}}$ 时电流振荡变化; (b)${V_{\text{a}}} = 150 {\text{m}}/{\text{s}}$ 时电流振荡随时演化; (c)${V_{\text{a}}} = 200 {\text{m}}/{\text{s}}$ 时电流振荡随时演化; (d) 3种原子速度下电离区中心位置随时演化Fig. 10. The influence of channel atomic velocity on current oscillation and ionization region position: (a) The evolution of current oscillation over time when
${V_{\text{a}}} = 100 {\text{m}}/{\text{s}}$ ; (b) the evolution of current oscillation over time when${V_{\text{a}}} = 150 {\text{m}}/{\text{s}}$ ; (c) the evolution of current oscillation over time when${V_{\text{a}}} = 200 {\text{m}}/{\text{s}}$ ; (d) the evolution of the ionization zone position under different atomic velocities.
图 11 不同预电离率和原子速度下的电流振幅分布特性
Fig. 11. The current amplitude distribution under different preionization rates and atomic velocities.
-
[1] Morozov A I 2003 Plasma Phys. Rep. 29 235
Google Scholar
[2] 杨三祥, 王倩楠, 高俊, 贾艳辉, 耿海, 郭宁, 陈新伟, 袁兴龙, 张鹏 2022 71 105201
Google Scholar
Yang S X, Wang Q N, Gao J, Jia Y H, Geng H, Guo N, Chen X W, Yuan X L, Zhang P 2022 Acta Phys. Sin. 71 105201
Google Scholar
[3] 魏立秋, 李文博, 蔡海阔, 孙建宁, 杨鑫勇, 于达仁 2020 宇航学报 41 666
Google Scholar
Wei L Q, Li W B, Cai H K, Sun J N Yang X Y, Yu D R 2020 J. Astronaut. 41 666
Google Scholar
[4] Duan P, Bian X Y, Cao A N, Liu G R, Chen L, Yin Y 2016 Plasma Sci. Technol. 18 525
Google Scholar
[5] Duan P, Liu G R, Bian X Y, Chen L, Yin Y, Cao A N 2016 Plasma Sci. Technol. 18 382
Google Scholar
[6] Wei L Q, Li W B, Ding Y J, Yu D R 2018 Plasma Sci. Technol. 20 075502
Google Scholar
[7] 韩轲, 汪颖, 鲁海峰 2020 推进技术 41 1434
Google Scholar
Han K, Wang Y, Lu H F 2020 Journal of Propulsion Technology 41 1434
Google Scholar
[8] Wei L Q, Han L, Ding Y J, Yu D R, Zhang C H 2017 Rev. Sci. Instrum. 88 073502
Google Scholar
[9] Wang C, Wei L, Yu D 2011 Contrib. Plasma Phys. 51 981
Google Scholar
[10] Han L, Ding Y J, Wei L Q, Yu D R 2014 Rev. Sci. Instrum. 85 066113
Google Scholar
[11] Chauhan S, Ranjan M, Bandyopadhyay M, Mukherjee S 2016 Phys. Plasmas 23 123524
Google Scholar
[12] Yu D R, Wei L Q, Zhao Z Y, Han K, Yan G J 2008 Phys. Plasmas 15 043502
Google Scholar
[13] Wang C S, Wei L Q, Ning Z X, Yu D R 2011 Phys. Plasmas 18 013507
Google Scholar
[14] Fife J, Martinez-Sanchez M, Szabo J 1997 33rd Joint Propulsion Conference and Exhibit (Seattle: AIAA) p3052
[15] Morozov A I, Savel’ev V 2000 Plasma Phys. Rep. 26 219
Google Scholar
[16] Morozov A I, Savel’ev V 2000 Plasma Phys. Rep. 26 875
Google Scholar
[17] Boeuf J P, Garrigues L 1998 J. Appl. Phys. 84 3541
Google Scholar
[18] 张雯, 史锐, 费王华 2015 导弹与航天运载技术 4 99
Zhang W, Shi R, Fei W H 2015 Missiles Space Veh. 4 99
[19] Hara K, Mikellides I G 2018 Joint Propulsion Conference (Cincinnati: AIAA) p4904
[20] Wei L Q, Li W B, Ding Y J, Han L, Yu D R, Cao Y 2017 Eur. Phys. J. Plus 132 452
Google Scholar
[21] Lafleur T, Chabert P, Bourdon A 2021 J. Appl. Phys. 130 053305
Google Scholar
[22] Dorf L, Raitses Y, Fisch N J 2006 Phys. Plasmas 13 057104
Google Scholar
[23] Bareilles J, Hagelaar G J M, Garrigues L, Boniface C, Boeuf J P, Gascon N 2004 Phys. Plasmas 11 3035
Google Scholar
[24] Hara K 2015 Ph. D. Dissertation (Ann Arbor: University of Michigan)
[25] Yu D R, Wang C S, Wei L Q, Gao C, Yu G 2008 Phys. Plasmas 15 113503
Google Scholar
[26] Barral S, Ahedo E 2009 Phys. Rev. E 79 046401
Google Scholar
[27] Garrigues L, Heron A, Adam J C 2000 Plasma Sources Sci. Technol. 9 219
Google Scholar
[28] Chapurin O, Smolyakov A I, Hagelaar G, Raitses Y 2021 J. Appl. Phys. 129 233307
Google Scholar
[29] 王雨龙 2013 硕士学位论文 (哈尔滨: 哈尔滨工业大学)
Wang Y L 2013 M. S. Dissertation (Harbin: Harbin Institute of Technology) (in Chinese)
[30] Hara K, Sekerak M J, Boyd I D, Gallimore A D 2014 Phys. Plasmas 21 122103
Google Scholar
[31] Dale E T, Jorns B A 2019 Phys. Plasmas 26 013516
Google Scholar
[32] Hara K 2019 Plasma Sources Sci. Technol. 28 044001
Google Scholar
[33] Andreussi T, Giannetti V, Leporini A, Saravia M M, Andrenucci M 2017 Phys. Plasmas 60 014015
Google Scholar
[34] Lafleur T, Baalrud S D, Chabert P 2016 Phys. Plasmas 23 053503
Google Scholar
[35] 权路路, 邢伟, 鹿畅, 龚科瑜, 曹勇 2016 中国空间科学技术 36 51
Quan L L, Xing W, Lu C, Gong K Y, Cao Y 2016 Chin. Space Sci. Technol. 36 51
下载:
计量
- 文章访问数: 2608
- PDF下载量: 64
- 被引次数: 0
