-
研究了三元过冷熔体中柱状晶体在非等温条件下受straining流作用的生长问题, 给出了柱状晶体生长形态的近似解析表达式. 发现流入的straining流加快了界面的生长速度, 而流出的straining流减缓了界面的生长速度, 即straining流使得柱状晶体的界面发生变形. 同时发现, 随着流动速度的增大, 界面变形也更为显著. 通过比较straining流对纯熔体、二元熔体、三元熔体中柱状晶体界面的影响, 发现相比于纯熔体, 柱状晶体在稀合金熔体中的界面形态受straining流的影响更大.
-
关键词:
- 柱状晶体 /
- 三元熔体 /
- straining流 /
- 界面形态
As an important microstructure, columnar crystal growth technology, especially the growth technology of single columnar crystal plays an important role in improving the performances of semiconductor, optical devices and other related products. In many practical applications, because the alloy is composed of multi-component and there is inevitably flow in the melt, it is necessary to study the growth of columnar crystals in multi-component melt with flow separately. The growth of columnar crystal in a ternary undercooled melt subjected to straining flow under non-isothermal conditions is studied, and the approximate analytical expression for growth morphology of columnar crystal is given by using asymptotic method. It can be seen from the expression that straining flow is an important reason for irregular columnar crystal. When analyzing the effect of straining flow on the growth of columnar crystal in ternary melt, it is found that the incoming flow accelerates the growth velocity of the interface, while the outgoing straining flow reduces the growth velocity of the interface, namely, the straining flow makes the interface of columnar crystal deformed. At the same time, it is found that the interface deformation becomes more intense with the increase of flow velocity. The above conclusion can also be applied to the effect of straining flow on the interface morphology of columnar crystal in pure melt and binary melt. The comparison of the effects of straining flow on the interface of columnar crystal among pure melt, binary melt and ternary melt, shows that the interface morphology of columnar crystal in dilute alloy melt is more affected by straining flow than in the pure melt, but the more components are more easily affected by flow. However, the number of components in melt is not a decisive factor for the change of interface morphology of the columnar crystal, but the constitutional undercooling is an important factor for determining the interface morphology of multicomponent alloy. According to the conclusion of this paper, the influence of straining flow on the interface morphology of columnar crystal growth can be quantitatively predicted, which provides the necessary theoretical guidance in accurately controlling the interface morphology in the future.-
Keywords:
- columnar crystal /
- ternary melt /
- straining flow /
- interfacial morphology
[1] Mullins W W, Sekerka R F 1963 J. Appl. Phys. 34 323
Google Scholar
[2] Flood S C, Hunt J D 1987 J. Cryst. Growth 82 543
Google Scholar
[3] Libbrecht K G, Yu H 2001 J. Cryst. Growth 222 822
Google Scholar
[4] Ares A E, Gueijman S F, Schvezov C E 2002 J. Cryst. Growth 241 235
Google Scholar
[5] Viardin A, Založnik M, Souhar Y, Apel M, Combeau H 2017 Acta Mater. 122 386
Google Scholar
[6] Wang L, Wang N, Provatas N 2017 Acta Mater. 126 302
Google Scholar
[7] Debroy P P, Sekerka R F 1996 Phys. Rev. E 53 6244
Google Scholar
[8] Ren S, Li P, Jiang D, Tan Y, Li J, Zhang L 2016 Appl. Therm. Eng. 106 875
Google Scholar
[9] Lü C, Ai Y, Yu Q, Chen W, He W, Zhang J, Min X 2019 J. Cryst. Growth 507 395
Google Scholar
[10] Battaglioli S, Robinson A J, McFadden S 2018 Int. J. Heat Mass Tran. 126 66
Google Scholar
[11] Buchholz A, Engler S 1996 Comput. Mater. Sci. 7 221
Google Scholar
[12] Lee S Y, Lee S M, Hong C P 2000 ISIJ Int. 40 48
Google Scholar
[13] Coriell S R, Parker R L 1965 J. Appl. Phys. 36 632
Google Scholar
[14] 陈亚军, 陈琦, 王自东, 胡汉起, 刘玉敏, 连玉栋 2004 清华大学学报(自然科学版) 44 1464
Google Scholar
Chen Y J, Chen Q, Wang Z D, Hu H Q, Liu Y M, Lian Y D 2004 Tsinghua Sci. Technol. 44 1464
Google Scholar
[15] Du L, Zhang P, Yang S, Chen J, Du H 2018 Mod. Phys. Lett. B 32 1850078
[16] Murakami K, Aihara H, Okamoto T 1984 Acta Metall. 32 933
Google Scholar
[17] Szajnar J 2004 J. Mater. Process. Technol. 157 761
[18] Altieri A L, Davis S H 2017 J. Cryst. Growth 467 162
Google Scholar
[19] Colin J 2018 J. Cryst. Growth 493 76
Google Scholar
[20] Vogel A, Cantor B 1977 J. Cryst. Growth 37 309
Google Scholar
[21] Fan H L, Chen M W, Shan Y Y 2019 Surf. Rev. Lett. 11 1950170
-
图 1 在
$Oxy$ 平面上应变流对柱状晶体形态演化的影响, 其中$t = 396, $ $\varGamma = 0.25, $ $M_{\rm{C}}^1=0.01, $ $M_{\rm{C}}^2=0.02, $ $C_{{\rm{L}}, \infty }^1 = $ 1.0,$C_{{\rm{L}}, \infty }^2 = 3.0, $ ${A_\lambda } = 3.3, $ ${m_1} = - 1.6, $ ${m_2} = $ –2.33,$E = 0.3, $ ${M_{\rm{k}}} = 0.01, $ ${k_{\rm{T}}} = 1.23, $ ${\lambda _{\rm{S}}} = 0.01, $ $\lambda _{\rm{D}}^1 = $ 0.01,$\lambda _{\rm{D}}^2 = 0.02, $ $\varepsilon = 0.05$ Fig. 1. The morphology evolution of columnar crystal in a straining flow on the cross-section of
$Oxy$ plane at$t = 396, $ where$\varGamma = 0.25, $ $M_{\rm{C}}^1=0.01, $ $M_{\rm{C}}^2=0.02, $ $C_{{\rm{L}}, \infty }^1 = 1.0, $ $C_{{\rm{L}}, \infty }^2 = 3.0, $ ${A_\lambda } = 3.3, $ ${m_1} = - 1.6, $ ${m_2} = - 2.33, $ E = 0.3,${M_{\rm{k}}} = 0.01, $ ${k_{\rm{T}}} = 1.23, $ ${\lambda _{\rm{S}}} = 0.01, $ $\lambda _{\rm{D}}^1 = 0.01, $ $\lambda _{\rm{D}}^2 = $ 0.02,$ \varepsilon = 0.05$ 
图 2 在
$t = 396$ 时, 柱状晶体的界面形态. 其中$\varGamma = 0.25, $ $M_{\rm{C}}^{\rm{1}}=0.01, $ $M_{\rm{C}}^{\rm{2}}=0.02, $ $C_{{\rm{L}}, \infty }^1 = 1.0, $ $C_{{\rm{L}}, \infty }^2 = 3.0, $ ${A_\lambda } = 3.3, $ ${m_1} = - 1.6, $ ${m_2} = - 2.33, $ $E = 0.3, $ ${M_{\rm{k}}} = 0.01, $ ${k_{\rm{T}}} = 1.23, $ ${\lambda _{\rm{S}}} = 0.01, $ $\lambda _{\rm{D}}^1 = 0.01, $ $\lambda _{\rm{D}}^{\rm{2}} = 0.02, $ $\varepsilon = 0.05, $ $A = 0.9$ Fig. 2. The morphology evolution of columnar crystal in a straining flow at
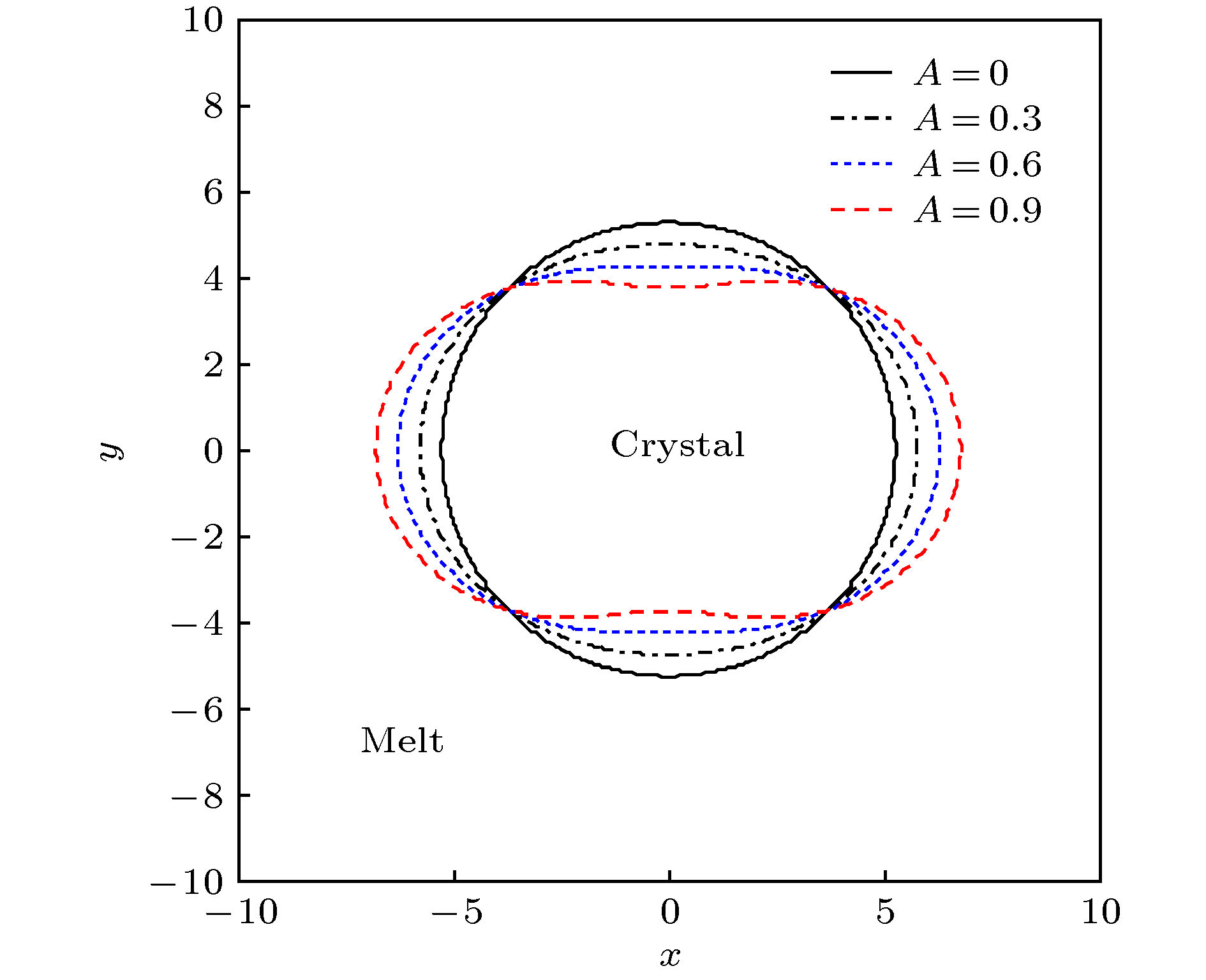
$t = 396, $ where$\varGamma = 0.25, $ $M_{\rm{C}}^{\rm{1}}=0.01, $ $M_{\rm{C}}^{\rm{2}}=0.02, $ $C_{{\rm{L}}, \infty }^1 = 1.0, $ $C_{{\rm{L}}, \infty }^2 = 3.0, $ ${A_\lambda } = 3.3, $ ${m_1} = - 1.6, $ ${m_2} = - 2.33, $ $E = 0.3, $ ${M_{\rm{k}}} = 0.01, $ ${k_{\rm{T}}} = 1.23, $ ${\lambda _{\rm{S}}} = 0.01, $ $\lambda _{\rm{D}}^1 = 0.01, $ $\lambda _{\rm{D}}^{\rm{2}} = 0.02, $ $\varepsilon = 0.05, $ $A = 0.9$ 
图 3 不同强度的应变流对柱状晶体界面形态的影响, 其中
$t \!=\! 256, $ $\varGamma \!=\! 0.25, $ $M_{\rm{C}}^1 \!=\! 0.01, $ $M_{\rm{C}}^2\! =\! 0.02, $ $C_{{\rm{L}}, \infty }^1 = 1.0, $ $C_{{\rm{L}}, \infty }^2 = 3.0, $ ${A_\lambda } = 3.3, $ ${m_1} = - 1.6, $ ${m_2} = - 2.33, $ $E = 0.3, $ ${M_{\rm{k}}} = 0.01, $ ${k_{\rm{T}}} = 1.23, $ ${\lambda _{\rm{S}}} = 0.01, $ $\lambda _{\rm{D}}^1 = 0.01, $ $\lambda _{\rm{D}}^{\rm{2}} = 0.02, $ $\varepsilon = 0.05.$ A由左向右分别为0.9, 0.6, 0.3, 0Fig. 3. Interface morphology of columnar crystals affected by different sizes of straining flow, where
$t = 256, $ $\varGamma = 0.25, $ $M_{\rm{C}}^1=0.01, $ $M_{\rm{C}}^2=0.02, $ $C_{{\rm{L}}, \infty }^1 = 1.0, $ $C_{{\rm{L}}, \infty }^2 = 3.0, $ ${A_\lambda } = 3.3, $ ${m_1} = - 1.6, $ ${m_2} = - 2.33, $ $E = 0.3, $ ${M_{\rm{k}}} = 0.01, $ ${k_{\rm{T}}} = 1.23, $ ${\lambda _{\rm{S}}} = 0.01, $ $\lambda _{\rm{D}}^1 = 0.01, $ $\lambda _{\rm{D}}^{\rm{2}} = 0.02, $ $\varepsilon = 0.05.$ A is 0.9, 0.6, 0.3, 0 from left to right, respectively.
图 4 在
$Oxy$ 平面上柱状晶体界面随时间的演化, 其中$\varGamma \!=\! 0.25, $ $M_{\rm{C}}^1 \!=\! 0.01, $ $M_{\rm{C}}^{\rm{2}} \!=\! 0.02, $ $C_{{\rm{L}}, \infty }^1 \!=\! 1.0, $ $C_{{\rm{L}}, \infty }^2 \!=\! 3.0, $ ${A_\lambda } = 3.3, $ ${m_1} = - 1.6, $ ${m_2} = - 2.33, $ E = 0.3, Mk = 0.01, kT = 1.23,${\lambda _{\rm{S}}} = 0.01, $ $\lambda _{\rm{D}}^{\rm{1}} = 0.01, $ $\lambda _{\rm{D}}^{\rm{2}} = 0.02, $ $\varepsilon = 0.05, $ $A = 0.9$ Fig. 4. Evolution of columnar crystal interface with time in the
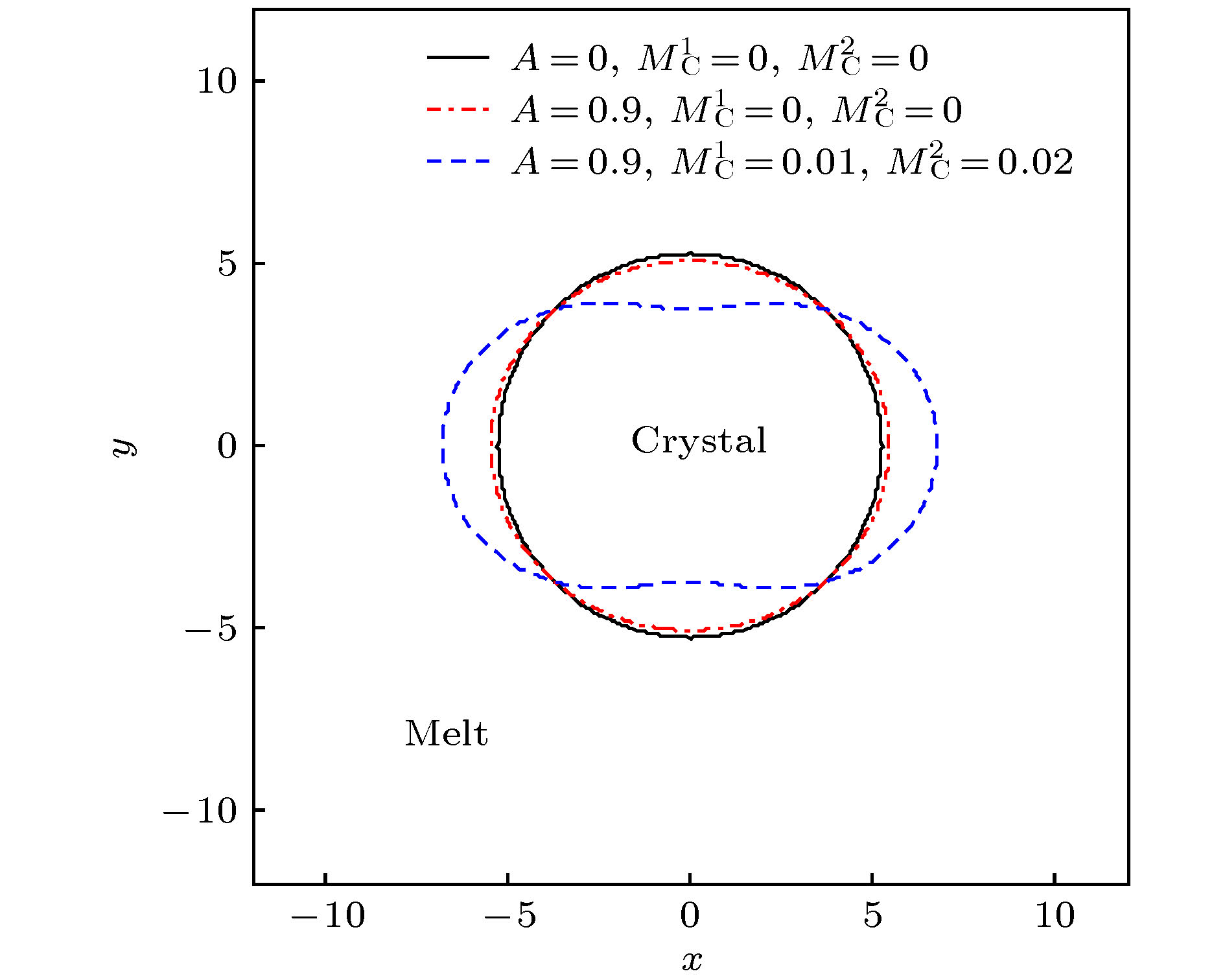
$Oxy$ plane, where$\varGamma = 0.25, $ $M_{\rm{C}}^1=0.01, $ $M_{\rm{C}}^{\rm{2}}=0.02, $ $C_{{\rm{L}}, \infty }^1 = 1.0, $ $C_{{\rm{L}}, \infty }^2 = 3.0, $ ${A_\lambda } = 3.3, $ ${m_1} = - 1.6, $ m2 = –2.33, E = 0.3, Mk = 0.01, kT = 1.23,${\lambda _{\rm{S}}} = 0.01, $ $\lambda _{\rm{D}}^{\rm{1}} = 0.01, $ $\lambda _{\rm{D}}^{\rm{2}} = 0.02, $ $\varepsilon = 0.05, $ $A = 0.9$ 
图 5 应变流对不同杂质含量柱状晶体界面形态的影响, 其中
$t = 256, $ $\varGamma = 0.25, $ $C_{{\rm{L}}, \infty }^1 = 1.0, $ $C_{{\rm{L}}, \infty }^2 = 3.0, $ ${A_\lambda } = 3.3, $ ${m_1} = - 1.6, $ ${m_2} = - 2.33, $ $E = 0.3, $ ${M_{\rm{k}}} = 0.01, $ ${k_{\rm{T}}} = 1.23, $ ${\lambda _{\rm{S}}} = 0.01, $ $\lambda _{\rm{D}}^{\rm{1}} = 0.01, $ $\lambda _{\rm{D}}^{\rm{2}} = 0.02, $ $\varepsilon = 0.05$ Fig. 5. Effect of straining flow on the interface morphology of columnar crystals in different impurity content, where
$t = 256, $ $\varGamma = 0.25, $ $C_{{\rm{L}}, \infty }^1 = 1.0, $ $C_{{\rm{L}}, \infty }^2 = 3.0, $ ${A_\lambda } = 3.3, $ ${m_1} = - 1.6, $ ${m_2} = - 2.33, $ $E = 0.3, $ ${M_{\rm{k}}} = 0.01, $ ${k_{\rm{T}}} = 1.23, $ ${\lambda _{\rm{S}}} = 0.01, $ $\lambda _{\rm{D}}^{\rm{1}} = 0.01, $ $\lambda _{\rm{D}}^{\rm{2}} = 0.02, $ $\varepsilon = 0.05$ 
图 6 应变流对不同杂质含量柱状晶体界面形态的影响, 其中
$t = 256, $ $\varGamma = 0.25, $ $C_{{\rm{L}}, \infty }^1 = 1.0, $ $C_{{\rm{L}}, \infty }^2 = 3.0, $ ${A_\lambda } = 3.3, $ ${m_1} = - 1.6, $ ${m_2} = - 2.33, $ $E = 0.3, $ ${M_{\rm{k}}} = 0.01, $ ${k_{\rm{T}}} = 1.23, $ ${\lambda _{\rm{S}}} = 0.01, $ $\lambda _{\rm{D}}^{\rm{1}} = 0.01, $ $\lambda _{\rm{D}}^{\rm{2}} = 0.02, $ $\varepsilon = 0.05$ Fig. 6. Effect of straining flow on the interface morphology of columnar crystals in different impurity content, where
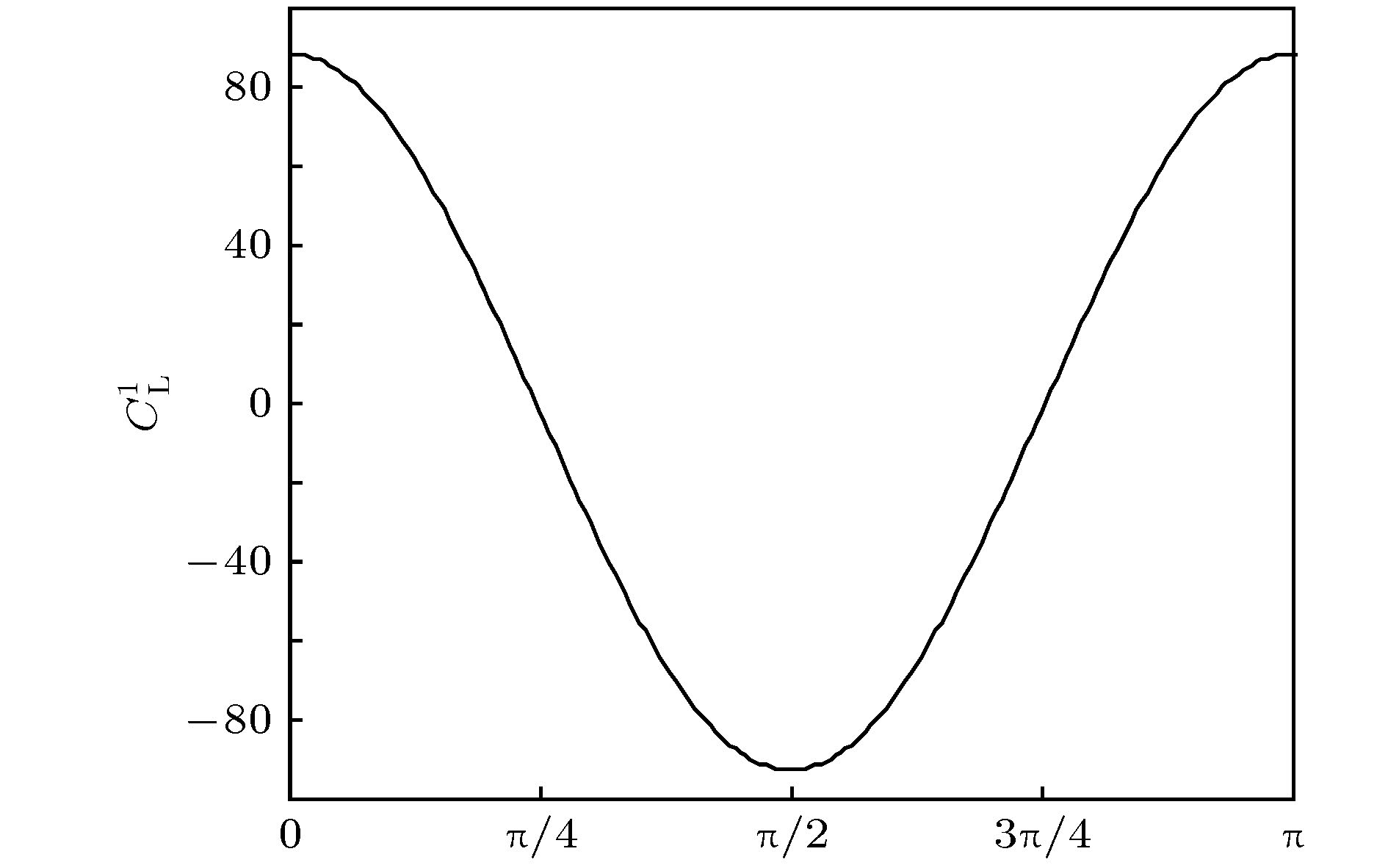
$t = 256, $ $\varGamma = 0.25, $ $C_{{\rm{L}}, \infty }^1 = 1.0, $ $C_{{\rm{L}}, \infty }^2 = 3.0, $ ${A_\lambda } = 3.3, $ ${m_1} = - 1.6, $ ${m_2} = - 2.33, $ $E = 0.3, $ ${M_{\rm{k}}} = 0.01, $ ${k_{\rm{T}}} = 1.23, $ ${\lambda _{\rm{S}}} = 0.01, $ $\lambda _{\rm{D}}^{\rm{1}} = 0.01, $ $\lambda _{\rm{D}}^{\rm{2}} = 0.02, $ $\varepsilon = 0.05$ 
图 7 在
$R, \theta $ 平面上, 柱状晶体界面杂质浓度$C_{\rm{L}}^1$ 随$\theta $ 的变化情况, 其中$t = 256, $ $\varGamma = 0.25, $ $M_{\rm{C}}^{\rm{1}}=0.01, $ $M_{\rm{C}}^{\rm{2}}=0.02, $ $C_{{\rm{L}}, \infty }^1 = 1.0, $ $C_{{\rm{L}}, \infty }^2 = 3.0, $ ${A_\lambda } = 3.3, $ ${m_1} = - 1.6, $ ${m_2} = - 2.33, $ $E = 0.3, $ ${M_{\rm{k}}} = 0.01, $ ${k_{\rm{T}}} = 1.23, $ ${\lambda _{\rm{S}}} = 0.01, $ $\lambda _{\rm{D}}^{\rm{1}} = 0.01, $ $\lambda _{\rm{D}}^{\rm{2}}=0.02, $ $\varepsilon = 0.05, $ $A = 0.9$ Fig. 7. The change of impurity concentration at the interface of columnar crystal in the
$R, \theta $ plane, where$t = 256, $ $\varGamma = 0.25, $ $M_{\rm{C}}^{\rm{1}}=0.01, $ $M_{\rm{C}}^{\rm{2}}=0.02, $ $C_{{\rm{L}}, \infty }^2 = 3.0, $ ${A_\lambda } = 3.3, $ ${m_1} = - 1.6, $ ${m_2} = $ –2.33$E = 0.3, $ ${M_{\rm{k}}} = 0.01, $ ${k_{\rm{T}}} = 1.23, $ ${\lambda _{\rm{S}}} = 0.01, $ $\lambda _{\rm{D}}^{\rm{1}} = 0.01, $ $\lambda _{\rm{D}}^{\rm{2}}=0.02, $ $\varepsilon = 0.05, $ $A = 0.9$ -
[1] Mullins W W, Sekerka R F 1963 J. Appl. Phys. 34 323
Google Scholar
[2] Flood S C, Hunt J D 1987 J. Cryst. Growth 82 543
Google Scholar
[3] Libbrecht K G, Yu H 2001 J. Cryst. Growth 222 822
Google Scholar
[4] Ares A E, Gueijman S F, Schvezov C E 2002 J. Cryst. Growth 241 235
Google Scholar
[5] Viardin A, Založnik M, Souhar Y, Apel M, Combeau H 2017 Acta Mater. 122 386
Google Scholar
[6] Wang L, Wang N, Provatas N 2017 Acta Mater. 126 302
Google Scholar
[7] Debroy P P, Sekerka R F 1996 Phys. Rev. E 53 6244
Google Scholar
[8] Ren S, Li P, Jiang D, Tan Y, Li J, Zhang L 2016 Appl. Therm. Eng. 106 875
Google Scholar
[9] Lü C, Ai Y, Yu Q, Chen W, He W, Zhang J, Min X 2019 J. Cryst. Growth 507 395
Google Scholar
[10] Battaglioli S, Robinson A J, McFadden S 2018 Int. J. Heat Mass Tran. 126 66
Google Scholar
[11] Buchholz A, Engler S 1996 Comput. Mater. Sci. 7 221
Google Scholar
[12] Lee S Y, Lee S M, Hong C P 2000 ISIJ Int. 40 48
Google Scholar
[13] Coriell S R, Parker R L 1965 J. Appl. Phys. 36 632
Google Scholar
[14] 陈亚军, 陈琦, 王自东, 胡汉起, 刘玉敏, 连玉栋 2004 清华大学学报(自然科学版) 44 1464
Google Scholar
Chen Y J, Chen Q, Wang Z D, Hu H Q, Liu Y M, Lian Y D 2004 Tsinghua Sci. Technol. 44 1464
Google Scholar
[15] Du L, Zhang P, Yang S, Chen J, Du H 2018 Mod. Phys. Lett. B 32 1850078
[16] Murakami K, Aihara H, Okamoto T 1984 Acta Metall. 32 933
Google Scholar
[17] Szajnar J 2004 J. Mater. Process. Technol. 157 761
[18] Altieri A L, Davis S H 2017 J. Cryst. Growth 467 162
Google Scholar
[19] Colin J 2018 J. Cryst. Growth 493 76
Google Scholar
[20] Vogel A, Cantor B 1977 J. Cryst. Growth 37 309
Google Scholar
[21] Fan H L, Chen M W, Shan Y Y 2019 Surf. Rev. Lett. 11 1950170
下载:
计量
- 文章访问数: 4875
- PDF下载量: 50
- 被引次数: 0
