-
In this paper, we introduce some new excited states of magnetization in ferromagnetic nanowires, including Akhmediev breathers, Kuznetsov-Ma soliton and rogue wave in isotropic ferromagnetic nanowires, and rogue wave in anisotropic ferromagnetic nanowires driven by spin-polarized current. The isotropic case demonstrates a spatial periodic process of a magnetic soliton forming the petal with four pieces and a localized process of the spin-wave background. In a limit case, we get rogue waves and clarify its formation mechanism. In the case of anisotropy, it is found that the generation of rogue waves mainly comes from the accumulation of energy and rapid dispersion in the center. In addition, rogue waves are unstable, the spin-polarized current can control the exchange rate of magnons between the envelope soliton and the background. These results can be useful for the exploration of nonlinear excitation in Bosonic and fermionic ferromagnet.
-
Keywords:
- breathers /
- magnetic rogue wave /
- spin-transfer torque /
- modulation instability
[1] Bu K M, Kwon H Y, Kang S P, Kim H J, Won C 2013 J. Magn. Magn. Mater. 343 32
Google Scholar
[2] Moon J H, Seo S M, Lee K J, Kim K W, Ryu J, Lee H W, McMichael R D, Stiles M D 2013 Phys. Rev. B 88 184404
Google Scholar
[3] Yu X Z, Kanazawa N, Onose Y, Kimoto K, Zhang W Z, Ishiwata S, Matsui Y, Tokura Y 2011 Nat. Mater. 10 106
Google Scholar
[4] Rohart S, Thiaville A 2013 Phys. Rev. B 88 184422
Google Scholar
[5] Robler U K, Bogdanov A N, Pfleiderer C 2006 Nature 442 797
Google Scholar
[6] Allwood D A, Xiong G, Faulkner C C, Atkinson D, Petit D, Cowburn R P 2005 Science 309 1688
Google Scholar
[7] Parkin S S P, Hayashi M, Thomas L 2008 Science 320 190
Google Scholar
[8] Li Z D, Hu Y C, He P B, Sun L L 2018 Chin. Phys. B 27 077505
Google Scholar
[9] He P B, Xie X C, Liu W M 2005 Phys. Rev. B 72 172411
Google Scholar
[10] Tretiakov O A, Liu Y, Abanov Ar 2012 Phys. Rev. Lett. 108 247201
Google Scholar
[11] Li Q Y, Zhao F, He P B, Li Z D 2015 Chin. Phys. B 24 037508
Google Scholar
[12] Kosevich A M, Ivanov B A, Kovalev A S 1990 Phys. Rep. 194 117
Google Scholar
[13] Mikeska H J, Steiner M 1991 Adv. Phys. 40 191
Google Scholar
[14] Haazen P P J, Mure E, Franken J H, Lavrijsen R, H. Swagten J M, Koopmans B 2013 Nat. Mater. 12 299
Google Scholar
[15] Miron I M, Gaudin G, Auffret S, Rodmacq B, Schuhl A, Pizzini S, Vogel J, Gambardella P 2010 Nat. Mater. 9 230
Google Scholar
[16] Di K, Zhang V L, Lim H S, Ng S C, Kuok M H, Yu J, Yoon J, Qiu X, Yang H 2015 Phys. Rev. Lett. 114 047201
Google Scholar
[17] Wang W W, Albert M, Beg M, Bisotti M A, Chernyshenko D 2015 Phys. Rev. Lett. 114 087203
Google Scholar
[18] Li Z D, Cui H, Li Q Y, He P B 2018 Ann. Phys. 388 390
Google Scholar
[19] Chesi S, Coish W A 2015 Phys. Rev. B 91 245306
Google Scholar
[20] Li Z D, Liu F, Li Q Y, He P B 2015 J. Appl. Phys. 117 173906
Google Scholar
[21] Pfleiderer C, Julian S R, Lonzarich G G 2001 Nature 414 427
Google Scholar
[22] Uchida M, Onose Y, Matsui Y, Tokura Y 2006 Science 311 359
Google Scholar
[23] Meckler S, Mikuszeit N, Preßler A, Vedmedenko E Y, Pietzsch O, Wiesendanger R 2009 Phys. Rev. Lett. 103 157201
Google Scholar
[24] Moriya T 1960 Phys.Rev. 120 91
Google Scholar
[25] Zakharov D V, Deisenhofer J 2006 Phys. Rev. B 73 094452
Google Scholar
[26] Gangadharaiah S, Sun J M, Starykh O A 2008 Phys. Rev. B 78 054436
Google Scholar
[27] Albert F J, Emley N C, Myers E B, Ralph D C, Buhrman R A 2002 Phys. Rev. Lett. 89 226802
Google Scholar
[28] Mucciolo E R, Chamon C, Marcus C M 2002 Phys. Rev. Lett. 89 146802
Google Scholar
[29] Beach G S D, Knutson C, Nistor C, Tsoi M, Erskine J L 2006 Phys. Rev. Lett. 97 057203
Google Scholar
[30] Bertotti G, Serpico C, Mayergoyz I D, Magni A, Aquino M, Bonin R 2005 Phys. Rev. Lett. 94 127206
Google Scholar
[31] Garcia-Sanchez F, Borys P, Soucaille R, Adam J P, Stamps R L, Kim J V 2015 Phys. Rev. Lett. 114 247206
Google Scholar
[32] Katine J A, Albert F J, Buhrman R A, Myers E B, Ralph D C 2000 Phys. Rev. Lett. 84 3149
Google Scholar
[33] Tsoi M, Jansen A G M, Bass J, Chiang W C, Seck M, Tsoi V, Wyder P 1998 Phys. Rev. Lett. 80 4281
Google Scholar
[34] He P B, Liu W M 2005 Phys. Rev. B 72 064410
Google Scholar
[35] Li Z D, He P B, Liu W M 2014 Chin. Phys. B 23 117502
Google Scholar
[36] Tsoi M, Tsoi V, Bass J, Jansen A G M, Wyder P 2002 Phys. Rev. Lett. 89 246803
Google Scholar
[37] Tserkovnyak Y, Brataas A, Bauer G E W 2002 Phys. Rev. Lett. 88 117601
Google Scholar
[38] Li Z D, Li Q Y, He P B, Liang J Q, Liu W M, Fu G S 2010 Phys. Rev. A 81 015602
Google Scholar
[39] Freimuth F, Blugel S, Mokrousov Y 2014 Phys. Rev. B 90 174423
Google Scholar
[40] Santos T S, Lee J S, Migdal P, Lekshmi I C, Satpati B, Moodera J S 2007 Phys. Rev. Lett. 98 016601
Google Scholar
[41] Li Z, Zhang S 2004 Phys. Rev. Lett. 92 207203
Google Scholar
[42] Kasai S, Nakatani Y, Kobayashi K, Kohno H, Ono T 2006 Phys. Rev. Lett. 97 107204
Google Scholar
[43] Kasai S, Fischer P, Im M Y, Yamada K, Nakatani Y, Kobayashi K, Kohno H, Ono T 2008 Phys. Rev. Lett. 101 237203; Sugimoto S, Fukuma Y, Otani Y C 2011 IEEI. T. Magn. 47 2951
[44] Yamada K, Kasai S, Nakatani Y, Kobayashi K, Kohno H, Thiaville A, Ono T 2007 Nat. Mater. 6 270; Moriya R, Thomas L, Hayashi M, Bazaliy Y B, Rettner C, Parkin S S P 2008 Nat. Phys. 4 368
[45] Zhao L C, Ling L 2016 J. Opt. Soc. Am. B 33 850
Google Scholar
[46] Liu C, Yang Z Y, Zhao L C, Yang W L 2015 Phys. Rev. E 91 022904
Google Scholar
[47] Duan L, Zhao L C, Xu W H 2017 Phys. Rev. E 95 042212
Google Scholar
[48] Wang L, X Wuan, Zhang H Y 2018 Phys. Lett. A 382 2650
Google Scholar
[49] Wang L, Liu C, Wu X, Wang X, Sun W R 2018 Nonlinear Dyn. 94 977
Google Scholar
[50] Zakharov V E, Gelash A A 2013 Phys. Rev. Lett. 111 054101
Google Scholar
[51] Gelash A A, Zakharov V E 2014 Nonlinearity. 27 R1
Google Scholar
[52] Yan P, Wang X S, Wang X R 2011 Phys. Rev. Lett. 107 177207
Google Scholar
[53] Zhao F, Li Z D, Li Q Y, Wen L, Fu G S, Liu W M 2012 Ann. Phys. 327 2085
Google Scholar
[54] Li Z D, Li Q Y, Xu T F, He P B 2016 Phys. Rev. E 94 042220
Google Scholar
[55] Li Q Y, Li Z D, He P B, Song W W, Fu G S 2010 Can. J. Phys. 88 9
[56] Hasegawa A 1984 Opt. Lett. 9 288; Tai K, Tomita A, Jewell J L, Hasegawa A 1986 Appl. Phys. Lett. 49 236
[57] Akhmediev N N 2001 Nature. 413 267; Van Simaeys G, Emplit P, Haelterman M 2001 Phys. Rev. Lett. 87 033902; Mussot A, Kudlinski A, Droques M, Szriftgiser P, Akhmediev N 2014 Phys. Rev. X 4 011054
[58] Zhang H Q, Tian B, Xing L, Meng X H 2010 Physica A 389 367
Google Scholar
[59] Matveev V B, Salli M A 1991 Darboux Transformations and Solitons, Vol. 5 (Berlin: Springer) pp7−15
[60] 谷超豪, 胡和生, 周子翔 2005 孤立子理论中的达布变换及其几何应用(第二版)(上海: 上海科学技术出版社)第18−24页
Gu C H, Hu H S, Zhou Z X 2005 Darboux Transformation in Soliton Theory and Its Geometric Applications (Shanghai: Scientific and Technical Publishers) pp18−24 (in Chinese).
[61] Li Z D, Wu X, Li Q Y, He P B 2016 Chin. Phys. B 25 010507
Google Scholar
[62] Li Z D, Huo C Z, Li Q Y, He P B, Xu T F 2018 Chin. Phys. B 27 040505
Google Scholar
[63] Ho T L 1998 Phys. Rev. Lett. 81 742; Law C K, Ohmi T, Machida K, 1998 J. Phys. Soc. Jpn. 67 1822; Law C K, Pu H, Bigelow N P 1998 Phys. Rev. Lett. 81 5257
[64] Pu H, Zhang W P, Meystre P 2001 Phys. Rev. Lett. 87 140405
Google Scholar
[65] Li Z D, He P B, Li L, Liang J Q, Liu W M 2005 Phys. Rev. A 71 053611
Google Scholar
-
图 1 方程(3)在
$\mu \to {A_{\rm{s}}}{k_{\rm{s}}}, \nu = {k_{\rm{s}}}\sqrt {1 - A_{\rm{s}}^2} $ 的极限条件下, 磁化分量${m_3}$ 的渐近过程, 参数如下: As = 0.9, ks = 1 (a) μ = 0.82; (b) μ = 0.89; (c) μ = 0.89999; (d) μ = 1.1; (e) μ = 0.96; (f) μ = 0.9001Fig. 1. The asymptotic processes of the magnetic component
${m_3}$ in the limit processes$\mu \to {A_{\rm{s}}}{k_{\rm{s}}}$ and$\nu = {k_{\rm{s}}}\sqrt {1 - A_{\rm{s}}^2} $ in Eq. (3), where the parameters are as follows: As = 0.9, ks = 1: (a) μ = 0.82; (b) μ = 0.89; (c) μ = 0.89999; (d) μ = 1.1; (e) μ = 0.96; (f) μ = 0.9001, respectively.
图 2 方程(3)和(7)中磁化强度
${{m}} = \left( {{m_1}, {m_2}, {m_3}} \right)$ 的怪波演化图, 即(a)−(c)为亮怪波, (d)−(f)为暗怪波. 参数:${A_{\rm{s}}} = \sqrt 3 /2, {k_{\rm{s}}} = 1.5, \nu = {k_{\rm{s}}}\sqrt {1 - A_{\rm{s}}^2}, \mu = \pm 0.75 \sqrt 3 $ , ±分别表示亮怪波和暗怪波[54]Fig. 2. The graphical evolution of rogue waves for the magnetization
${{m}} = \left( {{m_1}, {m_2}, {m_3}} \right)$ in Eq. (3) and (7), i.e., bright rogue waves (a)−(c) and dark rogue waves (d)−(f). The parameters are as follows:${A_{\rm{s}}} = \sqrt 3 /2, {k_{\rm{s}}} = 1.5, \nu = {k_{\rm{s}}}\sqrt {1 - A_{\rm{s}}^2} $ , and$\mu = \pm 0.75 \sqrt 3 $ with the sign$ \pm $ corresponding to the bright and dark rogue waves, respectively[54].
图 3 方程(3)在As = 1,
$\mu \to {A_{\rm{s}}}{k_{\rm{s}}}, \nu = {k_{\rm{s}}}\sqrt {1 - A_{\rm{s}}^2} $ 的条件下, 磁化分量${{m_3}}$ 四片花瓣结构的演化图. 参数如下: As = 1,$ {k_{\rm{s}}} = 0.9, \mu = 0.8999$ Fig. 3. The formation of magnetic petal in the component
${{m_3}}$ of Eq.(3) under the special condition of As = 1,$ \mu \to {A_{\rm{s}}}{k_{\rm{s}}}, \nu = {k_{\rm{s}}}\sqrt {1 - A_{\rm{s}}^2}$ . The parameters are as follows:${A_{\rm{s}}} = 1, {k_{\rm{s}}} = 0.9, \mu = 0.8999$ .
图 4 不同的参数
${\mu _1}$ 下的磁振子密度分布图[53], 范围从0.09到0.29间隔0.05. 插图为怪波形成时的磁振子密度. 其余参数为${A_{\rm{c}}} = 0.2, {A_{\rm{J}}} = {k_c} = 0.1$ Fig. 4. The magnon density distribution against the background for the different parameter
${\mu _1}$ , which ranges from 0.09 to 0.29 in 0.05 steps[53]. The inset figure is the magnon density distribution against the background for the excited formation of magnetic rogue wave. Other parameters are${A_{\rm{c}}} = 0.2, {A_{\rm{J}}} = {k_{\rm{c}}} = 0.1$ .
图 5 (a)−(e)不同电流值激发怪波在区域
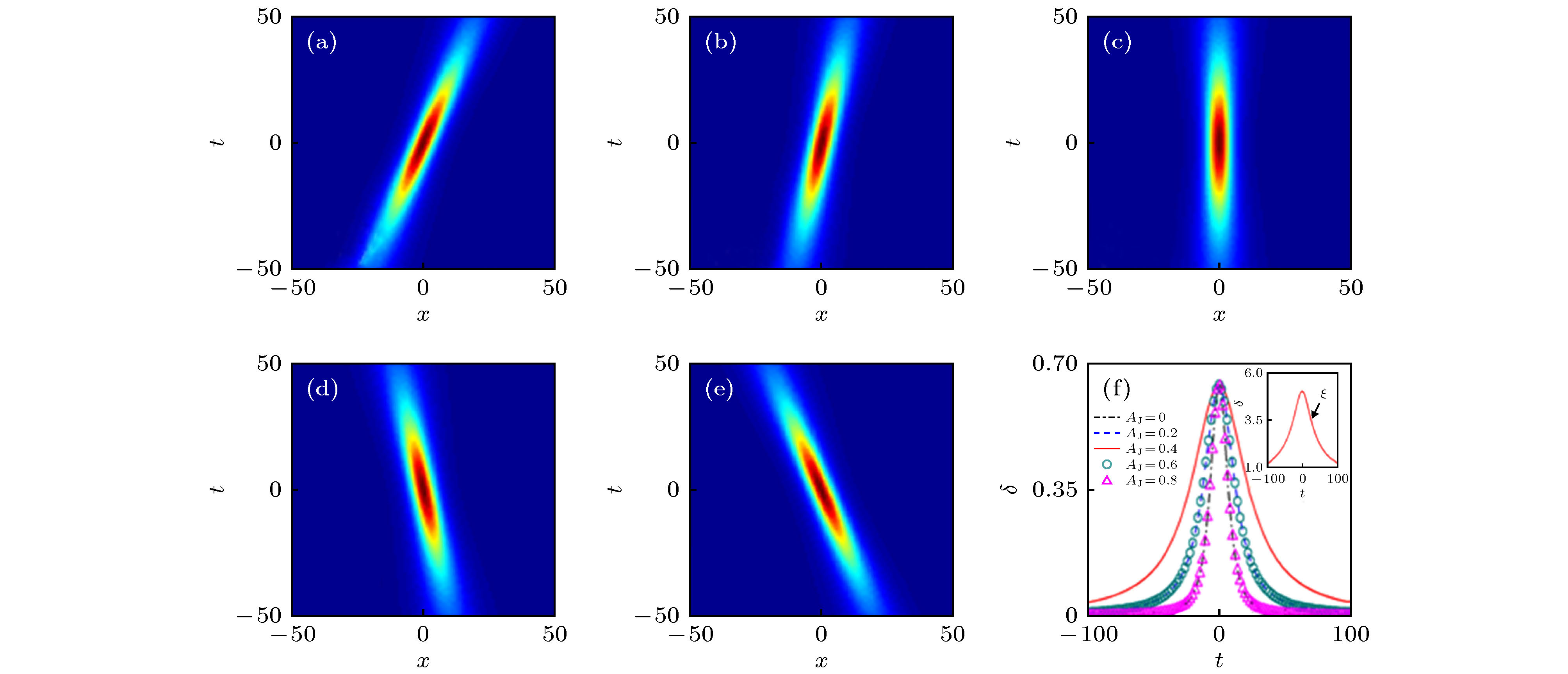
$\left( {x, t} \right)$ 的分布图[53], 电流从0到0.8, 间隔为0.2; (f)不同电流怪波形成时的图形, 插图为磁振子积聚的最大时情况. 临界电流${A_{\rm{J}}} = 2{k_{\rm{c}}}$ , 其它参数${A_{\rm{c}}} = {k_{\rm{c}}} = 0.2$ Fig. 5. (a)−(e) The formation region in space
$\left( {x, t} \right)$ for magnetic rogue wave with different current[53]. The parameter${A_J}$ ranges form 0 to 0.8 in 0.2 steps; (f) The nonuniform exchange of magnons between rogue wave and background for the different spin current. The inset figure in (f) denotes the maximal accumulation (or dissipation) process for the critical current value${A_{\rm{J}}} = 2{k_{\rm{c}}}$ . Other parameters are${A_{\rm{c}}} = {k_{\rm{c}}} = 0.2$ . -
[1] Bu K M, Kwon H Y, Kang S P, Kim H J, Won C 2013 J. Magn. Magn. Mater. 343 32
Google Scholar
[2] Moon J H, Seo S M, Lee K J, Kim K W, Ryu J, Lee H W, McMichael R D, Stiles M D 2013 Phys. Rev. B 88 184404
Google Scholar
[3] Yu X Z, Kanazawa N, Onose Y, Kimoto K, Zhang W Z, Ishiwata S, Matsui Y, Tokura Y 2011 Nat. Mater. 10 106
Google Scholar
[4] Rohart S, Thiaville A 2013 Phys. Rev. B 88 184422
Google Scholar
[5] Robler U K, Bogdanov A N, Pfleiderer C 2006 Nature 442 797
Google Scholar
[6] Allwood D A, Xiong G, Faulkner C C, Atkinson D, Petit D, Cowburn R P 2005 Science 309 1688
Google Scholar
[7] Parkin S S P, Hayashi M, Thomas L 2008 Science 320 190
Google Scholar
[8] Li Z D, Hu Y C, He P B, Sun L L 2018 Chin. Phys. B 27 077505
Google Scholar
[9] He P B, Xie X C, Liu W M 2005 Phys. Rev. B 72 172411
Google Scholar
[10] Tretiakov O A, Liu Y, Abanov Ar 2012 Phys. Rev. Lett. 108 247201
Google Scholar
[11] Li Q Y, Zhao F, He P B, Li Z D 2015 Chin. Phys. B 24 037508
Google Scholar
[12] Kosevich A M, Ivanov B A, Kovalev A S 1990 Phys. Rep. 194 117
Google Scholar
[13] Mikeska H J, Steiner M 1991 Adv. Phys. 40 191
Google Scholar
[14] Haazen P P J, Mure E, Franken J H, Lavrijsen R, H. Swagten J M, Koopmans B 2013 Nat. Mater. 12 299
Google Scholar
[15] Miron I M, Gaudin G, Auffret S, Rodmacq B, Schuhl A, Pizzini S, Vogel J, Gambardella P 2010 Nat. Mater. 9 230
Google Scholar
[16] Di K, Zhang V L, Lim H S, Ng S C, Kuok M H, Yu J, Yoon J, Qiu X, Yang H 2015 Phys. Rev. Lett. 114 047201
Google Scholar
[17] Wang W W, Albert M, Beg M, Bisotti M A, Chernyshenko D 2015 Phys. Rev. Lett. 114 087203
Google Scholar
[18] Li Z D, Cui H, Li Q Y, He P B 2018 Ann. Phys. 388 390
Google Scholar
[19] Chesi S, Coish W A 2015 Phys. Rev. B 91 245306
Google Scholar
[20] Li Z D, Liu F, Li Q Y, He P B 2015 J. Appl. Phys. 117 173906
Google Scholar
[21] Pfleiderer C, Julian S R, Lonzarich G G 2001 Nature 414 427
Google Scholar
[22] Uchida M, Onose Y, Matsui Y, Tokura Y 2006 Science 311 359
Google Scholar
[23] Meckler S, Mikuszeit N, Preßler A, Vedmedenko E Y, Pietzsch O, Wiesendanger R 2009 Phys. Rev. Lett. 103 157201
Google Scholar
[24] Moriya T 1960 Phys.Rev. 120 91
Google Scholar
[25] Zakharov D V, Deisenhofer J 2006 Phys. Rev. B 73 094452
Google Scholar
[26] Gangadharaiah S, Sun J M, Starykh O A 2008 Phys. Rev. B 78 054436
Google Scholar
[27] Albert F J, Emley N C, Myers E B, Ralph D C, Buhrman R A 2002 Phys. Rev. Lett. 89 226802
Google Scholar
[28] Mucciolo E R, Chamon C, Marcus C M 2002 Phys. Rev. Lett. 89 146802
Google Scholar
[29] Beach G S D, Knutson C, Nistor C, Tsoi M, Erskine J L 2006 Phys. Rev. Lett. 97 057203
Google Scholar
[30] Bertotti G, Serpico C, Mayergoyz I D, Magni A, Aquino M, Bonin R 2005 Phys. Rev. Lett. 94 127206
Google Scholar
[31] Garcia-Sanchez F, Borys P, Soucaille R, Adam J P, Stamps R L, Kim J V 2015 Phys. Rev. Lett. 114 247206
Google Scholar
[32] Katine J A, Albert F J, Buhrman R A, Myers E B, Ralph D C 2000 Phys. Rev. Lett. 84 3149
Google Scholar
[33] Tsoi M, Jansen A G M, Bass J, Chiang W C, Seck M, Tsoi V, Wyder P 1998 Phys. Rev. Lett. 80 4281
Google Scholar
[34] He P B, Liu W M 2005 Phys. Rev. B 72 064410
Google Scholar
[35] Li Z D, He P B, Liu W M 2014 Chin. Phys. B 23 117502
Google Scholar
[36] Tsoi M, Tsoi V, Bass J, Jansen A G M, Wyder P 2002 Phys. Rev. Lett. 89 246803
Google Scholar
[37] Tserkovnyak Y, Brataas A, Bauer G E W 2002 Phys. Rev. Lett. 88 117601
Google Scholar
[38] Li Z D, Li Q Y, He P B, Liang J Q, Liu W M, Fu G S 2010 Phys. Rev. A 81 015602
Google Scholar
[39] Freimuth F, Blugel S, Mokrousov Y 2014 Phys. Rev. B 90 174423
Google Scholar
[40] Santos T S, Lee J S, Migdal P, Lekshmi I C, Satpati B, Moodera J S 2007 Phys. Rev. Lett. 98 016601
Google Scholar
[41] Li Z, Zhang S 2004 Phys. Rev. Lett. 92 207203
Google Scholar
[42] Kasai S, Nakatani Y, Kobayashi K, Kohno H, Ono T 2006 Phys. Rev. Lett. 97 107204
Google Scholar
[43] Kasai S, Fischer P, Im M Y, Yamada K, Nakatani Y, Kobayashi K, Kohno H, Ono T 2008 Phys. Rev. Lett. 101 237203; Sugimoto S, Fukuma Y, Otani Y C 2011 IEEI. T. Magn. 47 2951
[44] Yamada K, Kasai S, Nakatani Y, Kobayashi K, Kohno H, Thiaville A, Ono T 2007 Nat. Mater. 6 270; Moriya R, Thomas L, Hayashi M, Bazaliy Y B, Rettner C, Parkin S S P 2008 Nat. Phys. 4 368
[45] Zhao L C, Ling L 2016 J. Opt. Soc. Am. B 33 850
Google Scholar
[46] Liu C, Yang Z Y, Zhao L C, Yang W L 2015 Phys. Rev. E 91 022904
Google Scholar
[47] Duan L, Zhao L C, Xu W H 2017 Phys. Rev. E 95 042212
Google Scholar
[48] Wang L, X Wuan, Zhang H Y 2018 Phys. Lett. A 382 2650
Google Scholar
[49] Wang L, Liu C, Wu X, Wang X, Sun W R 2018 Nonlinear Dyn. 94 977
Google Scholar
[50] Zakharov V E, Gelash A A 2013 Phys. Rev. Lett. 111 054101
Google Scholar
[51] Gelash A A, Zakharov V E 2014 Nonlinearity. 27 R1
Google Scholar
[52] Yan P, Wang X S, Wang X R 2011 Phys. Rev. Lett. 107 177207
Google Scholar
[53] Zhao F, Li Z D, Li Q Y, Wen L, Fu G S, Liu W M 2012 Ann. Phys. 327 2085
Google Scholar
[54] Li Z D, Li Q Y, Xu T F, He P B 2016 Phys. Rev. E 94 042220
Google Scholar
[55] Li Q Y, Li Z D, He P B, Song W W, Fu G S 2010 Can. J. Phys. 88 9
[56] Hasegawa A 1984 Opt. Lett. 9 288; Tai K, Tomita A, Jewell J L, Hasegawa A 1986 Appl. Phys. Lett. 49 236
[57] Akhmediev N N 2001 Nature. 413 267; Van Simaeys G, Emplit P, Haelterman M 2001 Phys. Rev. Lett. 87 033902; Mussot A, Kudlinski A, Droques M, Szriftgiser P, Akhmediev N 2014 Phys. Rev. X 4 011054
[58] Zhang H Q, Tian B, Xing L, Meng X H 2010 Physica A 389 367
Google Scholar
[59] Matveev V B, Salli M A 1991 Darboux Transformations and Solitons, Vol. 5 (Berlin: Springer) pp7−15
[60] 谷超豪, 胡和生, 周子翔 2005 孤立子理论中的达布变换及其几何应用(第二版)(上海: 上海科学技术出版社)第18−24页
Gu C H, Hu H S, Zhou Z X 2005 Darboux Transformation in Soliton Theory and Its Geometric Applications (Shanghai: Scientific and Technical Publishers) pp18−24 (in Chinese).
[61] Li Z D, Wu X, Li Q Y, He P B 2016 Chin. Phys. B 25 010507
Google Scholar
[62] Li Z D, Huo C Z, Li Q Y, He P B, Xu T F 2018 Chin. Phys. B 27 040505
Google Scholar
[63] Ho T L 1998 Phys. Rev. Lett. 81 742; Law C K, Ohmi T, Machida K, 1998 J. Phys. Soc. Jpn. 67 1822; Law C K, Pu H, Bigelow N P 1998 Phys. Rev. Lett. 81 5257
[64] Pu H, Zhang W P, Meystre P 2001 Phys. Rev. Lett. 87 140405
Google Scholar
[65] Li Z D, He P B, Li L, Liang J Q, Liu W M 2005 Phys. Rev. A 71 053611
Google Scholar
下载:
计量
- 文章访问数: 8709
- PDF下载量: 136
- 被引次数: 0
