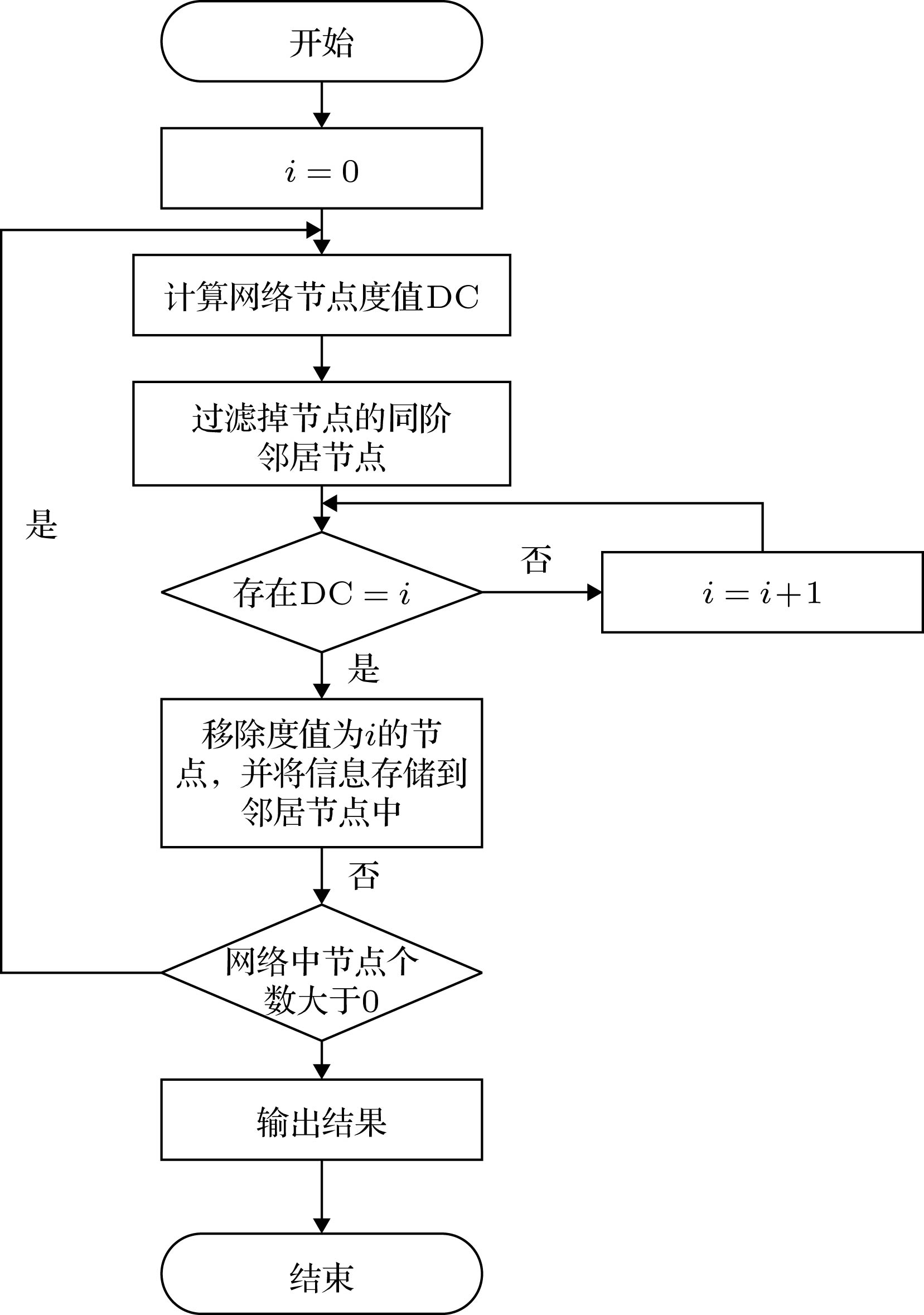
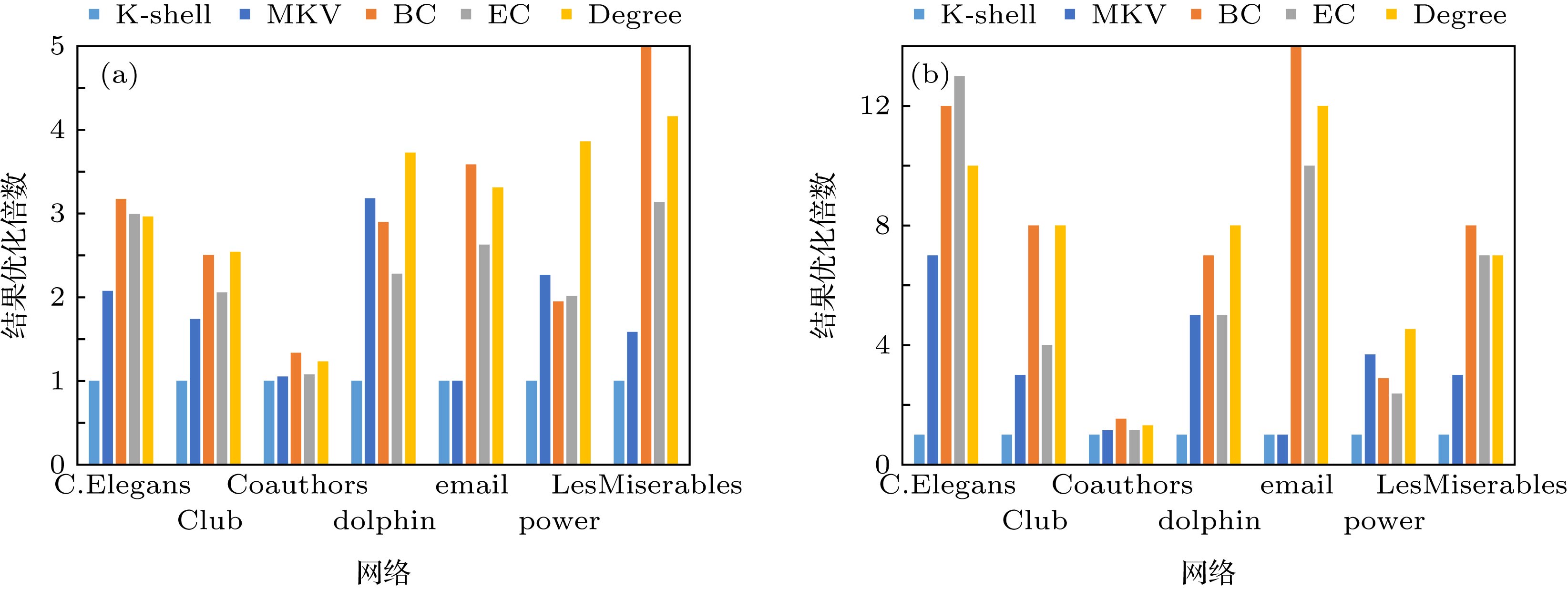
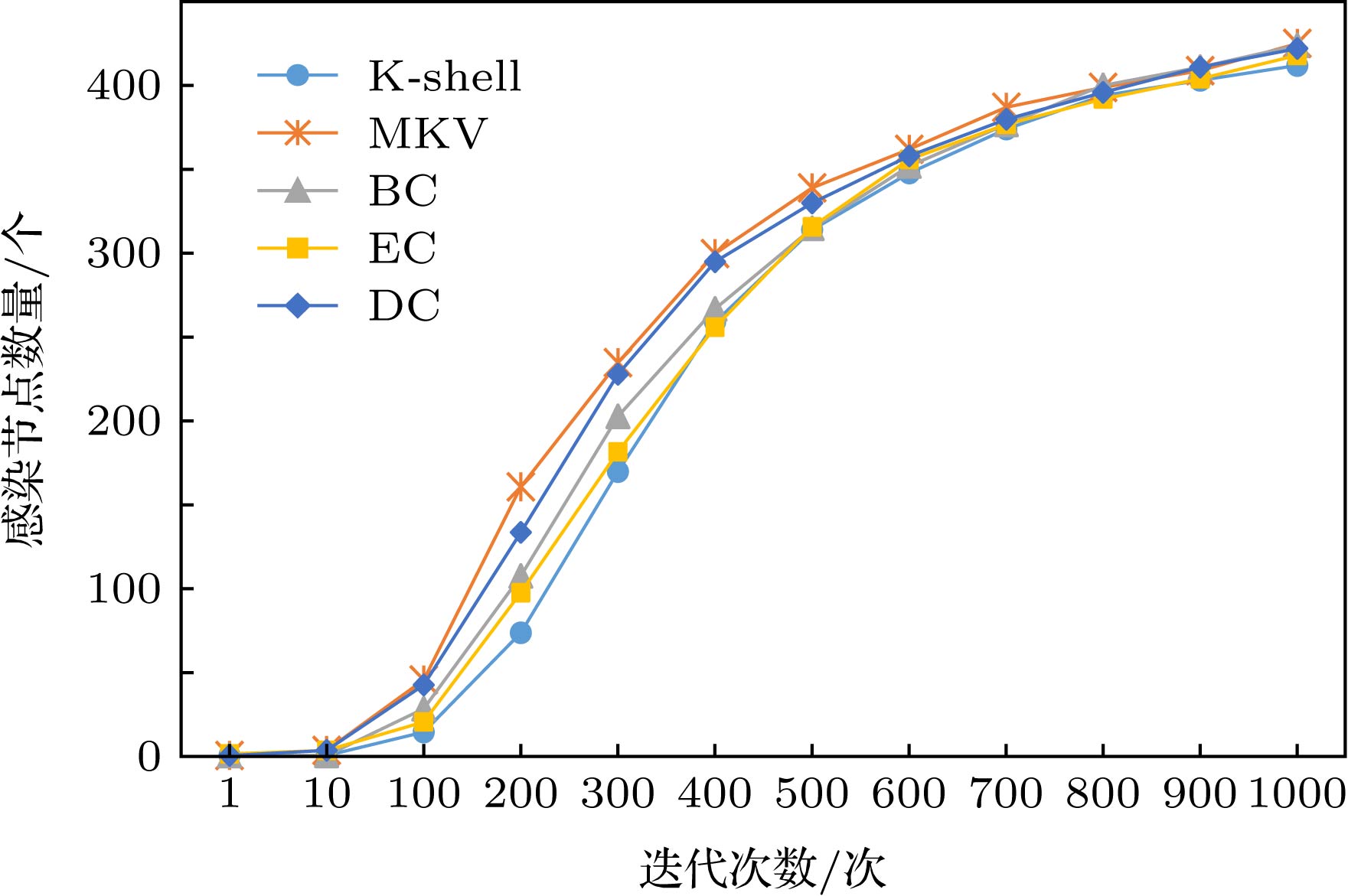
-
K-壳分解法在度量复杂网络中节点的重要性方面具有重要的理论意义和应用价值. 但K-壳方法中, 存在大量壳值相等的节点, 从而无法精确地比较这些具有相同壳值节点的相对重要性. 因此, 本文基于网络中节点自身壳值与其多阶邻居的壳值, 设计利用向量的形式来表示节点在复杂网络中的相对重要性程度, 提出了多阶邻居壳数向量中心性方法, 并设计了该中心性向量比较方法. 通过在七个真实网络中进行消息传播与静态攻击实验, 发现基于多阶邻居壳数向量的中心性方法具有计算复杂度低, 能够有效发现具有高传播能力的节点, 在传播实验中具有优越的性能. 并在静态攻击实验过程中倾向于优先破坏网络中的传播核心结构. 多阶邻居壳数向量中心性方法在保留K-壳中心性信息的前提下, 极大提高了节点重要性的区别程度, 平衡了对节点在复杂网络中联通结构的重要性的度量和对传播结构重要性的度量, 因此具有重要理论意义与应用价值.
-
关键词:
- 中心性 /
- 多阶邻居壳数向量中心性 /
- 易感-感染传播模型 /
- 静态攻击
The K-shell has important theoretical significance and application value in measuring the importance of nodes in complex networks. However, in the K-shell method, most of nodes possess an identical K-shell value so that the relative importance of the identical K-shell nodes cannot be further compared with each other. Therefore, based on the K-shell value of nodes in the complex network and the K-shell values of multi-order neighbors in complex networks, in this paper we use the vectors to represent the relative importance of node in each of complex networks, which is named multi-order K-shell vector. Multi-order K-shell vector centrality defines a vector indicating the number of multi-order neighbors with different K-shells and groups them into elements of the vector. Each vector infers to not only the original K-shell of the given node but also the number of its multi-order neighbors and their K-shell values, which indicates the propagation capability of the given node. An approach to comparing two multi-order K-shell vectors is also presented, which is used to sort the vectors to evaluate the node importance. The method is explored by comparing several existing centrality methods. Through the experiments of SI propagation and static attack experiments in seven real-world networks, it is found that multi-order K-shell vector centrality provides low computational complexity, effectively evaluates nodes with high propagation capability, which confirms the improved performance in susceptible infected model propagation experiments. On the other hand, the static attack experiments show that the multi-order K-shell vector tends to preferentially select the core structure with powerful propagation capability in the network. The multi-order K-shell vector greatly improves the difference rate of node centrality under the premise of preserving the K-shell structure information, as well as balancing the importance measure of nodes in the complex network and the structure evaluation of propagation capability. The multi-order K-shell vector is not appropriate for all types of networks when considering the result of network attacking. For the networks with low clustering coefficients and high average path lengths, multi-order K-shell vector method is dominant and the effect is relatively obvious. By contrast, multi-order K-shell vector surpasses most of centrality approaches when spreading information is our priority. In a few networks, eigenvector centrality presents a slightly better performance with a larger computational complexity. The proposed centrality measure is therefore of great theoretical and practical importance.-
Keywords:
- centrality /
- multi-order K-shell vector /
- susceptible infected model /
- static attack
[1] Barabasi A L, Albert R 1999 Science 286 509
Google Scholar
[2] Newman M, Girvan M 2004 Phys. Rev. E 69 423
[3] Ai J, Su Z, Li Y, Wu C X 2019 Physica A 527 121155
Google Scholar
[4] Ai J, Liu Y Y, Su Z, Zhang H , Zhao F Y 2019 Europhys. Lett. 126 38003
Google Scholar
[5] Brin S, Page L 2012 Computer Networks 56 3825
Google Scholar
[6] Newman M 2010 Networks: An introduction (Oxford: Oxford University Press) p327
[7] Cohen R, Erez K, Ben-Avraham D, Havlin S 2001 Phys. Rev. Lett. 86 3682
Google Scholar
[8] Keeling M J, Rohani P, Pourbohloul B 2008 Clinical Infectious Diseases 47 864
Google Scholar
[9] Moreno Y, Nekovee M, Pacheco A F 2004 Phys. Rev. E 69 066130
Google Scholar
[10] 张彦超, 刘云, 张海峰, 程辉, 熊菲 2011 60 050501
Google Scholar
Zhang Y C, Liu Y, Zhang H F, Cheng H, Xiong F 2011 Acta Phys. Sin. 60 050501
Google Scholar
[11] Li H, Gao G, Chen R 2019 Int. J. Software Engineer. Knowledge Engineer. 29 93
Google Scholar
[12] 刘建国, 任卓明, 郭强, 汪秉宏 2013 62 178901
Google Scholar
Liu J G, Ren Z M, Guo Q, Wang B H 2013 Acta Phys. Sin. 62 178901
Google Scholar
[13] 任晓龙, 吕琳媛 2014 科学通报 59 1175
Ren X L, Lü L Y 2014 Chin. Sci. Bull. 59 1175
[14] Chen D, Lin Y L, Shang M S 2012 Fuel and Energy Abstracts 391 1777
[15] Freeman L C 1977 Sociometry 40 35
Google Scholar
[16] Opsahl T, Agneessens F, Skvoretz J 2010 Social Networks 32 245
Google Scholar
[17] Kitsak M, Gallos L K, Havlin S, Liljeros F, Muchnik L, Stanley H E, Makse H A 2010 Nat. Phys. 6 888
Google Scholar
[18] 罗仕龙, 龚凯, 唐朝生, 周靖 2017 66 188902
Google Scholar
Luo S L, Gong K, Tang C S, Zhou J 2017 Acta Phys. Sin. 66 188902
Google Scholar
[19] Jiang L C, Zhao X, Ge B, Xiao W, Ruan Y 2019 Physica A 516 58
[20] Gong K, Kang L 2018 J. Syst. Sci. Inf. 6 366
[21] 朱晓霞, 赵雪, 刘萌萌 2017 计算机应用研究 34 2582
Google Scholar
Zhu X X, Zhao X, Liu M M 2017 Application Research of Computers 34 2582
Google Scholar
[22] 李慧嘉, 严冠, 刘志东, 李桂君, 章祥荪 2017 中国科学: 数学 47 16
Li H J, Yan G, Liu Z D, Li G J, Zhang X S 2017 Scientia Sinica Mathematica 47 16
[23] 李慧嘉, 李爱华, 李慧颖 2017 计算机学报 40 15
Li H J, Li A H, Li H Y 2017 Chinese Journal of Computers 40 15
[24] Zhang J P, Xu H, Yang J, Lun L J 2018 ICPCSEE Zhengzhou, China, September 21−24, 2018 p28
[25] 王浩, 张赞, 李磊, 汪萌 2016 电子学报 44 2330
Google Scholar
Wang H, Zhang Z, Li L, Wang M 2016 Acta Electronica Sinica 44 2330
Google Scholar
[26] Ai J, Zhao H, Carley K M, Su Z, Li H 2013 Eur. Phys. J. B 86 163
Google Scholar
[27] Freeman L C 1979 Social Networks 1 215
[28] Brandes U 2001 Math. Sociology 25
[29] E.Knuth D 1993 The Stanford GraphBase: A Platform for Combinatorial Computing (Vol.1)
[30] Watts D J, Strogatz S H 1998 Nature 393 440
Google Scholar
[31] Guimera R, Danon L, Diaz-Guilera A, Giralt F, Arenas A 2003 Phys. Rev. E 68 065103
Google Scholar
[32] Newman M 2006 Phys. Rev. E 74 036104
Google Scholar
[33] Lusseau D, Schneider K, Boisseau O, Haase P, Slooten E, Dawson S 2003 Behav. Ecol. Sociobiol. 54 396
Google Scholar
[34] Duch J, Arenas A 2005 Phys. Rev. E 72 027104
Google Scholar
[35] Zachary W W 1977 J. Anthropol. Res. 33 452
Google Scholar
[36] 汪小帆, 李翔, 陈关荣 2012 网络科学导论 (北京: 高等教育出版社) 第306−307页
Wang X F, Li X, Chen G R 2012 Network Science: an Introduction (Beijing: Higher Education Press) pp306−307 (in Chinese)
-
图 4 美国电力网power在静态攻击实验中最大连通子团变化情况
Fig. 4. Largest connected component during static attack experiment on power.
图 5 美国电力网power在蓄意攻击实验中子团数量变化情况
Fig. 5. Total component number during static attack experiment on power.
图 6 七个网络受到蓄意攻击中最大连通子团的统计情况(越小越好) (a) 最大连通子团平均值情况; (b) 最大连通子团最值情况
Fig. 6. Largest connected component during static attack experiments on the seven networks(the smaller, the better)
图 7 七个网络静态攻击重要节点的子团数量的统计情况(越大越好) (a) 子团数量平均值情况; (b) 子团数量最值情况
Fig. 7. The number of components during static attack experiments on the seven networks (the larger, the better)
图 9 美国电力网power在SI传播模型下感染节点的变化情况
Fig. 9. Change of infected nodes in Power of American Electric Power network under SI propagation model.
图 10 大肠杆菌代谢网络C. Elegans在SI传播模型下感染节点的变化情况
Fig. 10. Changes of infection nodes in C. Elegans network under SI propagation model.
图 11 七个网络传播感染节点的统计情况(越大越好) (a) SI传播节点数量比较平均值; (b) SI传播节点数量比较最值
Fig. 11. The statistical result of infected nodes of seven network (the larger, the better)
表 1 本文中用到的几个网络基本信息
Table 1. Several basic network information used in this paper.
网络 节点数量 边数量 平均度值 聚集系数 平均路径长度 同配系数 LesMiserables 77 254 6.597 0.736 2.641 –0.4756 PowerGrid 4941 6594 2.669 0.107 18.989 0.4616 Email 1134 6556 11.563 0.526 1.992 –0.0436 Coauthor 1589 2742 3.451 0.878 5.823 0.0035 Dolphin 62 159 5.129 0.303 3.357 –0.1652 C.Elegans 453 4596 9.007 0.657 2.664 –0.1085 Club 34 78 2.29 0.588 2.408 –0.2145 
下载: 导出CSV
表 2 中心性的区分度在不同网络中的取值情况(越大越好)
Table 2. Value of Centrality Distinction in Different Networks(the larger, the better).
DR BC EC Degree K-shell MKV C.Elegans 66.225% 88.962% 8.830% 2.208% 40.839% Club 61.765% 79.412% 32.353% 11.765% 47.059% Coauthors 9.880% 29.264% 1.447% 0.692% 9.880% Dolphin 87.097% 96.774% 19.355% 6.452% 70.968% Email 62.346% 94.533% 4.586% 0.970% 53.263% Power 59.279% 86.217% 0.324% 0.101% 8.561% LesMiserables 41.558% 67.532% 23.377% 10.390% 37.662%
下载: 导出CSV
-
[1] Barabasi A L, Albert R 1999 Science 286 509
Google Scholar
[2] Newman M, Girvan M 2004 Phys. Rev. E 69 423
[3] Ai J, Su Z, Li Y, Wu C X 2019 Physica A 527 121155
Google Scholar
[4] Ai J, Liu Y Y, Su Z, Zhang H , Zhao F Y 2019 Europhys. Lett. 126 38003
Google Scholar
[5] Brin S, Page L 2012 Computer Networks 56 3825
Google Scholar
[6] Newman M 2010 Networks: An introduction (Oxford: Oxford University Press) p327
[7] Cohen R, Erez K, Ben-Avraham D, Havlin S 2001 Phys. Rev. Lett. 86 3682
Google Scholar
[8] Keeling M J, Rohani P, Pourbohloul B 2008 Clinical Infectious Diseases 47 864
Google Scholar
[9] Moreno Y, Nekovee M, Pacheco A F 2004 Phys. Rev. E 69 066130
Google Scholar
[10] 张彦超, 刘云, 张海峰, 程辉, 熊菲 2011 60 050501
Google Scholar
Zhang Y C, Liu Y, Zhang H F, Cheng H, Xiong F 2011 Acta Phys. Sin. 60 050501
Google Scholar
[11] Li H, Gao G, Chen R 2019 Int. J. Software Engineer. Knowledge Engineer. 29 93
Google Scholar
[12] 刘建国, 任卓明, 郭强, 汪秉宏 2013 62 178901
Google Scholar
Liu J G, Ren Z M, Guo Q, Wang B H 2013 Acta Phys. Sin. 62 178901
Google Scholar
[13] 任晓龙, 吕琳媛 2014 科学通报 59 1175
Ren X L, Lü L Y 2014 Chin. Sci. Bull. 59 1175
[14] Chen D, Lin Y L, Shang M S 2012 Fuel and Energy Abstracts 391 1777
[15] Freeman L C 1977 Sociometry 40 35
Google Scholar
[16] Opsahl T, Agneessens F, Skvoretz J 2010 Social Networks 32 245
Google Scholar
[17] Kitsak M, Gallos L K, Havlin S, Liljeros F, Muchnik L, Stanley H E, Makse H A 2010 Nat. Phys. 6 888
Google Scholar
[18] 罗仕龙, 龚凯, 唐朝生, 周靖 2017 66 188902
Google Scholar
Luo S L, Gong K, Tang C S, Zhou J 2017 Acta Phys. Sin. 66 188902
Google Scholar
[19] Jiang L C, Zhao X, Ge B, Xiao W, Ruan Y 2019 Physica A 516 58
[20] Gong K, Kang L 2018 J. Syst. Sci. Inf. 6 366
[21] 朱晓霞, 赵雪, 刘萌萌 2017 计算机应用研究 34 2582
Google Scholar
Zhu X X, Zhao X, Liu M M 2017 Application Research of Computers 34 2582
Google Scholar
[22] 李慧嘉, 严冠, 刘志东, 李桂君, 章祥荪 2017 中国科学: 数学 47 16
Li H J, Yan G, Liu Z D, Li G J, Zhang X S 2017 Scientia Sinica Mathematica 47 16
[23] 李慧嘉, 李爱华, 李慧颖 2017 计算机学报 40 15
Li H J, Li A H, Li H Y 2017 Chinese Journal of Computers 40 15
[24] Zhang J P, Xu H, Yang J, Lun L J 2018 ICPCSEE Zhengzhou, China, September 21−24, 2018 p28
[25] 王浩, 张赞, 李磊, 汪萌 2016 电子学报 44 2330
Google Scholar
Wang H, Zhang Z, Li L, Wang M 2016 Acta Electronica Sinica 44 2330
Google Scholar
[26] Ai J, Zhao H, Carley K M, Su Z, Li H 2013 Eur. Phys. J. B 86 163
Google Scholar
[27] Freeman L C 1979 Social Networks 1 215
[28] Brandes U 2001 Math. Sociology 25
[29] E.Knuth D 1993 The Stanford GraphBase: A Platform for Combinatorial Computing (Vol.1)
[30] Watts D J, Strogatz S H 1998 Nature 393 440
Google Scholar
[31] Guimera R, Danon L, Diaz-Guilera A, Giralt F, Arenas A 2003 Phys. Rev. E 68 065103
Google Scholar
[32] Newman M 2006 Phys. Rev. E 74 036104
Google Scholar
[33] Lusseau D, Schneider K, Boisseau O, Haase P, Slooten E, Dawson S 2003 Behav. Ecol. Sociobiol. 54 396
Google Scholar
[34] Duch J, Arenas A 2005 Phys. Rev. E 72 027104
Google Scholar
[35] Zachary W W 1977 J. Anthropol. Res. 33 452
Google Scholar
[36] 汪小帆, 李翔, 陈关荣 2012 网络科学导论 (北京: 高等教育出版社) 第306−307页
Wang X F, Li X, Chen G R 2012 Network Science: an Introduction (Beijing: Higher Education Press) pp306−307 (in Chinese)
下载:
计量
- 文章访问数: 9646
- PDF下载量: 83
- 被引次数: 0
