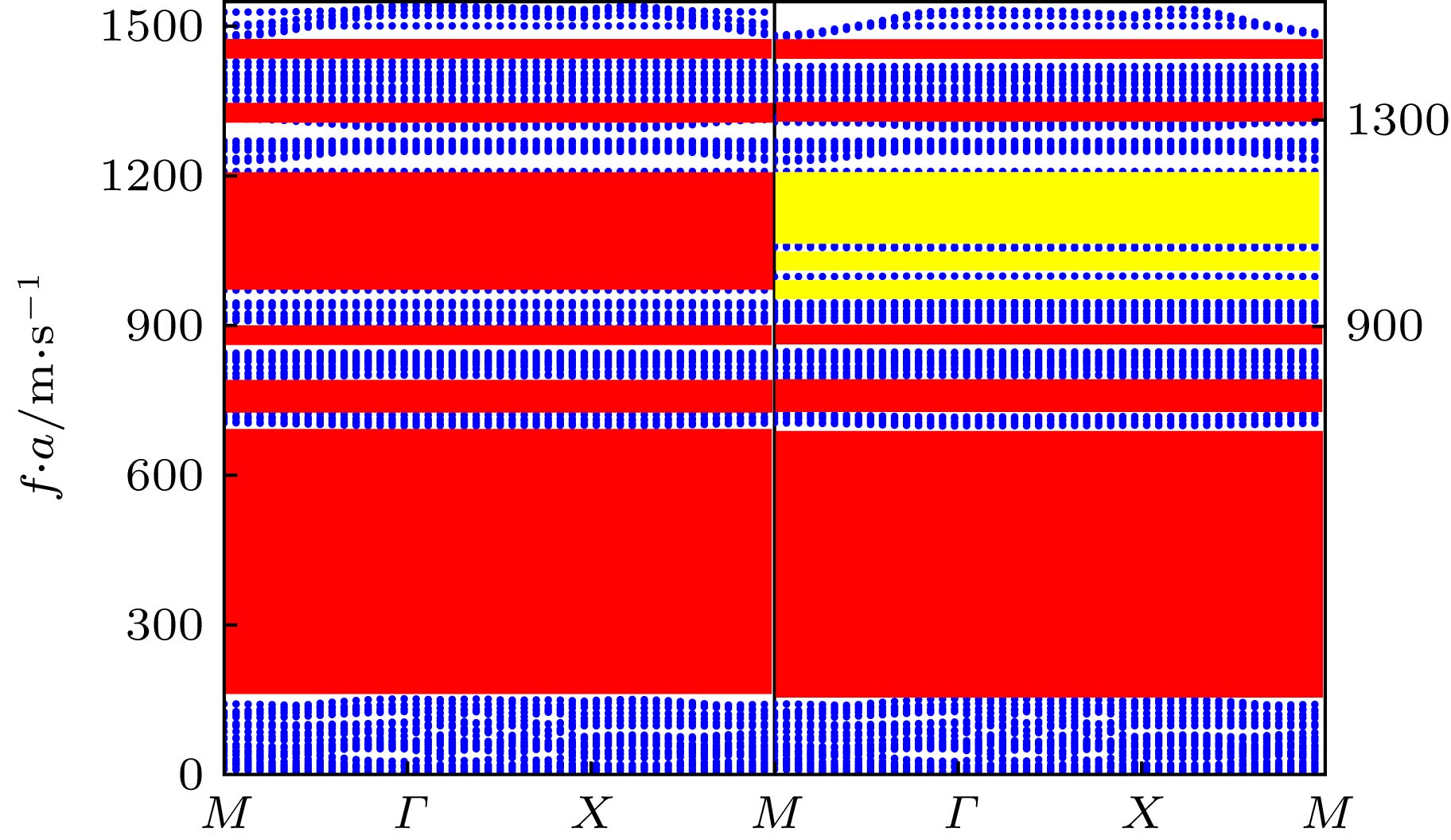
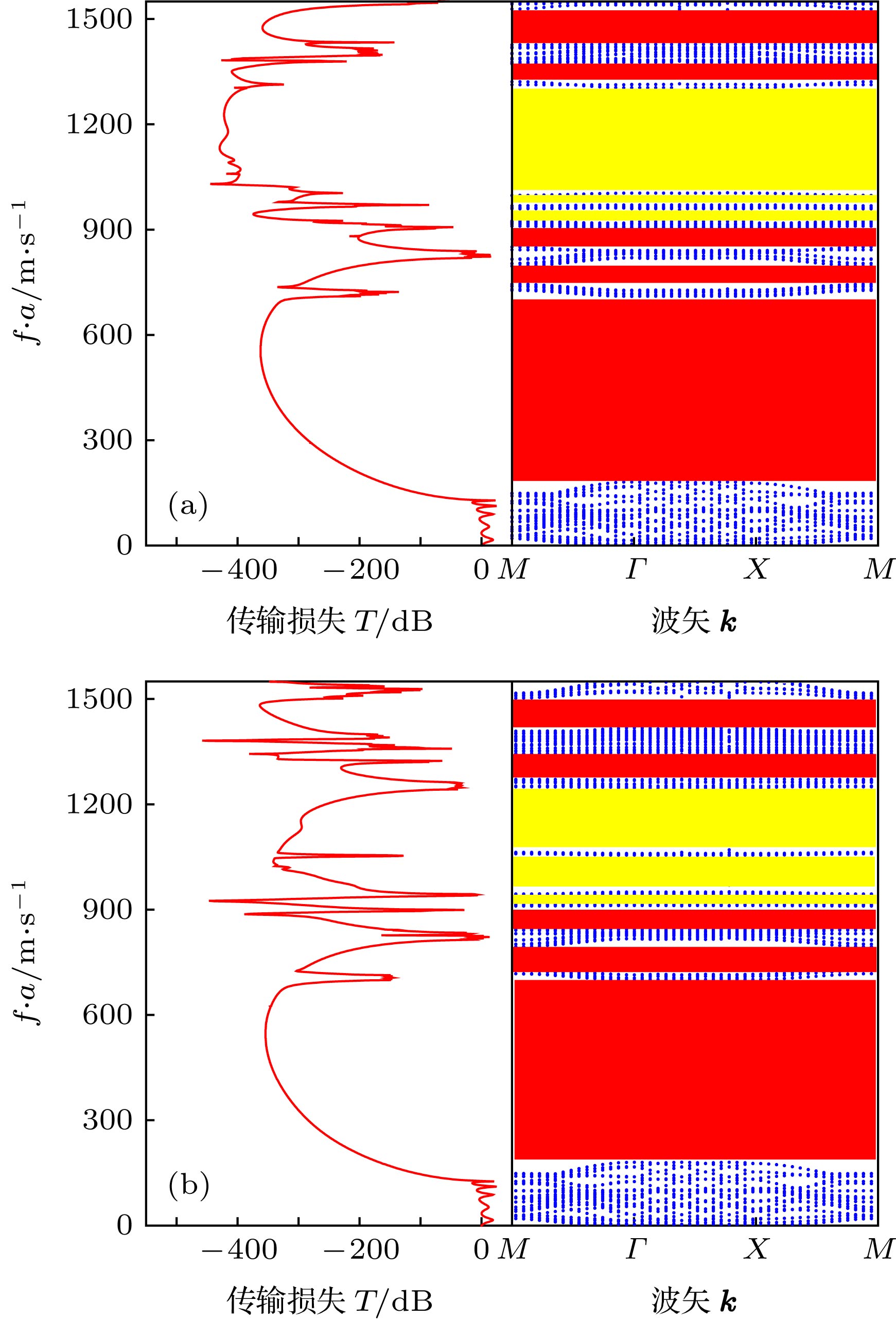
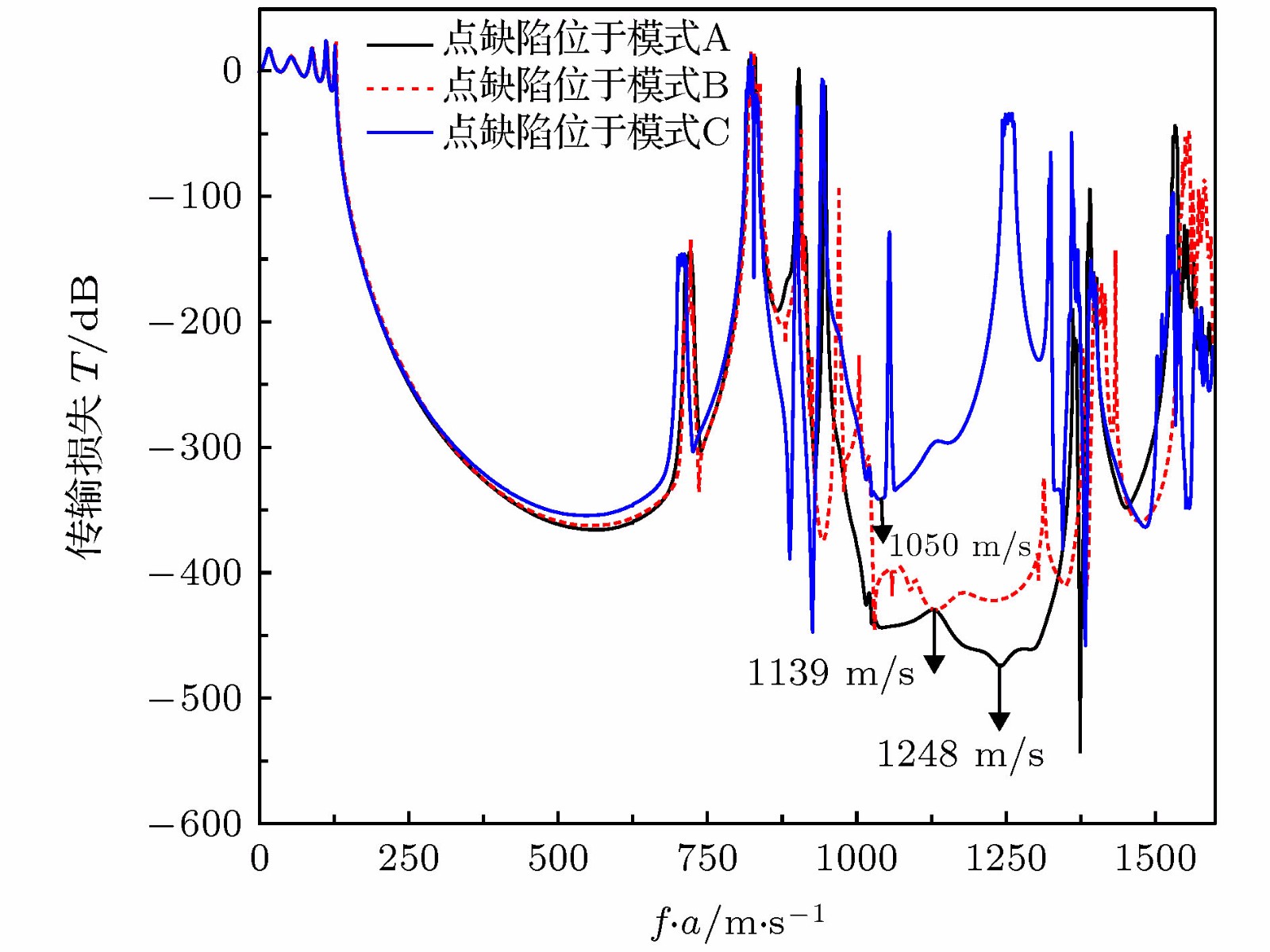
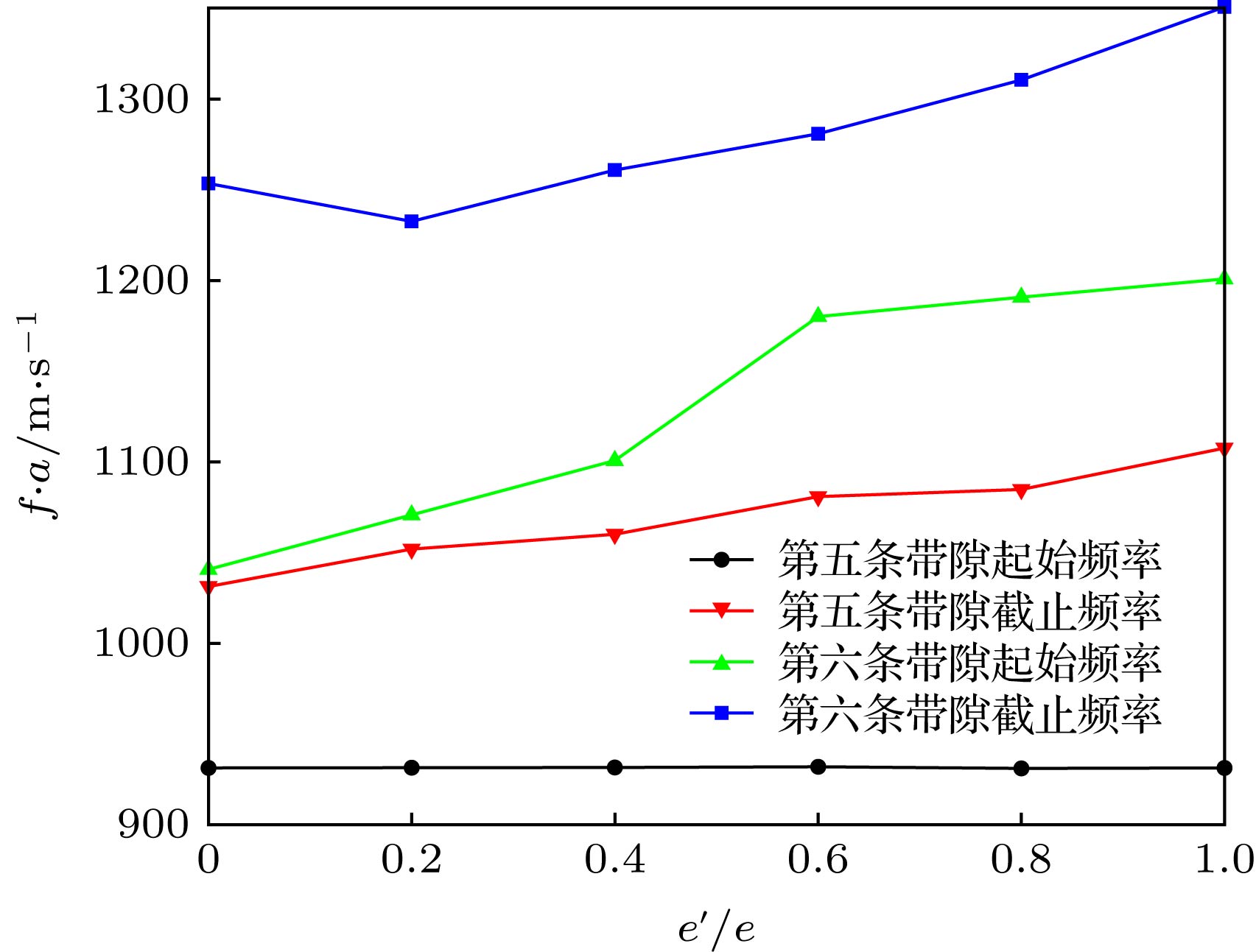
-
设计了一种由包裹有机玻璃涂层的四棱柱形压电材料按正方形晶格周期性连接在四个环氧树脂短板上构成的1×5新型二维压电声子晶体板, 并利用超元胞法结合有限元方法分别计算了完美声子晶体板和缺陷声子晶体板的能带结构和传输损失. 通过改变施加在压电散射体上下表面的电边界条件, 形成点缺陷波导, 以限制弹性波能量流, 该声子晶体板克服了材料参数和结构参数已确定的情况下振动波导方向不可变的局限性. 压电效应有利于完全带隙的扩大, 当缺陷态的频率出现在带隙内时, 缺陷态响应频率范围随之扩大, 因此可以收集更宽频率范围的机械能. 用振动能量回收电路连接缺陷处压电片上下表面的电极, 能够将振动所产生的机械能转化为电能.The band structure and transmission characteristics of a new two-dimensional (2D) piezoelectric phononic crystal plate consisting of four epoxy short plates periodically connected with a square lattice of a prismatic piezoelectric material coated with plexiglass are investigated by supercell method and finite element method. By changing the electric boundary conditions imposed on the upper and lower surfaces of piezoelectric scatterers, a point defect waveguide with adjustable paths is formed, which overcomes the limitation of immutability in the direction of the vibration waveguide, with material and structural parameters fixed. Then the controlling of the piezoelectric effect can change the material parameters of piezoelectric components in phononic crystals, showing that the piezoelectric constants have a great influence on the complete bandgap, which is beneficial to the formation of defect states; when the frequency of the defect state appears in the band gap, the frequency-responding range of the defect state expands. The analysis of the displacement vector field indicates that the strain energy in the resonance of the new structure is almost completely limited to the upper and lower surfaces of the central piezoelectric scatterer. We use the recycling circuit to connect the electrodes on the upper and lower surfaces of the piezoelectric sheet. At this time, the output electrical energy can supply the power to the DC load, and the mechanical energy of vibration can be converted into electrical energy. The results of this work provide a reference for the self-powered technology of waveguide and wireless sensor device with adjustable path.
-
Keywords:
- phononic crystal /
- finite element method /
- point defect /
- vibration energy recovery
[1] Kushwaha M S, Halevi P, Dobrzynski L, Djafari-Rouhani B 1993 Phys. Rev. Lett. 71 2022
Google Scholar
[2] Caballero D, Sánchez-Dehesa J, Rubio C, Mártinez-Sala R, Sánchez-Pérez J V, Meseguer F, Llinares J 1999 Phys. Rev. E 60 R6316
Google Scholar
[3] Wu F G, Liu Z Y, Liu Y Y 2004 Phys. Rev. E 69 066609
Google Scholar
[4] Lv H Y, Tian X Y, Wang M Y, Li D C 2013 Appl. Phys. Lett. 102 034103
Google Scholar
[5] Fox L, Zhang X 2011 Phys. Rev. B 83 214304
Google Scholar
[6] Khelif A, Djafari-Rouhani B, Vasseur J O, Deymier P A 2003 Phys. Rev. B 68 024302
[7] Zhao J F, Marchal R, Bonello B, Boyko O 2012 Appl. Phys. Lett. 101 261905
Google Scholar
[8] Jin Y B, Torrent D, Pennec Y, Pan Y D, Djafari-Rouhani B 2015 J. Appl. Phys. 117 244904
Google Scholar
[9] Kim M, Hong S, Miller D J, Dugundji J, Wardle B L 2011 Appl. Phys. Lett. 99 24
[10] Wu L Y, Chen L W, Liu C M 2009 Phys. Lett. A 373 1189
Google Scholar
[11] Sodano H A, Inman D J, Park G 2004 Shock Vib. Dig. 36 197
Google Scholar
[12] Anton S R, Sodano H A 2007 Smart Mater. Struct. 16 204
[13] Taylor G W, Burns J R, Kammann S M, Powers W B, Welsh T R 2001 IEEE J. Ocean. Eng. 26 539
Google Scholar
[14] Priya S, Chen C T, Fye D, Zahnd J 2005 J. Appl. Phys. 2 44
[15] Priya S 2005 Appl. Phys. Lett. 87 184101
Google Scholar
[16] Yu K P, Chen T N, Wang X P 2013 J. Appl. Phys. 416 12
[17] Hsu J C 2012 Jpn. J. Appl. Phys. 51 07GA04
Google Scholar
[18] Khelif A, Aoubiza B, Mohammadi S, Adibi A, Laude V 2006 Phys. Rev. E 74 046610
Google Scholar
[19] Wang W C, Chen L W, Liu C M 2010 Smart Mater. Struct. 19 045016
Google Scholar
[20] Lee K Y, Gupta M K, Kim S W 2015 Nano Energy 14 139
Google Scholar
[21] Poulin G, Sarraute E, Costa F 2004 Sens. Actuators 116 461
Google Scholar
[22] Roundy S, Leland E 2005 IEEE Pervasive Comput. 4 28
[23] Lu F, Lee H P, Lim S P 2004 Smart Mater. Struct. 13 57
Google Scholar
[24] Horowitz S B, Sheplak M, Cattafesta III L N, Nishida T 2006 J. Micromech. Microeng. 16 174
Google Scholar
[25] Liu F, Phipps A, Horowitz S, Ngo K, Cattafesta L, Nishida T, Sheplak M 2008 J. Acoust. Soc. Am. 123 1983
Google Scholar
[26] Wu L Y, Chen L W, Liu C M 2009 Appl. Phys. Lett. 95 013506
Google Scholar
[27] Qi S B, Oudich M, Li Y, Assouar B 2016 Appl. Phys. Lett. 108 263501
Google Scholar
[28] Gao W T, Xia J P, Sun H X, Yuan S Q, Ge Y, Liu X J 2019 Appl. Phys. Express 12 044002
Google Scholar
[29] Park C S, Shin Y C, Jo S H, Yoon H J, Choi W, Younb B D, Kim M 2019 Nano Energy 57 327
Google Scholar
[30] 廖涛, 孙小伟, 宋婷, 田俊红, 康太凤, 孙伟彬 2018 67 214208
Google Scholar
Liao T, Sun X W, Song T, Tian J H, Kang T F, Sun W B 2018 Acta Phys. Sin. 67 214208
Google Scholar
[31] COMSOL Multiphysics®v.5.3.cn.comsol.com. COMSOL AB, Stockholm, Sweden. http://cn.comsol.com/support/knowledge base/1223/[2018-1-1]
-
图 1 1 × 5超元胞二维压电声子晶体板及其初基原胞示意图 (a) 1 × 5超胞; (b)原胞立体图; (c)原胞平面图; (d)第一布里渊区(阴影部分为不可约布里渊区)
Fig. 1. 1 × 5 supercell two-dimensional piezoelectric phonon crystal plate and its primary cells: (a) The supercell plan; (b) the protocell stereogram; (c) the protocell floor plan; (d) the first Brillouin zone (the shadow part is the irreducible brillouin zone).
图 2 用于计算传输损失的有限结构
Fig. 2. The finite structure for the calculation of the transmission loss.
图 3 完美和缺陷周期性结构压电声子晶体板的能带结构示意图
Fig. 3. Schematic diagram of energy band structure of piezoelectric phononic crystal plate with perfect and periodic defect structures.
图 4 完美和缺陷周期性结构压电声子晶体板传输特性对比示意图
Fig. 4. Schematic diagram of transmission characteristics of piezoelectric phonon crystal plates with perfect and periodic defect structures.
图 5 1 × 5超元胞的两种不同点缺陷位置传输损失和能带结构 (a)点缺陷为模式B; (b)点缺陷为模式C
Fig. 5. The position transmission loss and band structures of two different defects in the 1 × 5 supercells: (a) The point defect is at pattern B; (b) the point defect is at pattern C.
图 6 超元胞的三种不同点缺陷位置的传输特性对比
Fig. 6. Comparison diagram of transmission characteristics of three different defect locations of the supercell.
图 7 压电声子晶体板缺陷态处于三种不同位置的位移矢量场
Fig. 7. Displacement vector fields of piezoelectric phonon crystal plates with defect states at three different positions.
图 8 压电常数对声子晶体板传输特性的影响
Fig. 8. Influence of piezoelectric constant on the transmission characteristics of phonon crystal plates.
图 9 压电常数e''对第五和第六完全带隙上边缘和下边缘(即缺陷态)的影响
Fig. 9. Effect of piezoelectric constant e'' on upper and lower edge (i.e. defect state) of fifth and sixth complete bandgap.
图 11 压电散射体上下表面电压波形图
Fig. 11. Voltage waveform of upper and lower surface of piezoelectric scatterer.
图 12 三种不同点缺陷位置的电阻R输出功率随激励电压的变化示意图
Fig. 12. Schematic diagram of resistance R output power varying with excitation voltage at three different defect positions
表 1 压电材料0.27PIN-0.4PMN-0.33PT的参数
Table 1. Piezoelectric material parameters of 0.27PIN-0.4PMN-0.33PT.
密度ρ/kg·m–3 弹性常数Cij/1010 N·m–2 压电常数e/C·m–2 介电常数ε/10–11 F·m–1 C11 C12 C13 C33 C44 C66 e15 e31 e33 ε11 ε33 8189 12.2 11.3 10.8 11.2 6.9 6.2 16.0 –2.7 18.6 4193 585 
下载: 导出CSV
表 2 弹性材料参数
Table 2. Material parameters of elastic materials.
密度ρ/kg·m–3 杨氏模量E/1010 Pa 剪切模量μ/1010 Pa 有机玻璃 1142 0.200 0.072 环氧树脂 1180 0.435 0.159
下载: 导出CSV
-
[1] Kushwaha M S, Halevi P, Dobrzynski L, Djafari-Rouhani B 1993 Phys. Rev. Lett. 71 2022
Google Scholar
[2] Caballero D, Sánchez-Dehesa J, Rubio C, Mártinez-Sala R, Sánchez-Pérez J V, Meseguer F, Llinares J 1999 Phys. Rev. E 60 R6316
Google Scholar
[3] Wu F G, Liu Z Y, Liu Y Y 2004 Phys. Rev. E 69 066609
Google Scholar
[4] Lv H Y, Tian X Y, Wang M Y, Li D C 2013 Appl. Phys. Lett. 102 034103
Google Scholar
[5] Fox L, Zhang X 2011 Phys. Rev. B 83 214304
Google Scholar
[6] Khelif A, Djafari-Rouhani B, Vasseur J O, Deymier P A 2003 Phys. Rev. B 68 024302
[7] Zhao J F, Marchal R, Bonello B, Boyko O 2012 Appl. Phys. Lett. 101 261905
Google Scholar
[8] Jin Y B, Torrent D, Pennec Y, Pan Y D, Djafari-Rouhani B 2015 J. Appl. Phys. 117 244904
Google Scholar
[9] Kim M, Hong S, Miller D J, Dugundji J, Wardle B L 2011 Appl. Phys. Lett. 99 24
[10] Wu L Y, Chen L W, Liu C M 2009 Phys. Lett. A 373 1189
Google Scholar
[11] Sodano H A, Inman D J, Park G 2004 Shock Vib. Dig. 36 197
Google Scholar
[12] Anton S R, Sodano H A 2007 Smart Mater. Struct. 16 204
[13] Taylor G W, Burns J R, Kammann S M, Powers W B, Welsh T R 2001 IEEE J. Ocean. Eng. 26 539
Google Scholar
[14] Priya S, Chen C T, Fye D, Zahnd J 2005 J. Appl. Phys. 2 44
[15] Priya S 2005 Appl. Phys. Lett. 87 184101
Google Scholar
[16] Yu K P, Chen T N, Wang X P 2013 J. Appl. Phys. 416 12
[17] Hsu J C 2012 Jpn. J. Appl. Phys. 51 07GA04
Google Scholar
[18] Khelif A, Aoubiza B, Mohammadi S, Adibi A, Laude V 2006 Phys. Rev. E 74 046610
Google Scholar
[19] Wang W C, Chen L W, Liu C M 2010 Smart Mater. Struct. 19 045016
Google Scholar
[20] Lee K Y, Gupta M K, Kim S W 2015 Nano Energy 14 139
Google Scholar
[21] Poulin G, Sarraute E, Costa F 2004 Sens. Actuators 116 461
Google Scholar
[22] Roundy S, Leland E 2005 IEEE Pervasive Comput. 4 28
[23] Lu F, Lee H P, Lim S P 2004 Smart Mater. Struct. 13 57
Google Scholar
[24] Horowitz S B, Sheplak M, Cattafesta III L N, Nishida T 2006 J. Micromech. Microeng. 16 174
Google Scholar
[25] Liu F, Phipps A, Horowitz S, Ngo K, Cattafesta L, Nishida T, Sheplak M 2008 J. Acoust. Soc. Am. 123 1983
Google Scholar
[26] Wu L Y, Chen L W, Liu C M 2009 Appl. Phys. Lett. 95 013506
Google Scholar
[27] Qi S B, Oudich M, Li Y, Assouar B 2016 Appl. Phys. Lett. 108 263501
Google Scholar
[28] Gao W T, Xia J P, Sun H X, Yuan S Q, Ge Y, Liu X J 2019 Appl. Phys. Express 12 044002
Google Scholar
[29] Park C S, Shin Y C, Jo S H, Yoon H J, Choi W, Younb B D, Kim M 2019 Nano Energy 57 327
Google Scholar
[30] 廖涛, 孙小伟, 宋婷, 田俊红, 康太凤, 孙伟彬 2018 67 214208
Google Scholar
Liao T, Sun X W, Song T, Tian J H, Kang T F, Sun W B 2018 Acta Phys. Sin. 67 214208
Google Scholar
[31] COMSOL Multiphysics®v.5.3.cn.comsol.com. COMSOL AB, Stockholm, Sweden. http://cn.comsol.com/support/knowledge base/1223/[2018-1-1]
下载:
计量
- 文章访问数: 10042
- PDF下载量: 129
- 被引次数: 0
