-
提出了一种结合大气声场模拟与中近程超压幅度衰减模型的爆炸声源能量估计方法, 针对传统声源能量估计公式未能充分利用大气参数导致估计误差过大的问题, 本方法通过对大气中传播损失的数值模拟, 大大提高了大气参数对于声源能量估计的修正效果, 提高对声源能量的估计精度. 在地表化学爆炸实验中, 使用300—2500 km距离的次声接收信号, 对比了传统能量估计公式与基于大气声场模拟的能量估计方法对爆炸声源的能量估计效果. 实验结果验证了相对于传统声源能量估计方法, 该方法降低声源能量估计误差的有效性.
-
关键词:
- 声源能量估计 /
- 非线性渐进波动方程 /
- 中近程超压幅值衰减模型
A method of estimating the explosion sound source energy is proposed by combining atmospheric sound field simulation with mid- and short-range overpressure amplitude attenuation model. Aiming at the problem that the traditional sound source energy estimation formula fails to make full use of the atmospheric parameters and the estimation error is too large, through numerically simulating the propagation loss in the atmosphere, the correction effectiveness of the atmospheric parameters to the sound source energy estimation is greatly improved and the estimation accuracy of the sound source energy is enhanced. In the surface chemical explosion experiment, the infrasound is used to receive the signal from 300 km to 2500 km away. A comparison of energy estimation effectiveness between the traditional energy estimation formula and the energy estimation method based on atmospheric sound field simulation of the explosion sound source is made. The experimental results indicate that this method is effective in improving the robustness of yield estimation in comparison with the traditional yield estimation method.-
Keywords:
- source energy estimation /
- nonlinear progressive wave equation /
- middle range overpressure attenuation model
[1] Clauter D, Blandford R 1998 Proceedings of the Infrasound Workshop for CTBT Monitoring Santa Fe, New Mexico, August 25–28, 1997, LANL report number LA-UR-98-56
[2] [3] Stevens J L, Divnov I I, Adams A A, Murphy J R, Bourchik V N 2002 Pure Appl. Geophys. 159 1045
Google Scholar
[4] Kulichkov S 2002 Izv. Atmos. Oceanic Phys. 38 582
[5] 杨训仁, 陈宇 2007 大气声学 (北京: 科学出版社) 第52—59页
Yang X R, Chen Y 2007 Atmospheric Acoustic (Beijing: Science Press) pp52–59 (in Chinese)
[6] 余师倩 2012 硕士学位论文 (武汉: 武汉大学)
Yu S Q 2012 M. S. Thesis (Wuhan: Wuhan University) (in Chinese)
[7] Edward M B, Piacsek A A 2011 J. Acoust. Soc. Am. 130 2648
Google Scholar
[8] Whitaker R W, Norris D E 2008 Handbook of Signal Processing in Acoustics (New York: Springer) pp1497–1519
[9] 钱祖文 2009 非线性声学 (北京: 科学出版社) 第33—35页
Qian Z W 2009 Nonlinear Acoustic (Beijing: Science Press) pp33–35 (in Chinese)
[10] Mcdonald B E 2000 Wave Motion 31 165
Google Scholar
[11] 傅竹风, 胡友秋 1995 空间等离子体数值模拟 (合肥: 安徽科学技术出版社) 第103, 104页
Fu Z F, Hu Y Q 1995 Numerical Simulation of Space Plasma (Hefei: Anhui Science and Technology Press) pp103, 104 (in Chinese)
[12] Védy E 2002 Noise Control Eng. J. 50 211
Google Scholar
[13] Collino F 1997 J. Comput. Phys. 131 164
Google Scholar
[14] Kim K, Rodgers A 2016 Geophys. Res. Lett. 43 6883
Google Scholar
[15] Kinney G F, Graham K J 2013 Explosive Shocks in Air (New York: Springer Science & Business Media) pp88–98
[16] Le Pichon A, Blanc E, Hauchecorne A 2019 Infrasound Monitoring for Atmospheric Studies (Cham: Springer Nature Switzerland AG) pp273–277
[17] 杨鑫, 石少卿, 程鹏飞 2008 爆破 25 15
Google Scholar
Yang X, Shi S Q, Cheng P F 2008 Blasting 25 15
Google Scholar
[18] 王儒策, 赵国志 1993 弹丸终点效应 (北京: 北京理工大学出版社) 第75—77页
Wang R C, Zhao G Z 1993 Terminal Effect of Projectile (Beijing: Beijing Institute of Technology Press) pp75–77 (in Chinese)
[19] [20] Fee D, Waxler R, Assink J, Gitterman Y, Given J, Coyne J, Mialle P, Garces M, Drob D, Kleinert D 2013 J. Geophys. Res. Atmos. 118 6122
Google Scholar
-
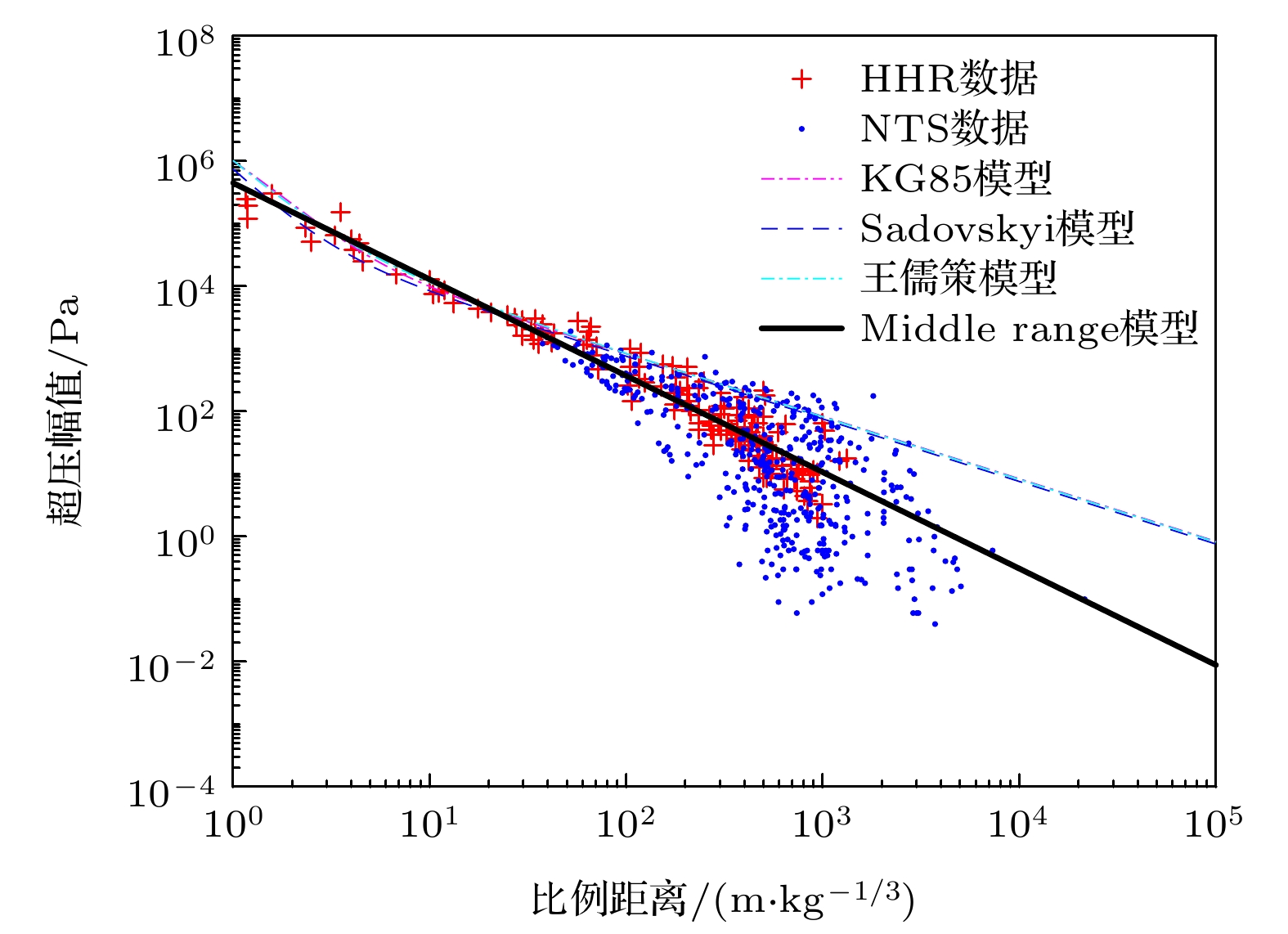
图 2 4个主要传播方向的NPE传播模拟结果 (a)方位角14°方向的传播损失分布; (b)方位角50°方向的传播损失分布; (c)方位角95.5°方向的传播损失分布; (d)方位角113°方向的传播损失分布
Fig. 2. NPE propagation simulations in four main propagation directions: (a) Propagation loss distribution in the azimuth of 14°; (b) propagation loss distribution in the azimuth of 50°; (c) propagation loss distribution in the azimuth of 95.5°; (d) distribution of propagation loss in the azimuth of 113°.
表 1 2011年Sayarim实验各接收点数据与参数
Table 1. Parameters of each array in Sayarim experiment in 2011.
站点 声压 距离 方位角 修正风速 Prt/Pa r/km $ \phi /(°) $ V50/(m·s–1) IN1 1.5 308.3 14.4 9.17 IN2 1 330 14.1 9.06 JO_NE 1 433.8 50.2 19.14 KU 0.25 1252.8 95.5 21.18 QA 0.25 1688.1 114 18.19 OM_N 0.05 2420.2 112.1 18.59 
下载: 导出CSV
表 2 参考距离为7, 20 km时声源能量估计结果对比
Table 2. Comparison between sound source energy estimation results at reference distance of 7, 20 km
站点 声压 能量 误差/% 能量 误差/% Prt/Pa ${\widehat{W} }_{7~\mathrm{k}\mathrm{m} }/\mathrm{t}$ $ {\widehat{W}}_{20\; \mathrm{k}\mathrm{m}}/\mathrm{t} $ IN1 1.5 8.99 21 37.34 405 IN2 1 5.54 –25 23.00 211 JO_NE 1 0.93 –87 4.21 –43 KU 0.25 0.34 –95 1.61 –78 QA 0.25 6.97 –6 33.28 350 OM_N 0.05 5.35 –28 25.55 245
下载: 导出CSV
表 3 2011年Sayarim实验声源能量估计结果对比
Table 3. Comparison between sound source energy estimation results in Sayarim experiment in 2011.
站点 声压 LANL
估计值误差/% NPE_MR
估计值误差/% Prt/Pa ${\widehat{ {W} } }_{\mathrm{L}\mathrm{A}\mathrm{N}\mathrm{L} }/\mathrm{t}$ ${\widehat{W} }_{7~\mathrm{k}\mathrm{m} }/\mathrm{t}$ IN1 1.5 217.07 2833 8.99 21 IN2 1 137.88 1763 5.54 –25 JO_NE 1 131.58 1678 0.93 –87 KU 0.25 126.76 1613 0.34 –95 QA 0.25 274.45 3609 6.97 –6 OM_N 0.05 51.52 599 5.35 –28
下载: 导出CSV
-
[1] Clauter D, Blandford R 1998 Proceedings of the Infrasound Workshop for CTBT Monitoring Santa Fe, New Mexico, August 25–28, 1997, LANL report number LA-UR-98-56
[2] [3] Stevens J L, Divnov I I, Adams A A, Murphy J R, Bourchik V N 2002 Pure Appl. Geophys. 159 1045
Google Scholar
[4] Kulichkov S 2002 Izv. Atmos. Oceanic Phys. 38 582
[5] 杨训仁, 陈宇 2007 大气声学 (北京: 科学出版社) 第52—59页
Yang X R, Chen Y 2007 Atmospheric Acoustic (Beijing: Science Press) pp52–59 (in Chinese)
[6] 余师倩 2012 硕士学位论文 (武汉: 武汉大学)
Yu S Q 2012 M. S. Thesis (Wuhan: Wuhan University) (in Chinese)
[7] Edward M B, Piacsek A A 2011 J. Acoust. Soc. Am. 130 2648
Google Scholar
[8] Whitaker R W, Norris D E 2008 Handbook of Signal Processing in Acoustics (New York: Springer) pp1497–1519
[9] 钱祖文 2009 非线性声学 (北京: 科学出版社) 第33—35页
Qian Z W 2009 Nonlinear Acoustic (Beijing: Science Press) pp33–35 (in Chinese)
[10] Mcdonald B E 2000 Wave Motion 31 165
Google Scholar
[11] 傅竹风, 胡友秋 1995 空间等离子体数值模拟 (合肥: 安徽科学技术出版社) 第103, 104页
Fu Z F, Hu Y Q 1995 Numerical Simulation of Space Plasma (Hefei: Anhui Science and Technology Press) pp103, 104 (in Chinese)
[12] Védy E 2002 Noise Control Eng. J. 50 211
Google Scholar
[13] Collino F 1997 J. Comput. Phys. 131 164
Google Scholar
[14] Kim K, Rodgers A 2016 Geophys. Res. Lett. 43 6883
Google Scholar
[15] Kinney G F, Graham K J 2013 Explosive Shocks in Air (New York: Springer Science & Business Media) pp88–98
[16] Le Pichon A, Blanc E, Hauchecorne A 2019 Infrasound Monitoring for Atmospheric Studies (Cham: Springer Nature Switzerland AG) pp273–277
[17] 杨鑫, 石少卿, 程鹏飞 2008 爆破 25 15
Google Scholar
Yang X, Shi S Q, Cheng P F 2008 Blasting 25 15
Google Scholar
[18] 王儒策, 赵国志 1993 弹丸终点效应 (北京: 北京理工大学出版社) 第75—77页
Wang R C, Zhao G Z 1993 Terminal Effect of Projectile (Beijing: Beijing Institute of Technology Press) pp75–77 (in Chinese)
[19] [20] Fee D, Waxler R, Assink J, Gitterman Y, Given J, Coyne J, Mialle P, Garces M, Drob D, Kleinert D 2013 J. Geophys. Res. Atmos. 118 6122
Google Scholar
下载:
计量
- 文章访问数: 3902
- PDF下载量: 60
- 被引次数: 0
