光子增加双模压缩真空态在马赫-曾德尔干涉仪相位测量中的应用

王帅; 眭永兴; 孟祥国

doi:10.7498/aps.69.20200179

摘要

量子度量学主要是利用量子效应来提高参数估计的精度, 以期突破标准量子极限, 甚至达到海森伯极限. 本文研究了一般光子增加双模压缩真空态作为马赫-曾德尔干涉仪的探测态时, 在何种情况下能够提高待测相位的测量精度. 根据量子Fisher信息理论, 尽管在探测态具有相同的平均光子数这一约束条件下, 对称的和非对称的光子增加操作并不能提高相位的测量精度. 但若是在给定初始压缩参数的情况下, 对称的和非对称的光子增加操作却能够增强相位的测量精度. 另外, 基于宇称测量的研究结果表明, 对于对称光子增加双模压缩真空态, 只有当待测相位趋于零时, 宇称测量才是最优测量. 而对于非对称光子增加双模压缩真空态, 宇称测量并不是最优测量方案.

关键词:

Abstract

Quantum metrology is to estimate accurately the value of an unknown parameter with the assistance of the quantum effects, in order to break through the standard quantum limit, even reach the Heisenberg limit. In this work, we study the performance of a general photon-added two-mode squeezed vacuum state that is taken as a detection state of a Mach-Zehnder interferometer. Based on quantum Fisher information, within the constraint on the total mean photon number, symmetric and asymmetric photon addition cannot improve the ultimate phase sensitivity. However, for a given initial squeezing parameter, on this occasion, the symmetric and asymmetric photon addition can improve the ultimate phase sensitivity. Compared with the asymmetric photon-added two-mode squeezed vacuum state, the symmetric one can well improve the ultimate phase sensitivity. This may be because it is always better to implement the symmetric photon addition rather than the asymmetric one in order to increase the mean photon number of the resulting state. On the other hand, via parity detection, the symmetric and asymmetric photon-added two-mode squeezed vacuum state can indeed improve the phase sensitivity of a Mach-Zehnder interferometer for a given initial squeezing parameter. Based on the parity detection, within a constraint on the mean photon number, although the two-mode squeezed vacuum state can give the better phase sensitivity at the optimal phase shift (φ = 0), the phase sensitivity offered by the symmetric and asymmetric photon-added two-mode squeezed vacuum states are both more stable around φ = 0 than by the two-mode squeezed vacuum state. In addition, we show that for the symmetric photon-added two-mode squeezed vacuum state, parity detection is an optimal detection only when the optimal phase shift approaches to zero. When the phase shift slightly deviates from zero, the parity detection is not an optimal detection scheme. Finally, for all values of the phase shift, our results also clearly show that the parity detection is not an optimal detection scheme for the asymmetric photon-added two-mode squeezed vacuum state serving as an interferometer state.

Keywords:

作者及机构信息

1.
江苏理工学院数理学院, 常州　213001

2.
聊城大学物理科学与信息工程学院, 聊城　252059

通信作者: 王帅, wshslxy@jsut.edu.cn

基金项目: 国家级-国家自然科学基金(11404040)

Authors and contacts

1.
School of Mathematics and Physics, Jiangsu University of Technology, Changzhou 213001, China

2.
School of Physics Science and Information Technology, Liaocheng University, Liaocheng 252059, China

Corresponding author: Wang Shuai, wshslxy@jsut.edu.cn

文章全文 : translate this paragraph

参考文献

[1]	Caves C M 1981 Phys. Rev. D 23 1693 Google Scholar
[2]	Holland M J, Burnett K 1993 Phys. Rev. Lett. 71 1355 Google Scholar
[3]	Campos R A, Gerry C C, Benmoussa A 2003 Phys. Rev. A 68 023810 Google Scholar
[4]	Wang S, Wang Y T, Zhai L J, Zhang L J 2018 J. Opt. Soc. Am. B 35 1046 Google Scholar
[5]	王帅, 吴世臣, 眭永兴 2018 聊城大学学报 120 77 Wang S, Wu S C, Sui Y X 2018 Journal of Liaocheng University 120 77
[6]	Dowling J P 2008 Contemp. Phys. 49 125 Google Scholar
[7]	Joo J, Munro W J, Spiller T P 2011 Phys. Rev. Lett. 107 083601 Google Scholar
[8]	Ou Z Y 1996 Phys. Rev. Lett. 77 2352 Google Scholar
[9]	Wenger J, Tualle-Brouri R, Grangier P 2004 Phys. Rev.Lett. 92 153601 Google Scholar
[10]	Zavatta A, Viciani S, Bellini M 2004 Science 306 660 Google Scholar
[11]	Zavatta A, Parigi V, Bellini M 2007 Phys. Rev. A 75 052106 Google Scholar
[12]	Gerrits T, Glancy S, Clement T S, Calkins B, Lita A E, Miller A J, Migdall A L, Nam S W, Mirin R P, Knill E 2010 Phys. Rev. A 82 031802(R)Google Scholar
[13]	León-Montiel R J, Magaña-Loaiza O S, Perez-Leija A, U’Ren A, Busch K, Lita A E, Nam S W, Gerrits T, Mirin R R 2018 Frontiers in Optics/Laser Science Washington, DC, USA, September 16–20, 2018 paper LM1B.6
[14]	Magaña-Loaiza O S, León-Montiel R J, Perez-Leija A, U’Ren A B, You C, Busch K, Lita A E, Nam S W, Mirin R R, Gerrits T 2019 npj Quantum Inf. 5 80
[15]	Dell’ Anno F, De Siena S, Illuminati F 2006 Phys. Rep. 428 53
[16]	Kim M S, 2008 J. Phys. B: At. Mol. Opt. Phys. 41 133001 Google Scholar
[17]	Wang S, Hou L L, Chen X F, Xu X F 2015 Phys. Rev. A 91 063832 Google Scholar
[18]	Navarrete-Benlloch C, García-Patrón R, Shapiro J H, Cerf N J 2012 Phys. Rev. A 86 012328
[19]	Liao Q, Guo Y, Huang D, Huang P, Zeng G H 2018 New J. Phys. 20 023015 Google Scholar
[20]	Guo Y, Ye W, Zhong H, Liao Q 2019 Phys. Rev. A 99 032327 Google Scholar
[21]	Carranza R, Gerry C C 2012 J. Opt. Soc. Am. B 29 2581 Google Scholar
[22]	Birrittella R, Gerry C C 2014 J. Opt. Soc. Am. B 31 586 Google Scholar
[23]	Gong Q K, Hu X L, Li D, Yuan C H, Ou Z Y, Zhang W 2017 Phys. Rev. A 96 033809 Google Scholar
[24]	Guo L L, Yu Y F, Zhang Z M 2018 Opt. Express 26 29099
[25]	Ouyang Y, Wang S, Zhang L 2016 J. Opt. Soc. Am. B 33 1373 Google Scholar
[26]	Wang S, Xu X X, Xu Y J, Zhang L J 2019 Opt. Commun. 444 102 Google Scholar
[27]	鹿博, 韩成银, 庄敏, 柯勇惯, 黄嘉豪, 李朝红 2019 68 040306 Google Scholar Lu B, Han C Y, Zhuang M, Ke Y G, Huang J H, Li C H 2019 Acta Phys. Sin. 68 040306 Google Scholar
[28]	Gerry C C, Mimih J 2010 Contemp. Phys. 51 497 Google Scholar
[29]	Lang M D, Caves C M 2013 Phys. Rev. Lett. 111 173601 Google Scholar
[30]	Stephen M B, Gergely F, Claire R G, Fiona C S 2018 Phys. Rev. A 98 013809 Google Scholar
[31]	Zhong W, Wang F, Zhou L, Xu P, Sheng Y B, 2020 Sci. China-Phys. Mech. Astron. 63 260312 Google Scholar
[32]	Anisimov P M, Raterman G M, Chiruvelli A, Plick W N, Huver S D, Lee H, Dowling J P 2010 Phys. Rev. Lett. 104 103602 Google Scholar
[33]	Eberle T, Händchen V, Schnabel R 2013 Opt. Express 21 11546 Google Scholar
[34]	Gerry C C, Mimih J 2010 Phys. Rev. A 82 013831 Google Scholar
[35]	Hou L L, Xue J Z, Sui Y X, Wang S 2019 Chin. Phys. B 28 094217 Google Scholar
[36]	Hu L Y, Xu X X, Fan H Y 2010 J. Opt. Soc. Am. B 27 286
[37]	Yurke B, McCall S L, Klauder J R 1986 Phys. Rev. A 33 4033 Google Scholar
[38]	Braunstein S L, Caves C M 1994 Phys. Rev. Lett. 72 3439 Google Scholar
[39]	Zhong W, Lu X M, Jing X X, Wang X G 2014 J. Phys. A: Math. Theor. 47 385304 Google Scholar
[40]	Ben-Aryeh Y 2012 J. Opt. Soc. Am. B 29 2754 Google Scholar
[41]	Tan Q S, Liao J Q, Wang X G, Nori F 2014 Phys. Rev. A 89 053822 Google Scholar
[42]	Pezzé L, Smerzi A 2008 Phys. Rev. Lett. 100 073601 Google Scholar
[43]	Seshadreesan K P, Kim S, Dowling J P, Lee H 2013 Phys. Rev. A 87 043833 Google Scholar
[44]	Yu X D, Li W, Zhu S Y, Zhang J 2016 Chin. Phys. B 25 020304 Google Scholar
[45]	Huang Z X, Motes K R, Anisimov P M, Dowling J P, Berry D W 2017 Phys. Rev. A 95 053837 Google Scholar
[46]	Fan H Y, Ruan T N 1983 Commun. Theor. Phys. 2 1563 Google Scholar
[47]	Hu L Y, Fan H Y 2008 J. Opt. Soc. Am. B 25 1955 Google Scholar
[48]	Oh C, Lee S Y, Nha H, Jeong H 2017 Phys. Rev. A 96 062304 Google Scholar
[49]	Steuernagel O, Scheel S 2004 J. Phys. B: Quantum Semicalss Opt. 6 S66 Google Scholar

施引文献

图 1 当对双模压缩真空态增加或扣除不同的光子数($ k, l $)时, 相应的非高斯态的平均光子数随压缩参数z的变化曲线　 (a)光子增加双模压缩真空态; (b)光子扣除双模压缩真空态; 不同颜色类型的曲线表示不同的($ k, l $)取值

Fig. 1. Added to or subtracted from a two-mode squeezed vacuum state with different photon numbers ($ k, l $), the mean photon number variation of these corresponding non-Gaussian states with the squeezed parameter z: (a) Photon-added two-mode squeezed vacuum state; (b) photon-subtracted two-mode squeezed vacuum state. Different color curves correspond to different values of ($ k, l $).

下载: 全尺寸图片幻灯片

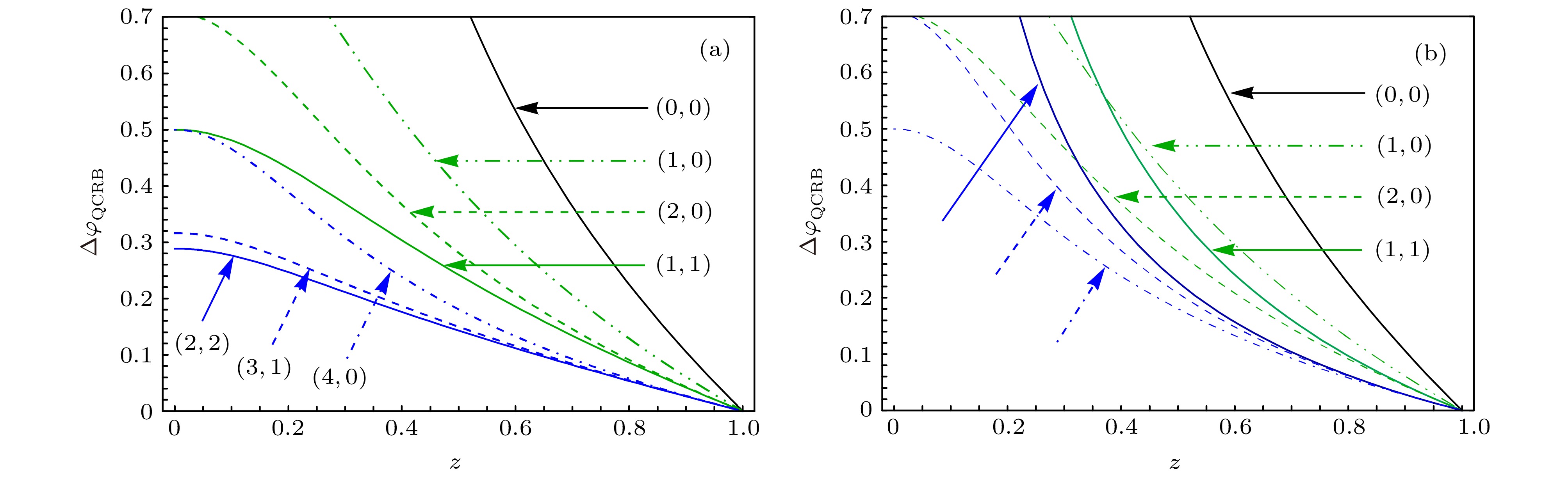
图 2 相位测量精度的最终极限$ \Delta \varphi _{\rm{QCRB}} $随压缩参数z的变化曲线　(a)光子增加双模压缩真空态; (b)光子扣除双模压缩真空态; 不同颜色类型的曲线表示对双模压缩真空态执行不同光子数($ k, l $)的增加或扣除操作

Fig. 2. Ultimate limit of the phase sensitivity $ \Delta \varphi _{\rm{QCRB}} $ as a function of the squeezing parameter z : (a) The photon-added two-mode squeezed vacuum state; (b) the photon-subtracted two-mode squeezed vacuum state. Different color curves correspond to add to or subtract from a two-mode squeezed vacuum state with different photon numbers ($ k, l $)

下载: 全尺寸图片幻灯片

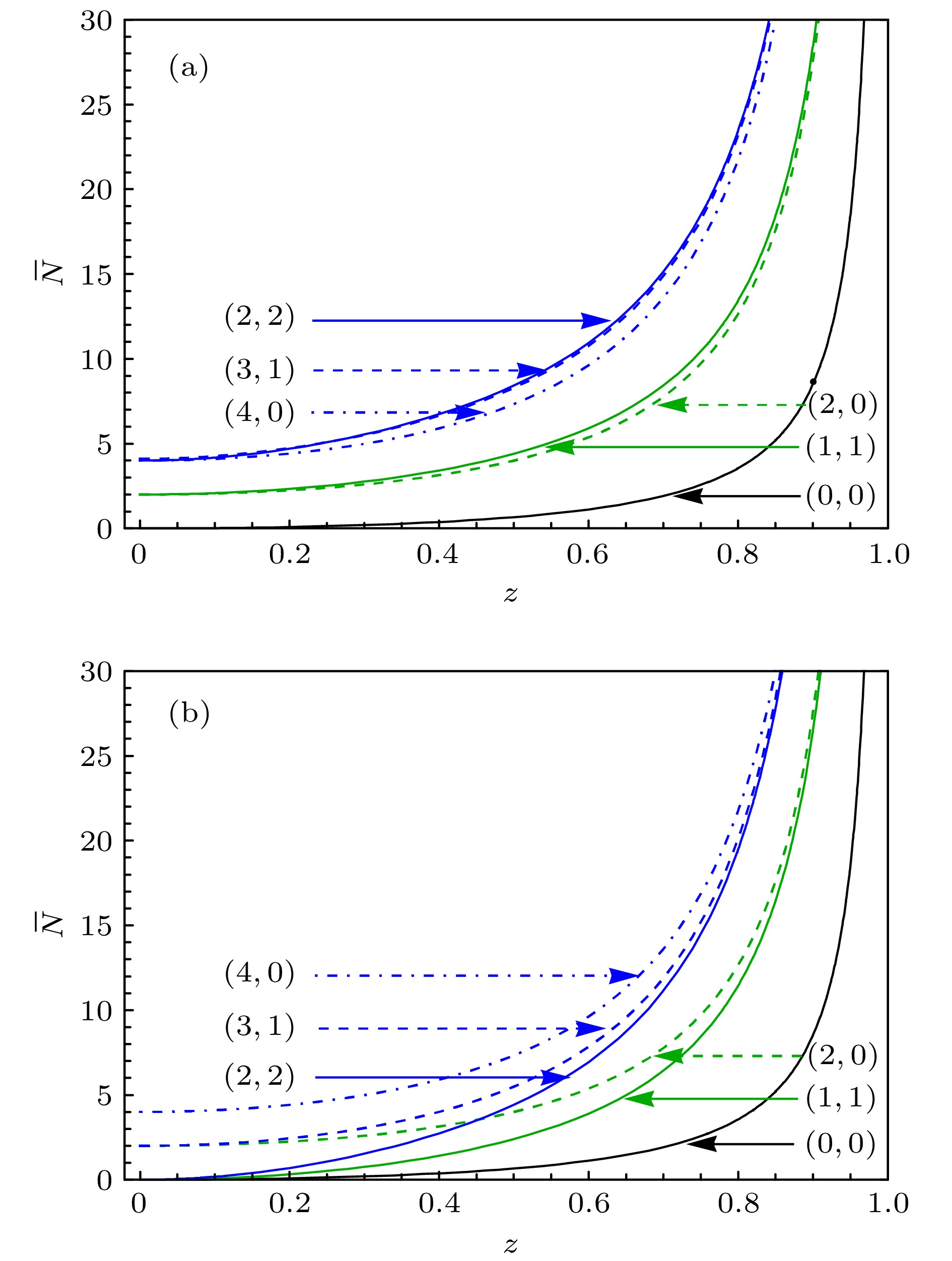
图 3 相位测量精度的最终极限$ \Delta \varphi _{\rm{QCRB}} $随平均光子数的变化曲线　(a)光子增加双模压缩真空态; (b)光子扣除双模压缩真空态; 不同颜色类型的曲线表示对双模压缩真空态执行不同光子数($ k, l $)的增加或扣除操作; 最上面的黑色虚线表示SNL极限, 而下面的黑色虚线则表示HL极限

Fig. 3. Ultimate limit variation of the phase sensitivity $ \Delta \varphi _{\rm{QCRB}} $ with the mean photon number: (a) The photon-added two-mode squeezed vacuum state; (b) the photon-subtracted two-mode squeezed vacuum state. Different color curves correspond to add to or subtract from a two-mode squeezed vacuum state with different photon numbers ($ k, l $). The upper black dashed line denotes the SNL limit, while the below black dashed line represents the HL limit.

下载: 全尺寸图片幻灯片

图 4 对于给定$ z = 0.6 $和一些不同的($ k, l $)值, 宇称测量的信号值随待测相位的变化曲线

Fig. 4. Plot of the signal values of the parity detection against the phase shift for $ z = 0.6 $ and some values of ($ k, l $)

下载: 全尺寸图片幻灯片

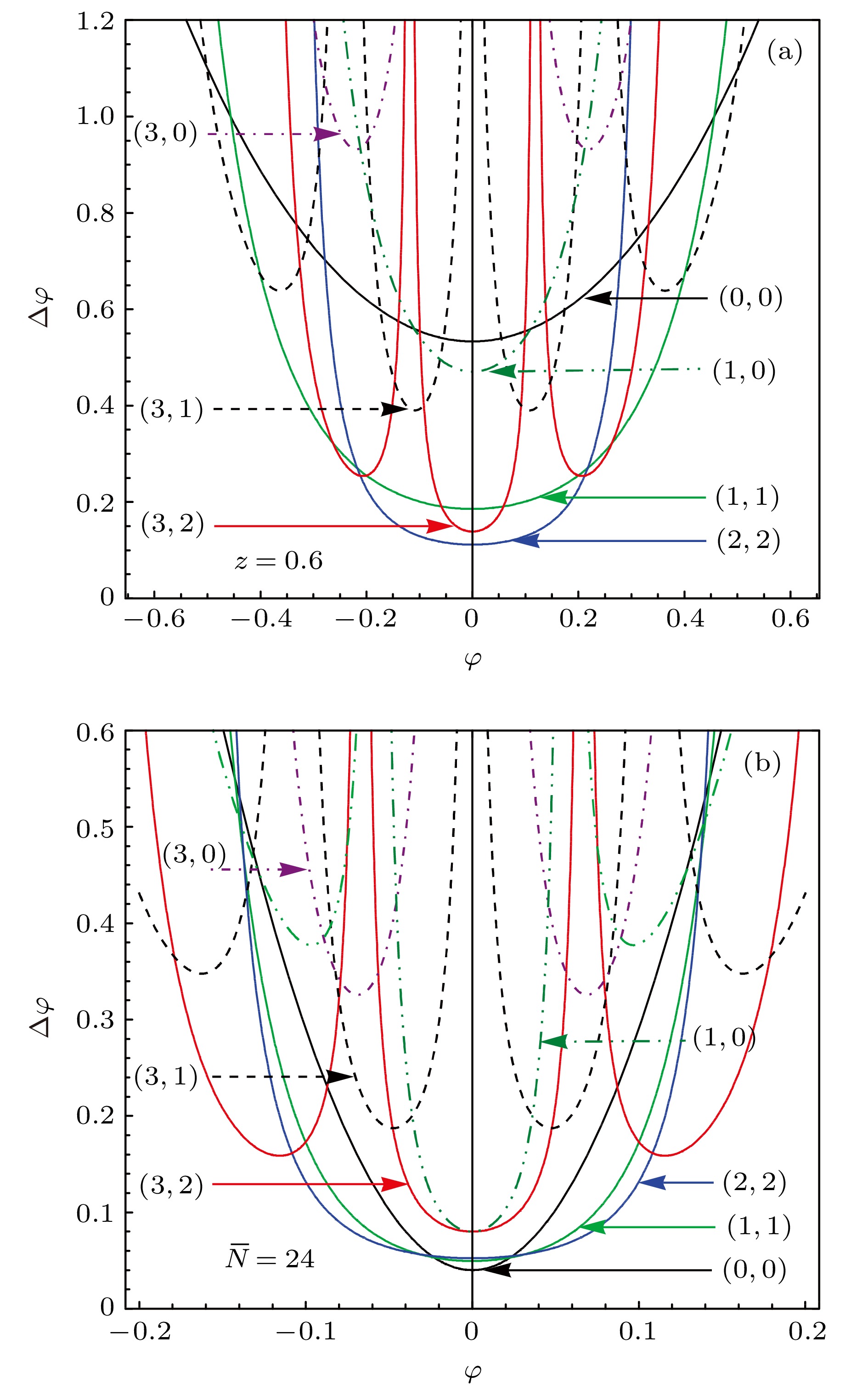
图 5 对于光子增加双模压缩真空态作为干涉仪的探测态, 当($ k, l $)取不同值时, $ \Delta \varphi $随待测相位$ \varphi $的变化　(a)给定初始压缩参数$ z = 0.6 $; (b)给定相同的平均光子数$ \bar{N} = 24 $

Fig. 5. Phase sensitivity $ \Delta \varphi $ as a function of the phase shift $ \varphi $ for some values of ($ k, l $) when the photon-added two-mode squeezed vacuum state is considered as interferometer states: (a) For a given initial squeezing parameter $ z = 0.6 $; (b) for a given mean photon number $ \bar{N} = 24 $.

下载: 全尺寸图片幻灯片

图 6 在$ \varphi = 10^{-3} $时, 对于光子增加双模压缩真空态的($ k, l $)不同取值, 相位测量精度$ \Delta \varphi $随平均光子数的变化曲线　(a)对称光子增加的情况($ k = l $); (b)非对称光子增加的情况($ k = l+1 $); 最上面的黑色虚线表示SNL极限, 下面的黑色虚线则表示HL极限

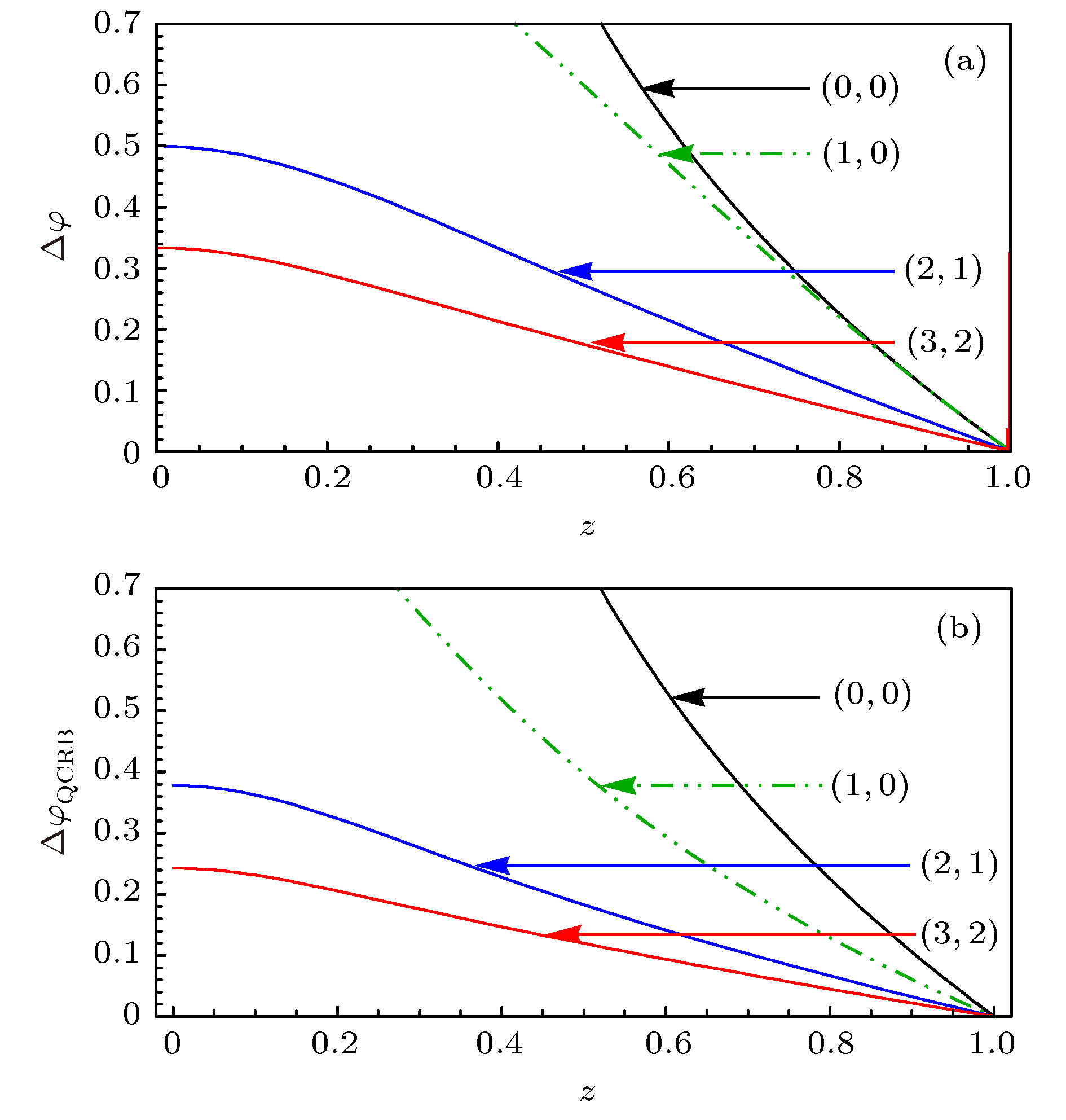
Fig. 6. Plots of the phase sensitivity $ \Delta \varphi $ as a function of the mean photon number of the photon-added two-mode squeezed vacuum state for some different values of ($ k, l $) at $ \varphi = 10^{-3} $: (a) Symmetric photon-added two-mode squeezed vacuum state ($ k = l $); (b) asymmetric photon-added two-mode squeezed vacuum state ($ k = l+1 $). The upper black dashed line denotes the SNL limit, while the below black dashed line represents the HL limit.

下载: 全尺寸图片幻灯片

图 7 $ \varphi = 10^{-3} $和给定不同($ k, l $)取值时, 相位测量精度随初始压缩参数z的变化　(a)基于宇称测量得到的相位测量精度; (b)基于量子Fisher信息得到的最终测量界限$ \Delta \varphi _{\rm{QCRB}} $

Fig. 7. Phase sensitivity as a function of the initial squeezing parameter z for different values of ($ k, l $) at $ \varphi = 10^{-3} $: (a) The phase sensitivity obtained by the parity detection; (b) the ultimate limit of phase sensitivity $ \Delta \varphi _{\rm{QCRB}} $ obtained by the quantum Fisher information.

下载: 全尺寸图片幻灯片

map

[1]	Caves C M 1981 Phys. Rev. D 23 1693 Google Scholar
[2]	Holland M J, Burnett K 1993 Phys. Rev. Lett. 71 1355 Google Scholar
[3]	Campos R A, Gerry C C, Benmoussa A 2003 Phys. Rev. A 68 023810 Google Scholar
[4]	Wang S, Wang Y T, Zhai L J, Zhang L J 2018 J. Opt. Soc. Am. B 35 1046 Google Scholar
[5]	王帅, 吴世臣, 眭永兴 2018 聊城大学学报 120 77 Wang S, Wu S C, Sui Y X 2018 Journal of Liaocheng University 120 77
[6]	Dowling J P 2008 Contemp. Phys. 49 125 Google Scholar
[7]	Joo J, Munro W J, Spiller T P 2011 Phys. Rev. Lett. 107 083601 Google Scholar
[8]	Ou Z Y 1996 Phys. Rev. Lett. 77 2352 Google Scholar
[9]	Wenger J, Tualle-Brouri R, Grangier P 2004 Phys. Rev.Lett. 92 153601 Google Scholar
[10]	Zavatta A, Viciani S, Bellini M 2004 Science 306 660 Google Scholar
[11]	Zavatta A, Parigi V, Bellini M 2007 Phys. Rev. A 75 052106 Google Scholar
[12]	Gerrits T, Glancy S, Clement T S, Calkins B, Lita A E, Miller A J, Migdall A L, Nam S W, Mirin R P, Knill E 2010 Phys. Rev. A 82 031802(R)Google Scholar
[13]	León-Montiel R J, Magaña-Loaiza O S, Perez-Leija A, U’Ren A, Busch K, Lita A E, Nam S W, Gerrits T, Mirin R R 2018 Frontiers in Optics/Laser Science Washington, DC, USA, September 16–20, 2018 paper LM1B.6
[14]	Magaña-Loaiza O S, León-Montiel R J, Perez-Leija A, U’Ren A B, You C, Busch K, Lita A E, Nam S W, Mirin R R, Gerrits T 2019 npj Quantum Inf. 5 80
[15]	Dell’ Anno F, De Siena S, Illuminati F 2006 Phys. Rep. 428 53
[16]	Kim M S, 2008 J. Phys. B: At. Mol. Opt. Phys. 41 133001 Google Scholar
[17]	Wang S, Hou L L, Chen X F, Xu X F 2015 Phys. Rev. A 91 063832 Google Scholar
[18]	Navarrete-Benlloch C, García-Patrón R, Shapiro J H, Cerf N J 2012 Phys. Rev. A 86 012328
[19]	Liao Q, Guo Y, Huang D, Huang P, Zeng G H 2018 New J. Phys. 20 023015 Google Scholar
[20]	Guo Y, Ye W, Zhong H, Liao Q 2019 Phys. Rev. A 99 032327 Google Scholar
[21]	Carranza R, Gerry C C 2012 J. Opt. Soc. Am. B 29 2581 Google Scholar
[22]	Birrittella R, Gerry C C 2014 J. Opt. Soc. Am. B 31 586 Google Scholar
[23]	Gong Q K, Hu X L, Li D, Yuan C H, Ou Z Y, Zhang W 2017 Phys. Rev. A 96 033809 Google Scholar
[24]	Guo L L, Yu Y F, Zhang Z M 2018 Opt. Express 26 29099
[25]	Ouyang Y, Wang S, Zhang L 2016 J. Opt. Soc. Am. B 33 1373 Google Scholar
[26]	Wang S, Xu X X, Xu Y J, Zhang L J 2019 Opt. Commun. 444 102 Google Scholar
[27]	鹿博, 韩成银, 庄敏, 柯勇惯, 黄嘉豪, 李朝红 2019 68 040306 Google Scholar Lu B, Han C Y, Zhuang M, Ke Y G, Huang J H, Li C H 2019 Acta Phys. Sin. 68 040306 Google Scholar
[28]	Gerry C C, Mimih J 2010 Contemp. Phys. 51 497 Google Scholar
[29]	Lang M D, Caves C M 2013 Phys. Rev. Lett. 111 173601 Google Scholar
[30]	Stephen M B, Gergely F, Claire R G, Fiona C S 2018 Phys. Rev. A 98 013809 Google Scholar
[31]	Zhong W, Wang F, Zhou L, Xu P, Sheng Y B, 2020 Sci. China-Phys. Mech. Astron. 63 260312 Google Scholar
[32]	Anisimov P M, Raterman G M, Chiruvelli A, Plick W N, Huver S D, Lee H, Dowling J P 2010 Phys. Rev. Lett. 104 103602 Google Scholar
[33]	Eberle T, Händchen V, Schnabel R 2013 Opt. Express 21 11546 Google Scholar
[34]	Gerry C C, Mimih J 2010 Phys. Rev. A 82 013831 Google Scholar
[35]	Hou L L, Xue J Z, Sui Y X, Wang S 2019 Chin. Phys. B 28 094217 Google Scholar
[36]	Hu L Y, Xu X X, Fan H Y 2010 J. Opt. Soc. Am. B 27 286
[37]	Yurke B, McCall S L, Klauder J R 1986 Phys. Rev. A 33 4033 Google Scholar
[38]	Braunstein S L, Caves C M 1994 Phys. Rev. Lett. 72 3439 Google Scholar
[39]	Zhong W, Lu X M, Jing X X, Wang X G 2014 J. Phys. A: Math. Theor. 47 385304 Google Scholar
[40]	Ben-Aryeh Y 2012 J. Opt. Soc. Am. B 29 2754 Google Scholar
[41]	Tan Q S, Liao J Q, Wang X G, Nori F 2014 Phys. Rev. A 89 053822 Google Scholar
[42]	Pezzé L, Smerzi A 2008 Phys. Rev. Lett. 100 073601 Google Scholar
[43]	Seshadreesan K P, Kim S, Dowling J P, Lee H 2013 Phys. Rev. A 87 043833 Google Scholar
[44]	Yu X D, Li W, Zhu S Y, Zhang J 2016 Chin. Phys. B 25 020304 Google Scholar
[45]	Huang Z X, Motes K R, Anisimov P M, Dowling J P, Berry D W 2017 Phys. Rev. A 95 053837 Google Scholar
[46]	Fan H Y, Ruan T N 1983 Commun. Theor. Phys. 2 1563 Google Scholar
[47]	Hu L Y, Fan H Y 2008 J. Opt. Soc. Am. B 25 1955 Google Scholar
[48]	Oh C, Lee S Y, Nha H, Jeong H 2017 Phys. Rev. A 96 062304 Google Scholar
[49]	Steuernagel O, Scheel S 2004 J. Phys. B: Quantum Semicalss Opt. 6 S66 Google Scholar

[1]	丁永今, 曹士英, 林百科, 王强, 韩羿, 方占军. 基于电光晶体马赫-曾德干涉仪的载波包络偏移频率调节方法. , 2022, 71(14): 144203. doi: 10.7498/aps.71.20220147
[2]	王坤, 段高燕, 郎佩琳, 赵玉芳, 刘尖斌, 宋钢. 基于银纳米链的马赫-曾德干涉仪结构的生物传感器. , 2022, 71(1): 017301. doi: 10.7498/aps.71.20211420
[3]	王坤, 段高燕, 郎佩琳, 赵玉芳, 刘尖斌, 宋钢. 基于银纳米链的马赫-曾德干涉仪结构的生物传感器. , 2021, (): . doi: 10.7498/aps.70.20211420
[4]	冯海冉, 李鹏, 岳现房. 初态对线型分子体系量子速度极限度量的影响. , 2019, 68(5): 050201. doi: 10.7498/aps.68.20181942
[5]	鹿博, 韩成银, 庄敏, 柯勇贯, 黄嘉豪, 李朝红. 超冷原子系综的非高斯纠缠态与精密测量. , 2019, 68(4): 040306. doi: 10.7498/aps.68.20190147
[6]	王灿灿. 量子纠缠与宇宙学弗里德曼方程. , 2018, 67(17): 179501. doi: 10.7498/aps.67.20180813
[7]	成健, 冯晋霞, 李渊骥, 张宽收. 基于量子增强型光纤马赫-曾德尔干涉仪的低频信号测量. , 2018, 67(24): 244202. doi: 10.7498/aps.67.20181335
[8]	贾玥, 陈肖含, 张好, 张临杰, 肖连团, 贾锁堂. Rydberg原子的电磁诱导透明光谱的噪声转移特性. , 2018, 67(21): 213201. doi: 10.7498/aps.67.20181168
[9]	赵军龙, 张译丹, 杨名. 噪声对一种三粒子量子探针态的影响. , 2018, 67(14): 140302. doi: 10.7498/aps.67.20180040
[10]	李诗宇, 田剑锋, 杨晨, 左冠华, 张玉驰, 张天才. 探测器对量子增强马赫-曾德尔干涉仪相位测量灵敏度的影响. , 2018, 67(23): 234202. doi: 10.7498/aps.67.20181193
[11]	王云新, 李虹历, 王大勇, 李静楠, 钟欣, 周涛, 杨登才, 戎路. 基于双平行马赫-曾德尔调制器的大动态范围微波光子下变频方法. , 2017, 66(9): 098401. doi: 10.7498/aps.66.098401
[12]	闫子华, 孙恒信, 蔡春晓, 马龙, 刘奎, 郜江瑞. 基于低频压缩光的声频信号测量. , 2017, 66(11): 114205. doi: 10.7498/aps.66.114205
[13]	孙新梅, 查新未, 祁建霞, 兰倩. 基于非最大纠缠的五粒子Cluster态的高效量子态共享方案. , 2013, 62(23): 230302. doi: 10.7498/aps.62.230302
[14]	靳爱军, 王泽锋, 侯静, 郭良, 姜宗福, 肖瑞. 复自相干度度量超连续谱相干性. , 2012, 61(15): 154201. doi: 10.7498/aps.61.154201
[15]	王昌辉, 赵国华, 常胜江. 基于光子晶体马赫-曾德尔干涉仪的太赫兹开关及强度调制器. , 2012, 61(15): 157805. doi: 10.7498/aps.61.157805
[16]	韩奎, 王子煜, 沈晓鹏, 吴琼华, 童星, 唐刚, 吴玉喜. 基于光子晶体自准直和带隙效应的马赫-曾德尔干涉仪设计. , 2011, 60(4): 044212. doi: 10.7498/aps.60.044212
[17]	刘玉玲, 满忠晓, 夏云杰. 用非最大纠缠信道对任意二粒子纠缠态的量子秘密分享. , 2008, 57(5): 2680-2686. doi: 10.7498/aps.57.2680
[18]	商娅娜, 王东, 闫智辉, 王文哲, 贾晓军, 彭堃墀. 利用非平衡光纤Mach-Zehnder干涉仪探测频率非简并纠缠态光场. , 2008, 57(6): 3514-3518. doi: 10.7498/aps.57.3514
[19]	汪大林, 孙军强, 王健. 基于周期极化反转铌酸锂光波导高速非归零码到归零码的转换. , 2008, 57(1): 252-259. doi: 10.7498/aps.57.252
[20]	王琛, 王伟, 孙今人, 方智恒, 吴江, 傅思祖, 马伟新, 顾援, 王世绩, 张国平, 郑无敌, 张覃鑫, 彭惠民, 邵平, 易葵, 林尊琪, 王占山, 王洪昌, 周斌, 陈玲燕. 利用x射线激光干涉诊断等离子体电子密度. , 2005, 54(1): 202-205. doi: 10.7498/aps.54.202

计量

文章访问数: 8214
PDF下载量: 185
被引次数: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

搜索

留言板

光子增加双模压缩真空态在马赫-曾德尔干涉仪相位测量中的应用