高速跟驰交通流动力学模型研究

陈永; 张薇

doi:10.7498/aps.69.20191251

摘要

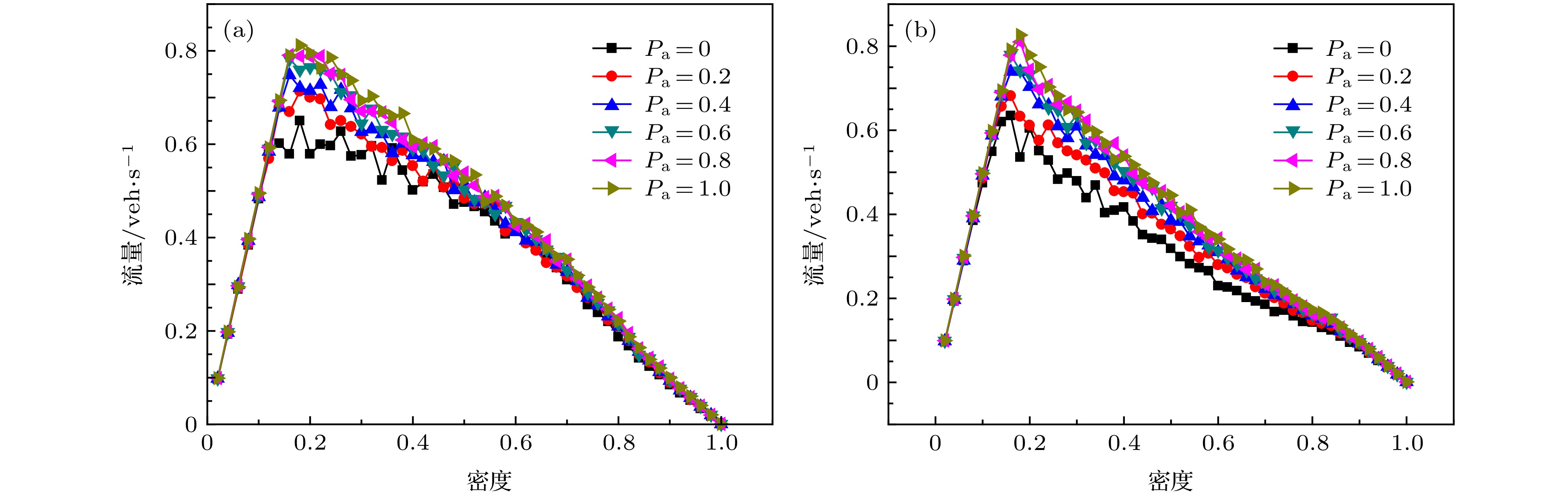
为研究道路交通中的高速跟驰物理现象, 针对高速跟驰车辆特点, 综合考虑了驾驶员换道决策行为以及随机慢化等因素, 结合前景理论等方法, 提出了一种用于模拟道路交通流中高速跟驰物理现象的动力学模型(简称HCCA模型). 通过计算机数值模拟, 研究了高速跟驰交通流物理现象演化机理及高速跟驰特性. 结果表明: 与对称的双车道元胞自动机动力学模型相比, 本文建立的HCCA动力学模型能够再现道路高速跟驰物理现象, 并得到了道路小间距高速跟驰率超过7%的结果与实测结果相符合, 最后模拟得到了丰富的交通物理现象, 再现了自由流、同步流及运动阻塞等复杂交通物理现象.

关键词:

Abstract

For the physical phenomenon of high-speed car following in the road traffic flow, all the vehicles with small spacing do not run at low speed. The speeds of the vehicles are significantly higher than those they normally should be when they are in this vehicles’s density. There are more than 7% of high-speed following vehicles in the measured data. At present, the traditional traffic flow model cannot simulate the phenomenon of high-speed car following, so a new nonlinear dynamic mathematical model is needed to describe and analyze the physical phenomenon. In order to study the physical phenomenon of high-speed car following in road traffic, a traffic flow dynamics model for simulating the phenomena is proposed, which combines with the prospect theory and takes into account some factors such as driver’s decision-making behavior and randomization. It is called HCCA (high-speed car following celluar automat) model. In the model, the prospect theory is used to analyze the driver’s lane changing behavior under the uncertain conditions. Combined with the characteristics of the radical driver, the dynamic prediction speed is considered for the front car followed by the radical driver, and the HCCA dynamics rules of high-speed following traffic flow mechanics are defined. By the computer numerical simulation, the evolution mechanism and the characteristics of high-speed car-following flow are studied. The results show that compared with the symmetric two-lane cellular automata (STCA) dynamic model, the HCCA dynamic model established in this paper can simulate abundant traffic physical phenomena, and reproduce complex traffic phenomena such as free flow, synchronous flow and wide-range motion congestion. Finally, the phenomenon of high-speed car following is simulated and the results of high-speed car following rate over 7% with small spacing are in good agreement with the measured results. It overcomes the shortage that traditional STCA model cannot simulate the synchronous flow. It is found that the larger the proportion of radical drivers, the larger the high-speed car following rate and traffic flow with small spacing are under the same road density. The high-speed car following traffic flow mechanics model proposed in this paper has a certain reference significance and practical value for analyzing the physical phenomenon of high-speed car following and enriching the traffic flow theory.

Keywords:

作者及机构信息

陈永¹,
张薇²

1.
兰州交通大学电子与信息工程学院, 兰州　730070

2.
兰州交通大学交通运输学院, 兰州　730070

通信作者: 陈永, edukeylab@126.com

基金项目: 国家级-国家自然科学基金面上项目(61841303，61963023)

Authors and contacts

Chen Yong¹,
Zhang Wei²

1.
School of Electronic and Information Engineering, Lanzhou Jiaotong University, Lanzhou 730070, China

2.
School of Traffic and Transportation, Lanzhou Jiaotong University, Lanzhou 730070, China

Corresponding author: Chen Yong, edukeylab@126.com

文章全文 : translate this paragraph

参考文献

[1]	Wolfram S 1983 Rev. Mod. Phys. 55 601 Google Scholar
[2]	陈永, 贺红, 张薇, 周宁 2018 力学学报 50 1219 Google Scholar Chen Y, He H, Zhang W, Zhou N 2018 Chin. J. Theor. Appl. Mech. 50 1219 Google Scholar
[3]	方辉, 薛桦, 汤倩玉, 张庆宇, 潘诗琰, 朱鸣芳 2019 68 048102 Google Scholar Fang H, Xue H, Tang Q Y, Zhang Q Y, Pan S Y, Zhu M F 2019 Acta Phys. Sin. 68 048102 Google Scholar
[4]	Souvik R 2019 Physica A 515 600 Google Scholar
[5]	金辉, 郭仁拥 2019 68 020501 Google Scholar Jin H, Guo R Y 2019 Acta Phys. Sin. 68 020501 Google Scholar
[6]	Li Y, Chen M, Dou Z, Zheng X P, Cheng Y 2019 Physica A 526 120752 Google Scholar
[7]	候磊, 刘建国, 潘雪, 郭强, 汪秉宏 2014 63 178902 Google Scholar Hou L, Liu J G, Pan X, Guo Q, Wang B H 2014 Acta Phys. Sin. 63 178902 Google Scholar
[8]	Mu R, Toshiyuki Y 2019 Transp. Res. A 124 217
[9]	Xue S Q, Jia B, Jiang R, Li X G, Shan J J 2017 Physica A 487 164 Google Scholar
[10]	Pang M B, Ren B N 2017 Chin. Phys. B 26 108901 Google Scholar
[11]	Sun Y Q, Ge H X, Cheng R J 2019 Physica A 527 121426 Google Scholar
[12]	张稷, 李艳芳, 董力耕 2018 67 240503 Google Scholar Zhang J, Wei Y F, Dong L G 2018 Acta Phys. Sin. 67 240503 Google Scholar
[13]	Echab H, Ez-Zahraouy H 2017 Int. J. Mod. Phys. C 28 1750134 Google Scholar
[14]	Nagel K, Schreckenberg M 1992 J. Phys. I France 2 2221
[15]	Chowdhury D, Wolf D E, Schreckenberg M 1997 Physica A 235 417 Google Scholar
[16]	彭莉娟, 康瑞 2009 58 830 Google Scholar Peng L J, Kang R 2009 Acta Phys. Sin. 58 830 Google Scholar
[17]	董长印, 王昊, 王炜, 李烨, 华雪东 2018 67 144501 Google Scholar Dong C Y, Wang H, Wang W, Li Y, Hua X D 2018 Acta Phys. Sin. 67 144501 Google Scholar
[18]	Deng J H, Feng H H 2019 Physica A 529 121545 Google Scholar
[19]	郑亮, 马寿峰, 贾宁 2010 59 4490 Google Scholar Zheng L, Ma S F, Jia N 2010 Acta Phys. Sin. 59 4490 Google Scholar
[20]	张柠溪, 祝会兵, 林亨, 黄梦圆 2015 64 024501 Google Scholar Zheng N X, Zhu H B, Lin H, Huang M Y 2015 Acta Phys. Sin. 64 024501 Google Scholar
[21]	Krzysztof M 2018 J. Comput. Sci. 28 32 Google Scholar
[22]	Damian N, Dailisan, May T 2019 Physica A 521 715 Google Scholar
[23]	Zhao H T, Liu X R, Chen X X, Lu J C 2018 Physica A 494 40 Google Scholar
[24]	吴胜春, 郑贤清, 郭明昊, 吴正 2011 中国科学: 物理学力学天文学 41 791 Google Scholar Wu S C, Zheng X Q, Guo M M, Wu Z 2011 Sci. Phys. Mech. Astron. 41 791 Google Scholar
[25]	Tversky A, Kahneman D 1992 J. Risk Uncertainty 5 297 Google Scholar
[26]	Kahneman D 2003 Am. Econ. Rev. 93 1449 Google Scholar
[27]	郑贤清 2011 博士学位论文 (上海: 复旦大学) Zheng X Q 2011 Ph. D. Dissertation (Shanghai: Fudan University) (in Chinese)

施引文献

图 1 换道车辆的空间关系示意图

Fig. 1. Diagram of spatial relations of lane-changing vehicles.

下载: 全尺寸图片幻灯片

图 2 不同激进型驾驶员比例下高速跟驰率　(a) 左车道高速跟驰率; (b) 右车道高速跟驰率

Fig. 2. Rate of high speed car-following and denisty relationship diagram under the different probability of aggressive drivers: (a) Rate of high speed car-following in left lane; (b) rate of high speed car-following in right lane.

下载: 全尺寸图片幻灯片

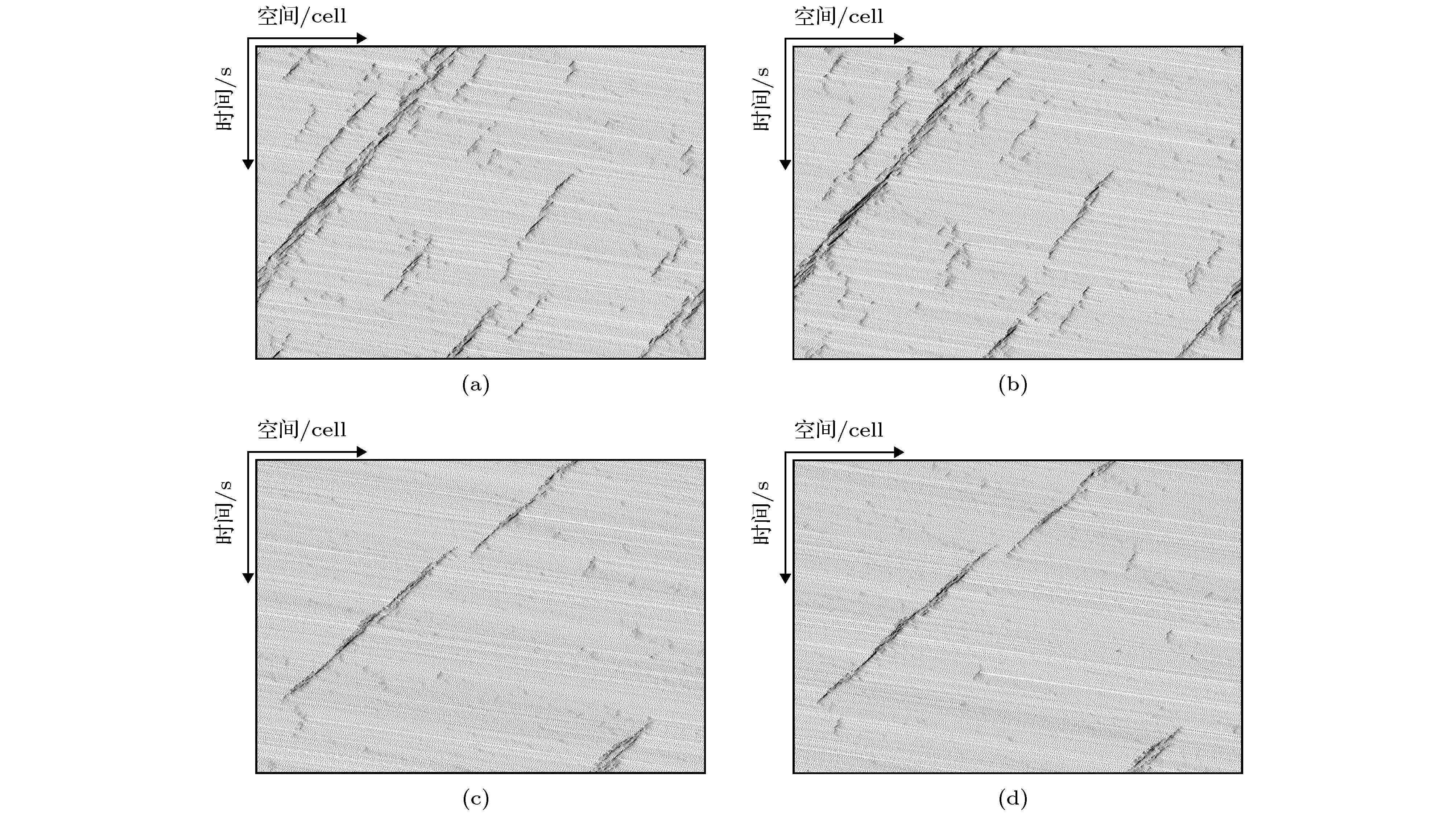
图 3 ρ₁ = 0.2, ρ₂ = 0.1, 不同车道时空图　(a) STCA演化左车道; (b) STCA演化右车道; (c) HCCA演化左车道; (d) HCCA演化右车道

Fig. 3. Space-time diagrams of different lanes under the condition of ρ₁ = 0.2 and ρ₂ = 0.1: (a) Left lane evolution with STCA rules; (b) right lane evolution with STCA rules; (c) left lane evolution with HCCA rules; (d) right lane evolution with HCCA rules.

下载: 全尺寸图片幻灯片

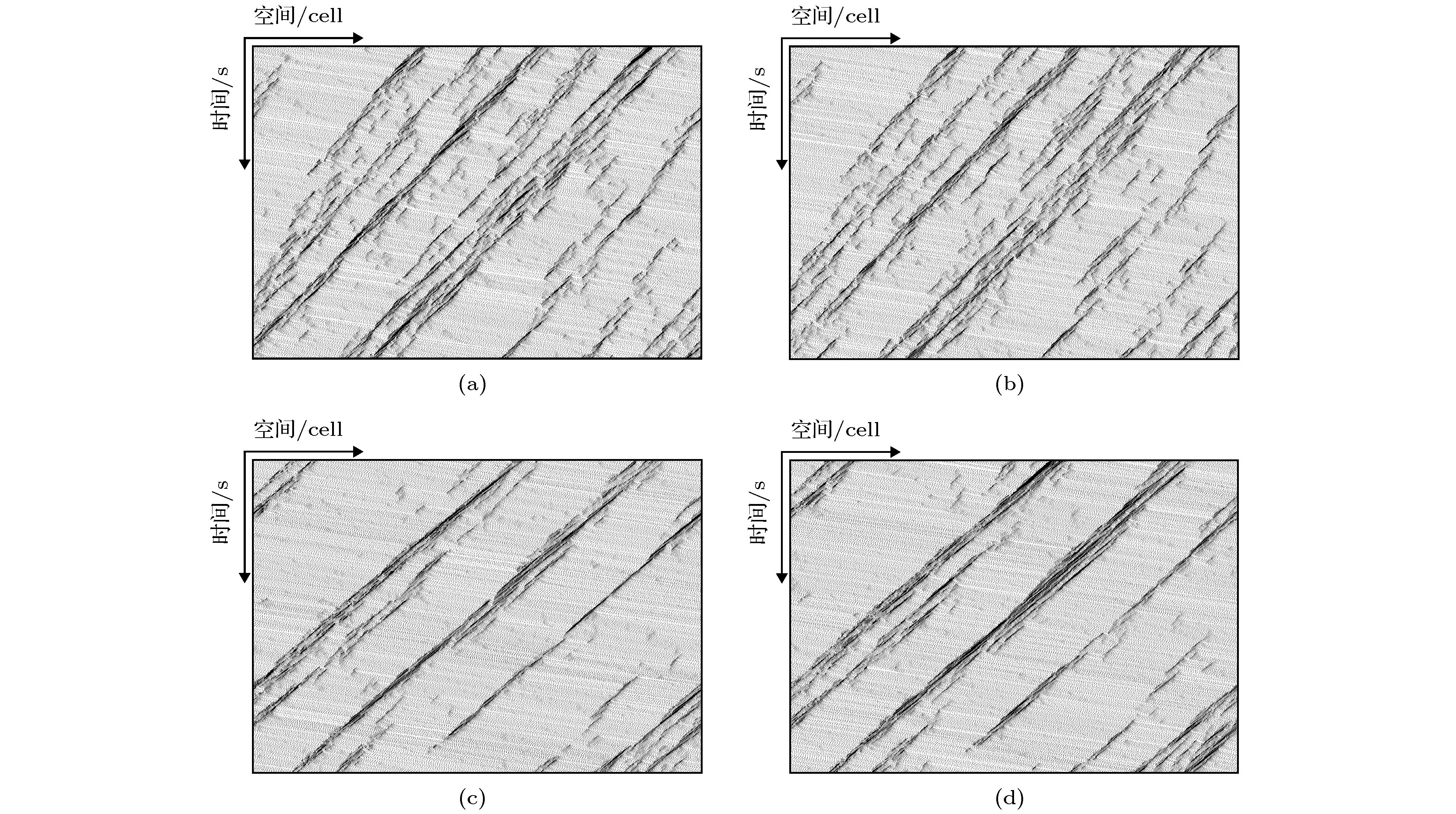
图 5 ρ₁ = 0.3, ρ₂ = 0.2, 不同车道时空图　(a) STCA演化左车道; (b) STCA演化右车道; (c) HCCA演化左车道; (d) HCCA演化右车道

Fig. 5. Space-time diagrams of different lanes under the condition of ρ₁ = 0.3 and ρ₂ = 0.2: (a) Left lane evolution with STCA rules; (b) right lane evolution with STCA rules; (c) left lane evolution with HCCA rules; (d) right lane evolution with HCCA rules.

下载: 全尺寸图片幻灯片

图 4 ρ₁ = 0.3, ρ₂ = 0.1, 不同车道时空图　(a) STCA演化左车道; (b) STCA演化右车道; (c) HCCA演化左车道; (d) HCCA演化右车道

Fig. 4. Space-time diagrams of different lanes under the condition of ρ₁ = 0.3 and ρ₂ = 0.1: (a) Left lane evolution with STCA rules; (b) right lane evolution with STCA rules; (c) left lane evolution with HCCA rules; (d) right lane evolution with HCCA rules.

下载: 全尺寸图片幻灯片

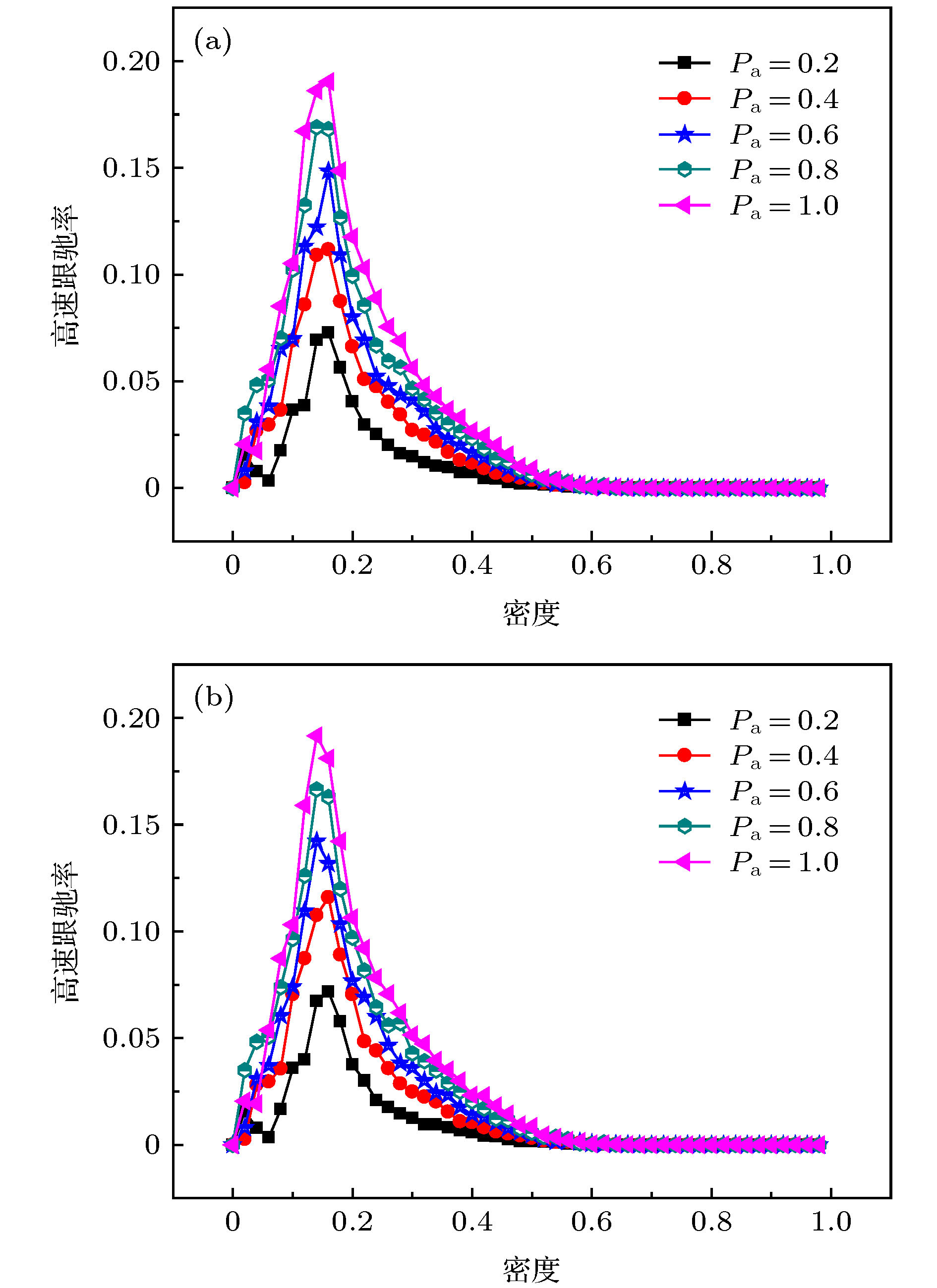
图 6 ρ₁ = 0.08, ρ₂ = 0.08, 速度分布图　(a) STCA演化左车道; (b) STCA演化右车道; (c) HCCA演化左车道; (d) HCCA演化右车道

Fig. 6. Velocity distribution diagram of different lanes under the condition of ρ₁ = 0.08 and ρ₂ = 0.08: (a) Left lane evolution with STCA rules; (b) right lane evolution with STCA rules; (c) left lane evolution with HCCA rules; (d) right lane evolution with HCCA rules.

下载: 全尺寸图片幻灯片

图 7 ρ₁ = 0.14, ρ₂ = 0.14, 速度分布图　(a) STCA演化左车道; (b) STCA演化右车道; (c) HCCA演化左车道; (d) HCCA演化右车道

Fig. 7. Velocity distribution diagram of different lanes under the condition of ρ₁ = 0.14 and ρ₂ = 0.14: (a) Left lane evolution with STCA rules; (b) right lane evolution with STCA rules; (c) left lane evolution with HCCA rules; (d) right lane evolution with HCCA rules.

下载: 全尺寸图片幻灯片

图 8 不同类型驾驶员混合比下密度与流量关系图　(a)左车道密度流量关系; (b)右车道密度流量关系图

Fig. 8. Density and flow relationship diagram under the mixing probability of different type drivers: (a) Density and flow relationship in left lane; (b) density and flow relationship in right lane.

下载: 全尺寸图片幻灯片

表 1 不同速度密度关系计算的误差比较

Table 1. Comparison of calculation errors of different velocity-denisty models.

速度密度计算模型	高峰时段误差	普通时段误差	雪天误差
Greenshields模型	–0.24	–0.11	–0.28
Greenberg模型	0.51	0.56	0.29
Underwood模型	0.26	0.49	0.22
本文HCCA模型	–0.02	0.03	–0.07

下载: 导出CSV

map

[1]	Wolfram S 1983 Rev. Mod. Phys. 55 601 Google Scholar
[2]	陈永, 贺红, 张薇, 周宁 2018 力学学报 50 1219 Google Scholar Chen Y, He H, Zhang W, Zhou N 2018 Chin. J. Theor. Appl. Mech. 50 1219 Google Scholar
[3]	方辉, 薛桦, 汤倩玉, 张庆宇, 潘诗琰, 朱鸣芳 2019 68 048102 Google Scholar Fang H, Xue H, Tang Q Y, Zhang Q Y, Pan S Y, Zhu M F 2019 Acta Phys. Sin. 68 048102 Google Scholar
[4]	Souvik R 2019 Physica A 515 600 Google Scholar
[5]	金辉, 郭仁拥 2019 68 020501 Google Scholar Jin H, Guo R Y 2019 Acta Phys. Sin. 68 020501 Google Scholar
[6]	Li Y, Chen M, Dou Z, Zheng X P, Cheng Y 2019 Physica A 526 120752 Google Scholar
[7]	候磊, 刘建国, 潘雪, 郭强, 汪秉宏 2014 63 178902 Google Scholar Hou L, Liu J G, Pan X, Guo Q, Wang B H 2014 Acta Phys. Sin. 63 178902 Google Scholar
[8]	Mu R, Toshiyuki Y 2019 Transp. Res. A 124 217
[9]	Xue S Q, Jia B, Jiang R, Li X G, Shan J J 2017 Physica A 487 164 Google Scholar
[10]	Pang M B, Ren B N 2017 Chin. Phys. B 26 108901 Google Scholar
[11]	Sun Y Q, Ge H X, Cheng R J 2019 Physica A 527 121426 Google Scholar
[12]	张稷, 李艳芳, 董力耕 2018 67 240503 Google Scholar Zhang J, Wei Y F, Dong L G 2018 Acta Phys. Sin. 67 240503 Google Scholar
[13]	Echab H, Ez-Zahraouy H 2017 Int. J. Mod. Phys. C 28 1750134 Google Scholar
[14]	Nagel K, Schreckenberg M 1992 J. Phys. I France 2 2221
[15]	Chowdhury D, Wolf D E, Schreckenberg M 1997 Physica A 235 417 Google Scholar
[16]	彭莉娟, 康瑞 2009 58 830 Google Scholar Peng L J, Kang R 2009 Acta Phys. Sin. 58 830 Google Scholar
[17]	董长印, 王昊, 王炜, 李烨, 华雪东 2018 67 144501 Google Scholar Dong C Y, Wang H, Wang W, Li Y, Hua X D 2018 Acta Phys. Sin. 67 144501 Google Scholar
[18]	Deng J H, Feng H H 2019 Physica A 529 121545 Google Scholar
[19]	郑亮, 马寿峰, 贾宁 2010 59 4490 Google Scholar Zheng L, Ma S F, Jia N 2010 Acta Phys. Sin. 59 4490 Google Scholar
[20]	张柠溪, 祝会兵, 林亨, 黄梦圆 2015 64 024501 Google Scholar Zheng N X, Zhu H B, Lin H, Huang M Y 2015 Acta Phys. Sin. 64 024501 Google Scholar
[21]	Krzysztof M 2018 J. Comput. Sci. 28 32 Google Scholar
[22]	Damian N, Dailisan, May T 2019 Physica A 521 715 Google Scholar
[23]	Zhao H T, Liu X R, Chen X X, Lu J C 2018 Physica A 494 40 Google Scholar
[24]	吴胜春, 郑贤清, 郭明昊, 吴正 2011 中国科学: 物理学力学天文学 41 791 Google Scholar Wu S C, Zheng X Q, Guo M M, Wu Z 2011 Sci. Phys. Mech. Astron. 41 791 Google Scholar
[25]	Tversky A, Kahneman D 1992 J. Risk Uncertainty 5 297 Google Scholar
[26]	Kahneman D 2003 Am. Econ. Rev. 93 1449 Google Scholar
[27]	郑贤清 2011 博士学位论文 (上海: 复旦大学) Zheng X Q 2011 Ph. D. Dissertation (Shanghai: Fudan University) (in Chinese)

[1]	梁经韵, 张莉莉, 栾悉道, 郭金林, 老松杨, 谢毓湘. 多路段元胞自动机交通流模型. , 2017, 66(19): 194501. doi: 10.7498/aps.66.194501
[2]	张柠溪, 祝会兵, 林亨, 黄梦圆. 考虑动态车间距的一维元胞自动机交通流模型. , 2015, 64(2): 024501. doi: 10.7498/aps.64.024501
[3]	赵韩涛, 毛宏燕. 有应急车辆影响的多车道交通流元胞自动机模型. , 2013, 62(6): 060501. doi: 10.7498/aps.62.060501
[4]	敬明, 邓卫, 王昊, 季彦婕. 基于跟车行为的双车道交通流元胞自动机模型. , 2012, 61(24): 244502. doi: 10.7498/aps.61.244502
[5]	钱勇生, 曾俊伟, 杜加伟, 刘宇斐, 王敏, 魏军. 考虑意外事件对交通流影响的元胞自动机交通流模型. , 2011, 60(6): 060505. doi: 10.7498/aps.60.060505
[6]	温坚, 田欢欢, 康三军, 薛郁. 混合交通流元胞自动机FI模型的能耗研究. , 2010, 59(11): 7693-7700. doi: 10.7498/aps.59.7693
[7]	梅超群, 黄海军, 唐铁桥. 城市快速路系统的元胞自动机模型与分析. , 2009, 58(5): 3014-3021. doi: 10.7498/aps.58.3014
[8]	康瑞, 彭莉娟, 杨凯. 考虑驾驶方式改变的一维元胞自动机交通流模型. , 2009, 58(7): 4514-4522. doi: 10.7498/aps.58.4514
[9]	田欢欢, 薛郁, 康三军, 梁玉娟. 元胞自动机混合交通流模型的能耗研究. , 2009, 58(7): 4506-4513. doi: 10.7498/aps.58.4506
[10]	彭莉娟, 康瑞. 考虑驾驶员特性的一维元胞自动机交通流模型. , 2009, 58(2): 830-835. doi: 10.7498/aps.58.830
[11]	梅超群, 黄海军, 唐铁桥. 高速公路入匝控制的一个元胞自动机模型. , 2008, 57(8): 4786-4793. doi: 10.7498/aps.57.4786
[12]	吴可非, 孔令江, 刘慕仁. 双车道元胞自动机NS和WWH交通流混合模型的研究. , 2006, 55(12): 6275-6280. doi: 10.7498/aps.55.6275
[13]	周华亮, 高自友, 李克平. 准移动闭塞系统的元胞自动机模型及列车延迟传播规律的研究. , 2006, 55(4): 1706-1710. doi: 10.7498/aps.55.1706
[14]	郭四玲, 韦艳芳, 薛郁. 元胞自动机交通流模型的相变特性研究. , 2006, 55(7): 3336-3342. doi: 10.7498/aps.55.3336
[15]	花伟, 林柏梁. 考虑行车状态的一维元胞自动机交通流模型. , 2005, 54(6): 2595-2599. doi: 10.7498/aps.54.2595
[16]	牟勇飚, 钟诚文. 基于安全驾驶的元胞自动机交通流模型. , 2005, 54(12): 5597-5601. doi: 10.7498/aps.54.5597
[17]	谭惠丽, 刘慕仁, 孔令江. 开放边界条件下改进的Nagel-Schreckenberg交通流模型的研究. , 2002, 51(12): 2713-2718. doi: 10.7498/aps.51.2713
[18]	薛郁, 董力耘, 戴世强. 一种改进的一维元胞自动机交通流模型及减速概率的影响. , 2001, 50(3): 445-449. doi: 10.7498/aps.50.445
[19]	吕晓阳, 孔令江, 刘慕仁. 一维元胞自动机随机交通流模型的宏观方程分析. , 2001, 50(7): 1255-1259. doi: 10.7498/aps.50.1255
[20]	汪秉宏, 王雷, 许伯铭, 胡斑比. 高速车随机延迟逐步加速交通流元胞自动机模型. , 2000, 49(10): 1926-1932. doi: 10.7498/aps.49.1926

计量

文章访问数: 8292
PDF下载量: 176
被引次数: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

搜索

留言板